Tuning and Amplifying the Interactions in Superconducting Quantum Circuits
with Subradiant Qubits
Abstract
We propose a tunable coupler consisting of fixed-frequency qubits, which can tune and even amplify the effective interaction between two superconducting quantum circuits. The tuning range of the interaction is proportional to , with a minimum value of zero and a maximum that can exceed the physical coupling rates between the coupler and the circuits. The effective coupling rate is determined by the collective magnetic quantum number of the qubit ensemble, which takes only discrete values and is free from collective decay and decoherence. Using single-photon -pulses, the coupling rate can be switched between arbitrary choices of the initial and final values within the dynamic range in a single step without going through intermediate values. A cascade of the couplers for amplifying small interactions or weak signals is also discussed. These results should not only stimulate interest in exploring the collective effects in quantum information processing, but also enable development of applications in tuning and amplifying the interactions in a general cavity-QED system.
I Introduction
Tuning the coupling rate between two fixed-frequency superconducting quantum circuits, instead of tuning the characteristic frequency of each individual part, has attracted an increasing interest in recent years as a promising way to scalable quantum computing [1, 2, 3, 4, 5, 6, 7, 8, 9]. The conventional method to realize such a tunable coupler is to place a Josephson junction, or SQUID, as a mediating element between the two circuits. From a circuit point of view, the junction can be regarded as a tunable positive inductance which, together with other circuit elements such as capacitors or mutual inductors, can be used to form a tunable coupler [10, 11, 12, 13, 14]. Alternatively, one may also consider the junction as an off-resonant qubit which mediates the exchange of virtual photons between the two circuits [15, 16, 17, 18, 19, 20, 21, 22]. A tunable coupling rate is achieved by adjusting the frequency detuning between the coupler qubit and the coupled circuits, while maintaining the former unexcited. This single-junction coupler has attracted a huge success in recent experiments. However, the dynamic range of the effective component-component coupling rate is limited to the second order of the physical dispersive coupling rate. Moreover, the coupler can be very sensitive to experimental imperfections, including both systematic and stochastic errors in certain parameter regimes, because of the nonlinearity of the Josephson inductance.
Here, we propose to use different steady states of one or several Josephson junctions to tune the coupling rate between two superconducting quantum circuits. The coupler is modeled by an ensemble of homogeneous fixed-frequency and off-resonant qubits, also known as the Dicke model [23], and is thus called the D-coupler. Instead of tuning the frequencies of the coupler qubits, a tunable interaction is achieved by preparing the qubits in different quantum states, corresponding to different collective angular and magnetic quantum numbers [16, 18, 24]. The dynamic range is proportional to the number of coupler qubits, where the maximum coupling rate may even exceed the individual coupling rates in the system for large . On the other hand, the name, D-coupler, may also be interpreted as “decay- and decoherence-free” if we prepare the qubits in subradiant states [23]. It can also be understood as a digital coupler if we further restrict the subradiant states to be pairwise. In this case, the effective coupling rate takes only discrete values that are proportional to the total excitation number of the qubit ensemble [24]. Control of the coupling rate, between arbitrary initial and final choices within the dynamic range, can be realized in a single step with single-photon -pulses [25]. In the meanwhile, the coupling rate needs not to go through any intermediate values during the tuning process. These properties make the D-coupler an ideal device in superconducting quantum circuits, despite the potential technical difficulty in sample design and fabrication, and should motivate more applications of the collective effects in quantum information processing [26, 27, 28, 29, 30, 31, 32, 33, 34, 35, 36, 37].
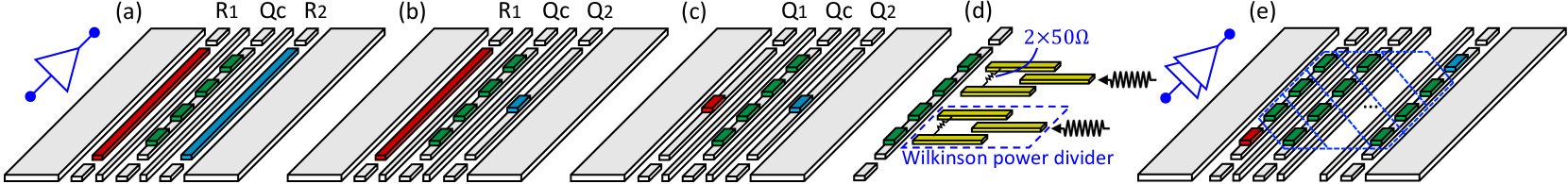
II The Dicke coupler
We consider a system where the interaction between two circuits, and , is mediated by an ensemble of qubits, . The system Hamiltonian may be written as () [38]
| (1) |
Here, , , and are the resonant frequency, raising, and lowering operators of circuit . Furthermore, and with are the characteristic frequency and the standard Pauli operators of the th coupler qubit, is the physical coupling rate between the two parts and , and takes all possible pairs in the ensemble. Depending on the commutation and anti-commutation relations between and , the above model can be used to describe a resonator-resonator (R-R) coupler (, ), a resonator-qubit (R-Q) coupler (, ), or a qubit-qubit (Q-Q) coupler (, ), as schematically shown in Fig. 1(a)-(c).
Assuming that the coupler qubits are largely detuned from the two circuits to be coupled, i.e., where and , a dispersive approximation (DA) may be applied to Eq. (1) that diagonalizes the coupler degree of freedom to the second order of . For simplicity and in the same spirit of the Dicke [23] or Tavis-Cummings model [39, 40], we further assume the qubits to be homogeneous and use the collective angular momentum operators, with , to describe the whole ensemble [41]. Then, the effective Hamiltonian can be written in a more compact form (see Appendix A for detailed derivation)
| (2) |
where , , , and is the commutation operator. One can verify that the angular quantum number, , which is determined by , is a conserved quantity. However, the magnetic quantum number, , which is an eigenvalue of , may not be a good quantum number because of the counter-rotating term . To be able to apply a rotating wave approximation (RWA), we further assume . Then, commutes with the Hamiltonian and can be replaced by its average during the time evolution if the system is initially prepared at its eigenstates. This results in an effective coupling rate between and ,
| (3) |
The above result indicates that an arbitrary coupling rate, which corresponds to , can be achieved by preparing the qubits in different collective states with , . One may also prepare the coupler in an arbitrary superposition or mixture of the eigenstates , which results into a quantum effective coupling strength between the two circuits and may leads to novel applications. However, we restrict the coupler to be eigenstates in the rest of this paper. For a sufficiently large , it is possible to achieve an effective coupling rate exceeding the physical rates between the coupler and either of the circuits, , while maintaining the requirements of DA and RWA. These properties show that a qubit ensemble described by the Dicke model can be used as a tunable coupler between two circuits and has a broad dynamic range proportional to the size of the ensemble.
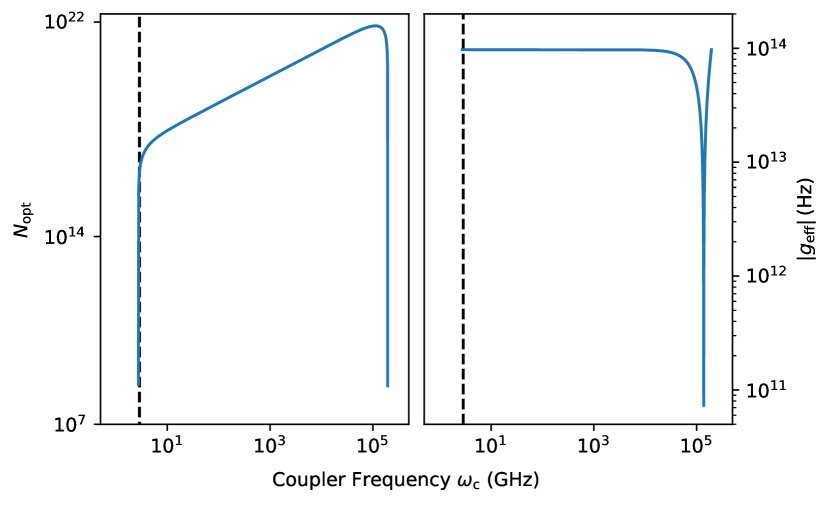
We note that the frequencies of the two circuits being coupled are not necessarily the same, and the above discussions apply to a general system which can be described by the Dicke model. One may consider using the D-coupler as a transducer which converts a microwave photon to an optical photon, and vice versa [15]. Here, the qubit ensemble may be made of a thin layer of spin- materials, for example, a huge and homogeneous array of NV-centers, at an intermediate frequency between microwave and optical frequencies. At the optimal condition
| (4) |
where the two physically off-resonant modes are effectively on resonance such that a single photon can be perfectly transferred between them for a time duration of for . This result may indicate a strong coupling rate between the microwave and optical modes for large and, correspondingly, a high conversion efficiency ideally approaching to the unity.
For a qualitative estimation, we choose the frequency of the microwave and optical modes to be and , respectively [42]. The coupler frequency is for an ensemble of NV centers, with a coupling strength of between the coupler and either of the two modes. We estimate that the optimal condition leads to an effective coupling rate at the scale of , which is in the deep strong coupling regime (Fig. 2 right). However, one should also note that the required number of homogeneous colored centers is approximately , which makes the optimal condition an extreme challenge to reach in real experiments (Fig. 2 left). In these regards, a practical implementation of the transducer requires a compromise between the effective coupling rate and the optimal condition. Alternatively, one may consider using two harmonic oscillators to form an effective spin- coupler, which is equivalent to an ensemble of spin- qubits [43, 44]. In either of the two cases, the collective effect of the Dicke model plays an important role in amplifying the weak interaction between the two off-resonant modes. A more detailed discussion of such a transducer relies on the specific parameters of the system and the compromises one may take, and is thus beyond the major interest of this study.
III The decay decoherence-free coupler
The D-coupler is free from collective decay and decoherence if one restricts the collective states to a subspace spanned by subradiant states [23]. By definition, a subradiant state is an eigenstate of the collective angular momentum, , with eigenvalue . In this regard, it is also an eigenstate of the collective lowering operator, , with eigenvalue zero. In an open environment, the dynamics of the system may be described by the master equation with the Born-Markov approximation [45]
| (5) |
where and are the energy relaxation and dephasing rates of the qubit ensemble, respectively, and is the Lindblad superoperator. One can verify that the subradiant states are also eigenstates of the Lindblad superoperator with eigenvalue zero. Thus, an arbitrary superposition of the subradiant states remains invariant during the dynamics of the open system with regard to collective decay and decoherence. Correspondingly, the effective coupling rate, which now takes only zero or negative eigenvalues of , is potentially stable in an open environment.
IV The digital coupler
Tuning of the coupling rate can be made ultrafast if we further restrict the subradiant states of the coupler to be pairwise, where each adjacent qubit pair takes only ground or singlet states, denoted as and [25]. However, the dynamic range of the effective coupling strength is not affected because the degeneracy of the subradiant states. This restriction also relaxes the original assumption of a collective decay and decoherence for all the coupler qubits into that for each qubit pair [24]. The coupling rate is controlled digitally by counting the total number of excitations in the qubit ensemble. Different from our earlier proposal for controlling the R-R coupling rate [24], we describe an alternative method that applies to a general system and results into an even faster switch of with beam splitters and single photon sources [25].
As schematically shown in Fig. 1(d), the control protocol may consist of a Wilkinson power divider which is modeled as a beam splitter routing an incident single microwave photon to two different paths simultaneously but with a -phase difference [46, 47, 48, 49]. At the end of each path, we couple one qubit to it and describe the photon-qubit interaction by a Jaynes-Cummings model [50]. Assuming that the two addressed qubits are initially in the ground state, , the single-photon drive increases the magnetic quantum number, , by one and results in a singlet state [25]. Alternatively, if the qubits are initially in the singlet state, they will end up in the ground state with [25]. For an ensemble of qubit pairs, one can apply single-photon -pulses to them in parallel, and switch the coupling from an arbitrary initial to the final value in a single step. Interestingly, the coupling rate needs not to go through any intermediate values during the tuning process if the skew among different beam splitters is negligibly small.
V Cascade of D-couplers
The D-coupler may also be cascaded in a chain with several layers of qubit ensembles, as schematically shown in Fig. 1(e). To simplify our discussion, we consider a system with layers of homogeneous qubits, where every two adjacent layers are coupled by an XY-type interaction
| (6) |
We focus on the case where all the qubits are initially prepared in the ground state, corresponding to the maximum effective coupling rate between and . For large , the collective qubits may be approximately described by a giant quantum oscillator with , , and [51, 52, 53, 54, 55].
Similar to the definition of magnons in a XY spin chain [56, 57, 58, 59], we diagonalize the coupler part of the Hamiltonian by introducing a collective operator
| (7) |
We are interested in the parameter regime where all the “magnons” are largely off-resonant to the two circuits, i.e., where is the coupling rate between and the magnon-like mode, , at frequency . Then, we apply a dispersive approximation (DA) to transform the component-coupler interactions into an effective interaction between and . The effective Hamiltonian is too complicated to display here (see Appendix B for detail), while the effective coupling rate is similar to the single-layer case
| (8) |
Surprisingly, is almost independent of the number of layers, . For a relatively small qubit number, , in each layer, i.e., , the value of scales quadratically with . With the increase of , the exponent of the power law increases monotonically until DA breaks down. In this case, one may enlarge the detuning frequency and add more layers and qubits at the same time to achieve a balance between the amplification rate and the validity of the effective model. However, we should note that an Ising-type interaction between two adjacent layers causes a correction in Eq. (8), as revealed in Appendix B, which may lead to a larger in certain parameter regimes. Besides, a varying-frequency design of the coupler qubits among different layers may also lead to a different expression of .
VI Simulation results
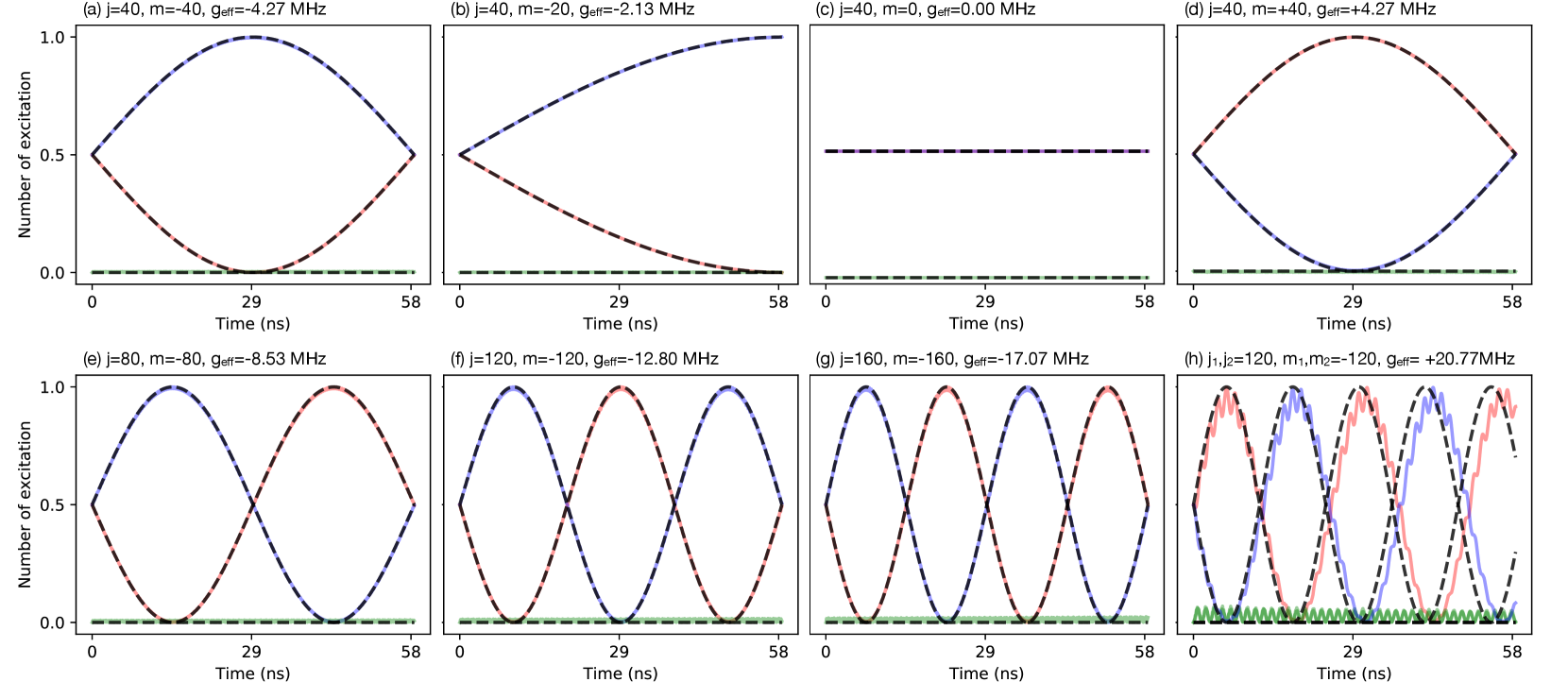
For illustration, we simulate the dynamics of a system which consists of two qubits and a D-coupler with qubits, as shown in Fig. 3(a)-(d). The two qubits are resonant at and largely detuned from the coupler qubits that are fixed at . The physical coupling rate, , is set as , which fulfills the requirements of both DA and RWA. The possibly small qubit crosstalk inside the coupler is neglected for simplisity. From (a) to (d), we control the effective coupling rate, from to , by engineering the qubit ensemble in different collective states. The coupling is completely turned off in (c), when the magnetic quantum number is zero. It also changes the sign comparing (a) and (d), which corresponds to the different signs of the magnetic quantum numbers.
Next, we keep all the coupler qubits in the ground state and increase the qubit number to , , and , as shown in Fig. 3(e)-(g). Correspondingly, the effective coupling rate increases linearly from through to . Here, the maximum value of is times larger than the physical coupling rate, , while the effective Hamiltonian, Eq. (2), still faithfully describes the dynamics of the system. This observation clearly demonstrates that an amplification of the interactions can be achieved in superconducting quantum circuits, which has not yet been reported in the literature. However, one may also note a small and periodic excitation of the coupler for increasingly large , which is mainly caused by the finite ratio of that limits the accuracy of the DA. These ripples can be effectively suppressed by increasing the detuning frequency and the qubit number by the same scaling factor, while keeping the effective coupling rate unchanged.
In Fig. 3(h), we simulate the system dynamics with a two-layer coupler, where each layer contains collective qubits. The coupling rate between two adjacent layers, , is assumed to be , while the other parameters are set identical to (a)-(g). Regardless of the noticeable ripples in the excitation numbers, we obtain an effective coupling rate of around , which is much larger than that for the single layer case shown in (f). The sign of the coupling rate is also different from (f). However, considering the total number of qubits in the two-layer coupler, adding more layers may not amplify the interactions as efficiently as increasing the number of qubits in a single layer, which is estimated to be for the same number of qubits. Moreover, the amplification rate may even decrease when adding an addition layer in certain scenarios. For example, we obtain an effective coupling rate of for two layers of qubits, while it is for a single layer of qubits, as shown in (e).
VII Conclusions outlook
We have proposed a so-called D-coupler based on the Dicke model, and showed that it can be used to tune and amplify the effective interactions between two general circuits. The effective coupling rate is controlled by the collective magnetic quantum number of the qubit ensemble, and it is free from collective decay and decoherence when the qubits are prepared in the subradiant states. The tuning procedure, from any initial to final values, is achieved in a single step by applying single-photon -pulses, without going through any intermediate values. The dynamic range scales linearly with the number of qubits, where the maximum can be made larger than the physical coupling rates between the coupler and the circuits.
We have also discussed a multi-layer design of the D-coupler. The circuit configuration is reminiscent to a traveling wave parametric amplifier (TWPA), which may be seen as a series configuration of the coupler qubits. In this perspective, amplification from the input to output fields may happen in the presence of a parametric driving field, when the effective coupling rate between the end qubits exceeds a threshold relating to their external decay rates [60]. However, our result may indicate that a parallel configuration of the coupler qubits may be an alternative and more efficient way to achieve quantum limited amplification at the same number of junctions.
Despite the collective properties, one major difference between the D-coupler and most of the existing proposals is that the effective coupling rate is controlled by the quantum state of the coupler but not the detuning frequency. Hence, the coupler can be made of only one or two fixed-frequency qubits, which significantly simplifies the sample fabrication, and, most importantly, leads to a flux-free design of superconducting quantum circuits where the qubit properties can be optimized with regard to only one noise source, i.e., the charge noise. One may also consider engineering the coupler state in the steady states of a driven-dissipative setup, which relaxes the assumption of a collective reservoir and may result into a more robust implementation. An experimental realization of our protocol could offer significant advantages in designing superconducting quantum circuits for quantum information processing.
Acknowledgements
We thank David Castells-Graells and Cosimo C. Rusconi for fruitful discussions. We use the QUANTUM add-on for Mathematica [61, 62] for deriving the effective Hamiltonian, and the QuTiP package in Python [63] for simulation. We acknowledge support by the German Research Foundation via Germany’s Excellence Strategy (No. EXC-2111-390814868), the Elite Network of Bavaria through the program ExQM, and the European Union via the Quantum Flagship project QMiCS (No. 820505).
Appendix A Effective Hamiltonian with one layer
We consider a system, , where two general circuit components are coupled via a qubit ensemble
| (9) | ||||
| (10) |
Here, , , and are the resonant frequency, raising and lowering operators of the th system component . Furthermore, and with are the characteristic frequency and standard Pauli operators of the th qubit in the ensemble with a total qubit number of , is the coupling rate between the two components and , and takes all possible pairs in the ensemble. To transform the component-coupler interaction into an effective component-component interaction to the second order of , we apply the following unitary transformation to the original Hamiltonian [64]
| (11) |
On the one hand, we have . The transformation can be simplified as to the second-order accuracy of and . On the other hand, we have
| (12) | ||||
| (13) | ||||
| (14) | ||||
| (15) |
Here, we have omitted the summation symbols over and for the simplicity of notation, and indicate all the different numbers from and . In total, we obtain the effective Hamiltonian
| (16) |
For the cases with two resonators, one resonator and one qubit, and two qubits, respectively, we have the following effective Hamiltonians
| (17) | ||||
| (18) | ||||
| (19) |
If we further assume the homogeneity among the coupler qubits, we use the collective angular momentum operators to describe the whole qubit ensemble and arrive at a more compact form of the effective Hamiltonian. They are
| (20) | ||||
| (21) | ||||
| (22) |
Appendix B Effective Hamiltonian with multiple layers
We consider a system with layers of homogeneous qubits, where any two adjacent layers are coupled by an XY-type interaction
| (23) |
By assuming that , we introduce the following replacement of variables for large [51, 52, 53, 54, 55]
| (24) |
This gives
| (25) |
We note that the last term should be written as for an Ising-type interaction, described by , between two adjacent layers.
Similar to the definition of magnons in a XY-type spin chain [56, 57, 58, 59], we define
| (26) |
The Hamiltonian can be written as
| (27) | ||||
| (28) |
where , . For an Ising-type interaction, one may add in .
To derive the effective Hamiltonian, we apply the following unitary transformation
| (29) |
We obtain
| (30) | ||||
| (31) |
for the XY- and Ising-type interactions, respectively. The rest of the commutators are
| (32) | ||||
| (33) | ||||
| (34) | ||||
| (35) |
As before, we have omitted the summation symbol in the above formulae for the simplicity of notation. The component-coupler interaction can be eliminated to the second-order accuracy if
| (36) |
or
| (37) |
which gives the following effective Hamiltonian
| (38) |
Here, the underlined term exists only for an Ising-type interaction.
References
- Niskanen et al. [2007] A. O. Niskanen, K. Harrabi, F. Yoshihara, Y. Nakamura, S. Lloyd, and J. S. Tsai, Quantum coherent tunable coupling of superconducting qubits, Science 316, 723 (2007).
- Chow et al. [2011] J. M. Chow, A. D. Córcoles, J. M. Gambetta, C. Rigetti, B. R. Johnson, J. A. Smolin, J. R. Rozen, G. A. Keefe, M. B. Rothwell, M. B. Ketchen, and M. Steffen, Simple all-microwave entangling gate for fixed-frequency superconducting qubits, Phys. Rev. Lett. 107, 080502 (2011).
- McKay et al. [2016] D. C. McKay, S. Filipp, A. Mezzacapo, E. Magesan, J. M. Chow, and J. M. Gambetta, Universal gate for fixed-frequency qubits via a tunable bus, Phys. Rev. Applied 6, 064007 (2016).
- Caldwell et al. [2018] S. A. Caldwell, N. Didier, C. A. Ryan, E. A. Sete, A. Hudson, P. Karalekas, R. Manenti, M. P. da Silva, R. Sinclair, E. Acala, N. Alidoust, J. Angeles, A. Bestwick, M. Block, B. Bloom, A. Bradley, C. Bui, L. Capelluto, R. Chilcott, J. Cordova, G. Crossman, M. Curtis, S. Deshpande, T. E. Bouayadi, D. Girshovich, S. Hong, K. Kuang, M. Lenihan, T. Manning, A. Marchenkov, J. Marshall, R. Maydra, Y. Mohan, W. O’Brien, C. Osborn, J. Otterbach, A. Papageorge, J.-P. Paquette, M. Pelstring, A. Polloreno, G. Prawiroatmodjo, V. Rawat, M. Reagor, R. Renzas, N. Rubin, D. Russell, M. Rust, D. Scarabelli, M. Scheer, M. Selvanayagam, R. Smith, A. Staley, M. Suska, N. Tezak, D. C. Thompson, T.-W. To, M. Vahidpour, N. Vodrahalli, T. Whyland, K. Yadav, W. Zeng, and C. Rigetti, Parametrically activated entangling gates using transmon qubits, Phys. Rev. Applied 10, 034050 (2018).
- Arute et al. [2019] F. Arute, K. Arya, R. Babbush, D. Bacon, J. C. Bardin, R. Barends, R. Biswas, S. Boixo, F. G. S. L. Brandao, D. A. Buell, B. Burkett, Y. Chen, Z. Chen, B. Chiaro, R. Collins, W. Courtney, A. Dunsworth, E. Farhi, B. Foxen, A. Fowler, C. Gidney, M. Giustina, R. Graff, K. Guerin, S. Habegger, M. P. Harrigan, M. J. Hartmann, A. Ho, M. Hoffmann, T. Huang, T. S. Humble, S. V. Isakov, E. Jeffrey, Z. Jiang, D. Kafri, K. Kechedzhi, J. Kelly, P. V. Klimov, S. Knysh, A. Korotkov, F. Kostritsa, D. Landhuis, M. Lindmark, E. Lucero, D. Lyakh, S. Mandrà, J. R. McClean, M. McEwen, A. Megrant, X. Mi, K. Michielsen, M. Mohseni, J. Mutus, O. Naaman, M. Neeley, C. Neill, M. Y. Niu, E. Ostby, A. Petukhov, J. C. Platt, C. Quintana, E. G. Rieffel, P. Roushan, N. C. Rubin, D. Sank, K. J. Satzinger, V. Smelyanskiy, K. J. Sung, M. D. Trevithick, A. Vainsencher, B. Villalonga, T. White, Z. J. Yao, P. Yeh, A. Zalcman, H. Neven, and J. M. Martinis, Quantum supremacy using a programmable superconducting processor, Nature 574, 505 (2019).
- Foxen et al. [2020] B. Foxen, C. Neill, A. Dunsworth, P. Roushan, B. Chiaro, A. Megrant, J. Kelly, Z. Chen, K. Satzinger, R. Barends, F. Arute, K. Arya, R. Babbush, D. Bacon, J. C. Bardin, S. Boixo, D. Buell, B. Burkett, Y. Chen, R. Collins, E. Farhi, A. Fowler, C. Gidney, M. Giustina, R. Graff, M. Harrigan, T. Huang, S. V. Isakov, E. Jeffrey, Z. Jiang, D. Kafri, K. Kechedzhi, P. Klimov, A. Korotkov, F. Kostritsa, D. Landhuis, E. Lucero, J. McClean, M. McEwen, X. Mi, M. Mohseni, J. Y. Mutus, O. Naaman, M. Neeley, M. Niu, A. Petukhov, C. Quintana, N. Rubin, D. Sank, V. Smelyanskiy, A. Vainsencher, T. C. White, Z. Yao, P. Yeh, A. Zalcman, H. Neven, and J. M. Martinis (Google AI Quantum), Demonstrating a continuous set of two-qubit gates for near-term quantum algorithms, Phys. Rev. Lett. 125, 120504 (2020).
- Xu et al. [2020] Y. Xu, J. Chu, J. Yuan, J. Qiu, Y. Zhou, L. Zhang, X. Tan, Y. Yu, S. Liu, J. Li, F. Yan, and D. Yu, High-fidelity, high-scalability two-qubit gate scheme for superconducting qubits, Phys. Rev. Lett. 125, 240503 (2020).
- Bengtsson et al. [2020] A. Bengtsson, P. Vikstål, C. Warren, M. Svensson, X. Gu, A. F. Kockum, P. Krantz, C. Križan, D. Shiri, I.-M. Svensson, G. Tancredi, G. Johansson, P. Delsing, G. Ferrini, and J. Bylander, Improved success probability with greater circuit depth for the quantum approximate optimization algorithm, Phys. Rev. Applied 14, 034010 (2020).
- Collodo et al. [2020] M. C. Collodo, J. Herrmann, N. Lacroix, C. K. Andersen, A. Remm, S. Lazar, J.-C. Besse, T. Walter, A. Wallraff, and C. Eichler, Implementation of conditional phase gates based on tunable interactions, Phys. Rev. Lett. 125, 240502 (2020).
- Blais et al. [2003] A. Blais, A. M. van den Brink, and A. M. Zagoskin, Tunable coupling of superconducting qubits, Phys. Rev. Lett. 90, 127901 (2003).
- Gambetta et al. [2011] J. M. Gambetta, A. A. Houck, and A. Blais, Superconducting qubit with purcell protection and tunable coupling, Phys. Rev. Lett. 106, 030502 (2011).
- Bialczak et al. [2011] R. C. Bialczak, M. Ansmann, M. Hofheinz, M. Lenander, E. Lucero, M. Neeley, A. D. O’Connell, D. Sank, H. Wang, M. Weides, J. Wenner, T. Yamamoto, A. N. Cleland, and J. M. Martinis, Fast tunable coupler for superconducting qubits, Phys. Rev. Lett. 106, 060501 (2011).
- Chen et al. [2014] Y. Chen, C. Neill, P. Roushan, N. Leung, M. Fang, R. Barends, J. Kelly, B. Campbell, Z. Chen, B. Chiaro, A. Dunsworth, E. Jeffrey, A. Megrant, J. Y. Mutus, P. J. J. O’Malley, C. M. Quintana, D. Sank, A. Vainsencher, J. Wenner, T. C. White, M. R. Geller, A. N. Cleland, and J. M. Martinis, Qubit architecture with high coherence and fast tunable coupling, Phys. Rev. Lett. 113, 220502 (2014).
- Geller et al. [2015] M. R. Geller, E. Donate, Y. Chen, M. T. Fang, N. Leung, C. Neill, P. Roushan, and J. M. Martinis, Tunable coupler for superconducting xmon qubits: Perturbative nonlinear model, Phys. Rev. A 92, 012320 (2015).
- Sun et al. [2006] C. P. Sun, L. F. Wei, Y.-x. Liu, and F. Nori, Quantum transducers: Integrating transmission lines and nanomechanical resonators via charge qubits, Phys. Rev. A 73, 022318 (2006).
- Mariantoni et al. [2008] M. Mariantoni, F. Deppe, A. Marx, R. Gross, F. K. Wilhelm, and E. Solano, Two-resonator circuit quantum electrodynamics: A superconducting quantum switch, Phys. Rev. B 78, 104508 (2008).
- Srinivasan et al. [2011] S. J. Srinivasan, A. J. Hoffman, J. M. Gambetta, and A. A. Houck, Tunable coupling in circuit quantum electrodynamics using a superconducting charge qubit with a -shaped energy level diagram, Phys. Rev. Lett. 106, 083601 (2011).
- Baust et al. [2015] A. Baust, E. Hoffmann, M. Haeberlein, M. J. Schwarz, P. Eder, J. Goetz, F. Wulschner, E. Xie, L. Zhong, F. Quijandría, B. Peropadre, D. Zueco, J.-J. García Ripoll, E. Solano, K. Fedorov, E. P. Menzel, F. Deppe, A. Marx, and R. Gross, Tunable and switchable coupling between two superconducting resonators, Phys. Rev. B 91, 014515 (2015).
- Yan et al. [2018] F. Yan, P. Krantz, Y. Sung, M. Kjaergaard, D. L. Campbell, T. P. Orlando, S. Gustavsson, and W. D. Oliver, Tunable coupling scheme for implementing high-fidelity two-qubit gates, Phys. Rev. Applied 10, 054062 (2018).
- Mundada et al. [2019] P. Mundada, G. Zhang, T. Hazard, and A. Houck, Suppression of qubit crosstalk in a tunable coupling superconducting circuit, Phys. Rev. Applied 12, 054023 (2019).
- Li et al. [2020] X. Li, T. Cai, H. Yan, Z. Wang, X. Pan, Y. Ma, W. Cai, J. Han, Z. Hua, X. Han, Y. Wu, H. Zhang, H. Wang, Y. Song, L. Duan, and L. Sun, Tunable coupler for realizing a controlled-phase gate with dynamically decoupled regime in a superconducting circuit, Phys. Rev. Applied 14, 024070 (2020).
- Han et al. [2020] X. Y. Han, T. Q. Cai, X. G. Li, Y. K. Wu, Y. W. Ma, Y. L. Ma, J. H. Wang, H. Y. Zhang, Y. P. Song, and L. M. Duan, Error analysis in suppression of unwanted qubit interactions for a parametric gate in a tunable superconducting circuit, Phys. Rev. A 102, 022619 (2020).
- Dicke [1954] R. H. Dicke, Coherence in spontaneous radiation processes, Phys. Rev. 93, 99 (1954).
- Chen et al. [2018] Q.-M. Chen, Y.-x. Liu, L. Sun, and R.-B. Wu, Tuning the coupling between superconducting resonators with collective qubits, Phys. Rev. A 98, 042328 (2018).
- Scully [2015] M. O. Scully, Single photon subradiance: Quantum control of spontaneous emission and ultrafast readout, Phys. Rev. Lett. 115, 243602 (2015).
- Fink et al. [2009] J. M. Fink, R. Bianchetti, M. Baur, M. Göppl, L. Steffen, S. Filipp, P. J. Leek, A. Blais, and A. Wallraff, Dressed collective qubit states and the tavis-cummings model in circuit qed, Phys. Rev. Lett. 103, 083601 (2009).
- Filipp et al. [2011a] S. Filipp, A. F. van Loo, M. Baur, L. Steffen, and A. Wallraff, Preparation of subradiant states using local qubit control in circuit qed, Phys. Rev. A 84, 061805 (2011a).
- Filipp et al. [2011b] S. Filipp, M. Göppl, J. M. Fink, M. Baur, R. Bianchetti, L. Steffen, and A. Wallraff, Multimode mediated qubit-qubit coupling and dark-state symmetries in circuit quantum electrodynamics, Phys. Rev. A 83, 063827 (2011b).
- Delanty et al. [2011] M. Delanty, S. Rebić, and J. Twamley, Superradiance and phase multistability in circuit quantum electrodynamics, New J. Phys. 13, 053032 (2011).
- van Loo et al. [2013] A. F. van Loo, A. Fedorov, K. Lalumière, B. C. Sanders, A. Blais, and A. Wallraff, Photon-mediated interactions between distant artificial atoms, Science 342, 1494 (2013).
- Mlynek et al. [2014] J. Mlynek, A. Abdumalikov, C. Eichler, and A. Wallraff, Observation of dicke superradiance for two artificial atoms in a cavity with high decay rate, Nature Communications 5, 5186 (2014).
- Ma et al. [2019] R. Ma, B. Saxberg, C. Owens, N. Leung, Y. Lu, J. Simon, and D. I. Schuster, A dissipatively stabilized mott insulator of photons, Nature 566, 51 (2019).
- Song et al. [2019] C. Song, K. Xu, H. Li, Y.-R. Zhang, X. Zhang, W. Liu, Q. Guo, Z. Wang, W. Ren, J. Hao, H. Feng, H. Fan, D. Zheng, D.-W. Wang, H. Wang, and S.-Y. Zhu, Generation of multicomponent atomic schrödinger cat states of up to 20 qubits, Science 365, 574 (2019).
- Ye et al. [2019] Y. Ye, Z.-Y. Ge, Y. Wu, S. Wang, M. Gong, Y.-R. Zhang, Q. Zhu, R. Yang, S. Li, F. Liang, J. Lin, Y. Xu, C. Guo, L. Sun, C. Cheng, N. Ma, Z. Y. Meng, H. Deng, H. Rong, C.-Y. Lu, C.-Z. Peng, H. Fan, X. Zhu, and J.-W. Pan, Propagation and localization of collective excitations on a 24-qubit superconducting processor, Phys. Rev. Lett. 123, 050502 (2019).
- Yan et al. [2019] Z. Yan, Y.-R. Zhang, M. Gong, Y. Wu, Y. Zheng, S. Li, C. Wang, F. Liang, J. Lin, Y. Xu, C. Guo, L. Sun, C.-Z. Peng, K. Xia, H. Deng, H. Rong, J. Q. You, F. Nori, H. Fan, X. Zhu, and J.-W. Pan, Strongly correlated quantum walks with a 12-qubit superconducting processor, Science 364, 753 (2019).
- Wang et al. [2020] Z. Wang, H. Li, W. Feng, X. Song, C. Song, W. Liu, Q. Guo, X. Zhang, H. Dong, D. Zheng, H. Wang, and D.-W. Wang, Controllable switching between superradiant and subradiant states in a 10-qubit superconducting circuit, Phys. Rev. Lett. 124, 013601 (2020).
- Gong et al. [2021] M. Gong, S. Wang, C. Zha, M.-C. Chen, H.-L. Huang, Y. Wu, Q. Zhu, Y. Zhao, S. Li, S. Guo, H. Qian, Y. Ye, F. Chen, C. Ying, J. Yu, D. Fan, D. Wu, H. Su, H. Deng, H. Rong, K. Zhang, S. Cao, J. Lin, Y. Xu, L. Sun, C. Guo, N. Li, F. Liang, V. M. Bastidas, K. Nemoto, W. J. Munro, Y.-H. Huo, C.-Y. Lu, C.-Z. Peng, X. Zhu, and J.-W. Pan, Quantum walks on a programmable two-dimensional 62-qubit superconducting processor, Science 372, 948 (2021).
- Blais et al. [2004] A. Blais, R.-S. Huang, A. Wallraff, S. M. Girvin, and R. J. Schoelkopf, Cavity quantum electrodynamics for superconducting electrical circuits: An architecture for quantum computation, Phys. Rev. A 69, 062320 (2004).
- Tavis and Cummings [1968] M. Tavis and F. W. Cummings, Exact solution for an -molecule—radiation-field hamiltonian, Phys. Rev. 170, 379 (1968).
- Tavis and Cummings [1969] M. Tavis and F. W. Cummings, Approximate solutions for an -molecule-radiation-field hamiltonian, Phys. Rev. 188, 692 (1969).
- López et al. [2007] C. E. López, H. Christ, J. C. Retamal, and E. Solano, Effective quantum dynamics of interacting systems with inhomogeneous coupling, Phys. Rev. A 75, 033818 (2007).
- Forsch et al. [2019] M. Forsch, R. Stockill, A. Wallucks, I. Marinković, C. Gärtner, R. A. Norte, F. van Otten, A. Fiore, K. Srinivasan, and S. Gröblacher, Microwave-to-optics conversion using a mechanical oscillator in its quantum ground state, Nature Physics 16, 69 (2019).
- Schwinger [1952] J. Schwinger, On Angular Momentum (1952).
- Sakurai [1994] J. J. Sakurai, Modern Quantum Mechanics, revised ed. (Pearson, 1994).
- Carmichael [1999] H. J. Carmichael, Statistical methods in quantum optics 1: master equations and Fokker-Planck equations (Springer, 1999).
- Mariantoni et al. [2010] M. Mariantoni, E. P. Menzel, F. Deppe, M. A. Araque Caballero, A. Baust, T. Niemczyk, E. Hoffmann, E. Solano, A. Marx, and R. Gross, Planck spectroscopy and quantum noise of microwave beam splitters, Phys. Rev. Lett. 105, 133601 (2010).
- Menzel et al. [2010] E. P. Menzel, F. Deppe, M. Mariantoni, M. A. Araque Caballero, A. Baust, T. Niemczyk, E. Hoffmann, A. Marx, E. Solano, and R. Gross, Dual-path state reconstruction scheme for propagating quantum microwaves and detector noise tomography, Phys. Rev. Lett. 105, 100401 (2010).
- Menzel et al. [2012] E. P. Menzel, R. Di Candia, F. Deppe, P. Eder, L. Zhong, M. Ihmig, M. Haeberlein, A. Baust, E. Hoffmann, D. Ballester, K. Inomata, T. Yamamoto, Y. Nakamura, E. Solano, A. Marx, and R. Gross, Path entanglement of continuous-variable quantum microwaves, Phys. Rev. Lett. 109, 250502 (2012).
- Fedorov et al. [2016] K. G. Fedorov, L. Zhong, S. Pogorzalek, P. Eder, M. Fischer, J. Goetz, E. Xie, F. Wulschner, K. Inomata, T. Yamamoto, Y. Nakamura, R. Di Candia, U. Las Heras, M. Sanz, E. Solano, E. P. Menzel, F. Deppe, A. Marx, and R. Gross, Displacement of propagating squeezed microwave states, Phys. Rev. Lett. 117, 020502 (2016).
- Jaynes and Cummings [1963] E. T. Jaynes and F. W. Cummings, Comparison of quantum and semiclassical radiation theories with application to the beam maser, Proceedings of the IEEE 51, 89 (1963).
- Katriel et al. [1986] J. Katriel, A. I. Solomon, G. D’Ariano, and M. Rasetti, Multiboson holstein-primakoff squeezed states for su(2) and su(1,1), Phys. Rev. D 34, 2332 (1986).
- Bullough [1987] R. K. Bullough, Photon, quantum and collective, effects from rydberg atoms in cavities, Hyperfine Interactions 37, 71 (1987).
- Bullough et al. [1989] R. K. Bullough, G. S. Agarwal, B. M. Garraway, S. S. Hassan, G. P. Hildred, S. V. Lawande, N. Nayak, R. R. Puri, B. V. Thompson, J. Timonen, and M. R. B. Wahiddin, Giant quantum oscillators from rydberg atoms: Atomic coherent states and their squeezing from rydberg atoms, in Squeezed and Nonclassical Light, edited by P. Tombesi and E. R. Pike (Springer US, Boston, MA, 1989) pp. 81–106.
- Emary and Brandes [2003a] C. Emary and T. Brandes, Quantum chaos triggered by precursors of a quantum phase transition: The dicke model, Phys. Rev. Lett. 90, 044101 (2003a).
- Emary and Brandes [2003b] C. Emary and T. Brandes, Chaos and the quantum phase transition in the dicke model, Phys. Rev. E 67, 066203 (2003b).
- Karbach and Stolze [2005] P. Karbach and J. Stolze, Spin chains as perfect quantum state mirrors, Phys. Rev. A 72, 030301 (2005).
- Wójcik et al. [2005] A. Wójcik, T. Łuczak, P. Kurzyński, A. Grudka, T. Gdala, and M. Bednarska, Unmodulated spin chains as universal quantum wires, Phys. Rev. A 72, 034303 (2005).
- Wójcik et al. [2007] A. Wójcik, T. Łuczak, P. Kurzyński, A. Grudka, T. Gdala, and M. Bednarska, Multiuser quantum communication networks, Phys. Rev. A 75, 022330 (2007).
- Gratsea et al. [2018] A. Gratsea, G. M. Nikolopoulos, and P. Lambropoulos, Photon-assisted quantum state transfer and entanglement generation in spin chains, Phys. Rev. A 98, 012304 (2018).
- Clerk et al. [2010] A. A. Clerk, M. H. Devoret, S. M. Girvin, F. Marquardt, and R. J. Schoelkopf, Introduction to quantum noise, measurement, and amplification, Rev. Mod. Phys. 82, 1155 (2010).
- Muñoz and Delgado [2016] J. L. G. Muñoz and F. Delgado, QUANTUM: A Wolfram Mathematica add-on for Dirac Bra-Ket Notation, Non-Commutative Algebra, and Simulation of Quantum Computing Circuits, Journal of Physics: Conference Series 698, 012019 (2016).
- [62] The codes for deriving the effective Hamiltonian are available at www.notebookarchive.org/2021-07-duvj15v.
- Johansson et al. [2013] J. Johansson, P. Nation, and F. Nori, QuTiP 2: A python framework for the dynamics of open quantum systems, Computer Physics Communications 184, 1234 (2013).
- Zueco et al. [2009] D. Zueco, G. M. Reuther, S. Kohler, and P. Hänggi, Qubit-oscillator dynamics in the dispersive regime: Analytical theory beyond the rotating-wave approximation, Phys. Rev. A 80, 033846 (2009).