The electromagnetic duality and the 3+1D O(6) non-linear sigma model with a level-1 Wess-Zumino-Witten term
Abstract
We show that in (3+1)-D space-time dimensions, the O(6) non-linear sigma model, with a level-1 Wess-Zumino-Witten term, exhibits the electromagnetic duality. If we name the six components of the sigma field as the Neel and valence-bond-solid (VBS) order parameters, the hedgehogs of the Neel and VBS order play the role of monopole and charge. The duality corresponds to the exchange of monopole and charge hence the exchange of the Neel and VBS order. The condensation of monopole can trigger a direct Neel VBS phase transition. We conjecture that the critical point is self-dual, which is a generalization of the deconfined quantum critical point in (2+1)-D. In this theory, there exist two deconfined phases where the Neel/VBS hedgehogs are massive but cost finite energy. This leads to two fractionalized phases with particles carrying fractional spin or VBS quantum numbers and gapless gauge bosons.
Introduction Non-linear sigma (NL) models describe the dynamics of Goldstone mode. Due to the interaction between the Goldston bosons, NL models can develop a mass instead of being gapless [1]. A well-known example occurs in 1+1 dimensions, where the NL model is always gapful. On a different front, topological terms in the NL model can fundamentally modify the energy spectrum. For example, the NL model with term is gapful for while it is gapless for for [2]. In addition to the topological term, other topological terms, such as the Wess-Zumino-Witten [3] and the Hopf terms [4], are also known to change the Goldstone mode spectrum.
In Ref.[5], Senthil et al. proposed that despite the mismatch in symmetries, there can exist a continuous phase transition, which is dubbed “deconfined quantum critical point”, between the Neel and the valence-bond-solid (VBS) states in quantum magnets. Shortly after, it is proposed that the O(5) (3 Neel+2 VBS) NL model with a level-1 Wess-Zumino-Witten (WZW) term describes such a phase transition [6]. Recently, we point out that the same model can give rise to spin liquid and spin rotation symmetry breaking phases between the Neel and VBS order [7]. Moreover, an unexpected connection between the NL model approach, and the Schwinger boson approach [8] was revealed. This connection is interesting because, in the Schwinger boson approach, particles that fractionalize spins were built-in from the beginning. However, aside from the WZW term, the NL model in Ref.[7] only involves conventional order parameters. This connection between the WZW term and fractionalized particles is very intriguing, and it is natural to ask whether it can occur for higher dimensions.
The NL model with a level-1 Wess-Zumino-Witten (WZW) term in 3+1 space-time dimensions The action of this model read
| (1) |
For easy reference, we shall name the first three components of the Neel order parameters, and the last three components the valence bond solid (VBS) order parameters, i.e., . These names are motivated by the fact that Eq.(LABEL:o6) governs the low-energy dynamics of a three-dimensional Mott insulator with competing Neel and valence-bond solid (VBS) order [9] (see supplemental material section I). However, for the majority of this paper, these are just names we use to refer to the components of the .
In Eq.(LABEL:o6) the space-time manifold is spanned by and is spanned by and such that represents a one-parameter-family extension of the such that at , the configuration is trivial, say, , and at the . It can be shown that is independent of for as long as the coefficient in front of the WZW term is an integer multiple of .
The VBS hedgehog Consider the following configuration corresponding to a hedgehog in
| (3) |
where are the coordinates of the three dimensional space and is the Euclidean time. In Eq.(3), is a unit vector and is a smooth function satisfying for (hedgehog core size) and at . It is trivial to show that Plug Eq.(3) into Eq.(LABEL:o6), it is straightforward to show that
| (4) |
Eq.(4) is the Berry’s phase in the coherent state path integral of a spin 1/2 in 0+1 dimension. Therefore the hedgehog of the VBS order fractionalizes the spins! In the supplemental material section II, we present the microscopic theory of the hedgehog core.
If we define a two-component complex boson field
| (5) |
so that
| (6) |
it is simple to show that[7]
| (7) |
More generally, for a dynamic hedgehog where the world line forms a closed loop parametrized by , Eq.(7) becomes
| (8) |
This implies the hedgehog current couples to a gauge field, namely,
The statistics of VBS hedgehogs We can determine the statistics of the VBS hedgehogs by computing the Berry phase associated with the hedgehog exchange. Because our space-time is , the exchange process must be embedded in (i) vacuum creation of two pairs of hedgehog-anti-hedgehog, (ii) exchanging the two hedgehogs, and (iii) annihilating the hedgehogs with the anti-hedgehogs. The Berry phase contains two contributions: 1) that due to exchange of the hedgehogs and 2) that associated with the spin 1/2 in the cores of hedgehog and anti-hedgehog. To isolate the Berry phase due to the exchange, we lock the core spins in, say, the positive -direction.
In the following, we present an argument suggesting that under the space-time configuration discussed above, the Berry phase due to hedgehog exchange is zero. The argument involves two steps: (i) using the result in Ref.[10], one can show that when any one of the six components of is zero, the WZW term reduces to the topological -term (with ) for the remaining five components. This topological term is non-zero only when the wrapping number associated with the mapping from the space-time to the order parameter manifold formed by the non-zero components, in this case, , is non-zero. (ii) We note that in the present situation only four components of are non-zero. By counting the dimension of the space-time image, we conclude that any such cannot produce a non-zero wrapping number, hence the Berry phase vanishes. This argument suggests that the VBS hedgehogs are bosons. A similar argument can be used to deduce that the Neel hedgehog is bosons as well.
The CP1 theory of Neel order The NL model action for the Neel order parameter reads
| (9) |
It is well known that the above action can be rewritten as[11]
| (10) |
where is a compact dynamic gauge field and is the two-component complex boson field satisfying Eq.(5) and Eq.(6). Because the action in Eq.(10) is at most quadratic in the saddle point is exact, namely,
| (11) |
In Eq.(10), the condensation of the boson Higgs the gauge field , hence mods out the redundant local phase gauge of freedom in Eq.(6). The resulting phase exhibits the Neel long-range order. Combining Eq.(8) and the above discussion suggests that the field theory for the VBS hedgehog is given by
| (12) |
Here represents the boson field for the VBS hedgehog. Once again, we see that when supplemented with the WZW term, the conventional Ginzburg-Landau theory is equivalent to a theory with fractionalized particles and gauge field.
The Neel hedgehog A parallel discussion with “VBS” and “Neel” switched implies that the Neel hedgehog carries the Berry phase of the VBS order parameter. Specifically after the switch Eq.(4) becomes
| (13) |
If we define a two-component complex boson field
| (14) |
it is simple to show that
| (15) |
This implies the VBS hedgehog current couples to a gauge field, namely,
| (16) |
The CP1 theory of VBS order The NL model action for the VBS order parameter read
| (17) |
Similar to Eq.(10) we can rewrite Eq.(17) as
| (18) |
where is a compact dynamic gauge field and is the two-component complex boson field satisfying Eq.(14). In this case
| (19) |
Analogous to the discussion after Eq.(10), the condensation of the bosons induces the VBS long-range order. The above discussion suggests that the field theory for the Neel hedgehog is given by
Here represents the boson field for the Neel hedgehog.111When Eq.(LABEL:Neelhedgehog) is used to describe the acutal VBS order of a Mott insulator, there is an additional term which should be added to Eq.(LABEL:Neelhedgehog). This term describes the anisotropy imposed by the lattice of the Mott insulator. However, for our purpose the term Neel and VBS are just names we assign to the components of , therefore the above anisotropy term is absent.
The monopoles One can ask what do the Neel and VBS hedgehog correspond to in terms of the fields in Eq.(12) and Eq.(LABEL:Neelhedgehog). Consider a static Neel hedgehog at the spatial origin, namely,
where are the spatial spherical coordinates. Through , the corresponding is222Here due to the singular configuration of we need to take two patches to avoid the singularity around and . Specifically the northern hemisphere patch is and the southern hemisphere patch is These two patches are related by a phase transformation . In terms of the phase transformation is a gauge transformation. However in the following we shall compute the field strength which is independent of the gauge. Therefore we can use either gauge to do the calculation.
Eq.(11) implies the corresponding gauge field (one-form) is whose field strength (two-form) is
| (21) |
If we integrate Eq.(21) over any closed surface enclosing the hedgehog the result is . Hence the Neel hedgehog is a monopole in . The same calculation goes through if we change Neel to VBS and to , i.e., a VBS hedgehog is a monopole in .
The electromagnetic duality These discussions suggest the monopole in is the charge of and the monopole in is the charge of . We summarize such relation in Table 1.
| Neel | VBS | |
| Gauge field | ||
| VBS hedgehog | charge | monopole |
| Neel hedgehog | monopole | charge |
| Hedgehog field |
In this duality , and Neel order VBS order. Here means “is dual to”.
The Neel to VBS phase transition In the above duality, the condensation of, e.g., the monopole will confine the charge. According to Table 1 the charge is the monopole. Putting it differently, since the monopole is the charge, condensation of it will Higgs hence confine the monopole. Of course, the statement with and exchanged is true. If there is a direct continuous phase transition between the Neel (condensation of monopole or charge ) and VBS (condensation of charge or monopole) order, which is equivalent to a direct transition from the confinement to the Higgs phase in Eq.(12) and Eq.(LABEL:Neelhedgehog). At the critical point, neither the VBS nor the Neel hedgehog can condense but they can be both gapless. We conjecture that this happens at the self-dual point of the electromagnetic duality discussed in Table 1. The possibility of such a direct, continuous phase transition is supported by the Monte-Carlo simulation of the lattice version of Eq.(12) [12].
Spin liquids In Ref.[12], it is also shown that in the plane spanned by - in Eq.(12), there exists a deconfined Coulomb phase where gauge bosons are gapless. In this phase the charge or monopole is massive, but finite energy, excitations. Since the charge is the spinon, this phase is a spin liquid. A similar argument applies to Eq.(LABEL:Neelhedgehog). In the deconfined phase, the gauge bosons are gapless, and the charge is a finite energy excitation. Since the charge fractionalizes the VBS order parameter, this phase is a “VBS” liquid. The spin liquid phase discussed above is analogous to that found in Ref.[13] for O(3) NL model in (2+1)-D after the hedgehog suppression. In the phase diagram, these two fractionalized phases should sit symmetrically about the self-dual point.
The fractionalized phases discussed above are made possible by the fact that in 3+1 dimensions, the compact U(1) gauge theory has a deconfinement phase. This is very different from 2+1 dimensions, where compact U(1) gauge theory always confines [14]. In the latter case, deconfinement requires the condensation of an additional Higgs field carrying, e.g., two units of the . This Higgs the gauge group of to which has a deconfined phase.
Dimension reduction and the relation to O(5) NL model with WZW term in 2+1D
In general, given an NL model with WZW term in space-time dimensions, we can obtain an NL model with WZW term in space-time dimensions by the following dimension reduction. The idea is to create a domain wall in the, say, last component of . Specifically let us consider the following configuration where
| (22) |
Here is a smooth function satisfying for (the domain wall thickness) and at . Plug Eq.(22) into Eq.(LABEL:o6) it is straightforward to show that where
| (23) |
Eq.(23) is precisely the WZW term of NL model in (2+1)-D. Adopting the name that are the components of the NL model, and are the components of the NL model, we next show that the space-time Neel hedgehog in the NL model carries a Berry’s phase that depends on the direction of the VBS order parameter in the core.
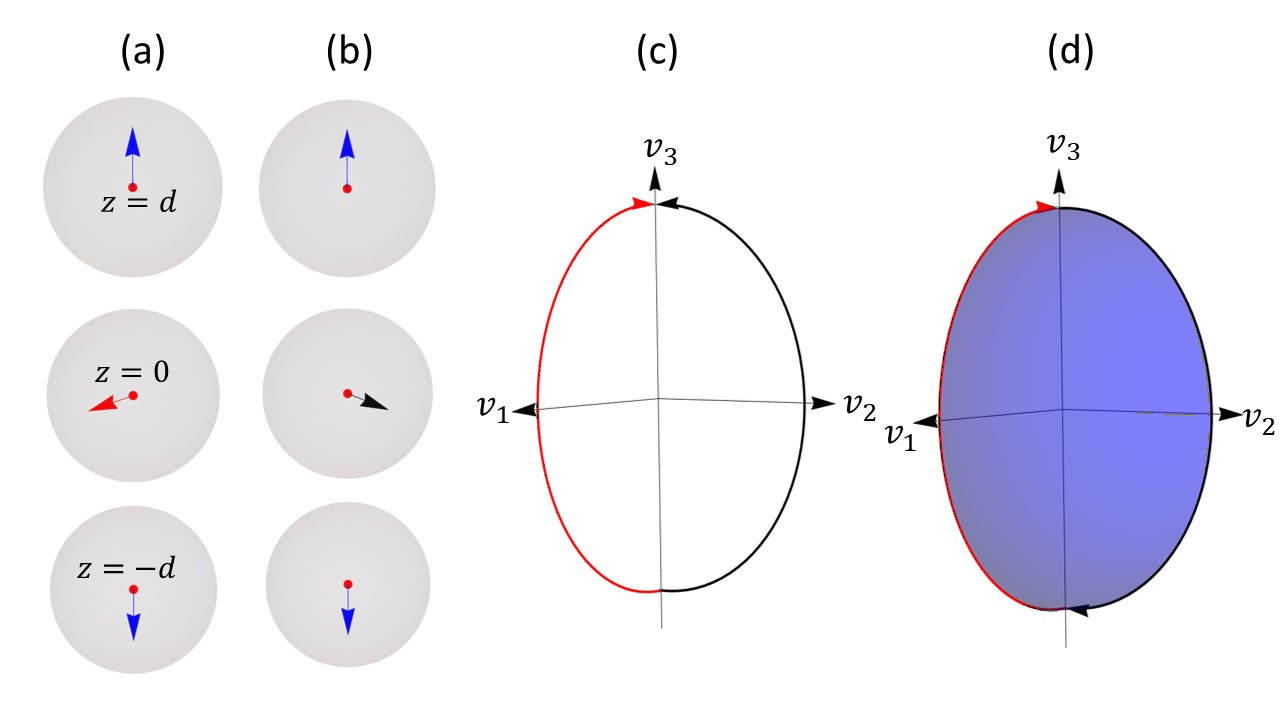
Consider the space-time configuration of the NL model shown in Fig. 1(a),(b). Here a dimensional domain wall in spanned by -- with width is centered at . A Neel hedgehog sits in the domain wall region with the core VBS order parameter points in different directions at as shown in Fig. 1(a) and (b). According to Eq.(16) the hedgehog couples to the Berry connection (see section III of the supplemental material for more details). In the order parameter space spanned by --, the core VBS order parameter in Fig. 1(a) and (b) traces out the red and black trajectories, respectively (see Fig. 1(c)). The Berry phase difference is one-half of the soliton angle sustained by the blue surface shown in Fig. 1(d). This is precisely the hedgehog Berry’s phase discussed by Haldane [15]. In his case the x-y plane is a square lattice favoring 4 different VBS angles (), namely, ( corresponds to the columnar VBS phase and the plaquette VBS phase). This gives rise to four relative hedgehog Berry’s phase, namely, , between the hedgehog with different VBS angles in the core, which causes destructive interference when hedgehog proliferate. The exception is hedgehogs with topological charge 4, which have the same Berry’s phase regardless of the core VBS order parameter. When such degree-4 hedgehogs proliferate and condense, it results in the four-fold degenerate VBS order.
Conclusion: In this paper we demonstrate that the O(6) NL model in (3+1)-D exhibits the electromagnetic duality. Novel phase transition and fractionalized phases associated with this duality are discussed.
References
- [1] A. Polyakov, “Interaction of goldstone particles in 2 dimensions - applications to ferromagnets and massive yang-mills fields,” Phys. Lett. B, vol. 59, no. 1, pp. 79–81, 1975.
- [2] F. Haldane, “Continuum dynamics of the 1-D Heisenberg anti-ferromagnet - identification with the O(3) non-linear sigma-model,” Phys. Lett. A, vol. 93, no. 9, pp. 464–468, 1983.
- [3] E. Witten, “Non-abelian bosonization in 2 dimensions,” Commun. Math. Phys., vol. 92, no. 4, pp. 455–472, 1984.
- [4] A. Polyakov, “Fermi-Bose transmutations induced by gauge-fields,” Mod. Phys. Lett. A, vol. 3, no. 3, pp. 325–328, 1988.
- [5] T. Senthil, A. Vishwanath, A. Balents, S. Sachdev, and M. Fisher, “Deconfined quantum critical points,” Science, vol. 303, no. 5663, pp. 1490–1494, 2004.
- [6] A. Tanaka and X. Hu, “Many-body spin berry phases emerging from the -flux state: Competition between antiferromagnetism and the valence-bond-solid state,” Phys. Rev. Lett., vol. 95, no. 3, 2005.
- [7] Y.-T. Huang and D.-H. Lee, “Competing orders, the Wess-Zumino-Witten term, and spin liquids,” arXiv:2204.12485v1, 2022.
- [8] S. Sachdev and N. Read, “Large N expansion for frustrated and doped quantum antiferromagnets,” Int. J. Mod. Phys. B, vol. 05, no. 01n02, pp. 219–249, 1991.
- [9] Y.-T. Huang and D.-H. Lee, “Non-abelian bosonization in two and three spatial dimensions and applications,” Nucl. Phys. B, vol. 972, p. 115565, 2021.
- [10] A. Abanov and P. Wiegmann, “Theta-terms in nonlinear sigma-models,” Nucl. Phys. B, vol. 570, no. 3, pp. 685–698, 2000.
- [11] E. Witten, “Instantons, the quark-model, and the 1/N expansion,” Nucl. Phys. B, vol. 149, no. 2, pp. 285–320, 1979.
- [12] K. Sawamura, T. Hiramatsu, K. Ozaki, I. Ichinose, and T. Matsui, “Four-dimensional + lattice gauge theory for three-dimensional antiferromagnets: Phase structure, gauge bosons, and spin liquid,” Phys. Rev. B, vol. 77, no. 22, 2008.
- [13] O. Motrunich and A. Vishwanath, “Emergent photons and transitions in the O(3) sigma model with hedgehog suppression,” Phys. Rev. B, vol. 70, no. 7, 2004.
- [14] A. Polyakov, “Quark confinement and topology of gauge theories,” Nucl. Phys. B, vol. 120, no. 3, pp. 429–458, 1977.
- [15] F. Haldane, “O(3) nonlinear sigma-model and the topological distinction between integer-spin and half-integer-spin antiferromagnets in 2 dimensions,” Phys. Rev. Lett., vol. 61, no. 8, pp. 1029–1032, 1988.