[longtable]LTcapwidth=0.96
Steady Rayleigh–Bénard convection between no-slip boundaries
Abstract
The central open question about Rayleigh–Bénard convection—buoyancy-driven flow in a fluid layer heated from below and cooled from above—is how vertical heat flux depends on the imposed temperature gradient in the strongly nonlinear regime where the flows are typically turbulent. The quantitative challenge is to determine how the Nusselt number depends on the Rayleigh number in the limit for fluids of fixed finite Prandtl number in fixed spatial domains. Laboratory experiments, numerical simulations, and analysis of Rayleigh’s mathematical model have yet to rule out either of the proposed ‘classical’ or ‘ultimate’ asymptotic scaling theories. Among the many solutions of the equations of motion at high are steady convection rolls that are dynamically unstable but share features of the turbulent attractor. We have computed these steady solutions for up to with and various horizontal periods. By choosing the horizontal period of these rolls at each to maximize , we find that steady convection rolls achieve classical asymptotic scaling. Moreover, they transport more heat than turbulent convection in experiments or simulations at comparable parameters. If heat transport in turbulent convection continues to be dominated by heat transport in steady rolls as , it cannot achieve the ultimate scaling.
keywords:
convection, coherent structure, heat transport1 Introduction
Rayleigh–Bénard convection (RBC) is the buoyancy-driven flow in a fluid layer heated from below and cooled from above in the presence of gravity. The emergent convective flow enhances heat flux from the warm bottom boundary to the cool top boundary beyond the conductive flux from diffusion alone. This dimensionless enhancement factor—the ratio of bulk-averaged vertical heat flux from both conduction and convection to the flux from conduction alone—defines the Nusselt number . In Rayleigh’s mathematical model (Rayleigh, 1916) depends on several dimensionless quantities characterizing the problem at hand: (i) what we now call the Rayleigh number , which is proportional to the imposed temperature drop across the layer, (ii) the fluid’s Prandtl number , which is the ratio of kinematic viscosity to thermal diffusivity, and (iii) details of the spatial domain, often captured by an aspect ratio that is a ratio of a horizontal length scale to the vertical layer height.
Convection is coherent at values not too far above the critical value beyond which the conductive no-flow state is linearly unstable. By coherent we mean flows with few scales present; spatial scales might include a horizontal period and the vertical thickness of boundary layers, and temporally the flow may be steady or time-periodic. Meanwhile, convection is turbulent at the large values pertinent to many engineering and scientific applications. Turbulent flows are complex and contain a range of spatial and temporal scales and, in the present context, have thermal and viscous boundary layers at the top and bottom boundaries from which thermal plumes emerge and mix the bulk. In a given domain it is expected that a scaling of with respect to both and will emerge in the limit (Kadanoff, 2001).
After nearly a century of increasingly sophisticated mathematical analysis, increasingly resolved direct numerical simulations (DNS), and increasingly refined laboratory experiments, two quantitatively distinct conjectures remain in contention for the heat transport scaling law at large (Chillà & Schumacher, 2012; Doering, 2020). The two conjectures follow from heuristic physical arguments that both seem plausible but give incompatible predictions: the ‘classical’ scaling and the ‘ultimate’ scaling , with the latter sometimes including logarithmic-in- modifications.
For RBC between flat, no-slip, isothermal boundaries, rigorous analysis of the governing equations has yielded upper bounds of the form uniformly in and (Howard, 1963; Doering & Constantin, 1996), but this still allows for either classical or ultimate scaling. Upper bounds that rule out ultimate scaling by being asymptotically smaller than have been derived in the limit of infinite (Doering et al., 2006; Otto & Seis, 2011; Whitehead & Doering, 2012) and for two-dimensional convection between stress-free boundaries (Whitehead & Doering, 2011). For the no-slip boundaries relevant to experiments, however, it remains an open question whether an upper bound asymptotically smaller than is possible.
In view of the problem’s stubbornness, a new strategy is called for to determine—or at least to bound— as a function of , and . Toward that end we have undertaken an indirect approach consisting of two parts. The first part is to study coherent flows for which one can reasonably hope to determine asymptotic heat transport, and the second part is to investigate how transport by those coherent flows compares with transport by turbulent convection. The simplest coherent flows are steady—i.e., time-independent—solutions of the equations of motion. Many such states exist, although they are generally unstable at large . We focus on what might be called the simplest type of steady states: two-dimensional convection rolls like the counter-rotating pairs shown in figure 1(, ). In horizontally periodic or infinite domains in two or three dimensions, such rolls bifurcate supercritically from the conductive state in the linear instability identified by Rayleigh (1916). A roll pair of any width-to-height aspect ratio admitted by the domain exists for sufficiently large .
For steady rolls, the dependence of on the parameters at asymptotically large is accessible to computation. As for whether heat transport by steady rolls can be connected to transport by turbulence, there are several reasons for optimism. Relationships between turbulent attractors and the unstable coherent states embedded therein have been established in models of wall-bounded shear flows (Graham & Floryan, 2021), where particular steady states, traveling waves, and time-periodic states have been found that closely reflect turbulent flows in terms of integral quantities as well as particular flow structures. Analogous study of RBC began only recently but indeed suggests that certain steady states capture qualitative aspects of turbulent convection (Waleffe et al., 2015; Sondak et al., 2015; Kooloth et al., 2021; Motoki et al., 2021). Our findings add to this evidence. The desire to understand and perhaps strengthen the mathematical bound is further motivation for studying unstable states since bounds apply to all solutions of the governing equations regardless of stability. It is an open question whether any solutions can achieve ultimate scaling, let alone turbulent solutions.
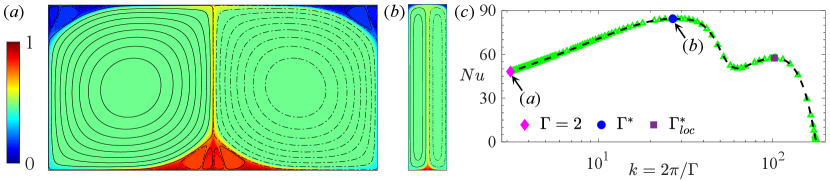
Here we report numerical computations of steady convection rolls for a fluid contained between no-slip isothermal top and bottom boundaries. We reach sufficiently large values to convincingly reveal several asymptotic scalings of , depending on the horizontal periods of the rolls. These are the first clearly asymptotic scalings found for any type of flow—steady, turbulent, or otherwise—for RBC in the no-slip case. Notably, the largest heat transport among steady rolls of all horizontal periods displays the classical scaling. We further observe that for these steady rolls is larger than turbulent from all laboratory experiments and two- or three-dimensional (2D or 3D) simulations at comparable parameters. This observation supports the conjecture that steady states maximize among all stable or unstable flows, as was recently verified for a truncated model of RBC (Olson et al., 2021) using methods that are not yet applicable to the full governing equations. If steady-roll transport continues to dominate turbulent transport as , then our finding of classical scaling for steady rolls would rule out ultimate scaling of turbulent convection.
The asymptotic scaling of steady rolls is already known in the case of stress-free velocity conditions at the top and bottom boundaries, which were considered for mathematical convenience in Rayleigh’s original work. In that case as at fixed and , and the aspect ratio of the roll pair maximizing at each and approaches (Chini & Cox, 2009; Wen et al., 2020). Recent computations of steady rolls in the no-slip case for pre-asymptotic values up to revealed significant differences from the stress-free problem (Waleffe et al., 2015; Sondak et al., 2015). The dependence for no-slip rolls at fixed and can have multiple local maxima, as shown in figure 1(), and the aspect ratio that globally maximizes approaches zero rather than a constant as . Steady rolls of -maximizing aspect ratios were reported in Sondak et al. (2015) for at , yielding fits of and . This heat transport scaling is faster than with fixed: computations in Waleffe et al. (2015) for at with fixed yield the fit . These best-fit scaling exponents are, however, not asymptotic.
Steady convection rolls are dynamically unstable at large and cannot be found by standard time integration, so we employed a purpose-written code that iteratively solves the time-independent equations. We computed rolls with fixed for and with the parameter-dependent aspect ratios and (cf. figure 1) that globally and locally maximize , respectively, for . These values are evidently large enough to reach asymptotia: the results reported below strongly suggest that fixed- rolls asymptotically transport heat like while the ever-narrowing rolls of aspect ratio achieve the classical scaling.
2 Computation of steady-convection-roll solutions
Following Rayleigh (1916), we model RBC using the Boussinesq approximation to the Navier–Stokes equations with constant kinematic viscosity , thermal diffusivity , and coefficient of thermal expansion . We nondimensionalize lengths by the layer height , temperatures by the fixed difference between the boundaries, velocities by the free-fall scale , and time by the free-fall time . Calling the horizontal coordinate and the vertical coordinate , the gravitational acceleration of magnitude is in the direction. The evolution equations governing the dimensionless velocity vector , temperature , and pressure are then
| (1a) | ||||
| (1b) | ||||
| (1c) | ||||
where
| (2,) | |||
The dimensionless spatial domain is , and all variables are horizontally periodic. The top and bottom boundaries are isothermal with and , respectively, while no-slip conditions require to vanish on both boundaries. The conductive state becomes unstable when increases past the critical value (Jeffreys, 1928), at which a roll pair with horizontal period bifurcates supercritically. As the horizontal period of the narrowest marginally stable roll pair decreases as , while the horizontal period of the fastest-growing linearly unstable mode decreases more slowly as .
In terms of the dimensionless solutions to 1, the Nusselt number is
| (3) |
where denotes an average over the spatial domain and infinite time. For steady states no time average is needed.
To compute rolls at values large enough to reach the asymptotic regime we developed a numerical scheme by adapting the approach of Wen et al. (2020) and Wen & Chini (2018) to the case of no-slip boundary conditions. In these numerics the temperature is represented using the deviation from the conductive profile, meaning , and the velocity is represented using a stream function , where so that the (negative) scalar vorticity is . In terms of these variables, steady () solutions of 1 satisfy
| (4a) | ||||
| (4b) | ||||
| (4c) | ||||
with fixed-temperature and no-slip boundary conditions,
| (5,,) | |||
To compute solutions of the time-independent equations 4 and 5 by an iterative method, we do not need to impose all boundary conditions precisely on each iteration—the conditions need to hold only for the converged solution. Thus we do not impose (5) exactly, instead using approximate boundary conditions on for equation 4a. These are derived by Taylor expanding about the top and bottom boundaries to find
| (6a) | ||||
| (6b) | ||||
where is small. Combining equations (4b) with (5,) and neglecting terms in 6 give the approximate boundary conditions,
| (7,) | |||
In computations we set to be the distance between the boundary and the first interior mesh point.
The time-independent equations (4) are solved numerically subject to boundary conditions (5,) and (7) using a Newton–GMRES (generalized minimal residual) iterative scheme. The spatial discretization is spectral, using a Fourier series in and a Chebyshev collocation method in (Trefethen, 2000). All of our computations had at least 20 collocation points in the viscous and thermal boundary layers. At just above the linear instability, iterations starting from the unstable eigenmode converge to the steady rolls we seek. At larger , already-computed steady rolls from nearby and values were used as the initial iterate. Every 2 to 4 Newton iterations, we change the boundary values of the iterate to match the boundary condition exactly. Prior to convergence this makes the boundary values slightly inconsistent with the governing equations, but the converged solutions satisfy the equations and the no-slip boundary conditions to high precision. Newton iterations were carried out until the Lebesgue -norm of the residual of the governing steady equations had a relative magnitude less than . To accurately locate and , rolls were computed at several nearby , and then was interpolated with cubic splines like those in figure 1(). Details of computational results, including resolutions used, are included in the supplementary material.
3 Results
We computed steady rolls for aspect ratios encompassing the three distinguished values indicated by figure 1(): the fixed value and the -dependent values and that globally and locally maximize over . As previously observed by Sondak et al. (2015), the curve has a single maximum when is small and develops a second local maximum at smaller when increases past roughly . The value of at this second local maximum remains less than the value at the first, so the picture remains as in figure 1() with on the left and on the right, in contrast to the and 100 cases (Sondak et al., 2015). For most values we did not compute rolls over a full sweep through as in figure 1(), instead searching over only as needed to locate and . The rest of this section reports Nusselt number and Reynolds number scalings for the computed steady rolls, and the supplementary material provides tabulated data.
3.1 Asymptotic heat transport
Figure 2 shows the dependence of on for steady rolls with aspect ratios , , and . In the top panel is compensated by , so the horizontal line approached by rolls of the -maximizing aspect ratios corresponds to classical scaling. The downward slopes of the data for aspect ratios 2 and correspond to scaling exponents smaller than 1/3. Values of at computed previously for (Sondak et al., 2015; Waleffe, 2020) are shown in figure 2 also, and they agree with our computations very precisely—e.g., the data point agrees with our value of to within 0.0008%.
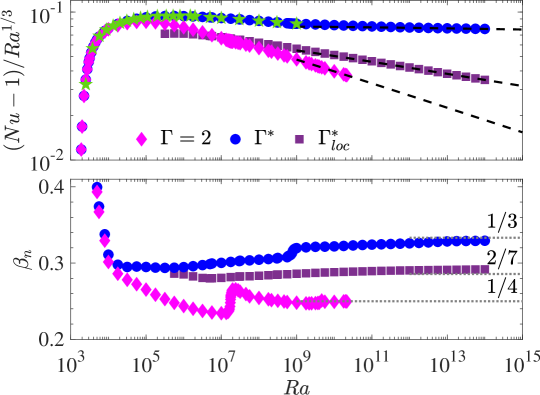
The bottom panel of figure 2 shows the -dependent local scaling exponent of the – relation for , , and . This quantity educes small variations not visible in the top panel. In particular, for rolls of aspect ratios , the exponent exhibits a small but rapid change just below , beyond which it smoothly approaches the classical exponent that appears to be the asymptotic behavior. This rapid change seems to coincide with the velocity becoming vertically uniform outside the boundary layers, as reflected in the streamlines of figure 1(); further details of the rolls’ structure will be reported elsewhere. Rolls with fixed undergo a similarly rapid change around and then approach scaling that appears to be asymptotic. Rolls of aspect ratio show intermediate scaling whose best-fit exponent over the last decade of data is 0.29.
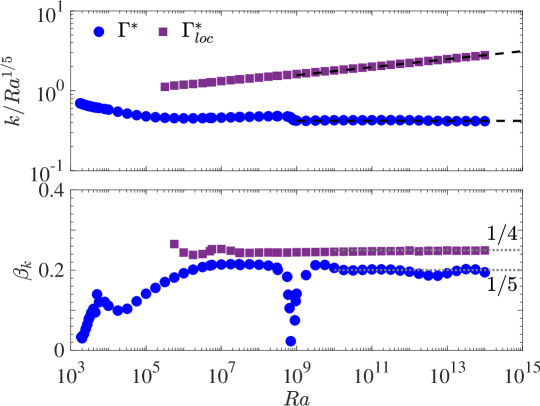
The top panel of figure 3 shows the -dependence of the wavenumber for and , compensated by . The compensated wavenumbers for approach a horizontal line, suggesting that the -maximizing rolls narrow according to the power law . This narrowing of is slow relative to the case of RBC in a porous medium, where (Wen et al., 2015).
The bottom panel of figure 3 shows the -dependence of the local scaling exponent . For the local scaling exponent remains close to after the transition around . For the exponent seems to approach , suggesting that has the same scaling as the narrowest marginally stable mode. Variations in beyond for are evident, but these might be due to numerical imprecision: depends very weakly on around the maximum of , as seen in figure 1(), so the value of cannot be determined nearly as precisely as the value of .
3.2 Asymptotic kinetic energy
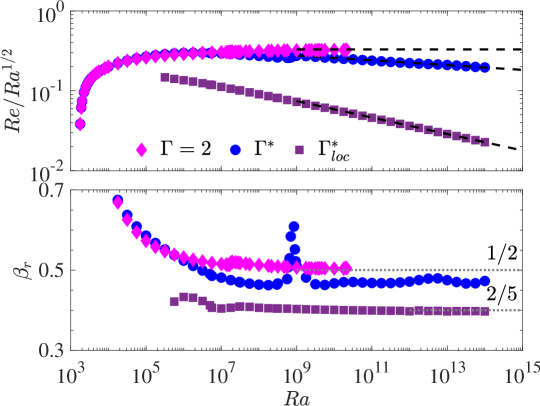
Another emergent quantity central to RBC is the bulk Reynolds number based on root-mean-squared velocity, which in terms of dimensionless solutions to 1 is
| (8) |
Figure 4 depicts the dependence of on for the steady rolls of aspect ratios , , and . The top panel shows compensated by while the bottom panel shows the local scaling exponent . Rolls with the fixed aspect ratio approach the asymptotic scaling that corresponds to the root-mean-squared velocity being proportional to the free-fall velocity . For rolls with -maximizing aspect ratios , the scaling fit over is , which is quite close to the scaling observed in recent 3D direct numerical simulations up to at in a slender cylinder with a height 10 times its diameter (Iyer et al., 2020). For the rolls the scaling exponent of is indistinguishable from . The measured exponents (0.50, 0.47, 0.40) are unchanged if is defined using the pointwise maximum velocity rather than using the root-mean-squared velocity as in 8. All three aspect ratios result in smaller speeds than steady rolls between stress-free boundaries, where for any fixed and (Wen et al., 2020).
4 Comparison with turbulent convection
To compare heat transport by steady rolls with that by turbulent thermal convection, we compiled Nusselt number data from high- DNS with or 0.7 and laboratory experiments where the estimated is between 0.7 and 1.3. Figure 5 shows these values compensated by , along with values of steady convection rolls at the -maximizing aspect ratios . Strikingly, heat transport by the -maximizing 2D steady rolls is larger than transport by turbulent convection in all cases.
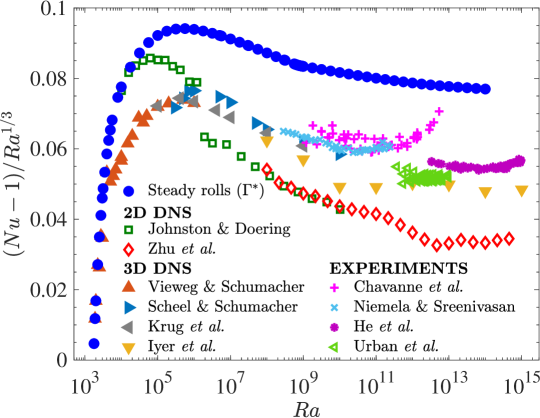
The turbulent data shown in figure 5, as detailed in the figure caption, include DNS in horizontally periodic 2D and 3D domains, wherein 2D steady rolls solve the equations of motion, as well as 3D DNS and laboratory experiments in cylinders that do not admit 2D rolls. Values of for steady rolls with fixed are omitted from figure 5 for clarity, but they lie below all turbulent values once approximately exceeds (cf. figure 2), and this gap would only widen at larger if their scaling persists. The laboratory data sets in figure 5 have unavoidably varying values that can be hard to estimate, as well as non-Oberbeck–Boussinesq effects (Urban et al., 2011, 2012, 2014). The figure includes only a narrow range of estimated values in order to avoid significant non-Oberbeck–Boussinesq effects. When data over a wider range of estimated is included, a few data points from the experiments of Chavanne et al. (2001) lie above the values of steady rolls, as shown in the supplementary material.
Our finding that steady rolls of -maximizing aspect ratios apparently display classical asymptotic scaling does not ineluctably imply anything about turbulent convection. Taking a dynamical systems point of view, however, steady solutions admitted by the domain are fixed points of 1, so they and their unstable manifolds are part of the global attractor. Turbulent trajectories may linger near these fixed points and so inherit some quantitative features (Kooloth et al., 2021), as has been found for unstable coherent states in shear flows (Nagata, 1990; Waleffe, 1998; Wedin & Kerswell, 2004; Gibson et al., 2008; Suri et al., 2020; Graham & Floryan, 2021). Indeed, figure 5 shows scaling similarities between steady and turbulent convection. Further exploration of the global attractor calls for study of 3D steady flows. Recently computed ‘multi-scale’ 3D steady states (Motoki et al., 2021) give larger values than all 2D rolls at moderate , but their scaling at large is unknown. Simpler 3D steady convection patterns remain to be computed as well. Analytically, it is an open challenge to construct approximations of 2D or 3D steady flows that are asymptotically accurate as , as has been done for 2D rolls between stress-free boundaries (Chini & Cox, 2009; Wen et al., 2020). Such constructions could be used to verify that is indeed the exact asymptotic scaling for the -maximizing rolls we have computed, as well as to determine the precise – scaling relations for rolls of both - and -maximizing aspect ratios.
More generally, figure 5 highlights the absence of reproducible evidence for ultimate scaling, and it raises the intriguing possibility that steady rolls with might transport more heat than turbulent convection as . We know of no counterexamples to this hypothesis, including in the case of stress-free boundaries (Wen et al., 2020). Heat transport by solutions of 1 with no-slip isothermal boundaries has been mathematically proved to be limited by (Howard, 1963; Doering & Constantin, 1996), but it remains unknown whether any solutions attain the ultimate scaling of this upper bound. One avenue for pursuing a stronger mathematical statement is to study two conjectures suggested by our computations: that steady convection maximizes among all solutions of 1 regardless of their stability or time-dependence, and that steady solutions of 1 are subject to an upper bound of the form . Therefore, although numerically computed flows can never determine scaling definitively, our results suggest a new mathematical approach that may be able to finally resolve the question of asymptotic scaling in turbulent convection.
Acknowledgements
After this manuscript was written our senior author, Charles Doering, passed away too soon. Beyond his many contributions to the present study, we are forever indebted to him for his mentorship, to say nothing of his many lasting contributions to the field of fluid dynamics. He will be deeply missed by us and many others. We also want to acknowledge helpful discussions about the present work with L.M. Smith, D. Sondak, and F. Waleffe. This work was supported by US National Science Foundation awards (DMS-1515161, DMS-1813003), Canadian NSERC Discovery Grants Program awards (RGPIN-2018-04263, RGPAS-2018-522657, DGECR-2018-00371), and computational resources provided by Advanced Research Computing at the University of Michigan.
Declaration of interests
The authors report no conflict of interest.
Supplementary Material
Numerical solutions
Tables LABEL:tab:Gamma2 to LABEL:tab:GammaLocal give the , , and values for numerical solutions with and , , and , respectively.
| 1 | 128 65 | 1.056590 | 1.617336 | ||
| 1 | 128 65 | 1.145807 | 2.682155 | ||
| 1 | 128 65 | 1.212037 | 3.317190 | ||
| 1 | 128 65 | 1.355410 | 4.550975 | ||
| 1 | 128 65 | 1.474455 | 5.537770 | ||
| 1 | 128 65 | 1.575599 | 6.391812 | ||
| 1 | 128 65 | 1.663162 | 7.159844 | ||
| 1 | 128 65 | 1.714193 | 7.624400 | ||
| 1 | 128 65 | 1.808754 | 8.526064 | ||
| 1 | 128 65 | 1.926775 | 9.740578 | ||
| 1 | 128 65 | 2.025985 | 10.85141 | ||
| 1 | 128 65 | 2.111714 | 11.88534 | ||
| 1 | 128 65 | 2.204811 | 13.09152 | ||
| 1 | 128 65 | 2.476330 | 17.05494 | ||
| 1 | 128 65 | 2.648664 | 20.07400 | ||
| 1 | 128 65 | 3.122843 | 29.50047 | ||
| 1 | 128 65 | 3.665041 | 42.29585 | ||
| 1 | 128 65 | 4.287042 | 59.56858 | ||
| 1 | 128 65 | 4.994322 | 82.84462 | ||
| 1 | 128 65 | 5.795869 | 114.2355 | ||
| 1 | 128 97 | 6.703915 | 156.5252 | ||
| 1 | 128 97 | 7.732236 | 213.4031 | ||
| 1 | 128 97 | 8.896615 | 289.7982 | ||
| 1 | 256 97 | 10.21546 | 392.2837 | ||
| 1 | 256 129 | 11.71065 | 529.6372 | ||
| 1 | 256 129 | 13.40898 | 713.6005 | ||
| 1 | 512 129 | 15.34493 | 959.9367 | ||
| 1 | 512 129 | 16.46456 | 1119.932 | ||
| 1 | 512 129 | 16.87881 | 1182.172 | ||
| 1 | 512 193 | 17.13944 | 1222.005 | ||
| 1 | 512 193 | 17.26636 | 1241.484 | ||
| 1 | 512 193 | 17.39216 | 1260.709 | ||
| 1 | 512 193 | 17.48282 | 1274.414 | ||
| 1 | 512 193 | 17.54351 | 1283.493 | ||
| 1 | 512 193 | 17.58987 | 1290.377 | ||
| 1 | 512 193 | 17.60946 | 1293.277 | ||
| 1 | 512 193 | 17.64500 | 1298.522 | ||
| 1 | 512 193 | 17.77160 | 1317.122 | ||
| 1 | 512 193 | 17.89693 | 1335.501 | ||
| 1 | 512 193 | 18.02044 | 1353.657 | ||
| 1 | 512 193 | 18.14193 | 1371.593 | ||
| 1 | 512 193 | 19.04221 | 1507.890 | ||
| 1 | 512 193 | 19.83413 | 1633.418 | ||
| 1 | 512 193 | 20.47798 | 1739.647 | ||
| 1 | 512 193 | 22.44036 | 2087.182 | ||
| 1 | 512 193 | 23.76002 | 2340.822 | ||
| 1 | 768 257 | 27.50669 | 3144.931 | ||
| 1 | 768 257 | 31.81154 | 4220.616 | ||
| 1 | 768 257 | 33.36657 | 4649.541 | ||
| 1 | 768 257 | 36.75427 | 5658.648 | ||
| 1 | 896 321 | 40.44652 | 6875.704 | ||
| 1 | 896 321 | 42.42917 | 7580.057 | ||
| 1 | 1024 321 | 48.94284 | 10145.79 | ||
| 1 | 1024 321 | 56.42926 | 13571.10 | ||
| 1 | 1024 321 | 59.13988 | 14935.81 | ||
| 1 | 1024 321 | 61.38714 | 16116.94 | ||
| 1 | 1024 321 | 65.06338 | 18145.61 | ||
| 1 | 1024 321 | 67.42466 | 19511.79 | ||
| 1 | 1024 321 | 68.96487 | 20429.24 | ||
| 1 | 1024 321 | 71.58241 | 22019.93 | ||
| 1 | 1024 321 | 75.09725 | 24262.22 | ||
| 1 | 1024 321 | 86.68318 | 32430.06 | ||
| 1 | 1024 321 | 100.0909 | 43339.02 | ||
| 1 | 1024 321 | 103.0738 | 45995.60 | ||
| 1 | 1024 321 | 104.9517 | 47705.08 |
| 1 | 3.116683 | 128 65 | 1.056697 | 1.619793 | |
| 1 | 3.123537 | 128 65 | 1.145870 | 2.683859 | |
| 1 | 3.128360 | 128 65 | 1.212070 | 3.318462 | |
| 1 | 3.143491 | 128 65 | 1.355411 | 4.550777 | |
| 1 | 3.161280 | 128 65 | 1.474516 | 5.535574 | |
| 1 | 3.180831 | 128 65 | 1.575828 | 6.387203 | |
| 1 | 3.202416 | 128 65 | 1.663668 | 7.152347 | |
| 1 | 3.216383 | 128 65 | 1.714937 | 7.614958 | |
| 1 | 3.247094 | 128 65 | 1.810118 | 8.512058 | |
| 1 | 3.292192 | 128 65 | 1.929322 | 9.719380 | |
| 1 | 3.329096 | 128 65 | 2.029942 | 10.82473 | |
| 1 | 3.378413 | 128 65 | 2.117243 | 11.84892 | |
| 1 | 3.426419 | 128 65 | 2.212421 | 13.04623 | |
| 1 | 3.575467 | 128 65 | 2.492199 | 17.05494 | |
| 1 | 3.665236 | 128 65 | 2.671348 | 19.99537 | |
| 1 | 3.880392 | 128 65 | 3.171063 | 29.49222 | |
| 1 | 4.118841 | 128 65 | 3.757873 | 42.57254 | |
| 1 | 4.419469 | 128 89 | 4.454688 | 60.44520 | |
| 1 | 4.793529 | 128 89 | 5.278963 | 84.69567 | |
| 1 | 5.242992 | 128 129 | 6.252782 | 117.4323 | |
| 1 | 5.782949 | 128 129 | 7.404680 | 161.3367 | |
| 1 | 6.420133 | 128 129 | 8.769978 | 219.8304 | |
| 1 | 7.171170 | 128 129 | 10.39171 | 297.1691 | |
| 1 | 8.051634 | 128 129 | 12.32188 | 398.7272 | |
| 1 | 9.073939 | 128 129 | 14.62274 | 531.4357 | |
| 1 | 9.997275 | 192 257 | 16.76769 | 665.2339 | |
| 1 | 10.25059 | 192 257 | 17.36827 | 704.3108 | |
| 1 | 11.59439 | 192 257 | 20.64616 | 929.1624 | |
| 1 | 13.12083 | 192 257 | 24.56044 | 1221.405 | |
| 1 | 14.68186 | 256 257 | 28.77198 | 1562.064 | |
| 1 | 14.84741 | 256 257 | 29.23512 | 1601.174 | |
| 1 | 16.80072 | 256 257 | 34.81847 | 2094.287 | |
| 1 | 18.99815 | 256 321 | 41.48855 | 2735.227 | |
| 1 | 21.45545 | 256 321 | 49.46027 | 3569.756 | |
| 1 | 23.89666 | 256 321 | 58.05030 | 4550.127 | |
| 1 | 24.15059 | 256 321 | 58.99612 | 4663.393 | |
| 1 | 26.84021 | 256 321 | 70.43089 | 6139.224 | |
| 1 | 27.08270 | 256 321 | 71.85714 | 6344.418 | |
| 1 | 27.31152 | 256 321 | 73.66207 | 6619.142 | |
| 1 | 27.35757 | 256 321 | 75.37932 | 6911.933 | |
| 1 | 26.83143 | 256 321 | 77.02411 | 7294.891 | |
| 1 | 26.28179 | 256 321 | 78.61210 | 7683.031 | |
| 1 | 26.25586 | 256 321 | 80.14209 | 7971.925 | |
| 1 | 26.36825 | 256 321 | 81.61573 | 8227.378 | |
| 1 | 26.54403 | 256 321 | 83.03697 | 8462.830 | |
| 1 | 26.73696 | 256 321 | 84.40976 | 8687.394 | |
| 1 | 29.78702 | 512 449 | 101.5246 | 11462.12 | |
| 1 | 33.65968 | 512 449 | 122.1559 | 14978.49 | |
| 1 | 38.04901 | 512 449 | 146.9986 | 19554.08 | |
| 1 | 42.83017 | 512 449 | 176.9293 | 25585.09 | |
| 1 | 48.06231 | 512 449 | 213.0247 | 33536.66 | |
| 1 | 53.90331 | 512 449 | 256.5802 | 43967.29 | |
| 1 | 60.50367 | 512 513 | 309.1454 | 57597.49 | |
| 1 | 67.95755 | 512 513 | 372.5844 | 75402.24 | |
| 1 | 76.33729 | 512 769 | 449.1508 | 98685.64 | |
| 1 | 85.71701 | 512 897 | 541.5753 | 129175.9 | |
| 1 | 96.12138 | 512 897 | 653.1727 | 169237.3 | |
| 1 | 107.6085 | 512 897 | 787.9764 | 221995.2 | |
| 1 | 120.1234 | 512 897 | 950.9070 | 291852.5 | |
| 1 | 133.7508 | 512 897 | 1147.971 | 384498.9 | |
| 1 | 148.8836 | 512 1025 | 1386.450 | 506738.9 | |
| 1 | 166.3042 | 512 1025 | 1675.036 | 666086.2 | |
| 1 | 186.3974 | 512 1025 | 2024.094 | 873176.4 | |
| 1 | 209.4395 | 512 1281 | 2446.172 | 1142290 | |
| 1 | 235.2120 | 512 1537 | 2956.470 | 1494811 | |
| 1 | 263.0987 | 512 1793 | 3573.640 | 1962459 |
| 1 | 14.09456 | 96 129 | 5.864201 | 82.92705 | |
| 1 | 16.41959 | 96 129 | 6.914669 | 105.6969 | |
| 1 | 18.89401 | 96 129 | 8.148261 | 135.6083 | |
| 1 | 21.66773 | 96 129 | 9.587445 | 173.8004 | |
| 1 | 24.88046 | 96 129 | 11.26803 | 221.7384 | |
| 1 | 27.86575 | 96 129 | 12.81012 | 267.8677 | |
| 1 | 28.70377 | 96 129 | 13.23878 | 280.9653 | |
| 1 | 33.19466 | 96 129 | 15.56038 | 354.6158 | |
| 1 | 38.29704 | 96 129 | 18.29931 | 448.0501 | |
| 1 | 43.50700 | 96 193 | 21.21050 | 554.8653 | |
| 1 | 44.06680 | 96 193 | 21.52859 | 566.9565 | |
| 1 | 50.67686 | 96 193 | 25.33533 | 717.1889 | |
| 1 | 58.31124 | 96 193 | 29.82540 | 906.1212 | |
| 1 | 67.11615 | 128 321 | 35.12519 | 1143.903 | |
| 1 | 76.25123 | 128 321 | 40.76618 | 1413.329 | |
| 1 | 77.23740 | 128 321 | 41.38311 | 1443.747 | |
| 1 | 88.88239 | 128 321 | 48.77387 | 1821.661 | |
| 1 | 102.3071 | 128 321 | 57.50461 | 2297.406 | |
| 1 | 117.7822 | 128 449 | 67.82060 | 2896.298 | |
| 1 | 135.6225 | 128 449 | 80.01178 | 3650.131 | |
| 1 | 156.1963 | 128 449 | 94.42106 | 4598.740 | |
| 1 | 179.9312 | 128 449 | 111.4542 | 5792.111 | |
| 1 | 207.3119 | 128 449 | 131.5910 | 7293.324 | |
| 1 | 238.9044 | 128 449 | 155.3995 | 9181.462 | |
| 1 | 275.3612 | 128 449 | 183.5515 | 11555.93 | |
| 1 | 317.4310 | 128 449 | 216.8420 | 14541.83 | |
| 1 | 365.9813 | 128 641 | 256.2116 | 18296.30 | |
| 1 | 422.0132 | 128 641 | 302.7737 | 23016.84 | |
| 1 | 486.6804 | 128 641 | 357.8457 | 28951.78 | |
| 1 | 561.6657 | 128 641 | 422.9866 | 36390.82 | |
| 1 | 647.4534 | 128 641 | 500.0423 | 45793.62 | |
| 1 | 746.8566 | 128 641 | 591.1965 | 57587.15 | |
| 1 | 861.4431 | 128 769 | 699.0342 | 72424.84 | |
| 1 | 994.2189 | 128 769 | 826.6155 | 91031.10 | |
| 1 | 1147.050 | 128 769 | 977.5620 | 114458.7 | |
| 1 | 1323.461 | 128 1025 | 1156.161 | 143907.6 | |
| 1 | 1527.004 | 128 1025 | 1367.486 | 180935.2 | |
| 1 | 1762.395 | 128 1025 | 1617.546 | 227422.7 |
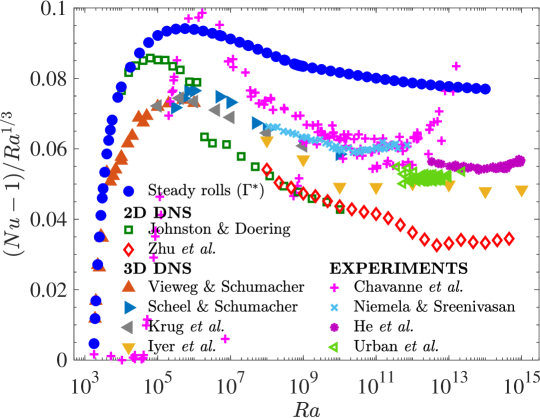
Comparison with turbulent convection
Figure 1S is nearly identical to figure 5 in the main text, comparing heat transport by -maximizing steady rolls with transport by turbulent convection, except that more experimental data with Prandtl numbers further from 1 are included. In figure 1S the criterion for inclusion is an estimated Prandtl number of rather than the range in figure 5 of the main text. (In fact all of the estimated are at least 0.6, so in figure 1S.) The working fluids in the experiments—gaseous helium or sulfur hexaflouride—are used near their critical points, leading to coupling and sensitive variation of material parameters that can be difficult to estimate. Faster variation of with is associated with increasing non-Oberbeck–Boussinesq effects as well; see Urban et al. (2011, 2012, 2014) for a discussion of experimental challenges. Data in figure 5 is truncated using the narrower range mainly to reduce non-Oberbeck–Boussinesq effects—we expect alone to have a more modest effect, even over the wider range .
References
- Chavanne et al. (2001) Chavanne, X., Chilla, F., Chabaud, B., Castaing, B. & Hebral, B. 2001 Turbulent Rayleigh–Bénard convection in gaseous and liquid He. Physics of Fluids 13, 1300–1320.
- Chillà & Schumacher (2012) Chillà, F. & Schumacher, J. 2012 New perspectives in turbulent Rayleigh–Bénard convection. The European Physical Journal E 35, 58.
- Chini & Cox (2009) Chini, G.P. & Cox, S.M. 2009 Large Rayleigh number thermal convection: Heat flux predictions and strongly nonlinear solutions. Physics of Fluids 21, 083603.
- Doering (2020) Doering, C.R. 2020 Turning up the heat in turbulent thermal convection. Proceedings of the National Academy of Sciences USA 117, 9671–9673.
- Doering & Constantin (1996) Doering, C.R. & Constantin, P. 1996 Variational bounds on energy dissipation in incompressible flows. III. Convection. Phys. Rev. E 53, 5957–5981.
- Doering et al. (2006) Doering, C.R., Otto, F. & Reznikoff, M.G. 2006 Bounds on vertical heat transport for infinite-Prandtl-number Rayleigh–Bénard convection. J. Fluid Mech. 560, 229–241.
- Gibson et al. (2008) Gibson, J.F., Halcrow, J. & Cvitanović, P. 2008 Visualizing the geometry of state space in plane Couette flow. Journal of Fluid Mechanics 611, 107–130.
- Graham & Floryan (2021) Graham, M.D. & Floryan, D. 2021 Exact coherent states and the nonlinear dynamics of wall-bounded turbulent flows. Annual Review of Fluid Mechanics 53, 227–253.
- He et al. (2012) He, X., Funfschilling, D., Nobach, H., Bodenschatz, E. & Ahlers, G. 2012 Transition to the ultimate state of turbulent Rayleigh–Bénard convection. Physical Review Letters 108, 024502.
- Howard (1963) Howard, L.N. 1963 Heat transport by turbulent convection. Journal of Fluid Mechanics 17, 405–432.
- Iyer et al. (2020) Iyer, K.P., Scheel, J.D., Schumacher, J. & Sreenivasan, K.R. 2020 Classical 1/3 scaling of convection holds up to Ra = 1015. Proceedings of the National Academy of Sciences USA 117, 7594–7598.
- Jeffreys (1928) Jeffreys, H. 1928 Some cases of instability in fluid motion. Proceedings of the Royal Society A 118, 195–208.
- Johnston & Doering (2009) Johnston, H. & Doering, C.R. 2009 Comparison of turbulent thermal convection between conditions of constant temperature and constant flux. Physical Review Letters 102, 064501.
- Kadanoff (2001) Kadanoff, L.P. 2001 Turbulent heat flow: Structures and scaling. Physics Today 54, 34–39.
- Kooloth et al. (2021) Kooloth, P., Sondak, D. & Smith, L.M. 2021 Coherent solutions and transition to turbulence in two-dimensional Rayleigh-Bénard convection. Phys. Rev. Fluids 6, 013501.
- Krug et al. (2020) Krug, D., Lohse, D. & Stevens, R.J.A.M. 2020 Coherence of temperature and velocity superstructures in turbulent Rayleigh–Bénard flow. J. Fluid Mech. 887, A2.
- Motoki et al. (2021) Motoki, S., Kawahara, G. & Shimizu, M. 2021 Multi-scale steady solution for Rayleigh–Bénard convection. Journal of Fluid Mechanics 914, A14.
- Nagata (1990) Nagata, M. 1990 Three-dimensional finite-amplitude solutions in plane couette flow: bifurcation from infinity. Journal of Fluid Mechanics 217, 519–527.
- Niemela & Sreenivasan (2006) Niemela, J.J. & Sreenivasan, K.R. 2006 Turbulent convection at high Rayleigh numbers and aspect ratio 4. Journal of Fluid Mechanics 557, 411–422.
- Olson et al. (2021) Olson, M.L., Goluskin, D., Schultz, W.W. & Doering, C.R. 2021 Heat transport bounds for a truncated model of Rayleigh–Bénard convection via polynomial optimization. Physica D 415, 132748.
- Otto & Seis (2011) Otto, F. & Seis, C. 2011 Rayleigh–Bénard convection: Improved bounds on the Nusselt number. J. Math. Phys. 52, 083702.
- Rayleigh (1916) Rayleigh, Lord 1916 On convection currents in a horizontal layer of fluid, when the higher temperature is on the under side. Philosophical Magazine 32, 529–546.
- Scheel & Schumacher (2017) Scheel, J.D. & Schumacher, J. 2017 Predicting transition ranges to fully turbulent viscous boundary layers in low Prandtl number convection flows. Physical Review Fluids 2, 123501.
- Sondak et al. (2015) Sondak, D., Smith, L.M. & Waleffe, F. 2015 Optimal heat transport solutions for Rayleigh–Bénard convection. Journal of Fluid Mechanics 784, 565–595.
- Suri et al. (2020) Suri, B., Kageorge, L., Grigoriev, R.O. & Schatz, M.F. 2020 Capturing turbulent dynamics and statistics in experiments with unstable periodic orbits. Physical Review Letters 125, 064501.
- Trefethen (2000) Trefethen, L.N. 2000 Spectral Methods in MATLAB. SIAM.
- Urban et al. (2012) Urban, P., Hanzelka, P., Kralik, T., Musilova, V. & Srnka, A .and Skrbek, L. 2012 Effect of boundary layers asymmetry on heat transfer efficiency in turbulent Rayleigh-Bénard convection at very high Rayleigh numbers. Physical Review Letters 109, 154301.
- Urban et al. (2014) Urban, P., Hanzelka, P., Musilová, V., Králík, T., La Mantia, M., Srnka, A. & Skrbek, L. 2014 Heat transfer in cryogenic helium gas by turbulent Rayleigh–Bénard convection in a cylindrical cell of aspect ratio 1. New Journal of Physics 16, 053042.
- Urban et al. (2011) Urban, P., Musilová, V. & Skrbek, L. 2011 Efficiency of heat transfer in turbulent Rayleigh-Bénard convection. Physical Review Letters 107, 014302.
- Vieweg & Schumacher (2020) Vieweg, P. & Schumacher, J. 2020 From 3D DNS with Pr and by P. Vieweg and J. Schumacher. Private Communication.
- Waleffe (1998) Waleffe, F. 1998 Three-dimensional coherent states in plane shear flows. Physical Review Letters 81, 4140.
- Waleffe (2020) Waleffe, F. 2020 Cases with the 6 largest are from computations of F. Waleffe. Private Communication.
- Waleffe et al. (2015) Waleffe, F., Boonkasame, A. & Smith, L.M. 2015 Heat transport by coherent Rayleigh–Bénard convection. Physics of Fluids 27, 051702.
- Wedin & Kerswell (2004) Wedin, H. & Kerswell, R.R. 2004 Exact coherent structures in pipe flow: travelling wave solutions. Journal of Fluid Mechanics 508, 333–371.
- Wen & Chini (2018) Wen, B. & Chini, G.P. 2018 Inclined porous medium convection at large Rayleigh number. Journal of Fluid Mechanics 837, 670–702.
- Wen et al. (2015) Wen, B., Corson, L.T. & Chini, G.P. 2015 Structure and stability of steady porous medium convection at large Rayleigh number. Journal of Fluid Mechanics 772, 197–224.
- Wen et al. (2020) Wen, B., Goluskin, D., LeDuc, M., Chini, G.P. & Doering, C.R. 2020 Steady Rayleigh–Bénard convection between stress-free boundaries. Journal of Fluid Mechanics 905, R4.
- Whitehead & Doering (2011) Whitehead, J.P. & Doering, C.R. 2011 Ultimate state of two-dimensional Rayleigh–Bénard convection between free-slip fixed-temperature boundaries. Phys. Rev. Lett. 106, 244501.
- Whitehead & Doering (2012) Whitehead, J.P. & Doering, C.R. 2012 Rigid bounds on heat transport by a fluid between slippery boundaries. J. Fluid Mech. 707, 241–259.
- Zhu et al. (2018) Zhu, X., Mathai, V., Stevens, R.J.A.M., Verzicco, R. & Lohse, D. 2018 Transition to the ultimate regime in two-dimensional Rayleigh–Bénard convection. Physical Review Letters 120, 144502.