Some rigidity results on compact hypersurfaces with capillary boundary in the hyperbolic space

Yimin Chen Department of Mathematics
Pusan National University
Busan 46241
Republic of Korea
sherlockpoe@pusan.ac.kr and Juncheol Pyo Department of Mathematics
Pusan National University
Busan 46241
Republic of Korea
jcpyo@pusan.ac.kr

Abstract.

In this paper, we prove a Heintze-Karcher type inequality for capillary hypersurfaces supported on various hypersurfaces in the hyperbolic space. The equality case only occurs on capillary totally umbilical hypersurfaces. Then we apply this result to prove the Alexandrov type theorem for embedded capillary hypersurfaces in the hyperbolic space. In addition, we prove some other rigidity results for capillary hypersurfaces supported on totally geodesic plane in $\mathbb{B}^{n+1}_{+}$ .

1. Introduction

Let $\mathbb{H}^{n+1}$ be the $(n+1)$ -dimensional hyperbolic space. We will use both the Poincaré ball model and the Poincaré half space model of $\mathbb{H}^{n+1}$ throughout this paper. Let $\delta$ be the Euclidean metric and $|\cdot|=\delta(\cdot,\cdot)^{1/2}$ be the Euclidean norm. The Poincaré ball model is defined as follows:

\displaystyle\left(\mathbb{B}^{n+1},\frac{4}{(1-|x|^{2})^{2}}\delta\right),

where $\mathbb{B}^{n+1}$ is the unit Euclidean ball centered at the origin.

The Poincaré half space model is defined as follows:

\displaystyle\left(\mathbb{R}^{n+1}_{+},\frac{1}{x_{n+1}^{2}}\delta\right),

where $\mathbb{R}^{n+1}_{+}$ is the half Euclidean space on which the last coordinate function $x_{n+1}$ is strictly positive.

Let $S$ be an umbilical hypersurface in $\mathbb{H}^{n+1}$ with its principal curvature $\lambda$ , and $\Sigma\hookrightarrow\mathbb{H}^{n+1}$ is an immersed $n$ -dimensional manifold into $\mathbb{H}^{n+1}$ such that $x(\partial\Sigma)\subset S$ . If $\Sigma$ intersects $S$ at a constant angle $\theta$ , $\Sigma$ is said to be a capillary hypersurface. In particular if $\theta=\frac{\pi}{2}$ , $\Sigma$ is said to be a free boundary hypersurface. Furthermore, we call $S$ the supporting hypersurface of the capillary hypersurface $\Sigma$ . The study of capillary hypersurfaces has a long history since the works by Young in [27] and Laplace in [12]. The notable result of Fraser and Schoen in [4] reveals the relation between the Steklov eigenvalue and the free boundary minimal hypersurfaces in the unit ball. Based on their significant work, properties of free boundary hypersurfaces have been established, including some geometric inequalities.

There are several results on geometric inequalities on capillary hypersurfaces in a space form. In [22] and [26], Scheuer, Wang, Weng and Xia have established a family of Alexandrov-Fenchel’s type inequalities on capillary hypersurfaces in a geodesic ball. In [24] and [7], the authors give a complete family of Alexandrov-Fenchel’s type inequalities on capillary hypersurfaces in Euclidean half space. And in [3], Chen, Hu and Li proved Perez type inequality on free boundary hypersurfaces in a geodesic ball.

In [21], Ros used Reilly’s formula (see [20]) to prove the following result.

Theorem A.

Let $\Omega$ be an $(n+1)$ -dimensional Riemannian manifold with boundary and non-negative Ricci curvature. Suppose the boundary $M=\partial\Omega$ is mean convex (i.e. the mean curvature $H_{M}>0$ ), then

\int_{M}\frac{1}{H_{M}}dA\geq(n+1)V(\Omega),

where $V(\Omega)$ denotes the volume of $\Omega$ . Moreover, the equality holds if and only if $\Omega$ is isometric to a Euclidean ball.

There are several results on (weighted) Heintze-Karcher type inequalities for embedded closed hypersurfaces in different Riemannian manifolds. In [2], Brendle proved a weighted Heintze-Karcher type inequality for embedded, closed mean convex hypersurfaces in a family of warped product spaces. In [19], Qiu and Xia proved a weighted Heintze-Karcher type inequality for the case in which the ambient space is a Riemannian manifold with a negative lower bound on its sectional curvature. In [13], Li and Xia proved a weighted Heintze-Karcher type inequality when the ambient space is a sub-static manifold.

For free boundary hypersurfaces, the second author proved a Heintze-Karcher type inequality for those supported on totally geodesics hyperplane in a space form in [18]. Guo and Xia proved the inequality for those supported on horospheres and equidistant hypersurfaces in [6]. In [25], Wang and Xia prove the case for those supported on geodesic balls.

Recently in the paper [10], Jia, Xia and Zhang proved a Heintze-Karcher type inequality for capillary hypersurfaces in Euclidean half space. Furthermore, they extended their results in Euclidean wedge and anisotropic half space (See [8] and [9]). Inspired by their result, we will prove a Heintze-Karcher type inequality for capillary hypersurfaces in $\mathbb{H}^{n+1}$ .

In Poincaré ball model $(\mathbb{B}^{n+1},\frac{4}{(1-|x|^{2})^{2}}\delta)$ , let $\Sigma$ be a compact, embedded capillary hypersurface in

\mathbb{B}^{n+1}_{+}=\{x\in\mathbb{B}^{n+1}:\delta(x,E_{n+1})\geq 0\}

supported on a totally geodesic plane

P=\{x\in\mathbb{B}^{n+1}:\delta(x,E_{n+1})=0\}.

Denote $\Omega$ the region enclosed by $\Sigma$ and $P$ , and $T\subset P$ the region in $P$ with $\partial\Omega=\Sigma\cup T$ . Denote $V_{0}=\frac{1+|x|^{2}}{1-|x|^{2}}$ , we have the following Heintze-Karcher type inequality.

Theorem 1.

Let $\Sigma\subset\mathbb{B}^{n+1}_{+}$ be a compact, embedded capillary hypersurface supported on $P$ . Let $\Omega$ be a domain enclosed by $\Sigma$ and $T\subset P$ . If the contact angle $\theta\in(0,\frac{\pi}{2}]$ , and the mean curvature $H_{1}>0$ on $\Sigma$ , we have

(1)

\displaystyle\int_{\Sigma}\frac{V_{0}}{H_{1}}dA\geq(n+1)\int_{\Omega}V_{0}d\Omega+n\cot\theta\frac{\left(\int_{T}V_{0}dA_{T}\right)^{2}}{\int_{\partial\Sigma}V_{0}ds}.

In addition, the equality holds if and only if $\Sigma$ is umbilical.

Next, we consider Poincaré half space model $(\mathbb{R}^{n+1}_{+},\frac{1}{x_{n+1}^{2}}\delta)$ of the hyperbolic space. Denote the position vector in Poincaré half space model by $\widetilde{x}$ . Fixing $a$ a vector field in $\mathbb{H}^{n+1}$ which is constant with respect to $(\mathbb{R}^{n+1},\delta_{+})$ and $0<\phi<\frac{\pi}{2}$ , we consider a umbilical hypersurface $L_{\phi,a}$ defined by

(2)

L_{\phi,a}=\{\widetilde{x}\in\mathbb{R}^{n+1}_{+}:x_{n+1}-1=\tan\phi x_{a}\},

where $x_{a}=\delta(\widetilde{x},a)$ and $a$ is a vector field in $\mathbb{H}^{n+1}$ which is constant with respect to $(\mathbb{R}^{n+1}_{+},\delta)$ and satisfies $\delta(a,E_{n+1})=0$ and $\delta(a,a)=1$ .It is well known that $L_{\phi,a}$ is a umbilical hypersurface with principal curvature $0<\cos\phi\leq 1$ . We define $B^{\mathrm{int}}_{\phi,a}$ as follows:

B^{\mathrm{int}}_{\phi,a}=\{\widetilde{x}\in\mathbb{H}^{n+1}:x_{n+1}-1\geq\tan\phi x_{a}\;\}.

In $B_{\phi}^{\mathrm{int}}$ , we consider compact embedded capillary hypersurfaces supported on $L_{\phi,a}$ .

Analogously, denote $V_{n+1}=\frac{1}{x_{n+1}}$ and we have the following Heintze-Karcher type inequality

Theorem 2.

Let $0\leq\phi<\frac{\pi}{2}$ and $\Sigma\subset B^{\mathrm{int}}_{\phi,a}$ be a compact, embedded capillary hypersurface. The supporting hypersurface is $L_{\phi}$ . Let $\Omega$ be the domain bounded by $\Sigma$ and $T\subset L_{\phi,a}$ . If the contact angle $\theta\in(0,\frac{\pi}{2}]$ , and the mean curvature $H_{1}>0$ on $\Sigma$ , we have

(3)

\displaystyle\int_{\Sigma}\frac{V_{n+1}}{H_{1}}dA\geq(n+1)\int_{\Omega}V_{n+1}d\Omega+n\cos\theta\frac{\left(\int_{T}V_{n+1}dA_{T}\right)^{2}}{\int_{\partial\Sigma}\overline{g}(-\cos\phi\widetilde{x}+\sin\phi a,\mu)ds}

where $\mu$ is the outward unit conormal vector field of $\partial\Sigma$ in $\Sigma$ . In addition, the equality holds if and only if $\Sigma$ is umbilical.

The classical Alexandrov type theorem says that the geodesic balls are the only closed embedded hypersurfaces with constant mean curvature (CMC) in space form. It has been proved using a method called moving planes (see [1]).

Another powerful tool to prove the Alexandrov type theorem is the method provided by Ros. In 1986, Ros used a Heintze-Karcher type formula in [21] to prove a higher-order version of an Alexandrov type theorem, which says that embedded hypersurfaces with constant $k$ -th mean curvature in Euclidean space can only be a sphere. For the general ambient space, Brendle [2] proved that any closed embedded CMC hypersurface in a family of space warped product spaces can only be a slice.

As we mentioned above, there exists certain Heintze-Karcher type inequalities on free boundary hypersurfaces on different supporting hypersurfaces, Wang and Xia [25] proved the Alexandrov type theorem for free boundary hypersurface supported on geodesic spheres in space forms. Moreover, for free boundary hypersurfaces supported on horospheres and equidistant hypersurfaces, we refer to Guo and Xia [6]. The second author [18] also studied the case of free boundary hypersurfaces supported on totally geodesic hyperplane in space forms. For capillary hypersurface, Jia, Xia and Zhang (see [10]) prove an Alexandrov type theorem for capillary hypersurfaces in the Euclidean half space and Euclidean ball. Jia, Wang, Xia and Zhang prove the capillary case of the support hypersurface being Euclidean wedges and the geodesic plane in anisotropic Euclidean half space (see [8] and [9]). In this paper, we will prove the following Alexandrov type theorems for capillary hypersurfaces in the hyperbolic space.

Theorem 3.

Let $\Sigma$ be a compact embedded CMC hypersurface in $\mathbb{B}^{n+1}_{+}$ supported on a the totally geodesic plane $P$ , and the contact angle satisfies $\theta\in(0,\frac{\pi}{2}]$ . Then $\Sigma$ is totally umbilical except for being totally geodesic.

Theorem 4.

Let $\Sigma$ be a compact embedded CMC capillary hypersurface in $B^{\mathrm{int}}_{\phi,a}$ supported on $L_{\phi,a}$ , and the contact angle satisfies $\theta\in(0,\frac{\pi}{2}]$ . In addition, we assume $\phi+\theta>\frac{\pi}{2}$ for $\phi>0$ or $\theta=\frac{\pi}{2}$ for $\phi=0$ . Then $\Sigma$ is umbilical except for being totally geodesic.

Remark.

The assumption “ $\phi+\theta>\frac{\pi}{2}$ for $\phi>0$ or $\theta=\frac{\pi}{2}$ for $\phi=0$ ” is only used for guaranteeing the existence of the convex point, which can be substituted by assuming that the constant mean curvature $H>0$ , see Corollary 1.

Intriguingly, we also prove some other rigidity results on immersed capillary hypersurfaces supported on a totally geodesic plane $P$ in the hyperbolic space. Koh and Lee [11] used the Minkowski identity for closed hypersurfaces in Euclidean space to classify the immersed hypersurfaces with constant ratio of higher-order mean curvatures in Euclidean space. Since we will give a Minkowski type formula on capillary hypersurfaces in the Section 3, we can prove the following result:

Theorem 5.

Let $\Sigma\subset\mathbb{B}^{n+1}_{+}$ be an immersed capillary hypersurface supported on $P$ . Suppose $k>l\geq 1$ . If there exists a constant $\alpha$ such that on $\Sigma$ ,

\displaystyle\frac{H_{k}}{H_{l}}=\alpha,\quad H_{l}>0,

we have that $\Sigma$ is totally umbilical except for being totally geodesic.

Remark.

We note that the second author proved the results in Theorem 1, Theorem 3 and Theorem 5 for the free boundary case in [18]. Guo and Xia proved the result in Theorem 2 and Theorem 4 for the free boundary case in [6].

Let $O\in\mathbb{H}^{n+1}$ be a point and $r(p)=\mbox{dist}(p,O)$ be the distance function in the hyperbolic space. The metric in the hyperbolic space can also be written as the following warped product form

\overline{g}=dr^{2}+\sinh rg_{S^{n}}.

It can be easily observed that the position vector field $x$ in Pioncaré ball model satisfies $x=\sinh r\partial r$ if we choose $O$ be the origin of $\mathbb{B}^{n+1}$ . A hypersurface $M$ in $\mathbb{H}^{n+1}$ is called star-shaped if $\overline{g}(x,\nu)=\overline{g}(\sinh r\partial r,\nu)>0$ . It has been proved that in some specific warped product manifolds (including the hyperbolic space), an immersed, compact, closed, star-shaped hypersurface with constant mean curvature must be a geodesic sphere (See [16, Corollary 5]). Now we give a version of capillary hypersurfaces in the hyperbolic space.

Theorem 6.

Let $\Sigma\hookrightarrow\mathbb{B}^{n+1}_{+}$ be a compact, immersed CMC capillary hypersurface supported on $P$ . If $\Sigma$ is star-shaped, $\Sigma$ is totally umbilical.

Since $x$ is embedding immediately if it is star-shaped, Theorem 3 implies Theorem 6 when $\theta\in(0,\frac{\pi}{2}]$ .

The remainder of this paper is organized as follows. In Section 2, we collect basic facts on the hyperbolic space. In Section 3, we prove Minkowski type formulae. In Section 4, we give proofs of Theorem 1 and Theorem 3. In Section 5, we give proofs of Theorem 2 and Theorem 4. In Section 6, we consider case of supporting hypersurfaces being geodesic spheres. Finally, in Section 7, we prove two other rigidity results for the capillary hypersurfaces supported on a totally geodesic plane in the hyperbolic space.

Acknowledgements.

This work is supported by the National Research Foundation of Korea (grant NRF No.2021R1A4A1032418). We would also like to thank Prof. Haizhong Li from Tsinghua University, Prof. Yingxiang Hu from Beihang University and Prof. Chao Xia from Xiamen University for their useful comments and constant supports.

2. Preliminaries

2.1. Capillary hypersurfaces and properties of the higher-order mean curvature $H_{r}$

Let $\overline{g}$ and $\overline{\nabla}$ be the hyperbolic metric and the Levi-Civita connection of $\mathbb{H}^{n+1}$ , respectively. Let $X:\Sigma\rightarrow\mathbb{\overline{}}B$ be an immersion of an $n$ -dimensional Riemannian manifold $\Sigma$ with boundary $\partial\Sigma$ into an $(n+1)$ -dimensional Riemannian manifold $B$ with boundary $\partial B$ . $\Sigma$ is called a capillary hypersurface in $B$ if the immersion satisfies the following

\displaystyle X(\mbox{int}(\Sigma))\subset\mbox{int}(B),\quad X(\partial\Sigma)\subset\partial B,

and $\Sigma$ and $\partial B$ intersects with a constant contact angle $\theta\in(0,\pi)$ along $\partial\Sigma$ . On $\partial\Sigma$ , there exists four unit normal vector fields, $\{\nu,\,\mu,\,\overline{N},\,\overline{\nu}\}$ , on a 2-dimensional subspace of $T_{p}\mathbb{H}^{n+1}$ as follows:

(i)

$\nu$ is the outward unit normal vector field of isometric immersion $X:\Sigma\rightarrow B$ .
(ii)

$\mu$ is the outward unit normal vector field of isometric immersion $y:\partial\Sigma\rightarrow\Sigma$ .
(iii)

$\overline{N}$ is the outward unit normal vector field of isometric immersion $z:\partial B\rightarrow B$ .
(iv)

$\overline{\nu}$ is the outward unit normal vector field of isometric immersion $w:\partial\Sigma\rightarrow\partial B$ .

The second fundamental form of $x:\Sigma\rightarrow\mathbb{H}^{n+1}$ denoted by $h$ is defined as follows:

\displaystyle h(Y,Z)=\overline{g}(\overline{\nabla}_{Y}\nu,Z),\mbox{ for }Y,\;Z\in T(\Sigma),

and the second fundamental form of $w:\partial\Sigma\rightarrow\partial B$ denoted by $h^{\partial\Sigma}$ is defined as follows:

\displaystyle h^{\partial\Sigma}(Y,Z)=\overline{g}(\overline{\nabla}_{Y}\overline{\nu},Z),\mbox{ for }Y,\;Z\in T(\partial\Sigma).

Figure 1. Capillary hypersurface supported on

\partial B

Now we have the following relation of these vector fields,

(6)

\displaystyle\left\{\begin{array}[]{l}\mu=\sin\theta\overline{N}+\cos\theta\overline{\nu},\\ \nu=-\cos\theta\overline{N}+\sin\theta\overline{\nu},\end{array}\right.

where $\theta$ denotes the angle between $\overline{N}$ and $-\nu$ . The following lemma is well-known and fundamental.

Lemma 1.

Let $X:\Sigma\rightarrow B\subset\mathbb{H}^{n+1}$ be an isometric immersion of a capillary hypersurface supported on the totally umbilical hypersurface $\partial B$ . Then $\mu$ is a principal direction of $\Sigma$ , that is,

(7)

\displaystyle\overline{\nabla}_{\mu}\nu=h(\mu,\mu)\mu.

Proof.

The proof can be found in [25, Proposition 2.1], we give a proof for completeness. Without loss of generality, let $\{e_{\alpha}\}$ be the orthonormal basis along $\partial\Sigma$ and $\alpha=1,2,\dots,n-1$ . Then, we have the following

	$\displaystyle\overline{\nabla}_{\mu}\nu=$	$\displaystyle\overline{g}(\overline{\nabla}_{\mu}\nu,\mu)\mu+\sum_{\alpha=1}^{n-1}\overline{g}(\overline{\nabla}_{\mu}\nu,e_{\alpha})e_{\alpha}$
	$\displaystyle=$	$\displaystyle h(\mu,\mu)\mu-\sum_{\alpha=1}^{n-1}\overline{g}(\overline{\nabla}_{e_{\alpha}}(-\cos\theta\overline{N}+\sin\theta\overline{\nu}),\sin\theta\overline{N}+\cos\theta\overline{\nu})e_{\alpha}$
	$\displaystyle=$	$\displaystyle h(\mu,\mu)\mu-h^{\partial\Sigma}(e_{\alpha},\overline{\nu})e_{\alpha}$
	$\displaystyle=$	$\displaystyle h(\mu,\mu)\mu,$

where the last equality holds because $\partial B$ is totally umbilical. ∎

Let $h_{ij}=h(e_{i},e_{j})$ and $\lambda=(\lambda_{1},\lambda_{2},\dots,\lambda_{n})$ be the eigenvalues of the matrix $(h_{ij})_{i,j=1}^{n}$ , that is, the principal curvatures of $\Sigma$ . Let $k\in\{1,2,\dots,n\}$ , the $k$ -th mean curvature is defined by

\displaystyle S_{k}=\frac{1}{k!}\sum\limits_{1\leq i_{1}<\cdots<i_{k}\leq n}\lambda_{1}\lambda_{2}\dots\lambda_{i_{k}},

and the normalized $k$ -th mean curvature is defined by $H_{k}=\binom{n}{k}^{-1}S_{k}$ . The associated Newton transformation is defined by induction,

\displaystyle T_{0}=I,

\displaystyle T_{k}=S_{k}I-T_{k-1}\circ h.

Using the induction formulae, we have the following properties.

\displaystyle\mbox{tr}(T_{k})=(n-k)S_{k}=(n-k)\binom{n}{k}H_{k},

\displaystyle\mbox{tr}(T_{k}\circ h)=\sum_{i,j=1}^{n}(T_{k})_{j}^{i}h_{i}^{j}=(k+1)S_{k+1}=(n-k)\binom{n}{k}H_{k+1}.

Let $\Gamma^{+}_{k}=\{\lambda\in\mathbb{R}^{n}:H_{i}>0,i=1,2,\dots,k\}$ . Then, for $1\leq l<k\leq n$ and $\lambda\in\Gamma^{+}_{l}$ , we have the following classic Newton-Maclaurin inequality,

(8)

\displaystyle\frac{H_{l}}{H_{l-1}}\geq\frac{H_{k}}{H_{k-1}}.

The equality in (8) holds if and only if $\lambda=c\mathcal{I}$ , where $c>0$ is a constant and $\mathcal{I}=(1,1,\dots,1)\in\mathbb{R}^{n}$ .

2.2. Properties in the Poincaré ball model

Let $x$ be the position vector in $\mathbb{B}^{n+1}$ . The following fact is well-known:

(9)

\displaystyle\overline{\nabla}x=V_{0}\overline{g},

where $V_{0}=\frac{1+|x|^{2}}{1-|x|^{2}}$ is a smooth function in $\mathbb{H}^{n+1}$ satisfying

\overline{\nabla}^{2}V_{0}=V_{0}\overline{g}.

A vector field $X$ is called a conformal Killing vector field in $(\overline{M},\overline{g})$ , if the Lie derivative $\mathcal{L}$ satisfies

\displaystyle\mathcal{L}_{X}\overline{g}=f\overline{g}.

In particular, $X$ is call a Killing vector if $f=0$ . In this case, the key Killing vector field $Y_{a}$ in Poincaré ball model we use is

\displaystyle Y_{a}=\frac{1}{2}(1+|x|^{2})a-\delta(x,a)x,

where $a$ is an arbitrary vector field in $\mathbb{H}^{n+1}$ which is constant with respect to $(\mathbb{B}^{n+1},\delta)$ . It is given by Wang and Xia in [25].

Let $E_{1},E_{2},\dots,E_{n+1}$ form the coordinate frame in the conformal Euclidean metric, and the corresponding normalized vectors $\overline{E}_{1},\overline{E}_{2},\dots,\overline{E}_{n+1}$ form an orthonormal frame on $(\mathbb{B}^{n+1},\overline{g})$ . For $A,B\in\{1,2,\dots,n+1\}$ , it holds that

(10)

\displaystyle\frac{1}{2}\left(\overline{g}(\overline{\nabla}_{\overline{E}_{A}}Y_{a},\overline{E}_{B})+\overline{g}(\overline{\nabla}_{\overline{E}_{B}}Y_{a},\overline{E}_{A})\right)=0.

This can be verified by the following properties.

Proposition 1 (See [25]).

Let $a=\sum\limits_{i=1}^{n+1}a_{i}E_{i}$ be an arbitrary vector field satisfying that $a_{i}$ are constant and $\delta(a,E_{n+1})=0$ . Denote the normalized vector of $a$ and $E_{n+1}$ in $(\mathbb{B}^{n+1}_{+},\frac{4}{(1-|x|^{2})^{2}}\delta)$ by $\bar{a}$ and $\bar{E}_{n+1}$ respectively, that is, $\bar{a}=\frac{1-|x|^{2}}{2}a$ and $\bar{E}_{n+1}=\frac{1-|x|^{2}}{2}E_{n+1}$ . Then, for any $Z\in T\mathbb{H}^{n+1}$ , we have the following:

(a)

$\overline{\nabla}_{Z}a=\overline{g}(Z,x)\bar{a}+\overline{g}(\bar{a},x)Z-\overline{g}(Z,\bar{a})x$ ,
(b)

$\overline{\nabla}_{Z}(\frac{1-|x|^{2}}{2}a)=\frac{1-|x|^{2}}{2}\left[\overline{g}(x,\bar{a})Z-\overline{g}(Z,\bar{a})x\right]$ ,
(c)

$\overline{\nabla}_{Z}V_{0}=\overline{g}(x,Z)$ ,
(d)

$\overline{\nabla}_{Z}Y_{a}=\overline{g}(x,Z)\bar{a}-\overline{g}(Z,\bar{a})x$ .

2.3. Properties in the Poincaré half space model

In Poincaré half space model $\mathbb{H}^{n+1}=(\mathbb{R}^{n+1}_{+},\frac{1}{x_{n+1}^{2}}\delta)$ , we denote $\widetilde{x}$ the position vector field in $\mathbb{R}^{n+1}_{+}$ .

Let $E_{1},E_{2},\dots,E_{n+1}$ form the coordinate frame in the Euclidean metric, and the normalized vectors $\overline{E}_{1},\overline{E}_{2},\dots,\overline{E}_{n+1}$ form an orthonormal frame on $(\mathbb{R}^{n+1}_{+},\overline{g})$ . A conformal Killing vector field given by

X_{n+1}:=\widetilde{x}-E_{n+1}

plays an important role in [6] and [5]. For $A,B\in\{1,2,\dots,n+1\}$ , it holds that

(11)

\displaystyle\frac{1}{2}\left(\overline{g}(\overline{\nabla}_{\overline{E}_{A}}X_{n+1},\overline{E}_{B})+\overline{g}(\overline{\nabla}_{\overline{E}_{B}}X_{n+1},\overline{E}_{A})\right)=V_{n+1}\overline{g},

where $V_{n+1}=\frac{1}{x_{n+1}}$ is a smooth function satisfying

\overline{\nabla}^{2}V_{n+1}=V_{n+1}\overline{g}.

Similarly, in the Poincaré half space model, we have the following proposition, the proof of which is omitted.

Proposition 2 (See [5]).

Let $a=\sum\limits_{i=1}^{n+1}a_{i}E_{i}$ be an arbitrary vector field satisfying that $a_{i}$ are constant and $\delta(a,E_{n+1})=0$ . Denote the normalized vector of $a$ and $E_{n+1}$ in $(\mathbb{R}^{n+1}_{+},\frac{1}{x_{n+1}^{2}}\delta)$ by $\bar{a}$ and $\bar{E}_{n+1}$ respectively, that is, $\bar{a}=x_{n+1}a$ and $\bar{E}_{n+1}=x_{n+1}E_{n+1}$ . Then, for any $Y\in T\mathbb{H}^{n+1}$ , we have the following:

(a)

$\overline{\nabla}_{Y}\widetilde{x}=-\overline{g}(Y,\overline{E}_{n+1})\widetilde{x}+\overline{g}(Y,\widetilde{x})\overline{E}_{n+1}$ ,
(b)

$\overline{\nabla}_{Y}a=-\overline{g}(Y,\overline{E}_{n+1})\overline{a}+\overline{g}(Y,\overline{a})E_{n+1}$ ,
(c)

$\overline{\nabla}_{Y}E_{n+1}=-\frac{1}{x_{n+1}}Y$ ,
(d)

$\overline{\nabla}_{Y}\overline{E}_{n+1}=\overline{g}(\overline{E}_{n+1},Y)\overline{E}_{n+1}-Y$ .

It is easy to see that (11) can be obtained from (a) and (c) in Proposition 2. Moreover, from (a) and (b) in Proposition 2, we have the following:

(12)

\displaystyle\frac{1}{2}\left(\overline{g}(\overline{\nabla}_{\overline{E}_{A}}a,\overline{E}_{B})+\overline{g}(\overline{\nabla}_{\overline{E}_{B}}a,\overline{E}_{A})\right)=0,

and

(13)

\displaystyle\frac{1}{2}\left(\overline{g}(\overline{\nabla}_{\overline{E}_{A}}\widetilde{x},\overline{E}_{B})+\overline{g}(\overline{\nabla}_{\overline{E}_{B}}\widetilde{x},\overline{E}_{A})\right)=0,

that is, $a$ and $\widetilde{x}$ are Killing vector fields in $(\mathbb{R}^{n+1}_{+},\frac{1}{x_{n+1}^{2}}\delta)$ .

3. Minkowski type formulae in the hyperbolic space

In [25], the authors give a Minkowski type formula for capillary hypersurface $M$ in a geodesic ball of radius $R$ in a space form $\mathbb{M}^{n+1}(K)$ ( $K=0,\,-1,\,1$ ),

\displaystyle\int_{M}\left(n(V_{a}+\mbox{sn}_{K}(R)\cos\theta\overline{g}(Y_{a},\nu))-S_{1}\overline{g}(X_{a},\nu)\right)dA=0,

where

\displaystyle\mbox{sn}_{K}(r)=\left\{\begin{array}[]{ll}r,&\mbox{ when }K=0;\\ \sin r,&\mbox{ when }K=1;\\ \sinh r,&\mbox{ when }K=-1,\\ \end{array}\right.

and $X_{a}$ is a conformal Killing vector field tangent to the geodesic sphere of radius $R$ , which is the supporting hypersurface of $M$ . We introduce our results on the case (supported on geodesic sphere in $\mathbb{H}^{n+1}$ ) in the Section 6.

3.1. A Minkowski type formula for capillary hypersurfaces in Poincaré ball model

In $\mathbb{B}^{n+1}_{+}$ , the outer unit normal vector field along $P$ is $\overline{N}=-\frac{1-|x|^{2}}{2}E_{n+1}$ . We note that the position vector field $x$ in the Poincaré ball model is tangent to $P$ , therefore $\overline{g}(x,\overline{N})=0$ .

Figure 2. Capillary hypersurface

\Sigma

supported on a totally geodesic plane

P

Let $Y_{n+1}=Y_{E_{n+1}}=\frac{1}{2}(1+|x|^{2})E_{n+1}-\delta(x,E_{n+1})x$ and $E_{n+1}$ be the unit constant vector with respect to $\delta$ . Then, we can derive a Minkowski type formula as follows.

Proposition 3.

Let $x:\Sigma\subset\mathbb{B}^{n+1}_{+}$ be a compact, immersed capillary hypersurface supported on $P$ , intersecting $P$ at a constant angle $\theta$ . For $k\in\{1,2,\dots,n\}$ , we have

(14)

\displaystyle\int_{\Sigma}\left[H_{k-1}(V_{0}-\cos\theta\overline{g}(Y_{n+1},\nu))-H_{k}\overline{g}(x,\nu)\right]dA=0.

Proof.

Restricting (9) to $\Sigma$ and let $\{e_{i}\}_{i=1}^{n}$ be the orthonormal frame on $\Sigma$ , we have

(15)

\displaystyle\overline{g}(\nabla_{e_{i}}x^{T},e_{j})=V_{0}\delta_{ij}-h_{ij}\overline{g}(x,\nu),

where $\nabla$ is the Levi-Civita connection of $(\Sigma,g)$ . Applying the Newton transformation $T_{k-1}$ on both sides of (15), we get

(16)		$\displaystyle(n-k+1)\binom{n}{k-1}\int_{\Sigma}V_{0}H_{k-1}-H_{k}\overline{g}(x,\nu)dA$
	$\displaystyle=$	$\displaystyle\int_{\Sigma}\mbox{div}_{\Sigma}(T_{k-1}(x^{T}))dA$
	$\displaystyle=$	$\displaystyle\int_{\partial\Sigma}T_{k-1}(x^{T},\mu)ds$
	$\displaystyle=$	$\displaystyle\cos\theta\int_{\partial\Sigma}S_{k-1;\mu}\overline{g}(x,\overline{\nu})ds.$

In the last equality, we use (6) and the fact that $\mu$ is a principal direction of $\Sigma$ and $\overline{g}(x,\overline{N})=0$ . Therefore, $T_{k-1}(x^{T},\mu)=S_{k-1;\mu}\overline{g}(x,\mu)=\cos\theta S_{k-1;\mu}\overline{g}(x,\overline{\nu})$ where $S_{k-1;\mu}$ is given by

\displaystyle S_{k-1;\mu}=\sum_{\begin{subarray}{c}1\leq\alpha_{1}<\dots<\alpha_{k-1}\leq n-1\\ \mu\notin\{e_{\alpha_{1}},\dots,e_{\alpha_{k-1}}\}\end{subarray}}\lambda_{\alpha_{1}}\lambda_{\alpha_{2}}\cdots\lambda_{\alpha_{k-1}}.

On the other hand, let $U_{n+1}=\overline{g}(\nu,e^{-u}E_{n+1})x-\overline{g}(x,\nu)e^{-u}E_{n+1}$ be a tangent vector field on $\Sigma$ . We can easily see that $\overline{g}(U_{n+1},\nu)=0$ along $\Sigma$ . Then using Proposition 1, we have

(17)	$\displaystyle\overline{g}(\nabla_{e_{i}}U_{n+1},e_{j})=$	$\displaystyle\overline{g}\left(\overline{\nabla}_{e_{i}}\left(\overline{g}(\nu,e^{-u}E_{n+1})x^{T}-\overline{g}(x,\nu)(e^{-u}E_{n+1})^{T}\right),e_{j}\right)$
	$\displaystyle=$	$\displaystyle-e^{-u}\overline{g}(x,\nu)\overline{g}(e^{-u}E_{n+1},e_{i})\overline{g}(x,e_{j})+\overline{g}(e^{-u}E_{n+1},\nu)(V_{0}\delta_{ij}$
		$\displaystyle-h_{ij}\overline{g}(x,\nu))-\overline{g}(x,\nu)\big{[}e^{-u}(\delta_{ij}\overline{g}(x,e^{-u}E_{n+1})$
		$\displaystyle-\overline{g}(e^{-u}E_{n+1},e_{i})\overline{g}(x,e_{j})-h_{ij}\overline{g}(\nu,e^{-u}E_{n+1})\big{]}$
	$\displaystyle=$	$\displaystyle\left(\overline{g}(e^{-u}E_{n+1},\nu)V_{0}-e^{-u}\overline{g}(x,\nu)\overline{g}(x,e^{-u}E_{n+1})\right)\delta_{ij}$
	$\displaystyle=$	$\displaystyle\overline{g}(Y_{n+1},\nu)\delta_{ij},$

where we use the fact that $e^{-u}V_{0}E_{n+1}-\langle x,E_{n+1}\rangle x=Y_{n+1}$ . Again, applying the Newton transformation $T_{k-1}$ on both sides of (17) and integrating on $\Sigma$ , we have

(18)		$\displaystyle(n-k+1)\binom{n}{k-1}\int_{\Sigma}H_{k-1}\overline{g}(Y_{n+1},\nu)dA$
	$\displaystyle=$	$\displaystyle\int_{\Sigma}(T_{k-1})_{ij}\overline{g}(\nabla_{e_{i}}U_{n+1},e_{j})dA=\int_{\partial\Sigma}T_{k-1}(U_{n+1},\mu)ds$
	$\displaystyle=$	$\displaystyle\int_{\partial\Sigma}S_{k-1;\mu}\overline{g}(U_{n+1},\mu)ds$
	$\displaystyle=$	$\displaystyle\int_{\partial\Sigma}S_{k-1;\mu}\overline{g}(x,\overline{\nu})ds,$

where in the last equality, we use (6) on $\partial\Sigma$ to obtain

	$\displaystyle\overline{g}(U_{n+1},\mu)=$	$\displaystyle\overline{g}(\nu,e^{-u}E_{n+1})\overline{g}(x,\mu)-\overline{g}(x,\nu)\overline{g}(e^{-u}E_{n+1},\mu)$
	$\displaystyle=$	$\displaystyle\left(\cos^{2}\theta\overline{g}(x,\overline{\nu})+\sin^{2}\theta\overline{g}(x,\overline{\nu})\right)=\overline{g}(x,\overline{\nu}).$

Combining (16) and (18), we obtain the Minkowski type formula (14).

∎

3.2. A Minkowski type formula for capillary hypersurfaces in Poincaré half space model

In Poincaré half space model, the inner normal vector field along $L_{\phi}$ is given by

(19)

\overline{N}=x_{n+1}(-\cos\phi E_{n+1}+\sin\phi a).

Similarly, it can be easily to observe that the conformal Killing vector field $X_{n+1}=\widetilde{x}-E_{n+1}$ is tangential to $L_{\phi}$ . Indeed, we have

(20)	$\displaystyle\overline{g}(X_{n+1},\overline{N})=$	$\displaystyle x_{n+1}\overline{g}(\widetilde{x}-E_{n+1},-\cos\phi E_{n+1}+\sin\phi a)$
	$\displaystyle=$	$\displaystyle-\cos\phi+\sin\phi\frac{x_{a}}{x_{n+1}}+\frac{\cos\phi}{x_{n+1}}$
	$\displaystyle=$	$\displaystyle 0,$

where in the last equality we use (2).

Figure 3. Capillary hypersurface

\Sigma

supported on an equidistant hypersurface

L_{\phi}

Thus, we have the following Minkowski type formula analogously.

Proposition 4.

Let $x:\Sigma\subset B^{\mathrm{int}}_{\phi,a}$ be a compact, immersed capillary hypersurface supported on $L_{\phi,a}$ , intersecting $L_{\phi,a}$ at a constant angle $\theta$ . For $k\in\{1,2,\dots,n\}$ , we have

(21)

\int_{\Sigma}[H_{k-1}(V_{n+1}-\cos\theta\sec\phi\overline{g}(\widetilde{x},\nu))-H_{k}\overline{g}(X_{n+1},\nu)]dA=0.

Proof.

As the proof of Proposition 3, restricting (11) to $\Sigma$ and using the divergence theorem, we have

(22)		$\displaystyle(n-k+1)\binom{n}{k-1}\int_{\Sigma}V_{n+1}H_{k-1}-H_{k}\overline{g}(X_{n+1},\nu)dA$
	$\displaystyle=$	$\displaystyle\int_{\partial\Sigma}T_{k-1}(X_{n+1},\mu)ds$
	$\displaystyle=$	$\displaystyle\cos\theta\int_{\partial\Sigma}S_{k-1;\mu}\overline{g}(X_{n+1},\overline{\nu})ds,$

where in the last equality we use (6), (20) and the fact that $\mu$ is a principal direction of $\Sigma$ along $\partial\Sigma$ .

On the other hand, consider a vector field $Z_{n+1}=\overline{g}(x,\nu)\overline{E}_{n+1}-\overline{g}(\overline{E}_{n+1},\nu)x$ . By a direct computation, we have

		$\displaystyle\overline{g}(\nabla_{e_{i}}Z_{n+1},e_{j})$
	$\displaystyle=$	$\displaystyle-\overline{g}(e_{i},\overline{E}_{n+1})\overline{g}(\widetilde{x},\nu)\overline{g}(\overline{E}_{n+1},e_{j})+\overline{g}(\widetilde{x},e_{i})\overline{g}(\overline{E}_{n+1},\nu)\overline{g}(\overline{E}_{n+1},e_{j})$
		$\displaystyle+h_{ik}\overline{g}(\widetilde{x},e_{k})\overline{g}(\overline{E}_{n+1},e_{j})+\overline{g}(\widetilde{x},\nu)(\overline{g}(\overline{E}_{n+1},e_{i})\overline{g}(\overline{E}_{n+1},e_{j})-\delta_{ij})$
		$\displaystyle-\overline{g}(\overline{E}_{n+1},e_{i})\overline{g}(\overline{E}_{n+1},\nu)\overline{g}(\widetilde{x},e_{j})-h_{ik}\overline{g}(\overline{E}_{n+1},e_{k})\overline{g}(\widetilde{x},e_{j})$
		$\displaystyle-\overline{g}(\overline{E}_{n+1},\nu)\left[-\overline{g}(e_{i},\overline{E}_{n+1})\overline{g}(\widetilde{x},e_{j})+\overline{g}(\widetilde{x},e_{j})\overline{g}(\overline{E}_{n+1},e_{i})\right]$
	$\displaystyle=$	$\displaystyle-\delta_{ij}\overline{g}(\widetilde{x},\nu)+\overline{g}(\overline{E}_{n+1},\nu)\left(\overline{g}(\widetilde{x},e_{i})\overline{g}(\overline{E}_{n+1},e_{j})-\overline{g}(\overline{E}_{n+1},e_{i})\overline{g}(\widetilde{x},e_{j})\right).$

Therefore,

(23)

\displaystyle\frac{1}{2}\left(\overline{g}(\nabla_{e_{i}}Z_{n+1},e_{j})+\overline{g}(\nabla_{e_{j}}Z_{n+1},e_{i})\right)=-\overline{g}(\widetilde{x},\nu)\delta_{ij}.

Using the divergence Theorem, (6), (7) and (19), we have

(24)		$\displaystyle-(n-k+1)\binom{n}{k-1}\int_{\Sigma}H_{k-1}\overline{g}(\widetilde{x},\nu)dA$
	$\displaystyle=$	$\displaystyle\int_{\partial\Sigma}T_{k-1}(Z_{n+1},\mu)ds$
	$\displaystyle=$	$\displaystyle\int_{\partial\Sigma}S_{k-1;\mu}\overline{g}(Z_{n+1},\mu)ds$
	$\displaystyle=$	$\displaystyle\int_{\partial\Sigma}S_{k-1;\mu}\left[\overline{g}(\widetilde{x},-\cos\theta\overline{N}+\sin\theta\overline{\nu})\overline{g}(\overline{E}_{n+1},\sin\theta\overline{N}+\cos\theta\overline{\nu})\right.$
		$\displaystyle-\left.\overline{g}(\overline{E}_{n+1},-\cos\theta\overline{N}+\sin\theta\overline{\nu})\overline{g}(\widetilde{x},\sin\theta\overline{N}+\cos\theta\overline{\nu})\right]ds$
	$\displaystyle=$	$\displaystyle-\int_{\partial\Sigma}S_{k-1;\mu}\left[(-\cos\phi+\sin\phi\frac{x_{a}}{x_{n+1}})\overline{g}(\overline{E}_{n+1},\overline{\nu})+\cos\phi\overline{g}(\widetilde{x},\overline{\nu})\right]ds$
	$\displaystyle=$	$\displaystyle-\int_{\partial\Sigma}\cos\phi S_{k-1;\mu}\overline{g}(X_{n+1},\overline{\nu})ds,$

where the last equality we use the fact from (2) that on $\partial\Sigma\subset L_{\phi,a}$ , $x_{n+1}=\tan\phi x_{a}+1$ . As consequence, the proof follows by combining (22) and (24). ∎

4. Proofs of Theorem 1 and Theorem 3

Reilly established an important integral formula for general Riemannian manifolds in [20]. Reilly’s formula is very powerful to obtain some properties on the hypersurfaces in Riemannian manifolds with non-negative Ricci curvatures. For instance, Ros used it to establish a Heintze-Karcher type inequality in [21]. Later on, Li and Xia [13] generalized it to a weighted Reilly-type formula to obtain a broader range of results. Let $(\overline{\nabla},\overline{\Delta},\overline{\nabla}^{2})$ and $(\nabla,\Delta,\nabla^{2})$ be the triples of Levi-Civita connection, Laplacian and Hessian operator on $(\overline{\Omega},\overline{g})$ and $(\partial\Omega,g)$ respectively. Then their Reilly-type formula is as follows:

Theorem 7 (See [13]).

Let $(\overline{\Omega},\overline{g})$ be a compact Riemannian manifold with piecewise smooth boundary $\partial\Omega$ , $V\in C^{\infty}(\overline{\Omega})$ be a positive smooth function. Suppose $\frac{\overline{\nabla}^{2}V}{V}$ is continuous up to $\partial\Omega$ . Then for any smooth function $f\in C^{\infty}(\overline{\Omega})$ , the following identity holds.

(25)		$\displaystyle\int_{\Omega}V\left(\left(\overline{\Delta}f-\frac{\overline{\Delta}V}{V}f\right)^{2}-\left\|\overline{\nabla}^{2}f-\frac{\overline{\nabla}^{2}V}{V}f\right\|^{2}\right)d\Omega$
	$\displaystyle=$	$\displaystyle\int_{\Omega}\left(\overline{\Delta}V\overline{g}-\overline{\nabla}^{2}V+V\overline{\mbox{Ric}}\right)\left(\overline{\nabla}f-\frac{\overline{\nabla}V}{V}f,\overline{\nabla}f-\frac{\overline{\nabla}V}{V}f\right)d\Omega$
		$\displaystyle+\int_{\partial\Omega}V\left(f_{\nu_{\partial\Omega}}-\frac{V_{\nu_{\partial\Omega}}}{V}f\right)\left(\Delta f-\frac{\Delta V}{V}f\right)dA$
		$\displaystyle-\int_{\partial\Omega}Vg\left(\nabla\left(f_{\nu_{\partial\Omega}}-\frac{V_{\nu_{\partial\Omega}}}{V}f\right),\nabla f-\frac{\nabla V}{V}f\right)dA$
		$\displaystyle+\int_{\partial\Omega}nVH_{1}\left(f_{\nu_{\partial\Omega}}-\frac{V_{\nu_{\partial\Omega}}}{V}f\right)^{2}dA$
		$\displaystyle+\int_{\partial\Omega}\left(h-\frac{V_{\nu_{\partial\Omega}}}{V}g\right)\left(\nabla f-\frac{\nabla V}{V}f,\nabla f-\frac{\nabla V}{V}f\right)dA,$

where $\nu_{\partial\Omega}$ is the outer normal vector field on $\partial\Omega$ , $h(\cdot,\cdot)$ and $H_{1}$ are the second fundamental form and mean curvature on $\partial\Omega$ respectively, and $\mathrm{Ric}$ is the Ricci curvature in $\Omega$ .

Let $\Omega$ be a domain in $\mathbb{B}_{+}^{n+1}$ enclosed by $\Sigma$ and $T$ , where $T$ is a domain on $P$ enclosed by $\partial\Sigma$ . Inspired by the idea in [10], we will give a proof of Theorem 1 now.

Proof of Theorem 1.

For $\theta=\frac{\pi}{2}$ , we refer to [18]. Therefore we will deal with the case of $\theta\in(0,\frac{\pi}{2})$ . We consider the following mixed boundary problem,

(29)

\displaystyle\left\{\begin{array}[]{ll}\overline{\Delta}f-(n+1)f=1,&\mbox{ in }\Omega;\\ f=0,&\mbox{ on }\Sigma;\\ f_{\overline{N}}=c_{0},&\mbox{ on }T,\end{array}\right.

where $c_{0}=-\frac{n}{n+1}\cot\theta\frac{\int_{T}V_{0}dA_{T}}{\int_{\partial\Sigma}V_{0}ds}$ . Since the idea about the existence and regularity is well established in [10], we will leave details of the following conclusion in the appendix: The solution $f$ of (29) satisfies $f\in C^{\infty}(\overline{\Omega}\setminus\partial\Sigma)\cup C^{2}(\Omega\cup T)\cup C^{1,\alpha}(\overline{\Omega})$ and $|\overline{\nabla}^{2}f|_{\overline{g}}\in L^{1}(T)$ . This makes $f$ applicable in the generalized Reilly’s formula (25) and by letting $V=V_{0}$ . Using (25), we have

(30)	$\displaystyle\frac{n}{n+1}\int_{\Omega}V_{0}d\Omega=$	$\displaystyle\frac{n}{n+1}\int_{\Omega}V_{0}\left(\overline{\Delta}f-\frac{\overline{\Delta}V_{0}}{V_{0}}f\right)^{2}d\Omega$
	$\displaystyle\geq$	$\displaystyle\int_{\Omega}V_{0}\left(\left(\overline{\Delta}f-\frac{\overline{\Delta}V_{0}}{V_{0}}f\right)^{2}-\left\|\overline{\nabla}^{2}f-\frac{\overline{\nabla}^{2}V_{0}}{V_{0}}f\right\|^{2}\right)d\Omega$
	$\displaystyle=$	$\displaystyle\int_{\Sigma}nV_{0}H_{1}f_{\nu}^{2}dA+c_{0}\int_{T}(V_{0}\Delta f-f\Delta V_{0})dA_{T}$
	$\displaystyle=$	$\displaystyle\int_{\Sigma}nV_{0}H_{1}f_{\nu}^{2}dA+c_{0}\int_{\partial\Sigma}V_{0}g(\nabla^{T}f,\overline{\nu})ds.$

In the second equality we use the fact that $\overline{\nabla}^{2}V_{0}=V_{0}\overline{g}$ , $\overline{\mbox{Ric}}=n\overline{g}$ and $(V_{0})_{\overline{N}}=\overline{g}(\widetilde{x},\overline{N})=0$ on $T$ , and in the third equality we apply the Green’s formula (See [17]).

Furthermore, since $f\in C^{1}(\overline{\Omega})$ and $f=0$ on $\Sigma$ , we have $f_{\mu}=0$ . From the relation (6), we have the following:

	$\displaystyle\overline{\nu}=$	$\displaystyle\cos\theta\mu+\sin\theta\nu$
	$\displaystyle=$	$\displaystyle\cos\theta\mu+\sin\theta(-\cos\theta\overline{N}+\sin\theta\overline{\nu}),$
	$\displaystyle=$	$\displaystyle\cos\theta\mu-\sin\theta\cos\theta\overline{N}+\sin^{2}\theta\overline{\nu}.$

Since $\overline{\nu}=\frac{1}{\cos\theta}\mu-\tan\theta\overline{N}$ , we have

	$\displaystyle c_{0}\int_{\partial\Sigma}V_{0}f_{\overline{\nu}}ds=$	$\displaystyle c_{0}\int_{\partial\Sigma}V_{0}\overline{g}(\overline{\nabla}f-f_{\overline{N}}\overline{N},\overline{\nu})ds$
	$\displaystyle=$	$\displaystyle c_{0}\int_{\partial\Sigma}V_{0}\overline{g}(\nabla^{T}f,\frac{1}{\cos\theta}\mu-\tan\theta\overline{N})ds$
	$\displaystyle=$	$\displaystyle-c_{0}^{2}\tan\theta\int_{\partial\Sigma}V_{0}ds$
	$\displaystyle=$	$\displaystyle-\left(\frac{n}{n+1}\right)^{2}\cot\theta\frac{\left(\int_{T}V_{0}dA_{T}\right)^{2}}{\int_{\partial\Sigma}V_{0}ds}.$

Therefore, (30) is equivalent to the following inequality,

(31)

\displaystyle\frac{n}{n+1}\left(\int_{\Omega}V_{0}d\Omega+\frac{n}{n+1}\cot\theta\frac{\left(\int_{T}V_{0}dA_{T}\right)^{2}}{\int_{\partial\Sigma}V_{0}ds}\right)\geq\int_{\Sigma}nV_{0}H_{1}f_{\nu}^{2}dA.

On the other hand, by Green’s formula to the equation above, we have

	$\displaystyle\int_{\Omega}V_{0}d\Omega=$	$\displaystyle\int_{\Omega}V_{0}\left(\overline{\Delta}f-\frac{\overline{\Delta}V_{0}}{V_{0}}f\right)d\Omega$
	$\displaystyle=$	$\displaystyle\int_{\Sigma}(V_{0}f_{\nu}-f(V_{0})_{\nu})dA+\int_{T}(V_{0}f_{\overline{N}}-f(V_{0})_{\overline{N}})dA_{T}$
	$\displaystyle=$	$\displaystyle\int_{\Sigma}V_{0}f_{\nu}dA+c_{0}\int_{T}V_{0}dA_{T}$
	$\displaystyle=$	$\displaystyle\int_{\Sigma}V_{0}f_{\nu}dA-\frac{n}{n+1}\cot\theta\frac{\left(\int_{T}V_{0}dA_{T}\right)^{2}}{\int_{\partial\Sigma}V_{0}ds}.$

Applying Hölder’s inequality, we obtain

(32)			$\displaystyle\int_{\Omega}V_{0}d\Omega+\frac{n}{n+1}\cot\theta\frac{\left(\int_{T}V_{0}d\nu_{T}\right)^{2}}{\int_{\partial\Sigma}V_{0}ds}$
(32)		$\displaystyle=$	$\displaystyle\int_{\Sigma}V_{0}f_{\mu}dA\leq\left(\int_{\Sigma}\frac{V_{0}}{nH_{1}}dA\right)^{\frac{1}{2}}\left(\int_{\Sigma}nV_{0}H_{1}f_{\nu}^{2}dA\right)^{\frac{1}{2}}.$

Combining (31) and (32), we have

(33)

\displaystyle\int_{\Sigma}\frac{V_{0}}{H_{1}}dA\geq(n+1)\int_{\Omega}V_{0}d\Omega+n\cot\theta\frac{\left(\int_{T}V_{0}dA_{T}\right)^{2}}{\int_{\partial\Sigma}V_{0}ds}.

If the equality in (33) holds, all the inequalities above become equalities. In particular from (30) we have,

\displaystyle\overline{\nabla}^{2}f-f\overline{g}=\overline{\nabla}^{2}f-\frac{\overline{\nabla}^{2}V_{0}}{V_{0}}f=\frac{1}{n+1}\left(\overline{\Delta}f-\frac{\overline{\Delta}V_{0}}{V_{0}}f\right)\overline{g}=\frac{1}{n+1}\overline{g},

which is equivalent to

(34)

\displaystyle\overline{\nabla}^{2}\left(f+\frac{1}{n+1}\right)=\left(f+\frac{1}{n+1}\right)\overline{g}

Restricting (34) to $\Sigma$ where $f=0$ , we can see that

f_{\nu}h_{ij}=\frac{1}{n+1}\overline{g}_{ij}.

This implies that $\Sigma$ is totally umbilical. ∎

Remark.

In the case of $\theta=\frac{\pi}{2}$ , we note that the last term of the right hand side in (30) vanishes, therefore there is no need for the Green’s formula used in the second equality in (30), the theory in [14] is sufficient. See the Appendix for detail.

Before giving a proof of Theorem 3, we need the following identities.

Proposition 5.

On the embedded capillary hypersurface $\Sigma\subset\mathbb{B}^{n+1}_{+}$ supported on $P$ , the following identities holds,

(35)

\displaystyle\int_{T}V_{0}dA_{T}=\int_{\Sigma}\overline{g}(Y_{n+1},\nu)dA,

and

(36)

\displaystyle\sin\theta\int_{\partial\Sigma}V_{0}ds=\int_{\Sigma}nH_{1}\overline{g}(Y_{n+1},\nu)dA.

Proof.

Since $Y_{n+1}$ is a Killing vector field, then $\overline{\mathrm{div}}(Y_{n+1})=0$ on $\Omega$ . Integrate on $\Omega$ , we have

	$\displaystyle 0=$	$\displaystyle\int_{\Omega}\overline{\mathrm{div}}(Y_{n+1})d\Omega$
	$\displaystyle=$	$\displaystyle\int_{\Sigma}\overline{g}(Y_{n+1},\nu)dA+\int_{T}\overline{g}(Y_{n+1},\overline{N})dA_{T}.$

Since $\overline{g}(Y_{n+1},\overline{N})=-V_{0}$ , we have

\displaystyle\int_{T}V_{0}dA_{T}=\int_{\Sigma}\overline{g}(Y_{n+1},\nu)dA.

Restricting (10) to $\Sigma$ and using the divergence theorem, we have

\displaystyle-\int_{\Sigma}nH_{1}\overline{g}(Y_{n+1},\nu)dA=\int_{\Sigma}\mathrm{div}_{\Sigma}(P_{\Sigma}Y_{n+1})dA=\int_{\partial\Sigma}\overline{g}(Y_{n+1},\mu)ds=-\sin\theta\int_{\partial\Sigma}V_{0}ds,

where $P_{\Sigma}$ denotes the projection on $T\Sigma$ . ∎

We are now ready to prove Theorem 3.

Proof of the Theorem 3.

Since $\Sigma$ is compact, there exists a $t\in(0,\pi)$ such that $\Sigma$ is contained in a domain enclosed by $P$ and consider 1-parameter family fo equidistant hypersurfaces $S_{t}$ .

\displaystyle S_{t}=\left\{x\in\mathbb{H}^{n+1}:|x+\tan tE_{n+1}|^{2}=\frac{1}{\cos^{2}t},\;t\in(0,\frac{\pi}{2})\right\}.

When $t\rightarrow 0+$ , $S_{t}$ tends to $\partial_{\infty}\mathbb{H}^{n+1}$ . On the other hand, when $t\rightarrow\frac{\pi}{2}-$ , $S_{t}$ tends to $P$ . Given that $\partial\Sigma$ is compact, and $\partial S_{t}=\partial P\subset\partial_{\infty}\mathbb{H}^{n+1}$ , therefore when $t\rightarrow\frac{\pi}{2}-$ , $S_{t}$ would only touch the interior of $\Sigma$ other than its boundary. As $t\rightarrow\frac{\pi}{2}$ , there must exist a $t_{0}$ such that $S_{t_{0}}$ touches $\mbox{int}(\Sigma)$ at first and tangent to $\Sigma$ at a point $p\in\mbox{int}(\Sigma$ ). Hence, we have $h(p)\geq\lambda_{S}(p)>0$ . Then $H_{1}\equiv H_{1}(p)>0$ . See the Figure 4.

Figure 4. Existence of the convex point on capillary hypersurface

\Sigma

supported on

P

Now applying (35) and (36) to the inequality (1), we can see that (1) is equivalent to the following:

(37)

\displaystyle\int_{\Sigma}\frac{V_{0}}{H_{1}}dA\geq(n+1)\int_{\Omega}V_{0}d\Omega+\cos\theta\frac{\left(\int_{\Sigma}\overline{g}(Y_{n+1},\nu)dA\right)^{2}}{\int_{\Sigma}H_{1}\overline{g}(Y_{n+1},\nu)dA}.

Since $H_{1}$ is constant, (37) can be written as

(38)	$\displaystyle\int_{\Sigma}\frac{V_{0}-\cos\theta\overline{g}(Y_{n+1},\nu)}{H_{1}}dA\geq$	$\displaystyle(n+1)\int_{\Omega}V_{0}d\Omega$
	$\displaystyle=$	$\displaystyle\int_{\Omega}\overline{\mathrm{div}}(x)d\Omega$
	$\displaystyle=$	$\displaystyle\int_{\Sigma}\overline{g}(x,\nu)dA.$

The last equality holds because $\overline{g}(x,\overline{N})=0$ on $T$ . Since $H_{1}$ is constant, from Proposition 3 ( $k=1$ ) we can see that the equality in (38) holds. According to the condition in Theorem 1, we obtain that $\Sigma$ is umbilical, but it is not totally geodesic since $H_{1}>0$ . ∎

Remark.

We consider the equality case of Theorem 1. From [25, Remark 4.2], we know that any function in $\{V\in C^{\infty}(\mathbb{H}^{n+1}):\overline{\nabla}^{2}V=V\overline{g}\}$ can be represented by the linear combination of the $n+2$ functions $\{V_{0},V_{1},\dots,V_{n+1}\}$ , where $V_{i}=\frac{2\langle x,E_{i}\rangle}{1-|x|^{2}}$ . From (34) we can see that there exists constants $B,\,c_{i}\in\mathbb{R}$ and a constant vector (in the Euclidean sense) $a\in\mathbb{R}^{n+1}$ such that

	$\displaystyle f+\frac{1}{n+1}=$	$\displaystyle BV_{0}+\sum\limits_{i=1}^{n+1}c_{i}V_{i}$
	$\displaystyle=$	$\displaystyle B\frac{1+\|x\|^{2}}{1-\|x\|^{2}}+\frac{2\langle x,C\rangle}{1-\|x\|^{2}}$
	$\displaystyle=$	$\displaystyle\frac{B(1+\|x\|^{2})+2\langle x,C\rangle}{1-\|x\|^{2}},$

where $C=\sum\limits_{i=1}^{n+1}c_{i}E_{i}$ .

(1)

When $B\neq-\frac{1}{n+1}$ , $\Sigma$ lies in a hypersurface defined by

\displaystyle\left\{x\in\mathbb{H}^{n+1}:\left|x+\frac{(n+1)}{1+(n+1)B}C\right|^{2}=\frac{1-(n+1)B}{1+(n+1)B}+\left(\frac{(n+1)|C|}{1+(n+1)B}\right)^{2}\right\}.

(2)

When $B=-\frac{1}{n+1}$ and $C\neq 0$ , $\Sigma$ lies in a hypersurface defined by

$\displaystyle\left\{x\in\mathbb{H}^{n+1}:\langle x,a\rangle=1/|C|\right\}.$

Therefore, $\Sigma$ is a totally umbilical hypersurface in $\mathbb{H}^{n+1}$ and can be a geodesic ball, a horosphere or an equidistant hypersurfaces, and $B,\,C\in\mathbb{R},a\in\mathbb{R}^{n+1}$ can be chosen such that $\Sigma$ is a part of one of the illustrated above and compact (compactness excludes the totally geodesic case). For example, a horosphere can be seen Figure 5.

Figure 5. Compact capillary horosphere

From the Figure 5, we can see

\displaystyle|OF|=\sqrt{\left|\frac{1-(n+1)B}{1+(n+1)B}\right|},\quad|OE|=\left|\frac{(n+1)}{1+(n+1)B}C\right|.

5. Proofs of Theorem 2 and Theorem 4

In this section, we consider the case when the supporting hypersurface is either an equidistant hypersurface or a horospheres. Before we give a proof of Theorem 2, we need the following lemma.

Proposition 6.

On the embedded capillary hypersurfaces $\Sigma\hookrightarrow\mathbb{R}^{n+1}_{+}$ supported on $L_{\phi,a}$ in the Poincaré half space model, the following identities holds

(39)

\int_{\Sigma}\overline{g}(\widetilde{x},\nu)dA=\cos\phi\int_{T}V_{n+1}dA_{T};

(40)

\int_{\Sigma}\overline{g}(a,\nu)dA=-\sin\phi\int_{T}V_{n+1}dA_{T};

(41)

\int_{\Sigma}(-nH_{1}\overline{g}(\widetilde{x},\nu))dA=\int_{\partial\Sigma}\overline{g}(\widetilde{x},\mu)ds;

(42)

\int_{\Sigma}(-nH_{1}\overline{g}(a,\nu))dA=\int_{\partial\Sigma}\overline{g}(a,\mu)ds;

and

(43)

\int_{\Sigma}n\cos\theta\overline{g}(\widetilde{x},\nu)dA=\int_{\partial\Sigma}\cos\phi\overline{g}(X_{n+1},\mu)ds.

Proof.

To prove (39) and (41), we know from (13) that $\overline{\mathrm{div}}\widetilde{x}=0$ . Let $\Omega$ be a domain enclosed by $\Sigma$ and $T$ . Using the divergence Theorem, we have

	$\displaystyle 0=$	$\displaystyle\int_{\Omega}\overline{\mathrm{div}}\widetilde{x}d\Omega$
	$\displaystyle=$	$\displaystyle\int_{\Sigma}\overline{g}(\widetilde{x},\nu)dA+\int_{T}\overline{g}(\overline{N},\widetilde{x})dA_{T}$
	$\displaystyle=$	$\displaystyle\int_{\Sigma}\overline{g}(\widetilde{x},\nu)dA+\int_{T}\frac{1}{x_{n+1}}(-\cos\phi x_{n+1}+\sin\phi x_{a})dA_{T}$
	$\displaystyle=$	$\displaystyle\int_{\Sigma}\overline{g}(\widetilde{x},\nu)dA-\int_{T}\cos\phi V_{n+1}dA_{T}.$

In the last equality, we use (2).

On the other hand, restricting (13) on $\Sigma$ , we have

\displaystyle\int_{\Sigma}\left(-nH_{1}\overline{g}(\widetilde{x},\nu)\right)dA=\int_{\Sigma}\mathrm{div}_{\Sigma}(P_{\Sigma}\widetilde{x})dA=\int_{\partial\Sigma}\overline{g}(\widetilde{x},\mu)ds,

where $P_{\Sigma}$ denotes the projection on $T\Sigma$ . Then we obtain (39) and (41). Using (12), we can prove (42) and (40). Moreover, (43) can be noted from in the proof of Proposition 4. ∎

Proof of Theorem 2.

Consider the following mixed boundary problem in the Poincaré half space model,

(44)

\left\{\begin{array}[]{ll}\overline{\Delta}f-(n+1)f=1,&\mbox{ in }\Omega;\\ f=0&\mbox{ on }\Sigma;\\ f_{\overline{N}}-\cos\phi f=c_{0}&\mbox{ on }T,\end{array}\right.

where

c_{0}=-\frac{n}{n+1}\cos\theta\frac{\int_{T}V_{n+1}dA_{T}}{\int_{\partial\Sigma}\overline{g}(-\cos\phi\widetilde{x}+\sin\phi a,\mu)ds}.

According to the Lieberman’s theory (see the appendix), there exists a solution $f$ such that $f\in C^{\infty}(\overline{\Omega}\setminus\partial\Sigma)\cup C^{2}(\Omega\cup T)\cup C^{1,\alpha}(\overline{\Omega})$ and $|\overline{\nabla}^{2}f|_{\overline{g}}\in L^{1}(T)$ . Therefore, for the same reason as the proof of Theorem 1, we can insert the solution $f$ to the generalized Reilly’s type formula (25).

	$\displaystyle\frac{n}{n+1}\int_{\Omega}V_{n+1}d\Omega=$	$\displaystyle\frac{n}{n+1}\int_{\Omega}V_{n+1}\left(\overline{\Delta}f-\frac{\overline{\Delta}V_{n+1}}{V_{n+1}}f\right)^{2}d\Omega$
	$\displaystyle\geq$	$\displaystyle\int_{\Omega}V_{n+1}\left(\left(\overline{\Delta}f-\frac{\overline{\Delta}V_{n+1}}{V_{n+1}}f\right)^{2}-\left\|\overline{\nabla}^{2}f-\frac{\overline{\nabla}^{2}V_{n+1}}{V_{n+1}}f\right\|^{2}\right)d\Omega$
	$\displaystyle=$	$\displaystyle\int_{\Sigma}nV_{n+1}H_{1}f_{\nu}^{2}dA+c_{0}\int_{T}(V_{n+1}\Delta f-f\Delta V_{n+1})dA_{T}$
		$\displaystyle+nc_{0}^{2}\cos\phi\int_{T}V_{n+1}dA_{T}$
	$\displaystyle=$	$\displaystyle\int_{\Sigma}nV_{n+1}H_{1}f^{2}_{\nu}dA+c_{0}\int_{\partial\Sigma}V_{n+1}\overline{g}(\nabla^{T}f,\overline{\nu})ds+nc_{0}^{2}\int_{\Sigma}\overline{g}(\widetilde{x},\nu)dA$
	$\displaystyle=$	$\displaystyle\int_{\Sigma}nV_{n+1}H_{1}f^{2}_{\nu}dA+c_{0}\int_{\partial\Sigma}V_{n+1}\overline{g}(\nabla^{T}f,\overline{\nu})ds$
		$\displaystyle+\frac{c_{0}^{2}\cos\phi}{\cos\theta}\int_{\partial\Sigma}\overline{g}(X_{n+1},\mu)ds,$

where we use the fact that $(V_{n+1})_{\overline{N}}=-\frac{1}{x_{n+1}^{2}}\langle E_{n+1},\overline{N}\rangle=\cos\phi V_{n+1}$ and (43) in the last equality. On $\partial\Sigma$ , using (6) and the boundary condition in (44), we have

	$\displaystyle\overline{g}(\nabla^{T}f,\overline{\nu})=$	$\displaystyle\overline{g}(\overline{\nabla}f-\overline{g}(\overline{\nabla}f,\overline{N})\overline{N},\frac{1}{\cos\theta}\mu)$
	$\displaystyle=$	$\displaystyle-\frac{c_{0}}{\cos\theta}\overline{g}(\overline{N},\mu).$

Then we have

	$\displaystyle\frac{n}{n+1}\int_{\Omega}V_{n+1}d\Omega\geq$	$\displaystyle\int_{\Sigma}nV_{n+1}H_{1}f_{\nu}^{2}dA-\frac{c_{0}^{2}}{\cos\theta}\int_{\partial\Sigma}V_{n+1}\overline{g}(\overline{N},\mu)ds$
		$\displaystyle+\frac{c_{0}^{2}\cos\phi}{\cos\theta}\int_{\partial\Sigma}\overline{g}(X_{n+1},\mu)ds$
	$\displaystyle=$	$\displaystyle\int_{\Sigma}nV_{n+1}H_{1}f_{\nu}^{2}dA-\frac{c_{0}^{2}}{\cos\theta}\int_{\partial\Sigma}\overline{g}(-\cos\phi\widetilde{x}+\sin\phi a,\mu)ds$
	$\displaystyle=$	$\displaystyle\int_{\Sigma}nV_{n+1}H_{1}f_{\nu}^{2}dA-\left(\frac{n}{n+1}\right)^{2}\cos\theta\frac{\left(\int_{T}V_{n+1}dA_{T}\right)^{2}}{\int_{\partial\Sigma}\overline{g}(-\cos\phi\widetilde{x}+\sin\theta a,\mu)ds}.$

Therefore,

(45)			$\displaystyle\frac{n}{n+1}\left(\int_{\Omega}V_{n+1}d\Omega+\frac{n}{n+1}\cos\theta\frac{\left(\int_{T}V_{n+1}dA_{T}\right)^{2}}{\int_{\partial\Sigma}\overline{g}(-\cos\phi\widetilde{x}+\sin\theta a,\mu)ds}\right)$
(45)		$\displaystyle\geq$	$\displaystyle\int_{\Sigma}nV_{n+1}H_{1}f_{\nu}^{2}dA.$

On the other hand, using the divergence theorem we have

(46)	$\displaystyle\int_{\Omega}V_{n+1}d\Omega=$	$\displaystyle\int_{\Omega}V_{n+1}\left(\overline{\Delta}f-\frac{\overline{\Delta}V_{n+1}}{V_{n+1}}f\right)d\Omega$
	$\displaystyle=$	$\displaystyle\int_{\Sigma}(V_{n+1}f_{\nu}-f(V_{n+1})_{\nu})dA+\int_{T}(V_{n+1}f_{\overline{N}}-f(V_{n+1})_{\overline{N}})dA_{T}$
	$\displaystyle=$	$\displaystyle\int_{\Sigma}V_{n+1}f_{\nu}dA+c_{0}\int_{T}V_{n+1}dA_{T}$
	$\displaystyle=$	$\displaystyle\int_{\Sigma}V_{n+1}f_{\nu}dA-\frac{n}{n+1}\cos\theta\frac{\left(\int_{T}V_{n+1}dA_{T}\right)^{2}}{\int_{\partial\Sigma}\overline{g}(-\cos\phi\widetilde{x}+\sin\phi a,\mu)ds}.$

Combining (45) and (46) and using the similar argument as the proof of Theorem 1, we prove the inequality in Theorem 2. The equality case is also the same as Theorem 1. ∎

At this point, we are ready to prove the Alexandrov type theorem for the capillary hypersurface in $B^{\mathrm{int}}_{\phi,a}$ .

Proof of the Theorem 4.

In order to prove the existence of convex points on $\Sigma$ , we consider both the case that $\phi>0$ and the case that $\phi=0$ independently.
Case 1 ( $\phi>0$ ): Now $L_{\phi,a}$ is an equidistant hypersurface. Let $\eta\in(0,\phi)$ , which will be determined later. We consider a set

P_{\eta}=\{\widetilde{x}\in\mathbb{H}^{n+1}:x_{n+1}=\tan\eta(x_{a}+1)\}.

Let $p\in P_{\eta}$ and $S_{p}$ be a family of equidistant hypersurface defined as

S_{p}=\{\widetilde{x}\in\mathbb{H}^{n+1}:|\widetilde{x}-p|=|p-\mathrm{dist}_{\mathbb{R}}(p,\partial P_{\eta})|\mbox{ and }p\in P_{\eta}\},

where $\mathrm{dist}_{\mathbb{R}}$ is the Euclidean distance in the Poincaré half space model. Since $\Sigma$ is a compact capillary hypersurface supported on $L_{\phi,a}$ , we can find a proper $p_{0}\in P_{\eta}$ sufficiently far (in the Euclidean sense) from $\partial P_{\eta}$ , such that $\Sigma$ is contained in the domain enclosed by $S_{p_{0}}$ and $L_{\phi,a}$ and $\eta>0$ guarantees that $\Sigma$ stays in the interior side of $S_{p_{0}}$ . Then for any $p\in P$ the contact angle $\theta_{0}$ between $L_{\phi,a}$ and $S_{p_{0}}$ is constant satisfying $\theta_{0}=\frac{\pi}{2}-\phi+\eta$ .

Since $\theta+\phi>\frac{\pi}{2}$ , there exists an $\eta\in(0,\phi)$ such that $\theta>\theta_{0}=\frac{\pi}{2}-\phi+\eta$ .

Now moving $p_{0}$ toward $\partial P_{\eta}$ such that $\mathrm{dist}_{\mathbb{R}}(p_{0},\partial P_{\eta})$ goes to $0$ , we can see that there exists $p_{1}$ such that $S_{p_{1}}$ firstly touches $\Sigma$ at some $q\in\mathrm{int}(\Sigma)$ since $\theta>\theta_{0}$ . Meanwhile, since $\eta>0$ , $\Sigma$ remains within the interior side of $S_{p_{1}}$ . See Figure 6.

Figure 6. Existence of the convex point for hypersurfaces supported on a equidistant hypersurface

Then we can see that the principal curvature $\lambda(q)\geq\lambda_{S_{p_{1}}}(q)>0$ of $\Sigma$ , then $H_{1}\equiv H_{1}(q)>0$ .
Case 2 ( $\phi=0$ ): Now $L_{\phi,a}$ is a horosphere and $\theta=\frac{\pi}{2}$ by assumption. Consider a set

P_{r_{0}}=\{x\in\mathbb{H}^{n+1}:x_{n+1}=r_{0}\},

for $0<r_{0}<1$ . Let $S_{R,p}$ be the hypersurface defined by

S_{R,p}=\{x\in\mathbb{H}^{n+1}:|\widetilde{x}-p|=R,p\in P_{r_{0}}\}.

Since $\Sigma$ is compact, there must exist $p_{0}\in P_{r_{0}}$ and a positive $R_{0}$ big enough such that $\Sigma$ is contained in the domain enclosed by $S_{R_{0},p_{0}}$ and $L_{\phi,a}$ . Now fix $p_{0}$ and shrinking the radius $R_{0}$ of $S_{R_{0},p_{0}}$ in the Euclidean sense until it touches $\Sigma$ at $p$ for the first time when the radius is $R_{1}$ . Since the contact angle of $S_{R_{1},p_{0}}$ is less than $\frac{\pi}{2}$ , the firstly touching point $q$ must be the interior point on $\Sigma$ . Since the contact angle of $S_{R_{1},p_{0}}$ and $\partial_{\infty}\mathbb{H}^{n+1}:=\{x_{n+1}=0\}$ is greater than $\frac{\pi}{2}$ , $\Sigma$ is staying in the interior side of $S_{R_{1},p_{0}}$ . See Figure 7.

Figure 7. Existence of the convex point for hypersurfaces supported on a horosphere

Then it holds that $\lambda(q)\geq\lambda_{S_{R_{1},p_{0}}}>0$ and $H_{1}\equiv H_{1}(q)>0$ . Therefore the Heintze-Karcher inequality in Theorem 2 can be applied. And from (39), (39), (42) and (40), we are able to rewrite (3) as

	$\displaystyle\int_{\Sigma}\frac{V_{n+1}}{H_{1}}dA\geq$	$\displaystyle(n+1)\int_{\Omega}V_{n+1}d\Omega+n\cos\theta\frac{\left(\int_{T}V_{n+1}dA_{T}\right)^{2}}{\int_{\partial\Sigma}\overline{g}(-\cos\phi\widetilde{x}+\sin\phi a,\mu)ds}$
	$\displaystyle=$	$\displaystyle(n+1)\int_{\Omega}V_{n+1}d\Omega+\cos\theta\frac{\left(\int_{T}V_{n+1}dA_{T}\right)^{2}}{H_{1}\int_{\Sigma}\overline{g}(\cos\phi\widetilde{x}-\sin\phi a,\nu)dA}$
	$\displaystyle=$	$\displaystyle(n+1)\int_{\Omega}V_{n+1}d\Omega+\frac{\sec\theta\cos\phi}{H_{1}}\int_{\Sigma}\overline{g}(\widetilde{x},\nu)dA.$

From (11), we have

	$\displaystyle(n+1)V_{n+1}d\Omega=$	$\displaystyle\int_{\Omega}\overline{\mathrm{div}}X_{n+1}d\Omega$
	$\displaystyle=$	$\displaystyle\int_{\Sigma}\overline{g}(X_{n+1},\nu)dA+\int_{T}\overline{g}(X_{n+1},\overline{N})dA_{T}$
	$\displaystyle=$	$\displaystyle\int_{\Sigma}\overline{g}(X_{n+1},\nu)dA.$

Now the rest is the same as the prove of Theorem 3. Together with Minkowski type formula (21), we prove that $\Sigma$ is umbilical. ∎

Remark.

Indeed, the following example indicate that there exists a totally umbilical capillary hypersurface in $B^{\mathrm{int}}_{\phi,a}$ without any convex point. Consider a totally geodesic hypersurface is given as follows

\Sigma=\{\widetilde{x}\in\mathbb{H}_{n+1}:\delta(\widetilde{x},\widetilde{x})=2\},

and the supporting hypersurface is $L_{\frac{\pi}{6}}$ . Then the totally geodesic $\Sigma$ is a capillary hypersurface supported on $L_{\frac{\pi}{6}}$ and the contact angle $\theta=\arccos\frac{\sqrt{6}}{4}\approx 0.912$ , but it is apparent to have not any convex point. Also we can see that $\theta+\phi\approx 1.435<\frac{\pi}{2}$ , which is not satisfying the condition in Theorem 4. See Figure 8.

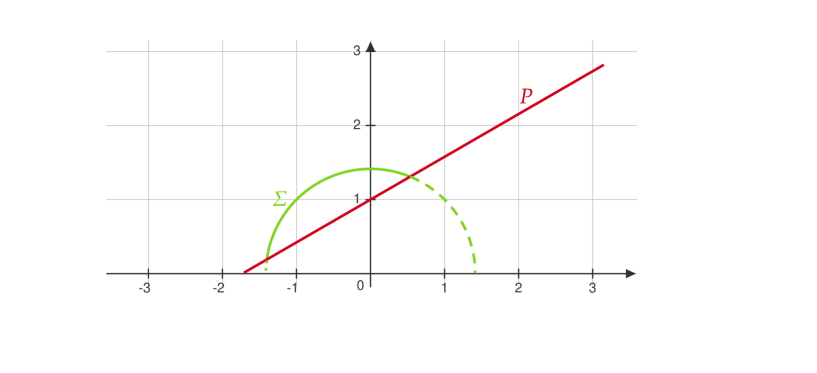
Refer to caption — Figure 8. Capillary hypersurfaces in $B^{\mathrm{int}}_{\phi,a}$ without any convex point

We can see from the proof of Theorem 4 that the assumption “ $\phi+\theta>\frac{\pi}{2}$ for $\phi>0$ or $\theta=\frac{\pi}{2}$ for $\phi=0$ ” can be replaced by $H_{1}>0$ . Then, we have the following corollary.

Corollary 1.

Let $\Sigma$ be a compactly embedded CMC capillary hypersurface contained in $B^{\mathrm{int}}_{\phi,a}$ supported on $L_{\phi,a}$ , and the contact angle satisfies $\theta\in(0,\frac{\pi}{2}]$ . Assume $H_{1}>0$ , then $\Sigma$ is umbilical except for being totally geodesic.

6. Capillary hypersurfaces in a geodesic ball

In this section, we give an Alexandrov type theorem for capillary hypersurfaces in a geodesic ball. Consider a geodesic ball $B_{R}$ centered at the origin in the Poincaré ball model, where $R$ the hyperbolic radius. Then $B_{R}$ can be given by

B_{R}=\{x\in\mathbb{H}^{n+1}:g(x,x)\leq R^{2}\}=\{x\in\mathbb{H}^{n+1}:|x|^{2}\leq R_{\delta}^{2}\},

where $R_{\delta}$ is the Euclidean radius of $B_{R}$ . Thus, the following may be easily noted:

\cosh R=\frac{1+R_{\delta}^{2}}{1-R_{\delta}^{2}}\quad\mbox{ and }\quad\sinh R=\frac{2R_{\delta}}{1-R_{\delta}^{2}}.

Then the unit outward normal $\overline{N}$ of $B_{R}$ satisfies that

(47)

\overline{N}=\frac{1}{\sinh R}x.

In the paper of Wang and Xia (See [25]), the authors give a family of conformal Killing vector fields $X_{a}$ . It is given by

X_{a}=\frac{2}{1-R_{\delta}^{2}}\left[\delta(x,a)x-\frac{1}{2}(|x|^{2}+R_{\delta}^{2})a\right],

where $a$ is a constant vector with respect to the Euclidean metric $(\mathbb{B}^{n+1},\delta)$ satisfying that

\frac{1}{2}\left(\overline{g}(\overline{\nabla}_{\overline{E}_{A}}X_{a},\overline{E}_{B})+\overline{g}(\overline{\nabla}_{\overline{E}_{B}}X_{a},\overline{E}_{A})\right)=V_{a}\overline{g},

where $V_{a}=\frac{2\delta(x,a)}{1-|x|^{2}}$ satisfying $\overline{\nabla}^{2}V_{a}=V_{a}\overline{g}$ . A direct computation shows that

(48)

\cosh RX_{a}=V_{a}x-\sinh^{2}RY_{a}.

Figure 9. Capillary hypersurface

\Sigma

supported on a geodesic ball

B_{R}

We have the following properties analogously,

Proposition 7.

Let $\Sigma$ be an embedded capillary hypersurface supported on the geodesic ball $B_{R}$ . Let $\Omega$ be the domain enclosed by $\Sigma$ and $\partial B_{R}$ and $\partial\Omega=\Sigma\cup T$ , we have

(49)

\int_{T}V_{a}dA_{T}=-\sinh R\int_{\Sigma}\overline{g}(Y_{a},\nu)dA,

(50)

\int_{\partial\Sigma}\overline{g}(Y_{a},\mu)ds=-\int_{\Sigma}nH_{1}\overline{g}(Y_{a},\nu)dA,

(51)

\int_{\Sigma}\left(V_{a}+\cos\theta\sinh R\overline{g}(Y_{a},\nu)-H_{1}\overline{g}(X_{a},\nu)\right)dA=0,

and

(52)

\int_{\Sigma}n\sinh R\cos\theta\overline{g}(Y_{a},\nu)dA=\int_{\partial\Sigma}\overline{g}(X_{a},\mu)ds.

Sketch of the proof.

The proof of (49) and (50) can be seen easily from the proof of other supporting hypersurfaces cases, using the fact that $Y_{a}$ is a Killing vector field, and (51) and (52) are given in the proof of [25, Prop 4.4]. ∎

Consider the following mixed boundary problem

(53)

\left\{\begin{array}[]{ll}\overline{\Delta}f-(n+1)f=1&\mbox{ in }\Omega;\\ f=0&\mbox{ on }\Sigma;\\ f_{\overline{N}}-\coth Rf=c_{0}&\mbox{ on }T.\end{array}\right.

Where $c_{0}=-\frac{n}{n+1}\cos\theta\frac{\int_{T}V_{a}dA_{T}}{\int_{\partial\Sigma}\sinh R\overline{g}(Y_{a},\mu)ds}$ is chosen. The existence and regularity of the solution to the problem (53) can be obtain from Lieberman’s theory. See the Appendix.

Theorem 8.

Let $B_{R,a+}=\{x\in B_{R}:V_{a}>0\}$ be a half geodesic ball where $V_{a}$ is positive. Let $\Sigma\subset B_{R,a+}$ be a compact, embedded capillary hypersurface in $B_{R,a+}$ , and the supporting hypersurface is $\partial B_{R}$ . Let $\Omega$ be the domain enclosed by $\Sigma$ and $L_{\phi,a}$ . If the contact angle $\theta\in(0,\frac{\pi}{2}]$ , and the mean curvature $H_{1}>0$ on $\Sigma$ , we have

(54)

\displaystyle\int_{\Sigma}\frac{V_{a}}{H_{1}}dA\geq(n+1)\int_{\Omega}V_{a}d\Omega+n\cos\theta\frac{\left(\int_{T}V_{a}dA_{T}\right)^{2}}{\int_{\partial\Sigma}\sinh R\overline{g}(Y_{a},\mu)ds},

where $\mu$ is the outward unit conormal vector field of $\partial\Sigma$ in $\Sigma$ . In addition, the equality holds if and only if $\Sigma$ is umbilical.

Proof.

Let $V=V_{a}$ and $f$ be the solution of (53) in (25). Using the relations (47), (48) and Proposition 7 and noting that $(V_{a})_{\overline{N}}=\coth RV_{a}$ , we have

	$\displaystyle\frac{n}{n+1}\int_{\Omega}V_{a}d\Omega\geq$	$\displaystyle\int_{\Sigma}nV_{a}H_{1}f_{\nu}^{2}dA+c_{0}\int_{T}(V_{a}\triangle f-f\triangle V_{a})dA_{T}+c_{0}^{2}\int_{T}n\coth RV_{a}dA_{T}$
	$\displaystyle=$	$\displaystyle\int_{\Sigma}V_{a}H_{1}f_{\nu}^{2}dA+c_{0}\int_{\partial\Sigma}V_{a}f_{\overline{\nu}}ds+c_{0}^{2}\int_{T}n\coth RV_{a}dA_{T}$
	$\displaystyle=$	$\displaystyle\int_{\Sigma}nV_{a}H_{1}f_{\nu}^{2}dA-\frac{c_{0}^{2}}{\cos\theta}\int_{\partial\Sigma}V_{a}\overline{g}(\mu,\frac{1}{\sinh R}x)ds$
		$\displaystyle-c_{0}^{2}\cosh R\int_{\Sigma}n\overline{g}(Y_{a},\nu)dA$
	$\displaystyle=$	$\displaystyle\int_{\Sigma}nV_{a}H_{1}f_{\nu}^{2}dA-\frac{c_{0}^{2}}{\cos\theta}\int_{\partial\Sigma}\overline{g}(\mu,\coth RX_{a}+\sinh RY_{a})ds$
		$\displaystyle+\frac{c_{0}^{2}}{\cos\theta}\int_{\partial\Sigma}\coth R\overline{g}(Y_{a},\mu)ds$
	$\displaystyle=$	$\displaystyle\int_{\Sigma}nV_{a}H_{1}f_{\nu}^{2}dA-\frac{c_{0}^{2}}{\cos\theta}\int_{\partial\Sigma}\sinh R\overline{g}(\mu,Y_{a})ds.$

Therefore,

(55)

\frac{n}{n+1}\left(\int_{\Omega}V_{a}d\Omega+\frac{n}{n+1}\cos\theta\frac{\left(\int_{T}V_{a}dA_{T}\right)^{2}}{\int_{\partial\Sigma}\sinh R\overline{g}(Y_{a},\mu)ds}\right)\geq\int_{\Sigma}nV_{a}H_{1}f_{\nu}^{2}dA.

On the other hand,

\displaystyle\int_{\Omega}V_{a}d\Omega=\int_{\Omega}(V_{a}\overline{\triangle}f-f\overline{\triangle}V_{a})d\Omega=\int_{\Sigma}V_{a}f_{\nu}dA+c_{0}\int_{T}V_{a}dA_{T}.

We have

(56)		$\displaystyle\int_{\Sigma}V_{a}f_{\nu}dA=$	$\displaystyle\int_{\Omega}V_{a}d\Omega-c_{0}\int_{T}V_{a}dA_{T}$
(56)		$\displaystyle=$	$\displaystyle\int_{\Omega}V_{a}d\Omega+\frac{n}{n+1}\cos\theta\frac{\left(\int_{T}V_{a}dA_{T}\right)^{2}}{\int_{\partial\Sigma}\sinh R\overline{g}(Y_{a},\mu)ds}.$

Hence, using (55) and (56) and the similar argument as the proof of Theorem 1, we prove the inequality in Theorem 8. The equality case is also the same as Theorem 1. ∎

Using (49), (50), (51) in Proposition 7 and the fact that $\overline{g}(X_{a},\overline{N})=0$ , we can prove the following Alexandrov type theorem.

Theorem 9.

Let $\Sigma$ be an embedded CMC capillary hypersurface contained in $B_{R,a+}$ supported on $\partial B_{R}$ . If $\theta\in(0,\frac{\pi}{2}]$ and $H_{1}>0$ , then $\Sigma$ is umbilical except for being totally geodesic.

Remark.

Since we do not have the existence of convex points on $\Sigma$ , we assume that $H_{1}>0$ .

7. Other rigidity results for capillary hypersurfaces

In this section, we will prove the Theorem 5 and Theorem 6. The proof of Theorem 5 is due to the Minkowski type formula in Proposition 3.

The proof of Theorem 5.

Since $(H_{k}/H_{l})(p)=\alpha>0$ , from Newton-Maclaurin inequality, we have

\displaystyle H_{k-1}H_{l}\geq H_{k}H_{l-1}=\alpha H_{l}H_{l-1}.

and since $H_{l}>0$ are positive on $\Sigma$ , we have $H_{k-1}-\alpha H_{l-1}\geq 0$ . On the other hand, applying Proposition 3, we have

(57)	$\displaystyle 0=$	$\displaystyle\int_{\Sigma}\left[H_{k-1}(V_{0}-\cos\theta\overline{g}(Y_{n+1},\nu))-H_{k}\overline{g}(x,\nu)\right]dA$
	$\displaystyle=$	$\displaystyle\int_{\Sigma}\left[H_{k-1}(V_{0}-\cos\theta\overline{g}(Y_{n+1},\nu))-\alpha H_{l}\overline{g}(x,\nu)\right]dA$
	$\displaystyle=$	$\displaystyle\int_{\Sigma}(H_{k-1}-\alpha H_{l-1})(V_{0}-\cos\theta\overline{g}(Y_{n+1},\nu))dA.$

Now, we consider the term $V_{0}-\cos\theta\overline{g}(Y_{n+1},\nu)$ . We see that

	$\displaystyle\overline{g}(Y_{n+1},Y_{n+1})=$	$\displaystyle\frac{1}{4}(1+\|x\|^{2})^{2}\overline{g}(E_{n+1},E_{n+1})+\langle x,E_{n+1}\rangle^{2}\overline{g}(x,x)$
		$\displaystyle-\langle x,E_{n+1}\rangle(1+\|x\|^{2})\overline{g}(x,E_{n+1})$
	$\displaystyle=$	$\displaystyle\frac{(1+\|x\|^{2})^{2}-4\langle x,E_{n+1}\rangle^{2}}{(1-\|x\|^{2})^{2}}.$

Since $\langle x,E_{n+1}\rangle>0$ on $\mbox{int}(\Sigma)$ , we have

|\overline{g}(Y_{n+1},\nu)|\leq\sqrt{\overline{g}(Y_{n+1},Y_{n+1})}<V_{0},

and therefore

(58)

\displaystyle V_{0}-\cos\theta\overline{g}(Y_{n+1},\nu)>0.

Hence, combining (57) and (58), we have $H_{k-1}-\alpha H_{l-1}=0$ . This results in

(59)

\displaystyle H_{k-1}H_{l}=H_{k}H_{l-1}.

Hence, examining the equality condition of Newton-Maclaurin inequality, we obtain that $\Sigma$ is totally umbilical, completing the proof of Theorem 5. ∎

Now we give a proof of Theorem 6.

Proof of Theorem 6.

Let $G$ be a smooth function defined by $G=nH_{1}V_{0}-n\overline{g}(x,\nu)$ . Integrating the Laplacian of $G$ , we have

	$\displaystyle\int_{\Sigma}\Delta GdA=$	$\displaystyle\int_{\partial\Sigma}\nabla_{\mu}Gds$
	$\displaystyle=$	$\displaystyle\int_{\partial\Sigma}(nH_{1}-nh(\mu,\mu))\overline{g}(x,\mu))ds$
	$\displaystyle=$	$\displaystyle\cos\theta\int_{\partial\Sigma}(nH_{1}-nh(\mu,\mu))\overline{g}(x,\overline{\nu})ds.$

For any $e\in T(\partial\Sigma)$ , we can see from(6) that

(60)

\displaystyle h(e,e)=\overline{g}(\overline{\nabla}_{e}\nu,e)=\overline{g}(\overline{\nabla}_{e}(\cos\theta\overline{N}+\sin\theta\overline{\nu}),e)=\sin\theta h^{\partial\Sigma}(e,e).

By letting $k=1$ in (18), we have

\displaystyle\int_{\Sigma}n\overline{g}(Y_{n+1},\nu)dA=\int_{\partial\Sigma}\overline{g}(x,\overline{\nu})ds.

Then we have

(61)	$\displaystyle\int_{\Sigma}\Delta GdA=$	$\displaystyle\cos\theta\int_{\partial\Sigma}(nH_{1}-nh(\mu,\mu))\overline{g}(x,\overline{\nu})ds$
	$\displaystyle=$	$\displaystyle\cos\theta\int_{\partial\Sigma}(n(nH_{1}-h(\mu,\mu))-n(n-1)H_{1})\overline{g}(x,\overline{\nu})ds$
	$\displaystyle=$	$\displaystyle\cos\theta\int_{\partial\Sigma}(n(n-1)\sin\theta H^{\partial\Sigma}_{1}-n(n-1)H_{1})\overline{g}(x,\overline{\nu})ds$
	$\displaystyle=$	$\displaystyle\cos\theta\left[\int_{\partial\Sigma}n(n-1)\sin\theta H^{\partial\Sigma}_{1}\overline{g}(x,\overline{\nu})ds-n^{2}(n-1)H_{1}\right.$
		$\displaystyle\quad\left.\times\int_{\Sigma}\overline{g}(Y_{n+1},\nu)dA\right]$
	$\displaystyle=$	$\displaystyle\cos\theta\int_{\partial\Sigma}(n(n-1)\sin\theta H^{\partial\Sigma}_{1}\overline{g}(x,\overline{\nu})-n(n-1)\sin\theta V_{0})ds$
	$\displaystyle=$	$\displaystyle 0.$

We use (36) in the fifth equality. In the last equality, we use a Minkowski type formula for closed hypersurface in $P$ (See [2]), which is the $(n-1)$ -dimensional hyperbolic space.

On the other hand, it is well known that in hypersurface

\displaystyle\Delta V_{0}=nV_{0}-nH_{1}\overline{\nabla}_{\nu}V_{0},

and

\displaystyle\Delta\overline{g}(x,\nu)=nH_{1}V_{0}-|h|^{2}\overline{g}(X,\nu)-n\overline{\nabla}_{\nu}V+n\overline{g}(X,\nu).

Together with (61), we have

\displaystyle 0=\int_{\Sigma}\Delta GdA=\int_{\Sigma}n(|h|^{2}-nH_{1}^{2})\overline{g}(x,\nu)dA.

Therefore, from the star-shapedness of $\Sigma$ , we can see that $\Sigma$ is umbilical. In addition, the totally geodesic case is excluded by the compactness assumption on $\Sigma$ . ∎

Remark.

All of our results require that $\Sigma$ is compact. Because $\Sigma$ is totally umbilical and contained in $\mathbb{B}^{n+1}_{+}$ , it can be compact and a part of a horosphere or an equidistant hypersurface simultaneously. If so, we notice that the contact angle $\theta$ must lie in the open interval $(0,\frac{\pi}{2})$ . See the Figure 10 and Figure 11.

Figure 10.

\Sigma

is a horosphere

Figure 11.

\Sigma

is an equidistant hypersurface

Remark.

Since the star-shapedness of $\Sigma$ implies that $\Sigma$ is an embedded hypersurface, Theorem 6 can be obtained immediately from Theorem 3 when the contact angle $\theta\in(0,\frac{\pi}{2}]$ .

Appendix

To obtain the existence and sufficient regularity of the solution of the mixed-boundary problem (29), (44) and (53), we write it in the conformal Euclidean space as

\displaystyle\left\{\begin{array}[]{ll}\mathcal{L}f:=e^{-2u}(\overline{\Delta}_{\delta}f+(n-1)du(f))-(n+1)f=1&\mbox{ in }\Omega;\\ f=0&\mbox{ on }\Sigma;\\ f_{\overline{N}}-\gamma f=c_{0}&\mbox{ on }T,\end{array}\right.

where $\overline{\nabla}_{\delta}$ and $\overline{\Delta}_{\delta}$ are the Levi-Civita connection and Laplacian with respect to the Euclidean metric $\delta$ respectively. Since the conformal map preserves the angle, the condition of $\theta$ is the same under both Euclidean metric and hyperbolic metric.

We notice that the coefficients $a_{ij}=e^{-2u}\delta_{ij},\,b^{i}=(n-1)e^{-2u}u^{i},\;c=-(n+1)$ satisfy the conditions in [14], page 435, and $\gamma\geq 0$ satisfies the condition in [23, Lemma 4.1]. From the theory of Lieberman and Theorem A.3 in [10], there exists a solution $f\in C^{\infty}(\overline{\Omega}\setminus\partial\Sigma)\cup C^{2}(\mathrm{int}(\Omega)\cup T)$ with respect to the conformal metric $\delta$ .

Let $d_{\partial\Sigma}$ be the distance function from $\partial\Sigma$ and define $\Omega_{\varepsilon}=\{x\in\Omega:d_{\partial\Sigma}(x)>\varepsilon\}$ . To apply the result of Lieberman, we need to introduce the norm

\displaystyle|f|^{b}_{a}=\sup_{\varepsilon>0}\varepsilon^{a+b}|f|_{a,\Omega_{\varepsilon}},

where $|f|_{a,\Omega_{\varepsilon}}=\sum\limits_{i=1}^{k}\sup_{x\in\Omega_{\varepsilon}}|\nabla_{\delta}^{k}f|+\sup_{x,y\in\Omega_{\varepsilon}}\frac{|D^{k}f(x)-D^{k}f(y)|}{|x-y|^{\alpha}}$ for $a>0$ and $a=k+\alpha$ for an integer $k$ and $0\leq\alpha<1$ .

Let $f$ be the solution of the following boundary value problem

\displaystyle\left\{\begin{array}[]{ll}\mathcal{L}f=g&\mbox{ in }\Omega;\\ f=0&\mbox{ on }\Sigma;\\ \langle\overline{\nabla}_{\delta}f,\overline{N}\rangle=h&\mbox{ on }T.\end{array}\right.

From the theory by Lieberman (See Theorem 4 in [15]) and Lemma A.1 in [10], we have the following estimate

\displaystyle|f|_{a}^{-\lambda}\leq C(|g|_{a-2}^{2-\lambda}+|h|_{a-1}^{1-\lambda}+|g|_{0}+|h|_{0}).

Here we notice that the maximum principle (see [23, Lemma 4.1]) applied in [10, Lemma A.1] holds for general operator $\mathcal{L}$ with $c=-(n+1)<0$ and $\gamma\geq 0$ . Furthermore, from the proof of Theorem 4 in [15], the construction of the key Miller’s type barrier requires $\lambda<\frac{\pi}{2\theta}$ (See [15, Lemma 4.1]).

From the discussion by Jia-Wang-Xia (See [10], Page 8-9 and Lemma A.1), we can see that $|\overline{\nabla}_{\delta}^{2}f|_{\delta}\in L^{2}(\Omega)$ requires $|\overline{\nabla}^{2}_{\delta}f|_{\delta}\leq Cd_{\partial\Sigma}^{-\beta}$ for $\beta\in(0,1)$ and $a>2$ . Then, we can also obtain that $|\nabla_{\delta}^{2}f|_{\delta}\in L^{1}(T)$ .

Meanwhile in the proof of the Theorem 1, we need the regularity of $f$ satisfying $f\in C^{1,\alpha}(\overline{\Omega})$ . From the definition and the monotonicity of the norm $|\cdot|_{a}^{b}$ , we have $|f|_{\lambda}=|f|_{\lambda}^{-\lambda}\leq|f|_{a}^{-\lambda}$ . Therefore it is sufficient for $f\in C^{1,\alpha}(\overline{\Omega})$ when $\lambda>1$ .

In conclusion, all the condition for the applicable regularity will be satisfied if we let $1<\lambda<\mbox{min}\{3,\frac{\pi}{2\theta}\}$ , $a=\frac{\lambda+3}{2}>2$ and $\beta=a-\lambda=\frac{3-\lambda}{2}\in(0,1)$ . Hence, the condition $\theta<\frac{\pi}{2}$ is sufficient. From a well-known fact, under conformal transformations of metrics we have

\displaystyle\overline{\nabla}f=e^{-2u}\overline{\nabla}_{\delta}f,

and

\displaystyle\overline{\nabla}^{2}_{ij}f=\overline{\nabla}^{2}_{\delta,\;ij}f-u_{i}f_{j}-u_{j}f_{i}-\delta(\overline{\nabla}_{\delta}f,\overline{\nabla}_{\delta}u)\delta_{ij}.

Then all the estimates above hold with respect to the hyperbolic metric $\overline{g}$ , that is, $f\in C^{\infty}(\overline{\Omega}\setminus\partial\Sigma)\cup C^{2}(\Omega\cup T)\cup C^{1,\alpha}(\overline{\Omega})$ and $|\overline{\nabla}^{2}f|_{\overline{g}}\in L^{1}(T)$ . Now we obtain the existence and the regularity of the solution of (29).

References

[1] A. D. Aleksandrov “Uniqueness theorems for surfaces in the large. III” In Vestnik Leningrad. Univ. 13.7, 1958, pp. 14–26
[2] Simon Brendle “Constant mean curvature surfaces in warped product manifolds” In Publ. Math. Inst. Hautes Études Sci. 117, 2013, pp. 247–269
[3] Yimin Chen, Yingxiang Hu and Haizhong Li “Geometric inequalities for free boundary hypersurfaces in a ball” In Annals of Global Analysis and Geometry 62.1 Springer, 2022, pp. 33–45
[4] Ailana Fraser and Richard Schoen “Sharp eigenvalue bounds and minimal surfaces in the ball” In Invent. Math. 203.3, 2016, pp. 823–890
[5] Jinyu Guo, Guofang Wang and Chao Xia “Stable capillary hypersurfaces supported on a horosphere in the hyperbolic space” In Adv. Math. 409, Part A Elsevier, 2022, pp. 108641
[6] Jinyu Guo and Chao Xia “A partially overdetermined problem in domains with partial umbilical boundary in space forms” In Adv. Calc. Var. De Gruyter, 2022
[7] Yingxiang Hu, Yong Wei, Bo Yang and Tailong Zhou “A complete family of Alexandrov-Fenchel inequalities for convex capillary hypersurfaces in the half-space” In arXiv preprint arXiv:2209.12479, 2022
[8] Xiaohan Jia, Guofang Wang, Chao Xia and Xuwen Zhang “Heintze-Karcher inequality and capillary hypersurfaces in a wedge” In arXiv preprint arXiv:2209.13839, 2022
[9] Xiaohan Jia, Guofang Wang, Chao Xia and Xuwen Zhang “Alexandrov’s theorem for anisotropic capillary hypersurfaces in the half-space” In Arch. Ration. Mech. Anal. 247.2 Springer, 2023, pp. 25
[10] Xiaohan Jia, Chao Xia and Xuwen Zhang “A Heintze–Karcher-Type Inequality for Hypersurfaces with Capillary Boundary” In J. Geom. Anal. 33.6 Springer, 2023, pp. 177
[11] Sung-Eun Koh and Seung-Won Lee “Addendum to the paper: Sphere theorem by means of the ratio of mean curvature functions” In Glasg. Math. J. 43.2 Cambridge University Press, 2001, pp. 275–276
[12] Pierre Simon Laplace “Traité de mécanique céleste; suppléments au Livre X” In Gauthier-Villars, Œuvres Complètes 4, 1805
[13] Junfang Li and Chao Xia “An integral formula and its applications on sub-static manifolds” In J. Differential Geom. 113.3, 2019, pp. 493–518
[14] Gary M. Lieberman “Mixed boundary value problems for elliptic and parabolic differential equations of second order” In J. Math. Anal. Appl. 113.2, 1986, pp. 422–440
[15] Gary M.Lieberman “Optimal Hölder regularity for mixed boundary value problems” In J. Math. Anal. Appl. 143.2, 1989, pp. 572–586
[16] Sebastián Montiel “Unicity of constant mean curvature hypersurfaces in some Riemannian manifolds” In Indiana Univ. Math. J. 48.2, 1999, pp. 711–748
[17] Filomena Pacella and Giulio Tralli “Overdetermined problems and constant mean curvature surfaces in cones” In Rev. Mat. Iberoam. 36.3, 2020, pp. 841–867
[18] Juncheol Pyo “Rigidity theorems of hypersurfaces with free boundary in a wedge in a space form” In Pacific J. Math. 299.2, 2019, pp. 489–510
[19] Guohuan Qiu and Chao Xia “A generalization of Reilly’s formula and its applications to a new Heintze-Karcher type inequality” In Int. Math. Res. Not. IMRN, 2015, pp. 7608–7619
[20] Robert C. Reilly “Applications of the Hessian operator in a Riemannian manifold” In Indiana Univ. Math. J. 26.3, 1977, pp. 459–472
[21] Antonio Ros “Compact hypersurfaces with constant higher order mean curvatures” In Rev. Mat. Iberoamericana 3.3-4, 1987, pp. 447–453
[22] Julian Scheuer, Guofang Wang and Chao Xia “Alexandrov-Fenchel inequalities for convex hypersurfaces with free boundary in a ball” In J. Differential Geom. 120.2 Lehigh University, 2022, pp. 345–373
[23] Chunquan Tang “Mixed boundary value problems for quasilinear elliptic equations” Thesis (Ph.D.)–Iowa State University ProQuest LLC, Ann Arbor, MI, 2013, pp. 80
[24] Guofang Wang, Liangjun Weng and Chao Xia “Alexandrov–Fenchel inequalities for convex hypersurfaces in the half-space with capillary boundary” In Math. Ann. Springer, 2023, pp. 1–34
[25] Guofang Wang and Chao Xia “Uniqueness of stable capillary hypersurfaces in a ball” In Math. Ann. 374.3-4, 2019, pp. 1845–1882
[26] Liangjun Weng and Chao Xia “Alexandrov-Fenchel inequality for convex hypersurfaces with capillary boundary in a ball” In Trans. Amer. Math. Soc. 375.12, 2022, pp. 8851–8883
[27] Thomas Young “An essay on the cohesion of fluids” In Philos. Trans. Roy. Soc. A The Royal Society London, 1805, pp. 65–87

	$\displaystyle\overline{g}(Y_{n+1},Y_{n+1})=$	$\displaystyle\frac{1}{4}(1+\|x\|^{2})^{2}\overline{g}(E_{n+1},E_{n+1})+\langle x,E_{n+1}\rangle^{2}\overline{g}(x,x)$
		$\displaystyle-\langle x,E_{n+1}\rangle(1+\|x\|^{2})\overline{g}(x,E_{n+1})$
	$\displaystyle=$	$\displaystyle\frac{(1+\|x\|^{2})^{2}-4\langle x,E_{n+1}\rangle^{2}}{(1-\|x\|^{2})^{2}}.$

Some rigidity results on compact hypersurfaces with capillary boundary in the hyperbolic space

Abstract.

1. Introduction

Theorem A.

Theorem 1.

Theorem 2.

Theorem 3.

Theorem 4.

Remark.

Theorem 5.

Remark.

Theorem 6.

Acknowledgements.

2. Preliminaries

2.1. Capillary hypersurfaces and properties of the higher-order mean curvature HrH_{r}

Lemma 1.

Proof.

2.2. Properties in the Poincaré ball model

Proposition 1 (See [25]).

2.3. Properties in the Poincaré half space model

Proposition 2 (See [5]).

3. Minkowski type formulae in the hyperbolic space

3.1. A Minkowski type formula for capillary hypersurfaces in Poincaré ball model

Proposition 3.

Proof.

3.2. A Minkowski type formula for capillary hypersurfaces in Poincaré half space model

Proposition 4.

Proof.

4. Proofs of Theorem 1 and Theorem 3

Theorem 7 (See [13]).

Proof of Theorem 1.

Remark.

Proposition 5.

Proof.

Proof of the Theorem 3.

Remark.

5. Proofs of Theorem 2 and Theorem 4

Proposition 6.

Proof.

Proof of Theorem 2.

Proof of the Theorem 4.

Remark.

Corollary 1.

6. Capillary hypersurfaces in a geodesic ball

Proposition 7.

Sketch of the proof.

Theorem 8.

Proof.

Theorem 9.

Remark.

7. Other rigidity results for capillary hypersurfaces

The proof of Theorem 5.

Proof of Theorem 6.

Remark.

Remark.

Appendix

References

2.1. Capillary hypersurfaces and properties of the higher-order mean curvature $H_{r}$