Relevant scales for the -metric with positive cosmological constant
Abstract
In this work, we study the weak and strong gravitational lensing in the presence of an accelerating black hole in a universe with positive cosmological constant . First of all, we derive new perturbative formulae for the event and cosmological horizons in terms of the Schwarzschild, cosmological and acceleration scales. In agreement with previous results in the literature, we find that null circular orbits for certain families of orbital cones originating from a saddle point of the effective potential are allowed and they do not exhibit any dependence on the cosmological constant. They turn out to be Jacobi unstable. We also show that it is impossible to distinguish a -black hole from a -black hole with if we limit us to probe only into effects associated to the Sachs optical scalars. This motivates us to analyze the weak and strong gravitational lensing when both the observer and the light ray belong to the aforementioned family of invariant cones. In particular, we derive analytical formulae for the deflection angle in the weak and strong gravitational lensing regimes.
pacs:
04.20.-q,04.70.-s,04.70.BwI Introduction
The -metric with a positive cosmological constant is a special case of the Plebnski-Deminski family of metrics Griff0 . It can be obtained from the line element (23) in Griff by setting the rotation parameter and further imposing that the electric and magnetic charges vanish, that is and it describes two causally disconnected black holes of mass each accelerating in opposite direction due to the presence of a force generated by conical singularities located along the axes and Griff . More precisely, in Boyer-Lindquist coordinates and in geometric units () the line element is expressed as
| (1) |
with
| (2) |
where , and . Note that denotes the event horizon, is the acceleration parameter and is the cosmological horizon. We present a detailed analysis of the horizons and their spatial ordering in terms of the relevant physical parameters in the section. Here, it suffices to mention that while for the case of a -metric the Schwarzschild horizon is smaller than the acceleration horizon whenever , it is not clear a priori if the same condition ensures that in the case of a -metric with positive cosmological constant. Furthermore, by adapting the tetrad (7) in Griff to the present case the only non-zero component of the Weyl tensor is
| (3) |
The above expression confirms that the spacetime with line element (1) is of algebraic type D and the only curvature singularity occurs at . Hence, the horizons and are just coordinate singularities. It is interesting to observe that for the metric in (1) becomes the metric of a Schwarzschild-de Sitter black hole while in the case of but (1) correctly reproduces the line element associated to the -metric as given in Maha . This fact means that any prediction regarding the bending of light in a manifold described by (1) should reproduce the corresponding results for the Schwarzschild-de Sitter case in the limit of as well as the gravitational lensing results for the -metric obtained in Maha when we let with kept constant. According to Griff , the conical singularity on can be removed by making the following choice for the parameter entering in the range of the angular variable
| (4) |
while the conical singularity with constant deficit angle along the half-axis which is computed by means of (22) in Griff as
| (5) |
can be explained in terms of a semi-infinite cosmic string pulling the black hole and/or of a strut pushing it. Similarly as for the -metric, this allows us to think of (1) as of a Schwarzschild-de Sitter-like black hole experiencing an acceleration along the direction due to the presence of a force, i.e. the tension of a cosmic string. Moreover, we observe that the length of interval for the range of the coordinate can be transformed to its standard value with the help of the rescaling so that . Moreover, Kof was able to provide an interpretation of the string/strut in terms of null dust. In the rest of this paper, we will work with the line element obtained after the aforementioned rescaling is introduced, namely
| (6) |
where
| (7) |
with , and given in (2).
In the present work, we study the geodesic motion of a massive particle and the light bending in a two black hole metric with positive cosmological constant. For this end, a preliminary study of the behaviour of the null geodesics turns out to be convenient in detecting some features of strong gravity in the aforementioned spacetime. The question of new phenomena arises if we consider a metric which in some limiting case reduces to the Schwarzschild metric (for examples see we1 ). Here, the fate of the circular orbit, already appearing in the Schwarzschild metric, and issues regarding its stability deserve careful attention because they will give us useful insights on how to construct an appropriate impact parameter. While the -metric has been extensively studied in the last decades, the same cannot be said for its counterpart with . The independence of null geodesics on the cosmological constant was first recognized in a seminal and extremely comprehensive paper on photon surfaces by Claudel where a general class of static spherically symmetric space-times was considered. The case of non spherically symmetric manifolds was addressed by Gibbons . There, among several physically relevant space-times, the case of a -metric with cosmological constant was studied and the authors discovered that a non-spherically symmetric photon surface continues to exist even in that scenario. The conclusion is that, while the Schwarzschild metric exhibits a photon sphere, the same cannot be said for the -metric. More precisely, Gibbons showed that instead of a photon sphere there is a photon surface displaying at least one conical singularity.
Regarding geodesic motion in a -black hole, radial time-like geodesics were analyzed by Far whereas the study of the circular motion of massive and massless particles was undertaken by Pravda . Moreover, Lim ; Bini0 offered an exhaustive treatment of time-like and null geodesics by a mixture of analytical and numerical methods. Finally, Bini probed into the motion of spinning particles around the direction of acceleration of the black hole. Furthermore, Maha determined the coordinate angle of the so-called photon cone and performed a Jacobi stability analysis to show that all circular null geodesic on the photon cone are radially unstable. The shadow of a -black hole was studied in Gr1 ; Gr2 while Frost derived inter alia an exact solution of the light-like geodesic equation by means of Jacobi elliptic functions and determined the angular radius of the shadow. We should also mention that the analysis of the light-like geodesics and the black hole shadow for a rotating -metric have been addressed in ZZ . Moreover, other probed into photon spheres and black hole shadows for dynamically evolving spacetimes. Finally, we refer to QNMs1 ; QNMs2 for the analysis of the QNMs and the stability properties for a -black hole.
Regarding a -black hole in an anti-de Sitter (AdS) or a de Sitter (dS) background, Griff1 ; Griff2 ; Dias1 ; Dias2 ; Krt1 ; Poldo ; Krt2 ; Xu studied in detail the geometric structure and the related properties of these spacetimes. The analysis of the circular motion of massive and massless particles in the -metric with a negative cosmological constant was performed by Chamb where the author proved that the circular null geodesics are unstable whereas Poldo completed the study of Chamb by considering some special characteristics associated to the null geodesics in the aforementioned metric. Recently, Lim in Lim21 classified all possible trajectories for photons in the (A)dS -metric in terms of the particle angular momentum and the energy scaled in units of the Carter constant. Similarly as in Frost , it was possible to construct exact solutions for null geodesics by means of Jacobi elliptic functions. However, the stability problem for such trajectories has not been addressed by Lim21 . We complete the results of Lim21 by concentrating on the interplay of different scales in the potentially observable or physical relevant case. To make a comparison with known results of the Schwarzschild- de Sitter metric more accessible, we work in Boyer-Lindquist coordinates.
Concerning gravitational lensing, detailed studies on light bending in the weak and strong regimes were pioneered by the George Ellis lensing group. Some of their exciting results which have relevance to the present work are Virb ; Virb1 ; Virb2 ; Virb3 ; Virb4 . A nice and thorough review article on this subject has been written by Perl . Finally, Gibbons01 exploited a novel geometrical approach based on the Gauss-Bonnet theorem applied to the optical metric of the gravitational lens in order to derive weak leansing formulae for spherically symmetric metrics generated by certain static, perfect non-relativistic fluids. Regarding the gravitational lensing Frost derived a lens equation and showed that the lens results of Ifti for the rotating -metric with NUT parameter does not contain as a special case the -metric (where both the acceleration and NUT parameters are set equal to zero). Moreover, Maha studied the strong and weak lensing for null rays on the photon cone. To the best of our knowledge, we could not identify any paper studying the bending of light and analyzing the (in)stability problem of null circular orbits for the -metric with positive cosmological constant. We hope to fill this gap with the present work.
The remainder of the paper is structured as follows. In Section II, we analyze the horizon structure of the dS -metric in terms of certain orderings among the Schwarzschild, the cosmological and the acceleration scales. In order to understand which scale orderings are physically relevant, we consider three typical black hole representatives: ultramassive, massive and light. In Section III, we derive the effective potential for massive and massless particles and we show that the null-orbits have the same radius and and take place on the same family of invariant cones as in the -metric, i.e. they do not depend on . This result is in agreement with Gibbons . Moreover, we find that the circular orbit is due to a saddle point in the effective potential, which requires an additional effort to probe into the associated stability problem. This is addressed in Section IV where we perform the Jacobi (in)stability analysis of the circular orbits. In Section V, since the Sachs optical scalars cannot be used to optically distinguish between - and a dS -black holes, we study the the gravitational lensing in the weak and strong regimes. More precisely, the corresponding deflection angles are analytically computed when the light propagation occurs on a certain family of invariant cones, and their dependence on the observer position is shown. Our formulae correctly reproduce the corresponding ones in the -metric case in the limit of vanishing and indicate that the deflection angles may depend on the cosmological constant if the position of the observer is close to the cosmological horizon.
II Analysis of the horizons
The structure of the horizons for the metric associated to the line element (1) can be unraveled by analyzing the roots of the equation with given as in (2). To this purpose, it is convenient to introduce the Schwarzschild, the cosmological and the acceleration length scales defined as , and , respectively. The appearance of several scales in the metric makes a precise study of the horizons a worthwhile undertaking since a priori it is not clear what structure of the horizons will emerge. Apart from that, we recall a curious fact from the Schwarzschild de Sitter metric with two horizons, one dominated by and the second one (the cosmological horizon) by . The Boyer-Lindquist coordinates which one uses to study this metric are valid within these two horizons where we locate ourselves and the rest of the universe. An observer outside might even claim that we are living inside a black hole. It is interesting to reconsider the unique position for the case of the C-metric as more scales enter the calculation. In terms of these scales the equation gives rise to the following cubic equation
| (8) |
with
| (9) |
First of all, we observe that the extrema of the cubic in (8) are located at
| (10) |
with , and moreover,
| (11) |
Since as , we conclude that is a maximum. The fact that for any positive value of the scales, as it can be immediately seen from (11), together with the observation that , allows us to conclude that the cubic (8) admits always a negative root, here denoted by while the other two zeroes may be positive and distinct, having algebraic multiplicity or being complex conjugate of each other. However,the scenario where the two positive roots coincide, i.e. the discriminant of (8) vanishes, is not physically relevant because as the discriminant tends to zero, the region between the two positive roots gets smaller and smaller and it is impossible to define a static observer in most of the spacetime. Furthermore, the case of two complex conjugate roots corresponds to the presence of a naked singularity at . Since the cubic (8) depends on the three scales , , and , it is imperative to understand which orderings among these scales are physically relevant. To this purpose, we consider three typical black hole representatives: ultramassive BHs such as TON618 in Canes Venatici TON whose Schwarzschild radius is times the distance from Pluto to the Sun (see Table 1), massive BHs like Sagittarius A∗ at the galactic centre of the Milky Way SAG whose event horizon is approximately times the sun radius and light BHs such as GW170817 in the shell elliptical galaxy NGC 4993 ABB with an event horizon diameter of km.
| BH name | (m) | (m/s2) | (m/s2) | |||
|---|---|---|---|---|---|---|
| TON618 | ||||||
| Sagittarius A∗ | ||||||
| GW170817 |
From Table 1, we immediately observe that we can always assume . Regarding the acceleration parameter , it is important to observe that for -black holes with cosmological constant the only constraint we need to impose on is that . The situation is dramatically different in the case of the -metric where the black hole mass and the acceleration parameter must satisfy the condition which is equivalent to require that . Let us discuss and interpret the roots of (8) for the following cases
-
1.
: in this scenario, given the acceleration parameter of the black hole in SI units is
(12) See Table 1 for typical values for . Note that this case has no corresponding physical counterpart for a -metric because in the limit of we would have a -BH such that the event and acceleration horizons coincide. Setting in (8) and introducing the small parameter , the discriminant of the reduced cubic is
(13) which is clearly positive. This observation together with the remark below equation (11) allows us to conclude that there is one negative root and two complex conjugate roots. Hence, this is the case of a naked singularity at and the coordinate can be extended up to space-like infinity. In comparison a naked singularity is not possible in the Schwarzschild-de Sitter metric.
-
2.
: if we rewrite (8) as
(14) we see that is the small parameter and a straightforward application of perturbation methods for algebraic equations shows that the event horizon and the cosmological horizon are represented by the following expansions
(15) (16) From the point of view of the horizon structure this is an interesting case. Even if appears in the corrections, the horizon associated with it disappears and in its place, we encounter which we could rightly call the acceleration horizon. As a consequence, we locate our position within the acceleration horizon. Note that the same expansion holds also for .
-
3.
: in this regime the acceleration parameter is completely determined by the cosmological constant and is given in SI units as
(17) Since , it must be . As we can see from the last column in Table 1, such a condition is violated by ultramassive and massive black holes. Hence, the present case may be relevant for light black holes such as GW170817. By means of the rescaling and the introduction of the same small parameter already defined in 1. we can rewrite (8) as
(18) The discriminant of the associated reduced cubic is
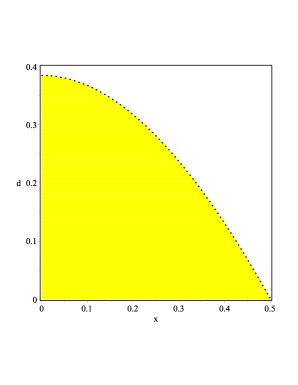
(19) From Fig. 1 we observe that also in this case the discriminant may become positive. More precisely, we have three distinct real roots if while the naked singularity case occurs when . Applying again perturbation methods to find expansions for the positive roots of (18) yields

Figure 1: Plot of the discriminant (19) for . (20) (21) This case resembles indeed the Schwarzschild-de Sitter order of horizons.
We conclude this section by observing that in general equation (8) can be transformed into the reduced third order polynomial equation
| (22) |
by means of the variable transformation . According to Bron the associated discriminant is
| (23) |
and we have the following classification
-
1.
three distinct real roots for ;
-
2.
two real roots where one root has algebraic multiplicity two whenever ;
-
3.
one real and two complex conjugate roots for .
Since the first case is physically relevant, we will stick to the condition . Then, if we introduce the additional parameter and the auxiliary angle defined as , then the roots are parametrized with the help of trigonometric functions and their inverses in the following form
| (24) |
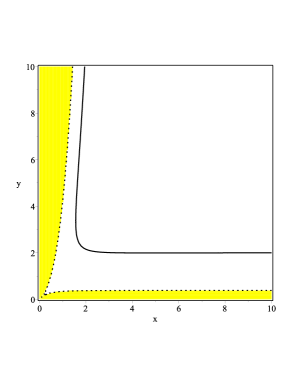
We observe that in addition to the inequality , there is the additional constraint that . These two constraints are not satisfied for any value of the scales entering in our problem as it can be seen in Figure 2. However, it can be seen that the condition ensures that .
III Geodesic equations and effective potential
When we turn our attention to the study of the geodesic motion, in addition to , and , a new scale associated to the angular momentum of the particle enters the scene. To probe into the interplay of these scales, the method of the effective potential seems most adequate. One might expect that the many new scales as compared to the Schwarzschild-de Sitter metric will result into a richer structure of critical points. It will turn out that this is partly true, but only if we pay careful attention to the emergence of saddle points. To study the motion of a particle in the gravitational field described by (6), we need to analyze the geodesic equations FL
| (25) |
subject to the constraint
| (26) |
with and for light-like and time-like particles, respectively. The system of coupled ODEs associated to (25) can be immediately obtained from equations (8)-(11) in Maha by replacing , and therein with the functions , and defined in (7) and noticing that the functions and remain the same. In view of this observation, one can proceed as in Maha and conclude that the dynamics is governed by the following coupled system of ODE
| (27) | |||||
| (28) |
where and are the energy per unit mass and the angular momentum per unit mass of the particle, respectively. Moreover, the constraint equation (26) can be cast into the form
| (29) |
where and the effective potential is given by
| (30) |
At this step, it is gratifying to observe that in the limit of vanishing and equation (29) reproduces correctly equation (25.26) in FL for the Schwarzschild case. Moreover, the functions , and are non negative for and therefore, as in classical mechanics. Finally, the equality, , corresponds to a circular orbit and a critical point of the effective potential. Since in the present work we are interested in the study of the light bending, we recall that in the case of null geodesics and hence, the effective potential simplifies as follows
| (31) |
To study the null circular orbits for the potential (31), we need to find its critical points. Imposing that leads to the following equations
| (32) |
At this point a comment is in order. First of all, the above equations does not contain . This is surprising because the spacetimes described by (6) and the -metric are not conformally related. The same phenomenon occurs when we study the null circular orbits for the Schwarzschild and Schwarzschild-de Sitter black holes, i.e. in both cases the corresponding photon spheres are characterized by a typical radius which is -independent Claudel . Hence, we can conclude as in Maha that null geodesics admit circular orbits with radius
| (33) |
only for a certain family of orbital cones with half opening angle given by
| (34) |
Note that due to the fact that and is a monotonically decreasing function in the variable , it follows that cannot take every value from to . More precisely, it can only vary on the interval with . To classify the critical point of our effective potential, we compute the determinant of the Hessian matrix associated with the effective potential (31) at the critical point . We find that the determinant of the Hessian matrix is
| (35) |
with , , . The functions and are the same as those computed in Maha , namely
| (36) | |||||
| (37) |
while the new contribution due to the cosmological constant is encoded in the function which is given by
| (38) |
Note that in the limit of equation (35) reproduces correctly (31) in Maha . From the analysis performed in Maha we already know that the function is always positive for . This signalizes that the sign of (35) is controlled by the term which is positive for in the interval and
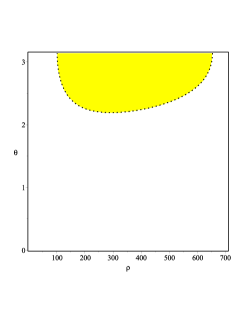
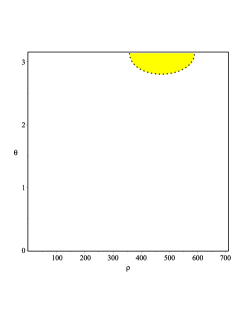
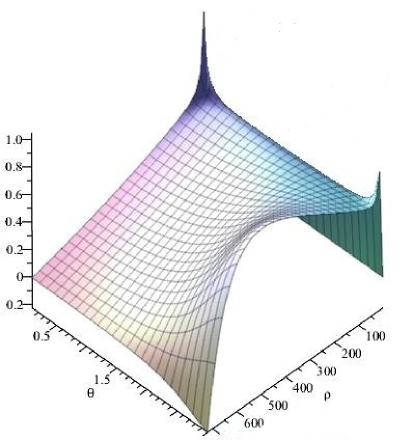
| (39) |
where is the function representing the dotted boundary of the yellow region in Fig. 3. Since black holes of astrophysical interest are characterized by , this implies that . The yellow part in Fig. 3 represents the region in the space of the parameters and where the function is positive. This is clearly the case for and . Hence, we conclude that the critical point of the effective potential is a saddle point.
III.1 Geodesic motion for massive particles
We study the motion of test particles for the -metric with positive cosmological constant. Since there are involved three physical scales, we may expect that they may combine in such a way to lead to new results. We recall that the equation of motion for a massive particle with proper time in the aforementioned metric is given by (29) with replaced by while the effective potential is represented by (30) with . In the case , the effective potential reads
| (40) |
By means of the rescaling , and in the regime we can cast (40) into the form
| (41) |
Concerning the behaviour of the effective potential at the cosmological horizon, we observe that at the quadratic order in the small parameter
| (42) |
and hence, is always negative there for any . Regarding the motion of a time-like particle, it follows from (29) that the particle dynamics is constrained to those regions where the reality condition
| (43) |
is satisfied. In the case displayed in Fig. 4, we see that depending on the value of the parameter , the geodesics can reside in different regions. For example, if , the particle neither falls into the event horizon nor into the cosmological horizon. More precisely, it stays inside the yellow compact region displayed in the first two panels of Fig. 4. Such a region becomes smaller as increases. When crosses a critical value , the particle will fall into the event or cosmological horizon.
Regarding the critical points of the effective potential (41), the condition is satisfied whenever or . In the case , it is not difficult to check that the geodesic equations (27) and (28) stay finite at the axes or . This signalizes that mathematically speaking, we can probe into geodesics going through the poles. As it was already noticed by Lim , such geodesics are not physically possible because the particles moving along these trajectories would undergo a collision with the cosmic string/strut causing the black hole to accelerate. This problem can be circumvented if we imagine these time-like geodesics to be arbitrarily close to the axis or , while keeping the geodesic equations at or as an approximation. In the following, we focus on the case which has not been covered by Lim21 .
III.1.1 Time-like radial geodesics along
If we impose that along the north pole, we end up with the cubic equation
| (44) |
Descartes’ rule of signs implies that that there is only one positive root, here denoted by because the polynomial (44) exhibits only one sign change due to the fact that ensures that the term is positive. On the other hand,
| (45) |
is negative, and therefore, the equilibrium point is unstable. This also signalizes that is a maximum for the effective potential. This implies that the associated geodesic is unstable and under any small perturbation, the particle will either cross the event horizon of the black hole or approach the acceleration horizon. The same behaviour occurs in the case of a vanishing cosmological constant. The latter scenario was studied in Lim . For typical values of we refer to Table 2.
| 1 | 706.857 | 22.606 | 499.875 | |
|---|---|---|---|---|
| 1 | 7070.817 | 70.959 | 4999.875 | |
| 1 | 70710.428 | 223.856 | 49999.875 |
In order to find an analytic expression for the maximum by applying the perturbative theory of algebraic equations, it is convenient to use a different rescaling, namely . Then, and the polynomial equation (44) becomes
| (46) |
Since is a small parameter and the associated unperturbed polynomial has roots at a straightforward application of perturbation methods for algebraic equations Murd shows that (46) has roots at
| (47) |
Moreover, Descartes’ rule of signs implies that that there is only one positive root because the polynomial (46) exhibits only one sign change due to the fact that ensures that the term is positive. Hence, we can conclude that are negative and the only positive critical point is represented by the root . From case 3. in Section II the cosmological horizon is located at . On the other hand, we find at quadratic order in that if while for . Since , we conclude that the critical point is given by
| (48) |
and by the analysis we performed previously, it must be a maximum for the effective potential.
III.1.2 Time-like radial geodesics along
In this scenario, the corresponding cubic equation is
| (49) |
If we apply Descartes’ rule of signs, we conclude that there are always 2 complex conjugate roots and one positive real root, here denoted by because the polynomial (49) exhibits three sign changes due to the fact that makes the term positive. Moreover,
| (50) |
from which we conclude that is not an equilibrium point for time-like particles moving along the south pole. Hence, a small perturbation will cause the particle to be either swallowed by the event horizon or to approach the cosmological horizon. For typical values of we refer to Table 2. Also in this case it possible to obtain an analytical expression for the maximum of the effective potential. Proceeding as before, we can rewrite (49) as
| (51) |
which has been obtained from (49) by setting so that . The unperturbed polynomial has roots at , , and if we apply perturbative methods, it can be easily verified that there are two complex conjugate roots and one real root given by . In particular, we have if and for . Since , we conclude that . Finally, we find that
| (52) |
III.1.3 The case
For , the rescaled effective potential in the case reads
| (53) |
with , and . Concerning the behaviour of the effective potential at the cosmological horizon, we observe that at the quadratic order in the small parameter
| (54) |
with and
| (55) |
At this point a comment is in order. Since the polynomial in (55) has roots at , the potential will diverge at the cosmological horizon along the rays and , i.e. along the direction of the cosmic string. On the other hand, for , the polynomial function is always positive as it can be seen from Fig. 5 and therefore, we conclude that for each fixed value of the effective potential takes on a negative value at the cosmological horizon.
In the following, we perform a numerical analysis of the critical points of . Imposing and leads to the following coupled system of algebraic equations
| (56) | |||
| (57) |
with
| (58) | |||||
| (59) | |||||
| (60) | |||||
| (61) | |||||
| (62) | |||||
| (64) | |||||
| (65) | |||||
| (66) |
First of all, we observe that even though are roots for the equation (57), they must be disregarded because the effective potential is singular there. Concerning the roots of the polynomial , Descartes’ rule of signs signalizes the presence of two or zero positive roots. However, these roots are not relevant to the present analysis because they coincide with the event and cosmological horizons. This can be easily seen by rewriting as the cubic equation (18) and taking into account that . These observations tell us that it suffices to consider the following system
| (67) |
In the Table 3, we classified the critical points of the effective potential (53) for in the range and between and . We observe that for small values of the potential admits only saddle points. However, as increases, a local minimum develops even if the values of the parameter decreases. In Table 4, we focus on the dynamics of the local minimum when increases while remains fixed. More precisely, a local minimum exists only if varies between some and . In particular for , we find and .
| (rad) | Type | |||
|---|---|---|---|---|
| 22.591 | 0.055 | sp | ||
| ” | 70.930 | 0.041 | sp | |
| ” | 223.803 | 0.031 | sp | |
| 22.449 | 0.175 | sp | ||
| ” | 70.666 | 0.131 | sp | |
| ” | 223.329 | 0.098 | sp | |
| 1 | 20.764 | 0.593 | sp | |
| ” | 67.776 | 0.428 | sp | |
| ” | 218.340 | 0.315 | sp | |
| 1.731 | 18.741 | 0.845 | sp | |
| ” | 64.980 | 0.584 | sp | |
| ” | 213.917 | 0.422 | sp | |
| 1.7317 | 2.998 | 1.561 | sp | |
| ” | ” | 3.007 | 1.561 | lm |
| ” | ” | 18.738 | 0.845 | sp |
| ” | 64.977 | 0.584 | sp | |
| ” | 213.912 | 0.422 | sp | |
| 1.733 | 2.892 | 1.562 | sp | |
| ” | ” | 3.122 | 1.560 | lm |
| ” | ” | 18.734 | 0.846 | sp |
| ” | 2.90377 | 1.56999 | sp | |
| ” | ” | 3.10287 | 1.56975 | lm |
| ” | ” | 64.972 | 0.585 | sp |
| ” | 2.90390 | 1.57071 | sp | |
| ” | ” | 3.10267 | 1.57069 | lm |
| ” | ” | 213.904 | 0.422 | sp |
| (rad) | Type | ||
|---|---|---|---|
| 1.8 | 2.358 | 1.566 | sp |
| ” | 4.136 | 1.548 | lm |
| ” | 18.488 | 0.871 | sp |
| 2.2 | 1.856 | 0.157 | sp |
| ” | 8.115 | 1.462 | lm |
| ” | 16.499 | 1.042 | sp |
| 2.4 | 1.773 | 1.569 | sp |
| ” | 11.059 | 1.356 | lm |
| ” | 14.406 | 1.185 | sp |
| 2.438 | 1.761 | 1.569 | sp |
| ” | 12.492 | 1.290 | lm |
| ” | 13.131 | 1.257 | sp |
| 2.440 | 1.760 | 1.569 | sp |
| 10 | 1.511 | 1.570 | lm |
| 1.500 | 1.570 | sp |
One can see from Table 4 the prevalence of saddle points. It is tempting to call the effective potential of the C-metric with positive cosmological constant the potential of saddle points. The latter is positive and becomes negative at large (see equation (42)). This achieved by a saddle point as we demonstrate in Fig. 7.
IV Jacobi stability analysis of the null circular Orbits
Since we do not know a priori which effect has on the stability of the null circular orbits found in the previous section, we need to study once again the salient features of the associated Jacobi stability problem. To this purpose, we need first to verify that the critical point of the effective potential is also a critical point for the geodesic equations (27) and (28). In that regard, it is convenient to rewrite (27) and (28) with the help of the constraint equation (29) and the definition of the effective potential (31) as follows
| (68) | |||
| (69) |
For a circular orbit with and all derivatives in the above equations vanish and we are left with the following system of equations
| (70) |
that can be simplified as follows
| (71) |
Since
| (72) |
and the derivatives above vanish when evaluated at and , we conclude that the equations in (71) are trivially satisfied. In order to study the Jacobi (in)stability of the null circular orbits, we will proceed as in Maha , that is, we first recognize that the equations (68) and (69) are a special case of the dynamical system
| (73) |
where
| (74) | |||||
| (75) |
with , , and for and then, we apply the Kosambi-Cartan-Chern (KCC) theory which has been widely used in the last decade as a powerful toll to probe the stability of several dynamical systems appearing in gravitation and cosmology 21 ; 22 ; 23 ; 28 ; H ; Baha ; Bom ; Bom1 ; Harko1 ; Danila ; Lake . Let us assume that and are smooth functions in a neighbourhood of the initial condition . The main result we will use is the following theorem: an integral curve of (73) is Jacobi stable if and only if the real parts of the eigenvalues of the second KCC invariant are strictly negative everywhere along , and Jacobi unstable otherwise. We recall that
| (76) |
where is called the Berwald connection Anto ; Miron . Observe that the term in (76) does not contribute because the system (73) is autonomous in the variable . For a proof of the above result we refer to 1 ; 6 ; Bom . In preparation to the application of this theorem, we introduce the matrix associated to the second KCC invariant, namely
| (77) |
where a tilde means evaluation at and . The associated characteristic equation for the eigenvalues is
| (78) |
First of all, we observe that with vanishes along the null circular orbit. This implies that the third term on the r.h.s. of the first equation in (76) does not give any contribution. By the same token, defined in (76) depends quadratically in and and hence, its first order partial derivatives with respect to are linear combinations in vanishing once evaluated at and . Hence, we have
| (79) |
After a lengthy but straightforward computation we find that
| (80) | |||||
By means of the first equation in (71) we immediately conclude that . This implies that the eigenvalues of the matrix (77) are given by
| (81) |
Let us analyze the sign of . We observe that by means of (71)
| (82) | |||||
Since the energy is positive, and is positive on the interval , we conclude that and the eigenvalue is always strictly positive. This implies that a photon circular orbit with radius on the cone are Jacobi unstable.
V Gravitational lensing
The cosmological constant seems to be an obstacle in calculating the deflection angle of light in a curved spacetime as it can be evinced from Arakida where a comparison of the different results in the Schwarschild-de Sitter spacetime has been provided. It is therefore of some interest to perform the calculation of light deflection in the C-metric with . We recall that distance measures, image distortion and image brightness of an astrophysical object hidden by a gravitational lens require the analysis of the equation of geodesic deviation and in particular the derivation of the so-called Sachs optical scalars allowing to study the null geodesic congruences Sachs ; Wald ; Seitz . Without much further ado we observe that a construction of a symmetric null tetrad in the spirit of Carter (see equation (5.119) therein) can be performed as in Maha by replacing there the function by given in (2). However, this procedure leads to a non-vanishing spin coefficient , thus signalizing that the null geodesics are not affinely parameterized. The solution to this problem consists in realizing that the spin coefficient is zero also in the case of the metric (6) and therefore, the construction of an affine parameterization can be achieved in terms of a rotation of class III (see 7(g) eq. (347) in CH ) which preserves the direction of the tetrad basis vector while keeping . As a consequence of this approach, the spin coefficient will provide access to the Sachs optical scalar describing the shear effect on the light beam due to the gravitational field. To this purpose, we consider the normalized null tetrad
| (83) |
and we recall that in the Newmann-Penrose formalism the ten independent components of the Weyl tensor are replaced by five scalar fields while the ten components of the Ricci tensor are expressed in terms of the scalar fields with and the Ricci scalar is written by means of the scalar field . The spin coefficients for our problem are computed to be
| (84) | |||||
| (85) |
and the only non-vanishing scalar fields , , and for a two black hole metric with positive cosmological constant are
| (86) | |||||
| (87) | |||||
| (88) |
At this point a remark is in order. First of all, the cosmological constant enters only in the spin coefficients and while the other spin coefficients are the same as those obtained for the -metric in Maha . Moreover, the fact that is real has a twofold implication: the congruence of null geodesics is hypersurface orthogonal and accordingly, the optical scalar must vanish. In other words, a light beam propagating in the metric described by (6) does not get twisted or rotated. Furthermore, if we consider equations (a) and (b) (see 8(d) p. 46 in CH ) describing how the spin coefficients and vary along the geodesics
| (89) | |||||
| (90) |
we immediately observe that the second equation is of no practical use because it is always trivially satisfied (). In addition, the vanishing of the spin coefficient is signalizing that a light beam does not experience any shear effect, i.e. if the light beam has initially a circular cross section such a cross section does not change its shape after the interaction with the black hole took place. Finally, the optical scalar which measures the contraction/expansion of a light beam travelling through the given gravitational field, is expressed in terms of the spin coefficient as
| (91) |
The fact that is negative implies that the light beam undergoes a compression process in the presence of a two black hole metric with positive cosmological constant. However, as does not depend on and it coincides with the corresponding optical scalar computed for the -metric in Maha , it is impossible to distinguish a -black hole from the one described by (6) if we limit us to probe only into effects in the optical scalar . This observation suggests that we need to study the weak and strong gravitational lensing in order to detect some distinguishing features among the aforementioned black hole solutions. We start by observing that in our situation the weak lensing problem can be tackled by a method similar to that adopted in Maha due to the fact that the saddle point of the effective potential (31) coincides with the critical point of the dynamical system (27)-(28). As in Maha , we will assume that the light ray and the observer are positioned on the cone . Then, the angular motion is controlled by the equations
| (92) |
the time-like variable is linked to the parameterization according to
| (93) |
while the radial motion is described by the following equation obtained by combining (29) with (31), namely
| (94) |
In order to determine the trajectory on the cone , a trivial application of the Chain Rule to combined with (94) leads to
| (95) |
where without loss of generality we picked the plus sign corresponding to a null ray approaching the black hole along an anticlockwise trajectory. Moreover, like in Weinberg , the quantity has the interpretation of an impact parameter defined as
| (96) |
where is the distance of closest approach and denotes the event horizon. In order to check the validity of (96), let us choose with denoting the radius of the circular orbits and recall that the critical impact parameter in the case of the Schwarzschild-de Sitter metric is given by usPRD
| (97) |
where the gravitational lensing can only be studied for because as from the left the event and cosmological horizons of the Schwarzschild-de Sitter black hole would shrink and coalesce with the radius of the photon sphere at . Then, the critical impact parameter can be obtained from (96) as
| (98) |
Moreover, let us remind the reader that in the case of vanishing acceleration, i.e. , our metric goes over into the Schwarzschild-de Sitter metric. A Taylor expansion of (98) around with leads to
| (99) |
It is gratifying to observe that (99) correctly reproduces the Schwarzschild-de Sitter critical impact parameter in the limit while it also agrees for with the critical impact parameter for a -black hole (see equation (93) in Maha ). Having determined the critical impact parameter for our problem allows to distinguish among the following scenarios
-
1.
if , the photon is captured by the black hole;
-
2.
if , deflection takes place and two further cases are possible, namely
-
(a)
if or equivalently , the trajectory is almost a straight line and we are in the regime of weak gravitational lensing.
-
(b)
If or equivalently , strong gravitational lensing occurs with the photon orbiting several times around the black hole before it flies off.
-
(a)
If we go back to (95), we observe that the function can never be negative while the same can not be said for the other square root. This means that some motion reality condition should be introduced. This can be easily done by rescaling the radial variable according to and setting and . Then,
| (100) |
and by means of (96) and (7) equation (95) becomes
| (101) | |||||
| (102) |
where is the rescaled distance of closest approach. At this point, it is interesting to observe a couple of facts. First of all, there is no dependence on the cosmological constant in the expression above. This feature is already present in the Schwarzschild-de Sitter case usPRD where can influence the trajectories of massive particles while it is absent in the coordinate orbital equation when photons are considered Islam . However, the cosmological constant can appear in the formula for the deflection angle in the weak regime when the observer is close to the cosmological horizon. In addition to the previous remark, equation (102) coincides with equation (98) in Maha . This implies that the analysis of the turning points performed by Maha for the -metric will continue to hold also in the present case. For this reason, we will limit us to recall only those basic facts that are necessary in order to proceed further with the analysis of the weak/strong gravitational lensing. First of all, we remind the reader that under the assumption we have and therefore, . Moreover, the cubic equation in (102) admits three real turning points, namely and where an analytic expression for is given by (100) in Maha . When integrating (102) is extremely important to know the spatial ordering of the points , , , and . For a proof of the results summarized here below we refer to Appendix C in Maha .
-
1.
Weak lensing: . If with representing the radius of the Schwarzschild photon sphere, it follows that for any . This implies that and the cubic in (102) is positive on the interval .
-
2.
Strong lensing: for . If is in the aforementioned range, then and . This ensures that the cubic in (102) is positive on the interval .
Let us focus on the weak gravitational lensing. By we denote the position of the observer which must be placed in the interval . At this point, by means of the angular transformation we can integrate (101) and cast the integral into the form
| (103) |
We remind the reader that, unlike the Schwarzschild case, the observer cannot be positioned in an asymptotic region approximated by the Minkowski metric. To overcome this problem, we assume that the deflection angle is described by the formula usPRD
| (104) |
with unknown constants and to be fixed so that the weak field approximation of (104) coincides with the weak field approximation for the Schwarzschild case in the limit of vanishing cosmological constant and acceleration parameter. The integral in (104) can be rewritten as
| (105) | |||||
| (106) |
For the discussion on why it is possible to apply a perturbative expansion in the small parameters and we refer to Maha . Therefore, let us expand as follows
| (107) |
with
| (108) | |||||
| (109) |
If we take into account that
| (110) |
and we let the integration over the functions and to be followed by an asymptotic expansion in powers of , the deflection angle in (104) becomes
| (111) |
where
| (112) | |||||
| (113) | |||||
| (114) |
In order to fix the unknown constants and in (111), we observe that in the limit of , the cosmological horizon and therefore, we can let in the above expressions. If in addition , equation (111) must reproduce the weak deflection angle for a light ray in the Schwarzschild metric. This is the case if and . Hence, at the first order in , we find that the weak deflection angle can be written as
| (115) |
Taking into account that for a vanishing cosmological constant, we can let , it is straightforward to check that (115) correctly reproduces the weak deflection angle formula (115) for the -metric obtained in Maha .
Regarding the strong gravitational lensing for the metric under consideration, we first observe that it can be analyzed by the same procedure adopted by Maha because in both cases the metrics involved admits the same family of null circular orbits. For this reason, we will not dive into the details of the derivation and we will limit us to remind the reader that one first solves the integral (104) in terms of an incomplete elliptic function of the first kind followed by an application of an asymptotic formula for the aforementioned elliptic function obtained by KNC when the sine of the modular angle and the elliptic modulus both approach one. The same strategy has been already successfully used in usPRD to derive the Schwarzschild deflection angle in the strong regime with a higher degree of precision than the corresponding formulae in Darwin ; Bozza . Without further delay, let us recall that it was found in Maha that the deflection angle in the strong gravitational lensing regime is given by
| (116) |
with
| (117) | |||||
| (118) |
A validity check of (116) was already run in Maha where it has been verified that (116) correctly reproduces the corresponding strong lensing formula in the Schwarzschild case as given in usPRD ; Bozza when . The main difference between (143) in Maha and (116) revolves around , i.e. the position of the observer, which in the present case is bounded from above by the cosmological horizon. It is interesting to observe that, in general, the above formula does not depend on . However, a dependence on the cosmological constant emerges only in the case the observer is placed very close to the cosmological horizon as it was already pointed out by usPRD in the context of the Schwarzschild-de Sitter metric.
VI Conclusions
In this paper, we have focussed on the classical connection between light and gravity, more precisely, the bending of light in a gravitational field and its lensing. For the -metric with positive cosmological constant, we showed that the effective potential for a massless particle exhibits a saddle point. If on one hand a local maximum in the effective potential corresponds to an unstable null circular orbit, on the other hand, the presence of a saddle point leads to a more challenging classification problem which needs a careful scrutiny. By means of a Jacobi analysis we showed that the light-like circular geodesics associated to the aforementioned saddle point are unstable. Furthermore, we constructed the impact parameter for the light scattering in the dS -metric and showed that the Sachs scalars do not depend on the cosmological constant, hence they cannot be used to optically discriminate among - and - black holes with . This obliged us to probe into the weak and strong gravitational lensing for which we computed the corresponding deflection angle in terms of the distance of closest approach and the position of the observer. Our results reveal that corrections of the cosmological constant appear only in the case the observer is located close to the cosmological horizon.
References
- (1) J. Podolsk and J. B. Griffiths, Accelerating Kerr-Newman black holes in (anti-) de Sitter space-time, Phys. Rev D 73, 044018 (2006)
- (2) J. B. Griffiths and J. Podolsk, A new look at the Plebnski–Deminski family of solutions, Int. J. Mod. Phys. D 15, 335 (2005)
- (3) D. Kofron̆, On the nature of cosmic strings in black hole spacetimes, Gen. Relativ. Gravit. 52, 91 2020
- (4) A. Balaguera-Antolinez, C. G. Boehmer and M. Nowakowski, Scales of the cosmological constant, Class. Quant. Grav. 23, 485 (2006); P. Bargueño, S. Barvo Medina, M. Nowakowski and D. Batic, Quantum mechanical corrections to the Schwarzschild black hole metric, Europ. Phys. lett. 117, 6006 (2017)
- (5) C.-M. Claudel, K. S. Virbhadra and G. F. R. Ellis, The geometry of photon surfaces, J. Math. Phys 42, 818 (2001)
- (6) G. W. Gibbons and C. M. Warnick, Aspherical photon and anti-photon surfaces, Phys. Lett. B 763, 169 (2016)
- (7) H. Farhoosh and R.L. Zimmermann, Killing horizons and dragging of the inertial frame about a uniformly accelerating particle, Phys. Rev. D 21, 317 (1980)
- (8) V. Pravda and A. Pravdov, Co-accelerated particles in the C-metric, Class. Quantum Grav. 18, 1205 (2001)
- (9) Y.-K. Lim, Geodesic motion in the vacuum C metric, Phys. Rev. D 89, 104016 (2014)
- (10) D. Bini, C. Cherubini, A. Geralico and R. T. Jantzen, Circular motion in accelerating black hole space-times, Int. J. Mod. Phys. D 16, 1813 (2007)
- (11) D. Bini, C. Cherubini, A. Geralico and B. Mashhoon, Spinning particles in the vacuum C metric, Class. Quantum Grav. 22, 709 (2005)
- (12) M. Alrais Alawadi, D. Batic and M. Nowakowski, Light bending in a two black hole metric, Class. Quantum Grav. 38, 045003
- (13) A. Grenzebach, V. Perlick and C. Lämmerzhal, Photon regions and shadows of accelerated black holes, Int. J. of Mod. Phys. D 24, 1542024 (2015)
- (14) A. Grenzebach, The Shadow of Black Holes (Springer Briefs in Physics, Heidelberg, 2016)
- (15) T. C. Frost and V. Perlick, Lightlike Geodesics and Gravitational Lensing in the Spacetime of an Accelerating Black Hole, Class. Quantum Grav. 38, 085016 (2021)
- (16) M. Zhang and J. Jiang, Shadows of the accelerating black holes, Phys. Rev. D 103, 025005 (2021)
- (17) A. K. Mishra, S. Chakraborty and S. Sarkar, Understanding photon sphere and black hole shadow in dynamically evolving spacetimes, Phys. Rev. D 99, 104080 (2019)
- (18) K. Destounis, R. D. Fontana and F. C. Mena, Accelerating black holes: Quasinormal modes and late-time tails, Phys. Rev. D 102, 044005 (2020)
- (19) K. Destounis, R. D. Fontana and F. C. Mena, Stability of the cauchy horizon in accelerating black-hole spacetimes, Phys. Rev. D 102, 104037 (2020)
- (20) J. Podolsk and J. B. Griffiths, Uniformly accelerating black holes in a de Sitter universe, Phys. Rev.D 63, 024006 (2000)
- (21) J. Podolsk, Accelerating black holes in anti–de Sitter universe, Czech. J. Phys. 52, 1 (2002)
- (22) O. J. Dias and J. P. Lemos, Pair of accelerated black holes in anti–de Sitter background: AdS C metric, Phys. Rev. D 67, 064001 (2003)
- (23) O. J. Dias and J. P. Lemos, Pair of accelerated black holes in a de Sitter background: The dS C metric, Phys. Rev. D 67, 084018 (2003)
- (24) P. Krtous̆ and J. Podolsk, Radiation from accelerated black holes in de Sitter universe, Phys. Rev. D 68, 024005 (2003)
- (25) J. Podolsk, M. Ortaggio and P. Krtous̆, Radiation from accelerated black holes in an anti-de Sitter universe, Phys. Rev. D 68, 124004 (2003)
- (26) P. Krtous̆, Accelerated black holes in an anti-de Sitter universe, Phys. Rev. D 72, 124019 (2005)
- (27) H. Xu, Minimal surfaces in AdS C-metric, Phys. Lett. B 773, 639 (2017)
- (28) A. Chamblin, Capture of bulk geodesics by brane-world black holes, Class. Quantum Grav. 18, L17 (2001)
- (29) Y.-K. Lim, Null geodesics in the C metric with a cosmological constant, Phys. Rev. D 103, 024007 (2021)
- (30) K. S. Virbhadra and G. F. R. Ellis, Schwarzschild black hole lensing, Phys. Rev. D 62, 084003 (2000)
- (31) K. S. Virbhadra and G. F. R. Ellis, Gravitational lensing by naked singularities, Phys. Rev. D 65, 103004 (2002)
- (32) K. S. Virbhadra, D. Narasimha and S. M. Chitre, Role of the scalar field in gravitational lensing, Astron. Astrophys. 337, 1 (1998)
- (33) K. S. Virbhadra and C. R. Keeton, Time delay and magnification centroid due to gravitational lensing by black holes and naked singularities, Phys. Rev. D 77, 124014 (2008)
- (34) K. S. Virbhadra, Relativistic images of Schwarzschild black hole lensing, Phys. Rev. D 79, 083004 (2009)
- (35) V. Perlick, Gravitational Lensing from a Spacetime Perspective, Living Rev. Relativ. 7, 9 (2004)
- (36) G. W. Gibbons and M. C. Werner, Applications of the Gauss-Bonnet theorem to gravitational lensing, Class. Quant. Grav. 25, 235009 (2008)
- (37) M. Sharif and S. Iftikhar, Equatorial gravitational lensing by accelerating and rotating black hole with NUT parameter, Astrophys. Space Sci. 361, 36 (2016)
- (38) O. Shemmer et al., Near-infrared spectroscopy of high-redshift active galactic nuclei: I. A metallicity-accretion rate relationship, ApJ 614, 547–557
- (39) S. Gillessen al., Monitoring stellar orbits around the Massive Black Hole in the Galactic Center, ApJ 692, 1075
- (40) B. P. Abbott et al. (LIGO, Virgo and other collaborations), Multi-messenger Observations of a Binary Neutron Star Merger, ApJ 848, L12 (2017)
- (41) M. Carmeli and T. Kuzmenko, Value of the cosmological constant: Theory versus experiment, AIP Conference Proceedings 586, 316 (2001)
- (42) I. Arraut, D. Batic and M. Nowakowski, Comparing two approaches to Hawking radiation of Schwarzschild-de Sitter black holes, Class. Quant. Grav. 26, 125006 (2009)
- (43) I. N. Bronshtein, K. A. Semendyayev, G. Musiol and H. Muehlig, Handbook of Mathematics, (Springer Verlag Berlin Heidelberg, 2015)
- (44) S. W. Hawking, Gravitationally collapsed objects of very low mass, Mon. Not. R. Astron. Soc. 152, 75 (1971)
- (45) J.R. Gott III et al., A Map of the Universe, Ap.J. 624, 463 (2005)
- (46) T. Fließbach, Allgemeine Relativitätstheorie, (Elsevier Spektrum Akademischer Verlag, 2006)
- (47) E. Cartan and D. D. Kosambi Observations sur le mmoir prcdent, Math. Zeitschrift 37, 619 (1933)
- (48) S.S. Chern, Sur la geometrie d’un systme d’equations differentialles du second ordre, Bull. Sci. Mat. 63, 206 (1939)
- (49) S.S. Chern, Selected Papers, Vol. II (Springer Verlag, Berlin, Heidelberg, 1989)
- (50) D.D. Kosambi, Parallelism and path space, Math. Zeitschrift 37 (1933), 608-618
- (51) T. Harko, P. Pantaragphong, and S.V. Sabau, Kosambi–Cartan–Chern (KCC) theory for higher-order dynamical systems, Int. J. of Geometric Methods in Modern Physics 13, 1650014 (2016)
- (52) S. Bahamonde, C.G. Böhmer, S. Carloni, E.J. Copeland, W. Fang, N. Tamanini, Dynamical systems applied to cosmology: dark energy and modified gravity Phys. Rep. 775, 1 (2018)
- (53) C. G. Böhmer, T. Harko, and S. V. Sabau, Jacobi stability analysis of dynamical systems - applications in gravitation and cosmology, Adv. Theor. Math. Phys. 16, 1145 (2012)
- (54) C. G. Böhmer and N. Chen, Dynamical systems in cosmology, LTCC Advanced Mathematics Series: Volume 5, Dynamical and Complex Systems 121 (2017)
- (55) T. Harko, C. Yin Ho, C. Sing Leung and S. Yip, Jacobi stability analysis of the Lorenz system, Int. J. of Geometric Methods in Modern Physics 12, 1550081 (2015)
- (56) B. Dănilă, T. Harko, M. K. Mak, P. Pantaragphong and S.V. Sabau, Jacobi stability analysis of scalar field models with minimal coupling to gravity in a cosmological background, Adv. High Energy Phys. (2016)
- (57) M. J. Lake and T. Harko, Dynamical behavior and Jacobi stability analysis of wound strings, Eur. Phys. J. C 76, 1 (2016)
- (58) P.L. Antonelli, On y-Berwald connections and Hutchinson’s ecology of social-interactions, Tensor, N. S. 52, 27 (1993)
- (59) R. Miron, D. Hrimiuc, H. Shimada, and V.S. Sabau, The Geometry of Hamilton and Lagrange Spaces, (Kluwer Academic Publishing, Dordrecht, 2001)
- (60) P.L. Antonelli, Equivalence problem for systems of second order ordinary differential equations, Encyclopedia of Math. (Kluwer Academic Publishers, 2000)
- (61) P.L. Antonelli, R. Ingarden and M. Matsumoto, The Theory of Sprays and Finsler Spaces with Applications in Physics and Biology, Fundamental Theories of Physics 58 (Springer Netherlands, 1993)
- (62) H. Arakida, Effect of the Cosmological Constant on Light Deflection: Time Transfer Function Approach, Universe 2016, 2, 5
- (63) J. A. Murdock, Perturbations Theory and Methods, (John Wiley & Sons, New York, 1991)
- (64) P. Jordan, J. Ehlers, and R. K. Sachs, Beiträge zur Theorie der reinen Gravitationsstrahlung, Akad. Wiss. Lit. Mainz, Abh. Math. Nat. Kl., (Akad. Wiss. Lit., Mainz, 1961).
- (65) R. M. Wald, General Relativity, (University of Chicago Press, Chicago, 1984)
- (66) S. Seitz, P. Schneider, and J. Ehlers, Light propagation in arbitrary spacetimes and the gravitational lens approximation, Class. Quantum Grav. 11, 2345 (1994)
- (67) B. Carter, Gravitation in Astrophysics, NATO ASI Series B, vol. 156, Plenum Press, 1987
- (68) S. Chandrasekhar, The Mathematical Theory of Black Holes, (Oxford University Press, 1983)
- (69) Steven Weinberg, Gravitation and Cosmology, (John Wiley & Sons, New York, 1972)
- (70) D. Batic, S. Nelson, and M. Nowakowski, Light on curved backgrounds, Phys.Rev. D 91, 104015 (2015)
- (71) N. J. Islam, The cosmological constant and classical tests of general relativity, Phys. Lett. A 97, 239 (1983)
- (72) E. L. Kaplan, Auxiliary table for the incomplete elliptic integrals, J. Math. and Phys. 27, 11 (1948); W. J. nellis and B. C. Carlson, Reductiona and evaluation of elliptic integrals, Math. Comp. 20, 223 (1966); B. C. Carlson. Special Functions of Applied Mathematics, (Academic Press, New York, 1977)
- (73) C. Darwin, The Gravity Field of a Particle, I, Proc. R.Soc. London A 249, 180 (1959)
- (74) V. Bozza, S. Capoziello, G. Iovane and G. Scarpetta, Strong Field Limit of Black Hole Gravitational Lensing, Gen.Rel.and Grav. 33, 1535 (2001); V. Bozza, Gravitational lensing in the strong field limit, Phys. Rev. D 66, 103001 (2002)