Regge trajectory relations for the universal description of the heavy-light systems: diquarks, mesons, baryons and tetraquarks
Abstract
Two newly proposed Regge trajectory relations are employed to analyze the heavy-light systems. One of the relations is , . Another reads . is the bound state mass. and are the masses of the heavy constituent and the light constituent, respectively. is the orbital angular momentum and is the radial quantum number. and are fitted. , and are input parameters. These two formulas consider both of the masses of heavy constituent and light constituent. We find that the heavy-light diquarks, the heavy-light mesons, the heavy-light baryons and the heavy-light tetraquarks satisfy these two formulas. When applying the first formula, the heavy-light systems satisfy the universal description irrespective of both of the masses of the light constituents and the heavy constituent. When using the second relation, the heavy-light systems satisfy the universal description irrespective of the mass of the heavy constituent. The fitted slopes differ distinctively for the heavy-light mesons, baryons and tetraquarks, respectively. When employing the first relation, the average values of () are , and (, and ) for the heavy-light mesons, the heavy-light baryons and the heavy-light tetraquarks, respectively. Upon application of the second relation, the mean values of () are , and (, and ) for the heavy-light mesons, the heavy-light baryons and the heavy-light tetraquarks, respectively. Moreover, the fitted results show that the Regge trajectories for the heavy-light systems are concave downwards in the and planes.
I Introduction
There is a vast amount of experimental data on the spectra of different types of hadrons ParticleDataGroup:2022pth. One of the widely used approaches in studying the hadron spectra is the Regge trajectory Regge:1959mz; Collins:1971ff; Collins:1977jy; Gross:2022hyw; Inopin:2001ub; Inopin:1999nf; Chew:1961ev; Chew:1962eu; Guo:2008he; Feng:2022hpj; MartinContreras:2023oqs; Brisudova:1999ut; Sergeenko:1994ck; Veseli:1996gy; Afonin:2014nya; Burns:2010qq; MartinContreras:2020cyg; Baldicchi:1998gt; Tang:2000tb; Badalian:2019lyz; Chen:2018nnr. The study of Regge trajectories of hadron spectra is beneficial for gaining insights into the strong interactions from various perspectives Chen:2016spr; Klempt:2012fy; Brodsky:2018vyy; Nielsen:2018uyn; Chen:2017fcs; Sonnenschein:2018fph; MartinContreras:2020cyg; Sharov:2013tga; Forkel:2007cm; A:2023bxv. Clearly, an unified dynamic mechanism will lead to the universal description of Regge trajectories for hadrons Brodsky:2018vyy; Nielsen:2018uyn; Sonnenschein:2018fph.
The meson Regge trajectories exhibit structures that vary in different energy regions Chen:2021kfw; Chen:2022flh. This variability is expected to hold true for other hadrons with a similar dynamic mechanism, such as baryons and tetraquarks in the diquark picture. For the light-light systems, it is well-known that they can be well described by the renowned linear Regge trajectories Chew:1961ev; Chew:1962eu. In case of the heavy-heavy systems, an universal description is presented in Refs. Chen:2023djq; Feng:2023txx. Regarding the heavy-light systems, the authors provide an universal description of the orbitally excited heavy-light mesons and baryons in Ref. Chen:2017fcs. In Ref. Chen:2023cws, we present the heavy-light diquark Regge trajectories. The proposed Regge trajectory relations can universally describe the heavy-light mesons and the heavy-light diquarks. In this study, we apply the Regge trajectory relations 111The Regge trajectories of hadrons are commonly plotted in the plane or in the plane, where . For simplicity, the figures plotted in the plane and in the plane are also called the Regge trajectories. In this work, we concentrate on the -mode of baryons and tetraquarks and the -mode excitations of diquarks are not considered. newly proposed in Ref. Chen:2023cws to the heavy-light baryons composed of a heavy quark and a light diquark or composed of a light quark and a heavy diquark, as well as to the heavy-light tetraquarks consisting of one heavy (light) diquark and one light (heavy) antidiquark.
This paper is organized as follows: In Sec. II, the Regge trajectory relations are obtained from the spinless Salpeter equation (SSE). In Sec. III, the universal description of the heavy-light diquarks, mesons, baryons and tetraquarks is investigated. Discussions on concavity of the Regge trajectories are given in Sec. IV and the conclusions are in Sec. V.
II Regge trajectory relations for the heavy-light systems
II.1 SSE
The spinless Salpeter equation (SSE) Godfrey:1985xj; Ferretti:2019zyh; Bedolla:2019zwg; Durand:1981my; Durand:1983bg; Lichtenberg:1982jp; Jacobs:1986gv reads as
| (1) |
where . is the bound state mass (diquark, meson, baryon and tetraquark). are the diquark wave function, the meson wave function, the baryon wave function, and the tetraquark wave function, respectively. are the diquark potential, the meson potential, the baryon potential, and the tetraquark potential, respectively. is the relativistic energy of constituent , and is of constituent ,
| (2) |
and are the effective masses of heavy constituent and light constituent , respectively.
In the present work, the Cornell-like potential is considered Eichten:1974af; Ferretti:2019zyh; Bedolla:2019zwg; Ferretti:2011zz,
| (3) |
where is the color Coulomb potential or a Coulomb-like interaction for different hadrons Ferretti:2019zyh; Ferretti:2011zz. The second term is the linear confining potential and is the string tension. is a fundamental parameter Gromes:1981cb; Lucha:1991vn. is the color-Casmir,
| (4) |
From Eqs. (1) and (3), we see that the heavy-light diquarks, the heavy-light mesons, the heavy-light baryons, and the heavy-light tetraquarks are described in an unified approach Ferretti:2019zyh; Bedolla:2019zwg. Therefore, it is expected that these heavy-light systems can be described universally by the Regge trajectory approach.
II.2 Regge trajectory relations
The mass of the light constituent is assumed to approach zero, in Refs. Veseli:1996gy; Chen:2017fcs or is considered by correction term in Refs. Selem:2006nd; Chen:2014nyo; Afonin:2014nya; Sonnenschein:2018fph; Jakhad:2023mni. In the limit and , Eq. (1) is reduced to be
| (5) |
By employing the Bohr-Sommerfeld quantization approach brau:04bs; brsom, we have from Eq. (5)
| (6) |
where
| (7) |
Using Eq. (6), the parameterized formula can be written as Chen:2023cws
| (8) |
The parameter in Eq. (8) reads as Chen:2021kfw
| (9) |
The constants [ and ] and are
| (10) |
Both and are theoretically equal to one and are fitted in practice. For the heavy-light mesons, the common choice of is Selem:2006nd; Chen:2021kfw; Jakhad:2023mni; Chen:2014nyo; Chen:2017fcs; Veseli:1996gy; Jia:2018vwl
| (11) |
, and are universal for the heavy-light systems. and , which vary with different Regge trajectories, are determined by fitting the given Regge trajectory.
The usual Regge trajectory Eq. (8) with (11), which is obtained in the limit and , cannot give agreeable results of the heavy-light diquarks. Corresponding to different ways to include the light constituent’s mass, two modified formulas are proposed in Ref. Chen:2023cws, which can describe universally both the heavy-light mesons and the heavy-light diquarks. One is Eq. (8) with
| (12) |
where
| (13) |
Another reads
| (14) |
if , where
| (15) |
where is in (9). (8) with (12) is an extension of Afonin:2014nya, while (14) with (15) is based on the results in Selem:2006nd; Sonnenschein:2018fph. As , these two modified formulas, formulas (8) with (12) and (14) with (15), become identical. As and is neglected, these two modified formulas reduce to the usual Regge trajectory formula for the heavy-light mesons, i.e., (8) with (11). In this work, we apply these two modified formulas to the heavy-light systems: diquarks, mesons, baryons, tetraquarks.
III Universal description of the heavy-light systems
In this section we present the universal description of different types of heavy-light systems by employing (8) with (12) and (14) with (15). The Regge trajectories for the heavy-light systems are fitted individually.
III.1 Parameters
The parameters are Ebert:2007rn; Ebert:2011kk; Ebert:2009ua
| (16) |
where denotes the axial-vector diquark and the scalar diquark. and vary with different Regge trajectories.
The values in Eq. (III.1) are used to calculate the masses of tetraquarks Ebert:2007rn, baryons Ebert:2011kk and mesons Ebert:2009ua. In Ref. Chen:2017fcs, the values are used to give an universal description of the heavy-light mesons and baryons. The values are also used to discuss diquarks Feng:2023txx; Chen:2023cws.
III.2 Heavy-light diquarks
Diquarks have been discussed by various approaches, for example, the QCD sum rules Dosch:1988hu; Zhang:2006xp; Jamin:1989hh; deOliveira:2023hma; Kleiv:2013dta; Wang:2011ab; Esau:2019hqw; Wang:2010sh. In Ref. Chen:2023cws, we show that the Regge trajectories for the heavy-light diquarks can be well described by (8) with (12) and (14) with (15). The spectra of the heavy-light diquarks , , and obtained by using the Regge trajectory approach agree with other theoretical predictions. See Chen:2023cws for more details.
In case of Eq. (8) with (12), the heavy-light diquarks satisfy the universal description irrespective of both mass of the light constituents and mass of the heavy constituent. In case of Eq. (14) with (15), the heavy-light diquarks satisfy the universal description irrespective of mass of the heavy constituents.
III.3 Heavy-light mesons
| State | Meson | PDG | EFG | Meson | PDG | EFG |
|---|---|---|---|---|---|---|
| 2.010 | 2.1122 | 2.111 | ||||
| 2.627 | 2.632 | 2.714 | 2.731 | |||
| 3.096 | 3.242 | |||||
| 3.482 | 3.669 | |||||
| 3.822 | 4.048 | |||||
| 2.4611 | 2.460 | 2.5691 | 2.571 | |||
| 2.7631 | 2.863 | 2.860 | 2.971 | |||
| 3.187 | 3.300 | |||||
| 3.473 | 3.595 |
| State | Meson | PDG | EFG | Meson | PDG | EFG |
|---|---|---|---|---|---|---|
| 5.326 | 5.4154 | 5.414 | ||||
| 5.906 | 5.992 | |||||
| 6.387 | 6.475 | |||||
| 6.786 | 6.879 | |||||
| 7.133 | 7.235 | |||||
| 5.7372 | 5.741 | 5.83986 | 5.842 | |||
| 6.091 | 6.191 | |||||
| 6.380 | 6.475 | |||||
| 6.634 | 6.726 |
| Radial Traj. | Orbital Traj. | |||
|---|---|---|---|---|
| Fit1 | Fit2 | Fit1 | Fit2 | |
| (1.038, 0.008) | (1.103, 0.17) | (1.093, 0.039) | (1.166, 0.23) | |
| (1.053, 0.0) | (1.152, 0.105) | (1.065, 0.0) | (1.168, 0.165) | |
| (1.025, 0.0) | (1.092, 0.15) | (0.991, 0.091) | (1.067, 0.26) | |
| (0.986, 0.0) | (1.085, 0.1) | (0.955, 0.005) | (1.054, 0.175) | |
| (0.827, 0.0) | (0.928, 0.15) | (0.773, 0.076) | (0.875, 0.275) | |
| (0.812, 0.0) | (0.912, 0.155) | (0.762, 0.093) | (0.864, 0.285) | |
| (0.789, 0.0) | (0.888, 0.21) | (0.693, 0.235) | (0.792, 0.44) | |
| (0.757, 0.0) | (0.854, 0.205) | (0.643, 0.232) | (0.732, 0.475) | |
| (0.763, 0.0) | (0.863, 0.205) | (0.692, 0.161) | (0.788, 0.415) | |
| (0.730, 0.0) | (0.827, 0.210) | (0.615, 0.280) | (0.710, 0.495) | |
| (0.756, 0.0) | (0.856, 0.205) | (0.692, 0.135) | (0.786, 0.425) | |
| (0.718, 0.0) | (0.814, 0.225) | (0.624, 0.149) | (0.705, 0.50) | |
| (0.902, 0.379) | (1.020, 0.410) | |||
| (0.903, 0.200) | (1.015, 0.22) | (0.869, 0.338) | (0.984, 0.37) | |
| (0.890, 0.349) | (1.032, 0.37) | |||
| (0.872, 0.196) | (1.004, 0.215) | (0.836, 0.336) | (0.971, 0.36) | |
| (0.611, 0.251) | (0.718, 0.285) | (0.618, 0.384) | (0.726, 0.44) | |
| (0.517, 0.300) | (0.606, 0.355) | (0.598, 0.352) | (0.701, 0.415) | |
| (0.579, 0.188) | (0.677, 0.27) | (0.548, 0.329) | (0.637, 0.48) | |
| (0.484, 0.214) | (0.564, 0.335) | (0.521, 0.290) | (0.605, 0.46) | |
| (0.563, 0.268) | (0.660, 0.315) | (0.618, 0.349) | (0.724, 0.41) | |
| (0.528, 0.196) | (0.615, 0.300) | (0.553, 0.281) | (0.643, 0.430) | |
| (0.614, 0.233) | (0.718, 0.300) | (0.594, 0.393) | (0.694, 0.505) | |
| (0.521, 0.289) | (0.608, 0.385) | (0.564, 0.388) | (0.658, 0.515) | |
| (0.564, 0.260) | (0.659, 0.34) | (0.599, 0.362) | (0.699, 0.475) | |




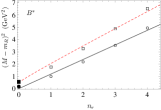






By using Eqs. (8) with (12) and (14) with (15) and data in Table 1, the radial and orbital Regge trajectories for the heavy-light mesons , , and are obtained, see Fig. 1. The fitted values are listed in Table 2. In Fig. 1, the Regge trajectories (the red dashed lines) obtained by employing (14) with (15) lie above the Regge trajectories (the black lines) obtained by applying (8) with (12). It is because for these two Regge trajectories are different, see Eqs. (12) and (15). The figures show that the heavy-light mesons satisfy both of these two Regge trajectory relations.
From Fig. 2 and the fitted values in Table 2, we can see that both of the radial and orbital Regge trajectories in the planes almost overlap with each other irrespective of both the light quark flavor and heavy quark flavor. It shows an universal description of these heavy-light mesons. For the heavy-light mesons, the Regge trajectories in the plane are universal only irrespective of heavy quark flavor.
As employing Eq. (8) with (12), the average values of and are and for the heavy-light mesons, respectively. When applying Eq. (14) with (15), the average values of and are and , respectively.
From Table 2, we can see that the fitted values of and decrease in the majority of cases as the masses of the constituents increase when employing (8) with (12). However, the fitted values of and obtained by using (14) with (15) frequently decrease with the increase of the masses of the heavy constituent and rise with the increase of the masses of the light constituent. This trend aligns with the results in Chen:2017fcs.
III.4 Heavy-light baryons
| Q[ud] State | Baryon | PDG ParticleDataGroup:2022pth | EFG Ebert:2011kk | Baryon | PDG ParticleDataGroup:2022pth | EFG Ebert:2011kk |
|---|---|---|---|---|---|---|
| 2.28646 | 2.286 | 5.61960 | 5.620 | |||
| 2.769 | 6.089 | |||||
| 3.130 | 6.455 | |||||
| 3.437 | 6.756 | |||||
| 3.715 | 7.015 | |||||
| 3.973 | 7.256 | |||||
| 2.62811 | 2.627 | 5.942 | ||||
| 2.88163 | 2.880 | 6.196 | ||||
| 3.078 | 6.411 | |||||
| 3.284 | 6.599 | |||||
| 3.460 | 6.766 |
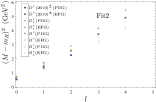
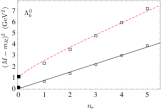
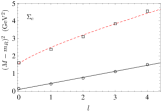
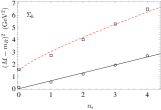
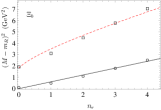
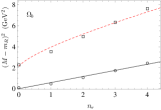
In the diquark picture, the baryons consisting of one light quark and one heavy diquark or consisting of one heavy quark and one light diquark are denoted as the heavy-light baryons and belong to the heavy-light systems. Employing (8) with (12) and (14) with (15) to the heavy-light baryons, the Regge trajectories are fitted. The used data are listed in Table 3 and the fitted values are in Table 2. The fitted Regge trajectories are in Fig. 3.
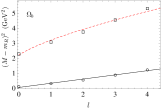
The difference of for two Regge trajectory relation leads to that the Regge trajectories (the red dashed lines) obtained by employing (14) with (15) lie above the Regge trajectories (the black lines) obtained by applying (8) with (12), see Fig. 3. From Fig. 4 and the fitted values in Table 2, we can see that the heavy-light baryons satisfy both of these two Regge trajectory relations. We also notice that some fitted Regge trajectories by employing (8) with (12) are better than the fitted Regge trajectories by applying (8) with (12), for example, the Regge trajectory for , see 3.
Similar to the meson case, from Fig. 4 and the fitted values in Table 2, we can see that both of the radial and orbital Regge trajectories for the heavy-light baryons plotted in the planes almost overlap with each other irrespective of both the light constituent and the heavy constituent. This shows an universal description of these heavy-light baryons. For the heavy-light baryons, the Regge trajectories in the plane are universal only irrespective of heavy constituent.
As employing Eq. (8) with (12), the average values of and are and for the heavy-light baryons, respectively. When applying Eq. (14) with (15), the average values of and are and , respectively. The fitted and for the heavy-light baryons are smaller than that for the heavy-light mesons. The fitted values for the doubly heavy baryons approximate the fitted values for the heavy-light mesons because the doubly heavy baryons strongly resemble the heavy-light mesons.
| 2.519 | 5.834 | 2.649 | 5.963 | 2.768 | 6.088 | 3.727 | 10.237 | 3.872 | 10.389 | |
| 2.936 | 6.226 | 3.026 | 6.342 | 3.123 | 6.461 | 10.860 | 10.992 | |||
| 3.293 | 6.583 | 3.396 | 6.695 | 3.510 | 6.811 | |||||
| 3.598 | 6.876 | 3.709 | 6.984 | 3.830 | 7.096 | |||||
| 3.873 | 7.129 | 3.989 | 7.234 | 4.114 | 7.343 | |||||
| 2.789 | 6.084 | 2.929 | 6.226 | 3.051 | 6.334 | 4.155 | 10.661 | 4.303 | 10.798 | |
| 3.013 | 6.260 | 3.147 | 6.414 | 3.283 | 6.517 | |||||
| 3.209 | 6.459 | 3.357 | 6.610 | 3.485 | 6.713 | |||||
| 3.386 | 6.635 | 3.536 | 6.728 | 3.665 | 6.884 |















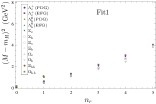
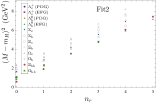
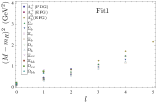
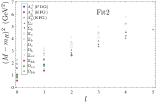
III.5 Heavy-light tetraquarks
The heavy-light tetraquarks denote tetraquarks consisting of one heavy diquark and one light antidiquark or consisting of one light diquark and one heavy antidiquark. The tetraquarks composed of a diquark and antidiquark in color and configurations are considered.
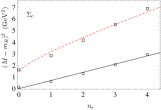
Applying Eqs. (8) with (12) and (14) with (15) and using data in Table 5, the radial and orbital Regge trajectories for the heavy-light tetraquarks are obtained, see Fig. 5. The fitted values are listed in Table 2. Similar to the heavy-light mesons and the heavy-light baryons, the Regge trajectories (the red dashed lines) obtained by employing (14) with (15) lie above the Regge trajectories (the black lines) obtained by applying (8) with (12), see Fig. 5.
From Fig. 6 and the fitted values in Table 2, we can see that the heavy-light tetraquarks satisfy both of these two Regge trajectory relations. Moreover, we can see that the universal description of the heavy-light tetraquarks approximately holds. We notice that the fitted results are not reliable enough because the experimental data is lack and the theoretical data on the -excited states are scarce. [The theoretical data on the -excited states of the tetraquarks are scarce because the masses of these states lie above the corresponding thresholds.]
As employing Eq. (8) with (12), the average values of and are and for the heavy-light tetraquarks, respectively. When applying Eq. (14) with (15), the mean values of and are and , respectively. The fitted and for the heavy-light tetraquarks are smaller than that for the heavy-light mesons and the heavy-light baryons.
| 3.961 | 10.489 | 4.141 | 10.664 | 4.151 | 10.685 | 7.246 | 7.423 | 7.441 | |
| 4.363 | 10.815 | 4.545 | 10.993 | 4.560 | 11.016 | 7.610 | 7.788 | 7.808 | |
| 4.253 | 10.778 | 4.410 | 10.928 | 4.430 | 10.951 | 7.545 | 7.705 | 7.728 |






















IV Concavity of the Regge trajectories
The Regge trajectories take different forms in different energy regions Chen:2021kfw; Chen:2022flh. In this section, the Regge trajectories are depicted in the planes. In Refs. Feng:2023txx; Chen:2023cws; Chen:2023ngj, it is shown that the Regge trajectories for all types of diquarks (including the doubly heavy diquarks, the heavy-light diquarks and the light diquarks) are concave downwards.
In Ref. Chen:2018bbr, it is shown that the meson Regge trajectories are concave downwards for the doubly heavy mesons and the heavy-light mesons. In the case of the light mesons, the Regge trajectories assume a linear form when the masses of the light quark and antiquark are taken as zero. However, upon taking into account the masses of the light quark and antiquark, the Regge trajectories for the light mesons also adopt a concave shape, see Refs. Selem:2006nd; Sonnenschein:2018fph.
In Ref. Chen:2023djq, we show that the Regge trajectories for the heavy-heavy baryons and tetraquarks are also concave downwards. In this work, we observe that the Regge trajectories for the heavy-light baryons and the heavy-light tetraquark also display a concave shape. Taking into account the masses of the light constituent, it is expected that the Regge trajectories for the light baryons and the light tetraquarks will similarly be concave.
The curvature of the Regge trajectories holds significant importance Chen:2018bbr. In potential models, the curvature is related to the dynamic equation and the confining potential. Based on the discussions in this section, we assume that, when considering the mass of the light constituent, all Regge trajectories for the diquarks, mesons, baryons and tetraquarks are concave downwards in the and planes. Future experimental data will either validate or challenge the concavity conjecture. It will improve understanding of hadron dynamics and promote the study of hadron spectra.
V Conclusions
We apply two newly proposed Regge trajectory relations (8) along with (12) and (14) along with (15) to analyze the heavy-light systems, presenting the numerical plots for clarity. We find that the heavy-light diquarks, the heavy-light mesons, the heavy-light baryons and the heavy-light tetraquarks satisfy these two formulas.
In the scenario where Eq. (8) is combined with (12), the heavy-light systems conform to the universal description regardless of the masses of the light constituents and the heavy constituent. When considering Eq. (14) alongside (15), the heavy-light systems adhere to the universal description regardless of the masses of the heavy constituents. It is observed that the fitted slopes for the radial Regge trajectories are greater than those for the orbital Regge trajectories across all heavy-light systems.
The fitted slopes exhibit distinctive differences among the heavy-light mesons, baryons and tetraquarks, respectively. When employing Eq. (8) with (12), the average values of () are , and (, and ) for the heavy-light mesons, the heavy-light baryons and the heavy-light tetraquarks, respectively. When applying Eq. (14) with (15), the average values of () are , and (, and ) for the heavy-light mesons, baryons and tetraquarks, respectively.
Furthermore, the fitted results indicate that the Regge trajectories for the heavy-light systems exhibit concave downward shapes in the and planes. It is expected that, when considering the mass of the light constituent, all Regge trajectories for the diquarks, mesons, baryons and tetraquarks are concave downwards in the and planes. Future experimental data will either validate or refute the concavity conjecture. It will improve understanding of hadron dynamics and promote the study of hadron spectra.
Acknowledgements We are very grateful to the anonymous referees for the valuable comments and suggestions.