Reduction of one-dimensional non-Hermitian point-gap topology by correlations
Abstract
In spite of extensive works on the non-Hermitian topology, correlations effects remain crucial questions. We hereby analyze correlated non-Hermitian systems with special emphasis on the one-dimensional point-gap topology. Specifically, our analysis elucidates that correlations result in reduction of the topological classification for systems of one synthetic dimension with charge symmetry and spin-parity symmetry. Furthermore, we analyze an extended Hatano-Nelson chain which exhibits striking correlation effects; correlations destroy the skin effect at the non-interacting level. This fragility of the skin effect against interactions is consistent with the reduction of the point-gap topology in the one spatial dimension. The above discoveries shed new light on the topology of correlated systems and open up new directions of researches on non-Hermitian topological physics.
Introduction–. Topological insulators and superconductors have been extensively analyzed in these 15 years Thouless et al. (1982); Hatsugai (1993); Kitaev (2001); Kane and Mele (2005a, b); Qi et al. (2008); Hasan and Kane (2010); Qi and Zhang (2011); Sato and Fujimoto (2016). In particular, considerable efforts have been devoted to understanding correlation effects on the non-trivial topology, which has revealed a variety of unique phenomena. For instance, correlation effects induce topological ordered phases Tsui et al. (1982); Laughlin (1983); Jain (1989); Wen (2004); Levin and Wen (2005); Kitaev (2003, 2006) which host anyons. In addition, it has turned out that correlation effects change -classification of topological superconductors at the mean-field level to -classification Fidkowski and Kitaev (2010). Such a reduction phenomenon of possible topological phases for a given symmetry class has been theoretically reported for arbitrary spatial dimensions Turner et al. (2011); Fidkowski and Kitaev (2011); Yao and Ryu (2013); Ryu and Zhang (2012); Qi (2013); Lu and Vishwanath (2013); Levin (2013); Isobe and Fu (2015); Yoshida et al. (2017); Fidkowski et al. (2013); Wang et al. (2014); Metlitski et al. (2014); Wang and Senthil (2014); You and Xu (2014); Morimoto et al. (2015). Furthermore, a theoretical work Jian and Xu (2018) has elucidated that the reduction can also occur in synthetic dimensions which are considered to be fabricated in cold atoms Boada et al. (2012); Celi et al. (2014); Nakajima et al. (2016); Lohse et al. (2016). These developments reveal the ubiquity of the reduction phenomena.
Along with the above significant progress, understanding of the non-Hermitian band topology has been rapidly developed in these years Hatano and Nelson (1996); Bender and Boettcher (1998); Hu and Hughes (2011); Esaki et al. (2011); Bergholtz et al. (2021); Ashida et al. (2020). Remarkably, it has been elucidated that the point-gap topology induces novel phenomena which do not have Hermitian counterparts Martinez Alvarez et al. (2018); Kunst et al. (2018); Yao and Wang (2018); Yao et al. (2018); Yokomizo and Murakami (2019); Edvardsson et al. (2019); Gong et al. (2018); Carlström and Bergholtz (2018); Carlström et al. (2019); Zhou and Lee (2019); Kawabata et al. (2019a). A prime example is the emergence of the exceptional points Katō (1966); Rotter (2009); Berry (2004); Heiss (2012); Shen et al. (2018) (and their symmetry-protected variants Budich et al. (2019); Yoshida et al. (2019a); Okugawa and Yokoyama (2019); Zhou et al. (2019); Kawabata et al. (2019b); Kimura et al. (2019); Yoshida et al. (2020a); Delplace et al. (2021); Mandal and Bergholtz (2021)) on which the point-gap topology induces band touching for both the real and the imaginary parts. Another remarkable phenomenon is a non-Hermitian skin effect which results in extreme sensitivity to the presence/absence of boundaries Yao and Wang (2018); Lee and Thomale (2019); Borgnia et al. (2020); Zhang et al. (2020a); Okuma et al. (2020); Yoshida et al. (2020b); Okugawa et al. (2020); Kawabata et al. (2020). So far, the non-Hermitian topological band theory has been applied to a wide range of systems from quantum Jin and Song (2009a); Lee (2016); San-Jose et al. (2016); Xu et al. (2017); Jin and Song (2009b); Kozii and Fu (2017); Zyuzin and Zyuzin (2018); Yoshida et al. (2018); Shen and Fu (2018); Papaj et al. (2019); Matsushita et al. (2019); Michishita and Peters (2020) to classical systems Guo et al. (2009); Rüter et al. (2010); Regensburger et al. (2012); Zhen et al. (2015); Hassan et al. (2017); Zhou et al. (2018); Takata and Notomi (2018); Ozawa et al. (2019); Xiao et al. (2020); Weidemann et al. (2020); Hofmann et al. (2020); Helbig et al. (2020); Yoshida and Hatsugai (2019); Ghatak et al. (2020); Scheibner et al. (2020).
While most of the studies have focused on the non-interacting cases so far, correlation effects on the non-Hermitian topology attract growing interests Yoshida et al. (2019b); Xi et al. (2021); Yoshida et al. (2020c); Guo et al. (2020a); Matsumoto et al. (2020); Zhang et al. (2020b); Guo et al. (2020b); Shackleton and Scheurer (2020); Yang et al. (2021); Zhang et al. (2020c); Liu et al. (2020); Xu and Chen (2020); Pan et al. (2020); Mu et al. (2020); Lee (2021); Zhang et al. (2022); Kawabata et al. (2022); Tsubota et al. (2021); Qin et al. (2022); Orito and Imura (2022) due to the potential presence of novel non-Hermitian phenomena. Such interest of correlation effects on the non-Hermitian topology is further enhanced by recent development of technology in cold atoms which allows us to experimentally tune both dissipation and two-body interactions Tomita et al. (2017); Takasu et al. (2020). Despite these efforts, current understanding of the point-gap topology in correlated systems is quite limited. In particular, the knowledge about the reduction of the point-gap topology is limited only to zero dimension Yoshida and Hatsugai (2021), which poses the following significant question: fate of the higher dimensional point-gap topology under correlations.
We hereby tackle this question with particular focus on the one-dimensional point-gap topology in both cases of synthetic and spatial dimensions. We start with the topology in one synthetic dimension. Our analysis reveals the reduction of for systems with charge symmetry and spin-parity symmetry. We end up this conclusion by analyzing a toy model, as well as by an argument in terms of topological invariants. Furthermore, we analyze an extended Hatano-Nelson chain where such reduction results in a striking phenomenon: fragility of a skin effect against correlations in one spatial dimension.
Topological invariants in one synthetic dimension–. Firstly, we provide a generic argument in terms of topological invariants. Consider a quantum dot whose many-body Hamiltonian reads
| (1) |
with and The second term denotes two-body interactions of fermions. Here, one-body Hamiltonian is non-Hermitian and satisfies . The synthetic dimension is parameterized by which corresponds to a tunable parameter in experiments (e.g., a hopping integral in cold atoms). The operator () creates (annihilates) a fermion in orbital () and spin state (). The subscript labels the set of and .
Throughout this paper, we suppose that the Hamiltonian (1) respects the charge symmetry and spin-parity symmetry. Namely, the zero-dimensional Hamiltonian satisfies
| (2) |
with and .
Here, let us discuss the point-gap topology of the above system. In terms of the one-body Hamiltonian, we can introduce two distinct -invariants. Because the one-body Hamiltonian is periodic in , we can introduce the winding number
| (3) |
with the reference energy . The derivative with respect to is denoted by . The symbol “” denotes the trace of a matrix (i.e., ).
In addition, we can introduce spin winding number
| (4) |
with . Here, takes () for (), and takes () for . For the spin winding number the spin-parity symmetry is essential; the one-body Hamiltonian satisfies in the presence of the spin-parity symmetry.
The above results indicates that in the presence of the symmetry and the spin-parity symmetry, the point-gap topology of is characterized by two distinct -invariants.
Now, let us discuss the point-gap topology of the many-body Hamiltonian. In the presence of the spin-parity symmetry, the Hamiltonian can be block-diagonalized with and . Here, denotes the operator of total number of fermions in the up-spin state. Therefore, for each Fock space, the following many-body winding number can be introduced sec ;
| (5) |
where and are eigenvalues of and , respectively. The reference energy is denoted by . By , we denote the many-body Hamiltonian for the subsector with . The symbol “” denotes the trace over the subsector of the Fock space.
In the absence of interactions, eigenvalues of the many-body Hamiltonian for each Fock space is computed from the eigenvalues of the one-body Hamiltonian whose point-gap topology is characterized by and .
The above results indicate that the point-gap topology of the one-body Hamiltonian is characterized by a set of two -invariants while the topology of the many-body Hamiltonian is characterized by the -invariant for each sector of the Fock space. This fact implies that the non-trivial topology characterized by is trivialized by introducing the interactions, which we see below.
Two orbital quantum dot: non-interacting case–. As a specific case of Eq. (1), let us consider a two-orbital quantum dot () with a diagonal matrix [] whose diagonal elements are written as
| (6) |
Here, and ( and ) are real numbers. At the non-interacting level, couplings between orbitals are absent. The one-body Hamiltonian of orbital corresponds to the small cycle limit of an extended Hatano-Nelson chain under the twisted boundary condition [see Eq. (13)].
The topology of is characterized as for and ().
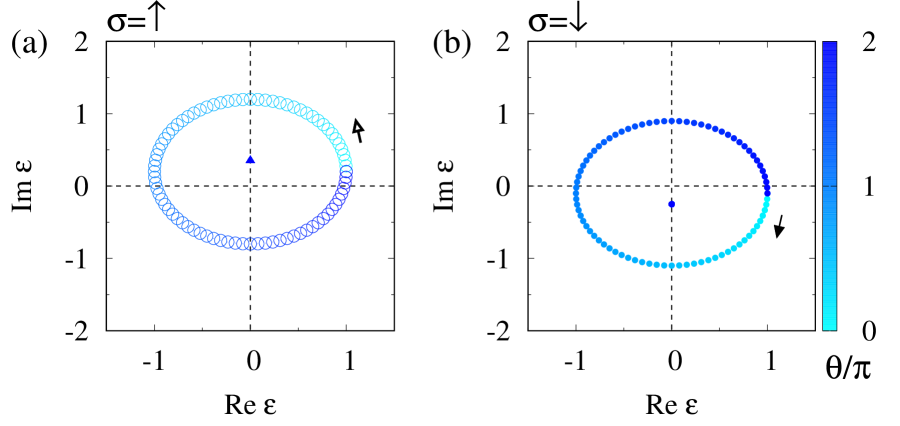
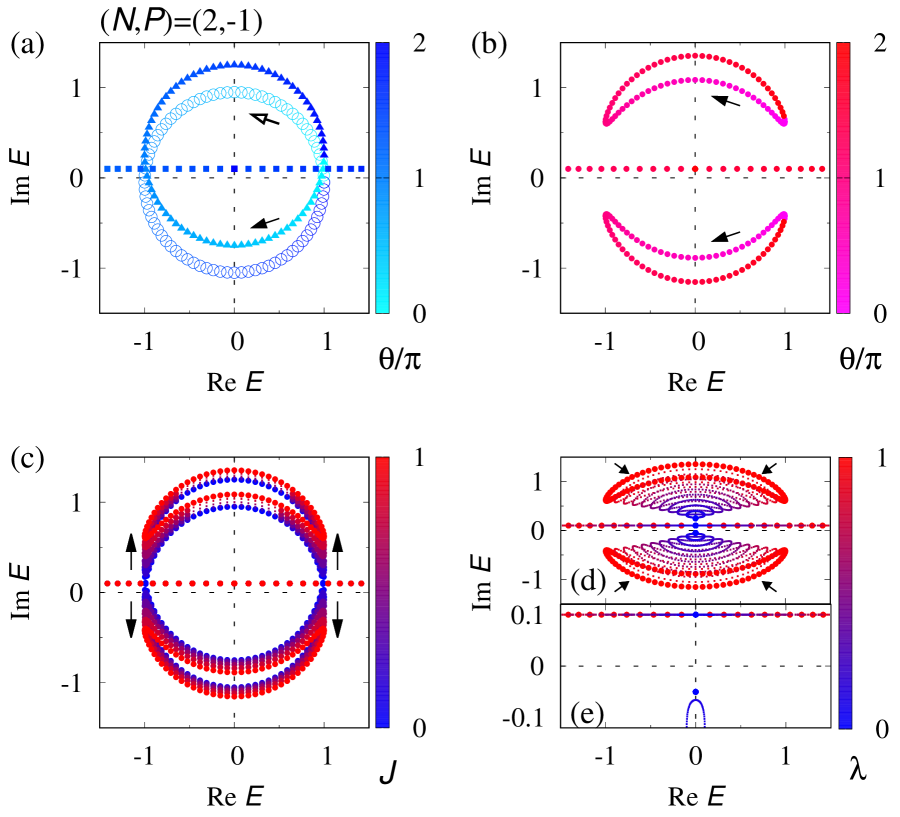
To be concrete, we plot a spectral flow of the one-body Hamiltonian in Fig. 1 for . This figure indicate that increasing from to , an eigenvalue winds around the origin in the clockwise (counter-clockwise) direction for the subsector (). The above numerical data support that the topology of is characterized as .
Figure 2(a) displays a spectral flow of the many-body Hamiltonian for the subsector with of the Fock space. We can observe the loop structure of the spectral flow due to the topology of the one-body Hamiltonian . However, this figure indicates that is topologically trivial [i.e., ] for because an eigenvalue winds around the origin in the clockwise direction, and the other eigenvalue winds around the origin in the opposite direction.
Two orbital quantum dot: interacting case–. Now, let us introduce the following two-body interaction
| (7) |
with real numbers and . Here, “” denotes the Hermitian conjugate of the corresponding operator [e.g., ]. The spin operator is defined as with being the () component of the spin operator for orbital . The above two-body interactions respect charge symmetry and spin-parity symmetry; applying the operator transforms the spin operators as , meaning that the interactions respect spin-parity symmetry.
For the sake of simplicity, we focus on the subsector with . The results for the subsector are provided in Sec. S1 of Supplemental Material sup . Figure 2(b) displays the spectral flow for . Remarkably, this figure indicate that the interactions open a imaginary gap; interactions split the loops which wind the origin at the non-interacting level [see Fig. 2(a)].
This fact indicates that interactions [Eq. (7)] allow a smooth deformation of the spectral flow for to that for without closing the point-gap at the latter of which is obviously trivial.
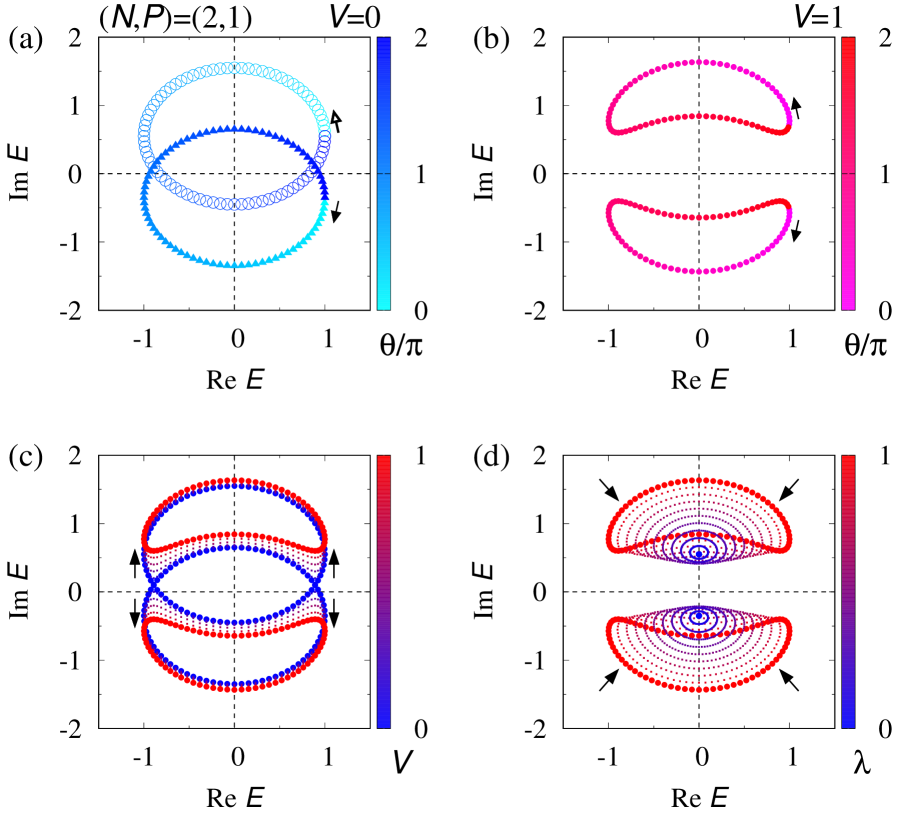
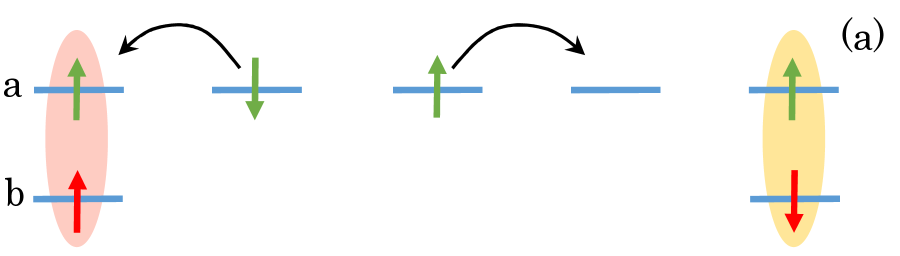
Indeed, the following deformation smoothly connects the Hamiltonian for and that for : (i) Increasing from to for and [see Fig. 2(c)]; (ii) Decreasing from to for [see Fig. 2(d)]. This deformation demonstrates that the many-body Hamiltonian is topologically trivial.
We note that difference of the symmetry constraint of the spin-parity symmetry is essential for the imaginary gap at in Fig. 2(b). As discussed above, the symmetry constraints (2), which results in , forbids hybridization terms between two distinct subsectors with . In contrast, the symmetry constraint allows such hybridization terms of two-body interactions . Therefore, the two-body interactions can destroy the loop structure arising from the non-trivial topology of the one-body Hamiltonian [see Figs. 2(a) and 2(b)].
For instance, in the subsector with , the Hamiltonian is written as
| (10) |
Here, we have chosen the following basis vectors spanning the subsector of the Fock space . The vacuum state is denoted by (i.e., for arbitrary and ).
Diagonalizing the above Hamiltonian, we obtain
| (11) |
with and . Equation (11) elucidates that spin-parity symmetry allows the hybridization term between states with and which opens the line-gap [see Fig. 2(b)]. In contrast, spin-parity symmetry forbids such hybridization terms for the quadratic Hamiltonian .
The above numerical results supports that the many-body Hamiltonian is topologically trivial despite the loop structure due to the topology of the one-body Hamiltonian with .
Putting the argument in terms of the topological invariants and the above results of the toy model together, we end up with the reduction of the point-gap topology .
Topology in one spatial dimension and fragility of a skin effect–. By analyzing an extended Hatano-Nelson chain [see Fig. 3(a)], we elucidate that correlations reduce the point-gap topology in one spatial dimension as is the case in one synthetic dimension. Remarkably, this reduction phenomenon results in fragility of a skin effect against interactions.
Let us consider an extended Hatano-Nelson chain [see Fig. 3(a)] whose Hamiltonian reads
| (12a) | |||||
| (12b) | |||||
with a diagonal matrix [, ] whose diagonal elements are
| (13) |
Here, we have imposed the twisted boundary condition in order to compute the winding numbers (for more details, see Sec. S2.1 of Supplemental Material sup ). The operator is the Fourier transformed annihilation operator with . The two-body term describes the interaction between fermions in orbital and localized fermions in orbital . This model also preserves charge and spin-parity symmetry, meaning that can be block-diagonalized with and where and are defined as and , respectively. The Hamiltonian also commutes with for , and thus, we suppose that orbital is occupied at both edges ().
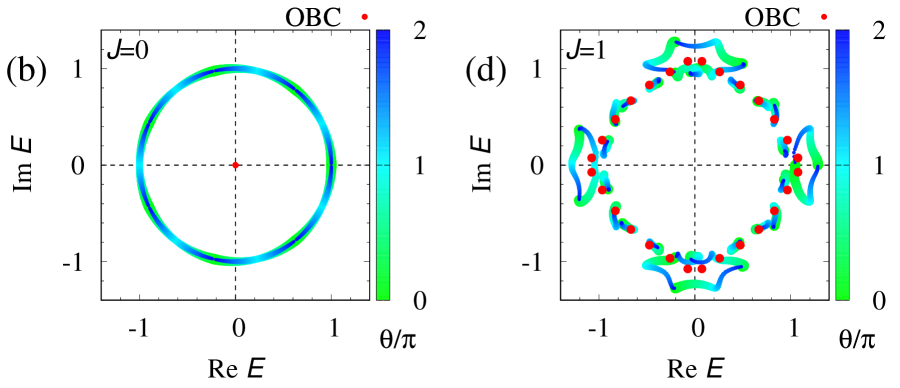
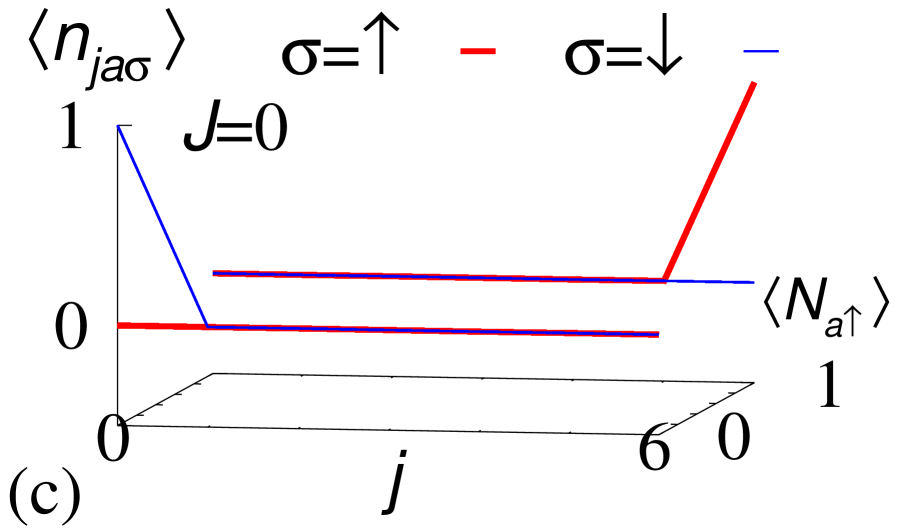
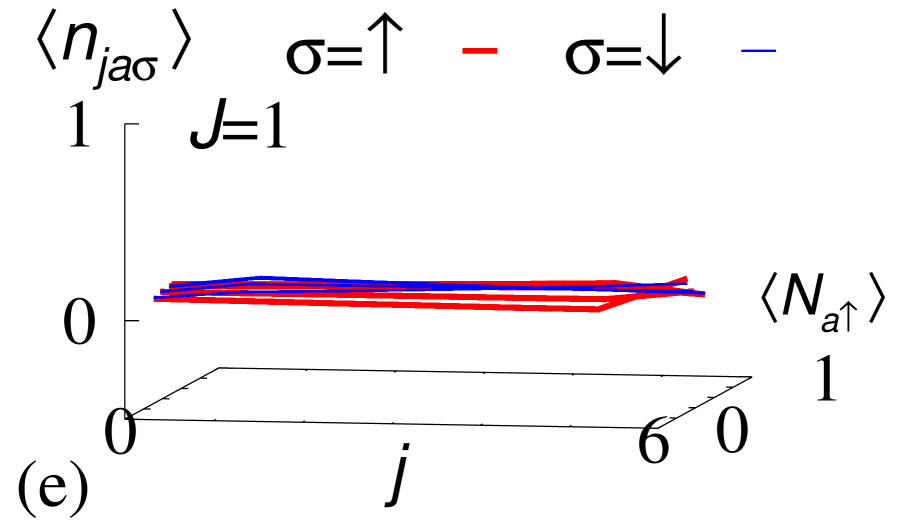
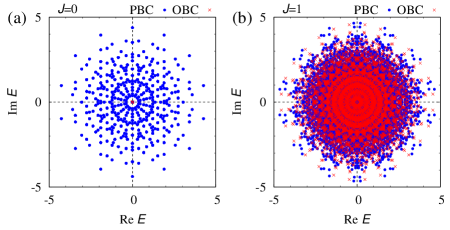
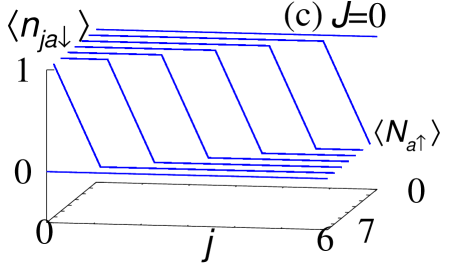
Now, we demonstrate that for (i.e., ), a skin effect observed at the non-interacting level is fragile against the two-body interactions due to trivial topology of the many-body Hamiltonian. Let us start with the non-interacting level. Under the twisted boundary condition, the spectral flow shows a loop structure [see Fig. 3(b)] due to the point-gap topology of one-body Hamiltonian characterized by for . This non-trivial topology of induces the skin effect at the non-interacting level. In the presence of the boundaries, all of the eigenvalues [ ()] become zero in contrast to the eigenvalues in the absence of the boundaries [see Fig. 3(b)]. In addition, a fermion in the up- (down-) spin state is localized around the right (left) edge under the open boundary condition [see Fig. 3(c)].
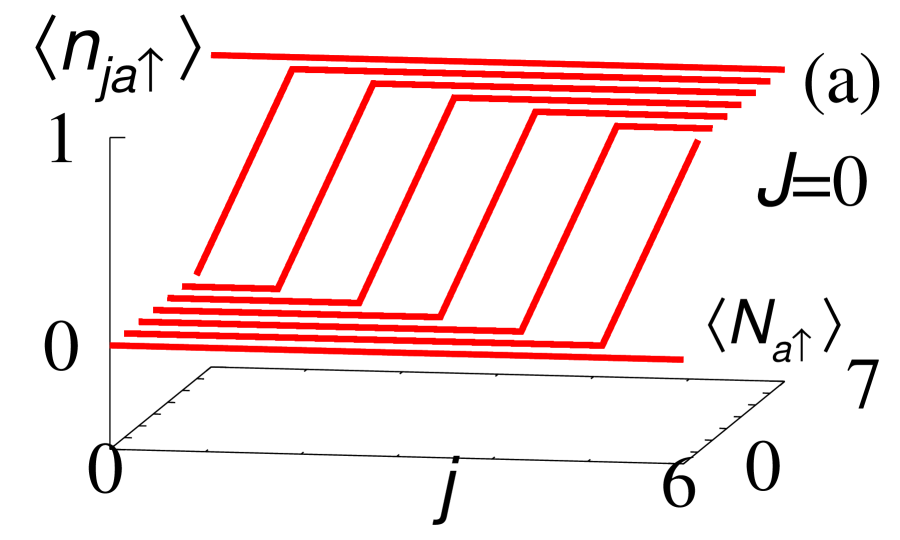
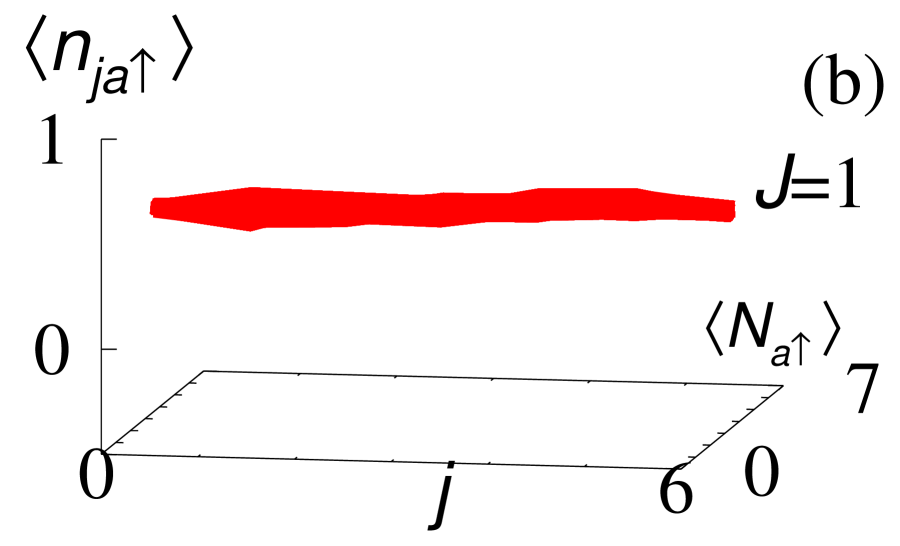
However, interactions destroy the above skin effect, which is due to the trivial topology of the many-body Hamiltonian for (for computation of the many-body winding number, see Fig. S2 of Supplemental Material sup ). Because of the trivial topology, we can observe that interactions destroy the loop structure of the spectral flow and open a line gap for [see Fig. 3(d)], which is also confirmed by analysis based on the perturbation theory (see Sec. S2.2 of Supplemental Material sup ). This result verifies the reduction of the point-gap topology for the subsector with . Correspondingly, the interactions destroy the extreme sensitivity of the spectrum to the presence/absence of boundaries [see Fig. 3(d)]. Furthermore, in the presence of interactions fermions extend to the bulk even under the open boundary condition [see Fig. 3(e)]. This result is also intuitively understood as follows: while the one-body term localizes the fermions in orbital and the up- (down-) spin state around the right (left) edge, the two-body interactions flip their spins at edges, which suppresses the effects of boundaries. The above results indicate that the skin effect observed at the non-interacting level is fragile against the two-body interactions. Our numerical calculation indicate that such fragility of the skin effect is also observed for the case of many fermions in orbital [see Fig. 4]. More detailed data are provided in Sec. S2.3 of Supplemental Material sup .
Summary and discussion–. We have analyzed correlation effects on the one-dimensional point-gap topology in both cases of synthetic and spatial dimensions. Our analysis has elucidated that the reduction occurs for systems of synthetic one dimension with charge symmetry and spin-parity symmetry. This conclusion is obtained by the argument of topological invariants as well as by explicit analysis of the toy model. Furthermore, we have also analyzed the extended Hatano-Nelson chain which exhibits striking correlation effects: correlations reduce the point-gap topology and destroy the skin effect at the non-interacting level.
The above discoveries shed new light on non-Hermitian correlated systems and open up a new directions of researches on non-Hermitian topological physics. For instance, the above results imply the possibility of similar reduction phenomena for other cases of symmetry and dimensions. As well as the above theoretical open question, experimental observation of the reduction is also a significant issue to be addressed. We expect that cold atoms are promising candidate where interactions and non-Hermiticity can be tuned in experiments.
Acknowledgements–. This work is also supported by JSPS KAKENHI Grant No. JP21K13850 and also by JST CREST, Grant No. JPMJCR19T1, Japan.
References
- Thouless et al. (1982) D. J. Thouless, M. Kohmoto, M. P. Nightingale, and M. den Nijs, Phys. Rev. Lett. 49, 405 (1982).
- Hatsugai (1993) Y. Hatsugai, Phys. Rev. Lett. 71, 3697 (1993).
- Kitaev (2001) A. Y. Kitaev, Physics-Uspekhi 44, 131 (2001).
- Kane and Mele (2005a) C. L. Kane and E. J. Mele, Phys. Rev. Lett. 95, 146802 (2005a).
- Kane and Mele (2005b) C. L. Kane and E. J. Mele, Phys. Rev. Lett. 95, 226801 (2005b).
- Qi et al. (2008) X.-L. Qi, T. L. Hughes, and S.-C. Zhang, Phys. Rev. B 78, 195424 (2008).
- Hasan and Kane (2010) M. Z. Hasan and C. L. Kane, Rev. Mod. Phys. 82, 3045 (2010).
- Qi and Zhang (2011) X.-L. Qi and S.-C. Zhang, Rev. Mod. Phys. 83, 1057 (2011).
- Sato and Fujimoto (2016) M. Sato and S. Fujimoto, Journal of the Physical Society of Japan 85, 072001 (2016).
- Tsui et al. (1982) D. C. Tsui, H. L. Stormer, and A. C. Gossard, Phys. Rev. Lett. 48, 1559 (1982).
- Laughlin (1983) R. B. Laughlin, Phys. Rev. Lett. 50, 1395 (1983).
- Jain (1989) J. K. Jain, Phys. Rev. Lett. 63, 199 (1989).
- Wen (2004) X.-G. Wen, Quantum field theory of many-body systems: from the origin of sound to an origin of light and electrons (OUP Oxford, 2004).
- Levin and Wen (2005) M. A. Levin and X.-G. Wen, Phys. Rev. B 71, 045110 (2005).
- Kitaev (2003) A. Kitaev, Annals of Physics 303, 2 (2003).
- Kitaev (2006) A. Kitaev, Annals of Physics 321, 2 (2006), january Special Issue.
- Fidkowski and Kitaev (2010) L. Fidkowski and A. Kitaev, Phys. Rev. B 81, 134509 (2010).
- Turner et al. (2011) A. M. Turner, F. Pollmann, and E. Berg, Phys. Rev. B 83, 075102 (2011).
- Fidkowski and Kitaev (2011) L. Fidkowski and A. Kitaev, Phys. Rev. B 83, 075103 (2011).
- Yao and Ryu (2013) H. Yao and S. Ryu, Phys. Rev. B 88, 064507 (2013).
- Ryu and Zhang (2012) S. Ryu and S.-C. Zhang, Phys. Rev. B 85, 245132 (2012).
- Qi (2013) X.-L. Qi, New J. Phys. 15, 065002 (2013).
- Lu and Vishwanath (2013) Y.-M. Lu and A. Vishwanath, arXiv preprint arXiv:1302.2634 (2013).
- Levin (2013) M. Levin, Phys. Rev. X 3, 021009 (2013).
- Isobe and Fu (2015) H. Isobe and L. Fu, arXiv preprint arXiv:1502.06962 (2015).
- Yoshida et al. (2017) T. Yoshida, A. Daido, Y. Yanase, and N. Kawakami, Phys. Rev. Lett. 118, 147001 (2017).
- Fidkowski et al. (2013) L. Fidkowski, X. Chen, and A. Vishwanath, Phys. Rev. X 3, 041016 (2013).
- Wang et al. (2014) C. Wang, A. C. Potter, and T. Senthil, 343, 629 (2014).
- Metlitski et al. (2014) M. A. Metlitski, L. Fidkowski, X. Chen, and A. Vishwanath, arXiv:1406.3032 (2014).
- Wang and Senthil (2014) C. Wang and T. Senthil, Phys. Rev. B 89, 195124 (2014).
- You and Xu (2014) Y.-Z. You and C. Xu, Phys. Rev. B 90, 245120 (2014).
- Morimoto et al. (2015) T. Morimoto, A. Furusaki, and C. Mudry, Phys. Rev. B 92, 125104 (2015).
- Jian and Xu (2018) C.-M. Jian and C. Xu, Phys. Rev. X 8, 041030 (2018).
- Boada et al. (2012) O. Boada, A. Celi, J. I. Latorre, and M. Lewenstein, Phys. Rev. Lett. 108, 133001 (2012).
- Celi et al. (2014) A. Celi, P. Massignan, J. Ruseckas, N. Goldman, I. B. Spielman, G. Juzeliūnas, and M. Lewenstein, Phys. Rev. Lett. 112, 043001 (2014).
- Nakajima et al. (2016) S. Nakajima, T. Tomita, S. Taie, T. Ichinose, H. Ozawa, L. Wang, M. Troyer, and Y. Takahashi, Nature Physics 12, 296 (2016).
- Lohse et al. (2016) M. Lohse, C. Schweizer, O. Zilberberg, M. Aidelsburger, and I. Bloch, Nature Physics 12, 350 (2016).
- Hatano and Nelson (1996) N. Hatano and D. R. Nelson, Phys. Rev. Lett. 77, 570 (1996).
- Bender and Boettcher (1998) C. M. Bender and S. Boettcher, Phys. Rev. Lett. 80, 5243 (1998).
- Hu and Hughes (2011) Y. C. Hu and T. L. Hughes, Phys. Rev. B 84, 153101 (2011).
- Esaki et al. (2011) K. Esaki, M. Sato, K. Hasebe, and M. Kohmoto, Phys. Rev. B 84, 205128 (2011).
- Bergholtz et al. (2021) E. J. Bergholtz, J. C. Budich, and F. K. Kunst, Rev. Mod. Phys. 93, 015005 (2021).
- Ashida et al. (2020) Y. Ashida, Z. Gong, and M. Ueda, Advances in Physics 69, 249 (2020).
- Martinez Alvarez et al. (2018) V. M. Martinez Alvarez, J. E. Barrios Vargas, and L. E. F. Foa Torres, Phys. Rev. B 97, 121401 (2018).
- Kunst et al. (2018) F. K. Kunst, E. Edvardsson, J. C. Budich, and E. J. Bergholtz, Phys. Rev. Lett. 121, 026808 (2018).
- Yao and Wang (2018) S. Yao and Z. Wang, Phys. Rev. Lett. 121, 086803 (2018).
- Yao et al. (2018) S. Yao, F. Song, and Z. Wang, Phys. Rev. Lett. 121, 136802 (2018).
- Yokomizo and Murakami (2019) K. Yokomizo and S. Murakami, Phys. Rev. Lett. 123, 066404 (2019).
- Edvardsson et al. (2019) E. Edvardsson, F. K. Kunst, and E. J. Bergholtz, Phys. Rev. B 99, 081302 (2019).
- Gong et al. (2018) Z. Gong, Y. Ashida, K. Kawabata, K. Takasan, S. Higashikawa, and M. Ueda, Phys. Rev. X 8, 031079 (2018).
- Carlström and Bergholtz (2018) J. Carlström and E. J. Bergholtz, Phys. Rev. A 98, 042114 (2018).
- Carlström et al. (2019) J. Carlström, M. Stålhammar, J. C. Budich, and E. J. Bergholtz, Phys. Rev. B 99, 161115 (2019).
- Zhou and Lee (2019) H. Zhou and J. Y. Lee, Phys. Rev. B 99, 235112 (2019).
- Kawabata et al. (2019a) K. Kawabata, K. Shiozaki, M. Ueda, and M. Sato, Phys. Rev. X 9, 041015 (2019a).
- Katō (1966) T. Katō, Perturbation theory for linear operators, Vol. 132 (Springer, 1966).
- Rotter (2009) I. Rotter, Journal of Physics A: Mathematical and Theoretical 42, 153001 (2009).
- Berry (2004) M. V. Berry, Czechoslovak Journal of Physics 54, 1039 (2004).
- Heiss (2012) W. D. Heiss, J. Phys. A 45, 444016 (2012).
- Shen et al. (2018) H. Shen, B. Zhen, and L. Fu, Phys. Rev. Lett. 120, 146402 (2018).
- Budich et al. (2019) J. C. Budich, J. Carlström, F. K. Kunst, and E. J. Bergholtz, Phys. Rev. B 99, 041406 (2019).
- Yoshida et al. (2019a) T. Yoshida, R. Peters, N. Kawakami, and Y. Hatsugai, Phys. Rev. B 99, 121101 (2019a).
- Okugawa and Yokoyama (2019) R. Okugawa and T. Yokoyama, Phys. Rev. B 99, 041202 (2019).
- Zhou et al. (2019) H. Zhou, J. Y. Lee, S. Liu, and B. Zhen, Optica 6, 190 (2019).
- Kawabata et al. (2019b) K. Kawabata, T. Bessho, and M. Sato, Phys. Rev. Lett. 123, 066405 (2019b).
- Kimura et al. (2019) K. Kimura, T. Yoshida, and N. Kawakami, Phys. Rev. B 100, 115124 (2019).
- Yoshida et al. (2020a) T. Yoshida, R. Peters, N. Kawakami, and Y. Hatsugai, Progress of Theoretical and Experimental Physics 2020, 12A109 (2020a).
- Delplace et al. (2021) P. Delplace, T. Yoshida, and Y. Hatsugai, Phys. Rev. Lett. 127, 186602 (2021).
- Mandal and Bergholtz (2021) I. Mandal and E. J. Bergholtz, Phys. Rev. Lett. 127, 186601 (2021).
- Lee and Thomale (2019) C. H. Lee and R. Thomale, Phys. Rev. B 99, 201103 (2019).
- Borgnia et al. (2020) D. S. Borgnia, A. J. Kruchkov, and R.-J. Slager, Phys. Rev. Lett. 124, 056802 (2020).
- Zhang et al. (2020a) K. Zhang, Z. Yang, and C. Fang, Phys. Rev. Lett. 125, 126402 (2020a).
- Okuma et al. (2020) N. Okuma, K. Kawabata, K. Shiozaki, and M. Sato, Phys. Rev. Lett. 124, 086801 (2020).
- Yoshida et al. (2020b) T. Yoshida, T. Mizoguchi, and Y. Hatsugai, Phys. Rev. Research 2, 022062 (2020b).
- Okugawa et al. (2020) R. Okugawa, R. Takahashi, and K. Yokomizo, Phys. Rev. B 102, 241202 (2020).
- Kawabata et al. (2020) K. Kawabata, M. Sato, and K. Shiozaki, Phys. Rev. B 102, 205118 (2020).
- Jin and Song (2009a) L. Jin and Z. Song, Phys. Rev. A 80, 052107 (2009a).
- Lee (2016) T. E. Lee, Phys. Rev. Lett. 116, 133903 (2016).
- San-Jose et al. (2016) P. San-Jose, J. Cayao, E. Prada, and R. Aguado, Scientific Reports 6, 21427 (2016).
- Xu et al. (2017) Y. Xu, S.-T. Wang, and L.-M. Duan, Phys. Rev. Lett. 118, 045701 (2017).
- Jin and Song (2009b) L. Jin and Z. Song, Phys. Rev. A 80, 052107 (2009b).
- Kozii and Fu (2017) V. Kozii and L. Fu, arXiv preprint arXiv:1708.05841 (2017).
- Zyuzin and Zyuzin (2018) A. A. Zyuzin and A. Y. Zyuzin, Phys. Rev. B 97, 041203 (2018).
- Yoshida et al. (2018) T. Yoshida, R. Peters, and N. Kawakami, Phys. Rev. B 98, 035141 (2018).
- Shen and Fu (2018) H. Shen and L. Fu, Phys. Rev. Lett. 121, 026403 (2018).
- Papaj et al. (2019) M. Papaj, H. Isobe, and L. Fu, Phys. Rev. B 99, 201107 (2019).
- Matsushita et al. (2019) T. Matsushita, Y. Nagai, and S. Fujimoto, Phys. Rev. B 100, 245205 (2019).
- Michishita and Peters (2020) Y. Michishita and R. Peters, Phys. Rev. Lett. 124, 196401 (2020).
- Guo et al. (2009) A. Guo, G. J. Salamo, D. Duchesne, R. Morandotti, M. Volatier-Ravat, V. Aimez, G. A. Siviloglou, and D. N. Christodoulides, Phys. Rev. Lett. 103, 093902 (2009).
- Rüter et al. (2010) C. E. Rüter, K. G. Makris, R. El-Ganainy, D. N. Christodoulides, M. Segev, and D. Kip, Nature physics 6, 192 (2010).
- Regensburger et al. (2012) A. Regensburger, C. Bersch, M.-A. Miri, G. Onishchukov, D. N. Christodoulides, and U. Peschel, Nature 488, 167 (2012).
- Zhen et al. (2015) B. Zhen, C. W. Hsu, Y. Igarashi, L. Lu, I. Kaminer, A. Pick, S.-L. Chua, J. D. Joannopoulos, and M. Soljacic, Nature 525, 354 (2015).
- Hassan et al. (2017) A. U. Hassan, B. Zhen, M. Soljačić, M. Khajavikhan, and D. N. Christodoulides, Phys. Rev. Lett. 118, 093002 (2017).
- Zhou et al. (2018) H. Zhou, C. Peng, Y. Yoon, C. W. Hsu, K. A. Nelson, L. Fu, J. D. Joannopoulos, M. Soljačić, and B. Zhen, 359, 1009 (2018).
- Takata and Notomi (2018) K. Takata and M. Notomi, Phys. Rev. Lett. 121, 213902 (2018).
- Ozawa et al. (2019) T. Ozawa, H. M. Price, A. Amo, N. Goldman, M. Hafezi, L. Lu, M. C. Rechtsman, D. Schuster, J. Simon, O. Zilberberg, and I. Carusotto, Rev. Mod. Phys. 91, 015006 (2019).
- Xiao et al. (2020) L. Xiao, T. Deng, K. Wang, G. Zhu, Z. Wang, W. Yi, and P. Xue, Nature Physics 16, 761 (2020).
- Weidemann et al. (2020) S. Weidemann, M. Kremer, T. Helbig, T. Hofmann, A. Stegmaier, M. Greiter, R. Thomale, and A. Szameit, Science 368, 311 (2020).
- Hofmann et al. (2020) T. Hofmann, T. Helbig, F. Schindler, N. Salgo, M. Brzezińska, M. Greiter, T. Kiessling, D. Wolf, A. Vollhardt, A. Kabaši, C. H. Lee, A. Bilušić, R. Thomale, and T. Neupert, Phys. Rev. Research 2, 023265 (2020).
- Helbig et al. (2020) T. Helbig, T. Hofmann, S. Imhof, M. Abdelghany, T. Kiessling, L. W. Molenkamp, C. H. Lee, A. Szameit, M. Greiter, and R. Thomale, Nature Physics 16, 747 (2020).
- Yoshida and Hatsugai (2019) T. Yoshida and Y. Hatsugai, Phys. Rev. B 100, 054109 (2019).
- Ghatak et al. (2020) A. Ghatak, M. Brandenbourger, J. van Wezel, and C. Coulais, Proceedings of the National Academy of Sciences 117, 29561 (2020).
- Scheibner et al. (2020) C. Scheibner, W. T. M. Irvine, and V. Vitelli, Phys. Rev. Lett. 125, 118001 (2020).
- Yoshida et al. (2019b) T. Yoshida, K. Kudo, and Y. Hatsugai, Scientific Reports 9, 16895 (2019b).
- Xi et al. (2021) W. Xi, Z.-H. Zhang, Z.-C. Gu, and W.-Q. Chen, Science Bulletin 66, 1731 (2021).
- Yoshida et al. (2020c) T. Yoshida, K. Kudo, H. Katsura, and Y. Hatsugai, Phys. Rev. Research 2, 033428 (2020c).
- Guo et al. (2020a) C.-X. Guo, X.-R. Wang, C. Wang, and S.-P. Kou, Phys. Rev. B 101, 144439 (2020a).
- Matsumoto et al. (2020) N. Matsumoto, K. Kawabata, Y. Ashida, S. Furukawa, and M. Ueda, Phys. Rev. Lett. 125, 260601 (2020).
- Zhang et al. (2020b) Q. Zhang, W.-T. Xu, Z.-Q. Wang, and G.-M. Zhang, Communications Physics 3, 209 (2020b).
- Guo et al. (2020b) C.-X. Guo, X.-R. Wang, and S.-P. Kou, EPL (Europhysics Letters) 131, 27002 (2020b).
- Shackleton and Scheurer (2020) H. Shackleton and M. S. Scheurer, Phys. Rev. Research 2, 033022 (2020).
- Yang et al. (2021) K. Yang, S. C. Morampudi, and E. J. Bergholtz, Phys. Rev. Lett. 126, 077201 (2021).
- Zhang et al. (2020c) D.-W. Zhang, Y.-L. Chen, G.-Q. Zhang, L.-J. Lang, Z. Li, and S.-L. Zhu, Phys. Rev. B 101, 235150 (2020c).
- Liu et al. (2020) T. Liu, J. J. He, T. Yoshida, Z.-L. Xiang, and F. Nori, Phys. Rev. B 102, 235151 (2020).
- Xu and Chen (2020) Z. Xu and S. Chen, Phys. Rev. B 102, 035153 (2020).
- Pan et al. (2020) L. Pan, X. Wang, X. Cui, and S. Chen, Phys. Rev. A 102, 023306 (2020).
- Mu et al. (2020) S. Mu, C. H. Lee, L. Li, and J. Gong, Phys. Rev. B 102, 081115 (2020).
- Lee (2021) C. H. Lee, Phys. Rev. B 104, 195102 (2021).
- Zhang et al. (2022) S.-B. Zhang, M. M. Denner, T. Bzdušek, M. A. Sentef, and T. Neupert, arXiv preprint arXiv:2201.12653 (2022).
- Kawabata et al. (2022) K. Kawabata, K. Shiozaki, and S. Ryu, Phys. Rev. B 105, 165137 (2022).
- Tsubota et al. (2021) S. Tsubota, H. Yang, Y. Akagi, and H. Katsura, arXiv preprint arXiv:2108.12860 (2021).
- Qin et al. (2022) F. Qin, R. Shen, and C. H. Lee, arXiv preprint arXiv:2202.10481 (2022).
- Orito and Imura (2022) T. Orito and K.-I. Imura, Phys. Rev. B 105, 024303 (2022).
- Tomita et al. (2017) T. Tomita, S. Nakajima, I. Danshita, Y. Takasu, and Y. Takahashi, 3 (2017), 10.1126/sciadv.1701513.
- Takasu et al. (2020) Y. Takasu, T. Yagami, Y. Ashida, R. Hamazaki, Y. Kuno, and Y. Takahashi, Progress of Theoretical and Experimental Physics 2020 (2020), 10.1093/ptep/ptaa094, 12A110.
- Yoshida and Hatsugai (2021) T. Yoshida and Y. Hatsugai, Phys. Rev. B 104, 075106 (2021).
- (126) We note that introducing additional terms makes other subsectors irrelevant. For instance adding the term with to Eq. (1) can make subsectors labeled by irrelevant to the topology for .
- (127) Supplemental Material for detailed results for the toy model in zero dimension and the extended Hatano-Nelson chain.
Supplemental Materials:
Reduction of one-dimensional non-Hermitian point-gap topology by correlations
S1 Analysis of a two orbital model for the subsector with
In the main text, we have seen that interactions open a line-gap for the subsector with , which is consistent with the trivial topology for . In this section, we show that a similar behavior is observed for the subsector with .
Despite the non-trivial topology of the one-body Hamiltonian, the many-body winding number takes zero [] for as shown in Fig. S1(a). Correspondingly, the spectrum of the many-body Hamiltonian can smoothly shrink to the points [see Figs. S1(b)-S1(d)].
In this subsector, the interaction is essential for destruction of the loop structure observed in Fig. S1(a), which can be seen as follows. In the subsector with , the Hamiltonian is written as
| (S1a) | |||||
| (S1b) | |||||
| (S1g) | |||||
with denoting a diagonal matrix. Here, we have chosen the following basis vectors spanning the subsector of the Fock space
| (S2) |
Diagonalizing the Hamiltonian, we obtain
| (S3) | |||||
| (S4) | |||||
| (S5) |
with and . The above results elucidate that the spin-parity symmetry allows the two-body interaction which splits loop structure observed in Fig. S1(a).
S2 Details of the extended Hatano-Nelson chain
S2.1 Hamiltonian under the twisted boundary condition
We provide the explicit form of the extended Hatano-Nelson chain under the twisted boundary condition. The Hamiltonian reads
| (S6a) | |||||
| (S6b) | |||||
| (S6c) | |||||
Under a gauge transformation , the one-body term is written as
| (S7) |
with and .
Applying the Fourier transformation to the above Hamiltonian yields Eq. (13). We note that under the open boundary condition, hopping terms between sites and [i.e., the first and the third terms of Eq. (S6b)] become zero.
In the presence of charge symmetry and spin-parity symmetry, the one-body Hamiltonian is characterized by and [Eqs. (3) and (4)] with . The topology of the many-body Hamiltonian for given subsector with is characterized by the many-body winding number [Eq. (5)] with . Here, denotes the many-body Hamiltonian of the extended Hatano-Nelson model for the given subsector with .
S2.2 Analysis based on the perturbation theory
Based on the perturbation theory, we confirm that interactions open a line-gap as shown in Fig. 3(d). As mentioned in the main text, we suppose that orbital is occupied at both edges ().
Suppose that interactions are sufficiently weak. In the subsector of , is written as
| (S12) |
with and the basis
| (S13) |
for given (). Here, is defined as with and . Matrices and ( and ) diagonalize the matrix ()
| (S14) |
which corresponds to the kinetic term of fermions in orbital and the up-spin state for [see Eq. (S6b) and Fig. 3(a)]. Here describes a diagonal matrix. Introducing the operators and , we have anti-commutation relations
| (S15) |
for and , which can be seen by noting the relations and . We note that holds due to the relation .
Now, let us compute energy eigenvalues at the first order of the interactions. Firstly, we note the following relations.
| (S16) | |||||
| (S17) | |||||
Here, we have used the relations , , and .
Thus, at the first order, the Hamiltonian is written as with in Eq. (S12) and
| (S26) |
for the basis defined in Eq. (S13).
The eigenvalues of are written as
| (S27a) | |||||
| (S27b) | |||||
| with | |||||
| (S27c) | |||||
| (S27d) | |||||
As well as by directly diagonalizing the matrix, the eigenvalues are obtained by taking square of the matrices (see below). These results indicate that interactions lift four-fold degeneracy observed for . Specifically, the imaginary parts of and lift the degeneracy. To see this, firstly, let us suppose that the imaginary parts are zero (), then, Eq. (S27a) indicates that exceptional points emerge at certain [i.e., holds, and can be satisfied]. On the other hand, the finite imaginary parts lift the degeneracy at without inducing exceptional points [i.e., for ].
Equations (S27c) and (S27d) indicate that the imaginary parts of and can be finite for proper choice of , , and . Therefore, the above result of the first-order perturbation theory indicate that interactions open a line-gap.
We show that eigenvalues (S27) can be obtained by taking squares of the matrices. Consider the following matrix
| (S28) |
with complex numbers , , , , , , and . Here and denote the -identity matrix. Pauli matrices are denoted by and (). Matrices () denote -matrices. For instance, is written as
| (S33) |
For the following parameter set,
| (S50) |
is reduced to the matrix .
S2.3 Numerical results
In the main text, we have briefly discussed the extended Hatano-Nelson chain. Here, let us numerically analyze this system in detail.
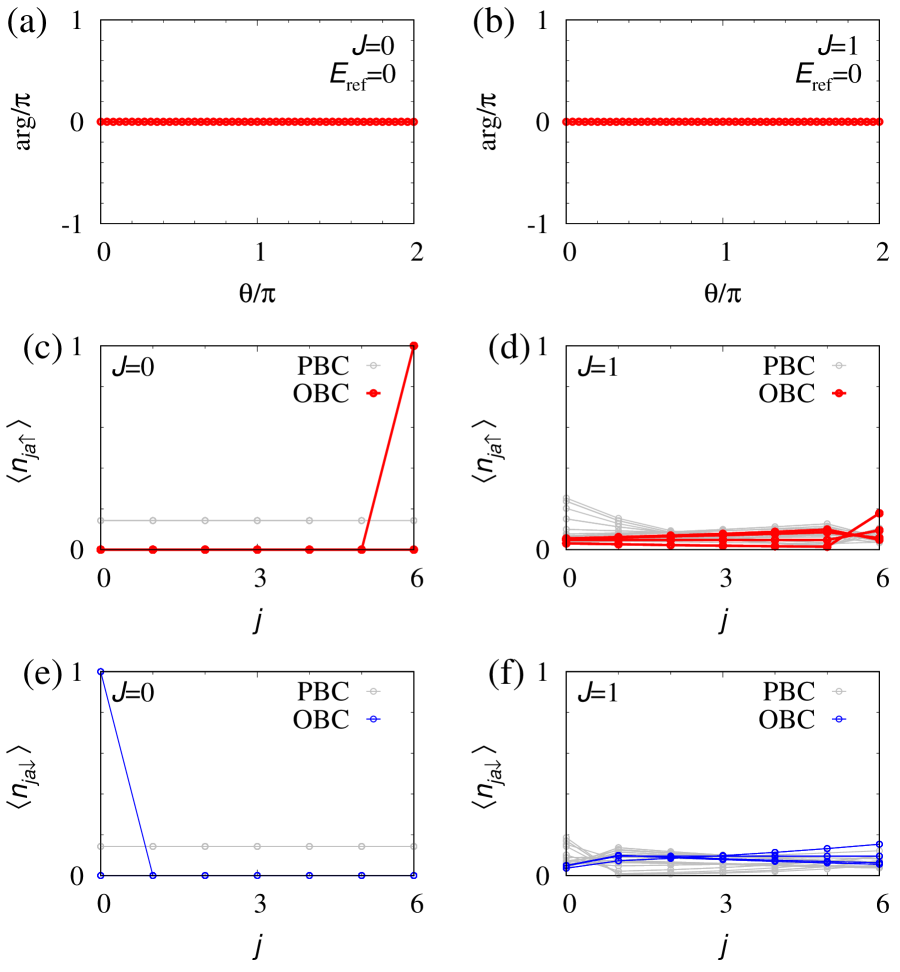
Firstly, we focus on the subsector with [see Fig. S2]. Although the topology of the one-body Hamiltonian is non-trivial [i.e., for ], the many-body Hamiltonian is topologically trivial [i.e., for ] as shown in Figs. S2(a) and S2(b). This fact results in the fragility of the skin effect against interactions. Namely, although the fermion with the up- (down-) spin state is localized at the right (left) edge due to the skin effect in the non-interacting case [see Figs. S2(c) and S2(e)], such localization cannot be observed in the presence of the interactions [see Figs. S2(d) and S2(f)]. Correspondingly, the extreme sensitivity of the energy spectrum to the boundary condition is not observed for [see Figs. 3(b) and 3(d)].
This fragility of the skin effect is intuitively understood as follows: the interactions flip the spin of fermions in orbital , which suppresses the effects of the boundaries.
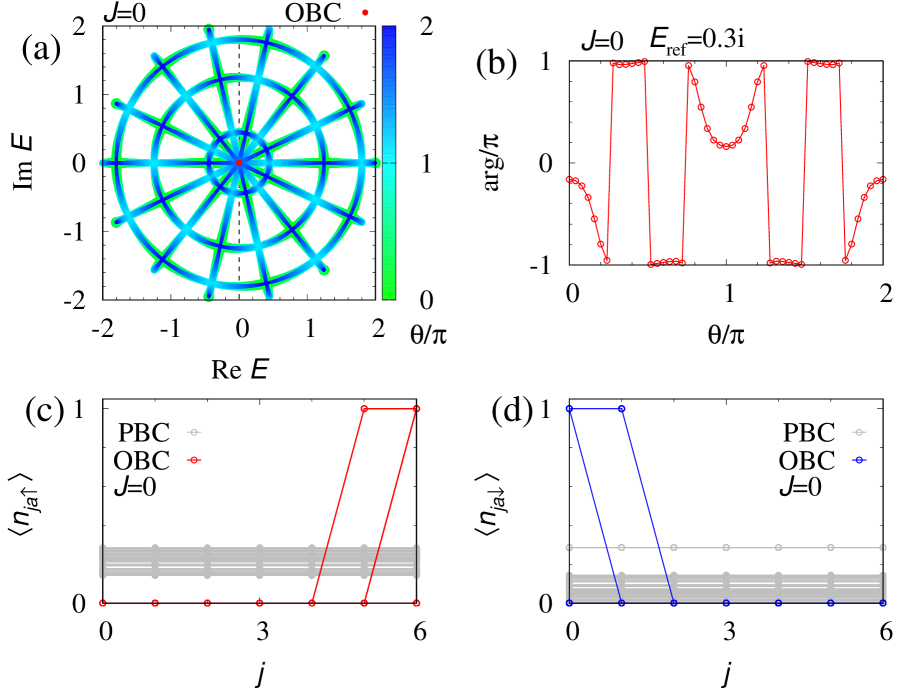
Now, let us focus on the subsector with [see Figs. S3 and S4]. Figures S3(a) and S3(b) indicate the topology of the many-body Hamiltonian is trivial. However, due to the topology of the one-body Hamiltonian, we can observe the extreme sensitivity of the spectrum and expectation values to the presence/absence of the boundaries [see Figs. S3(c) and S3(d)]. As is the case for , such extreme sensitivity is fragile against interactions [see Fig. S4].
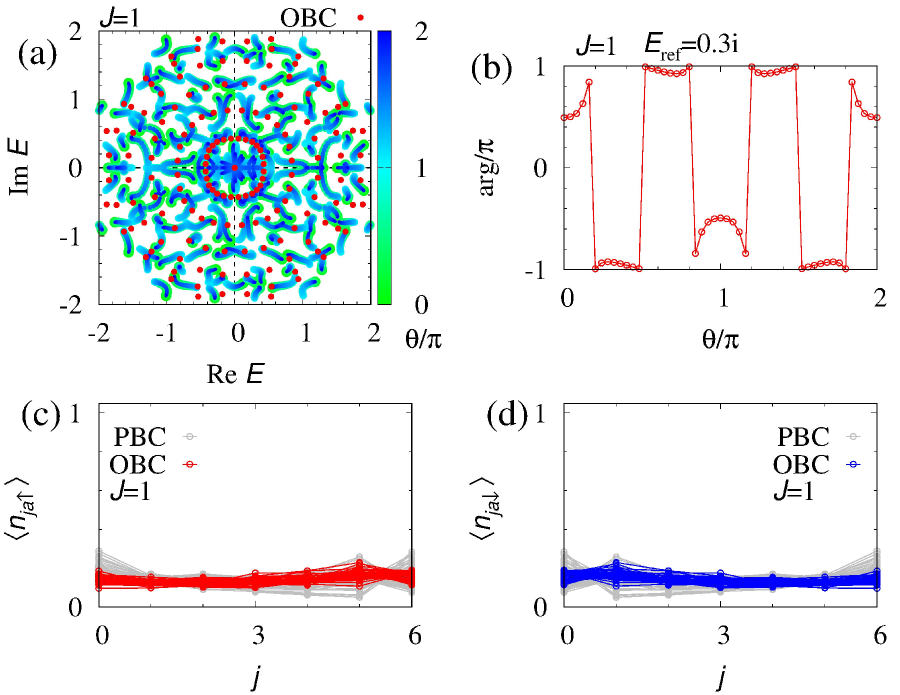
Finally, we discuss the case for where orbital is half-filled. Figure S5 indicates that the many-body Hamiltonian is topologically trivial, which results in fragility of the skin effect at the non-interacting level as discussed in the above.
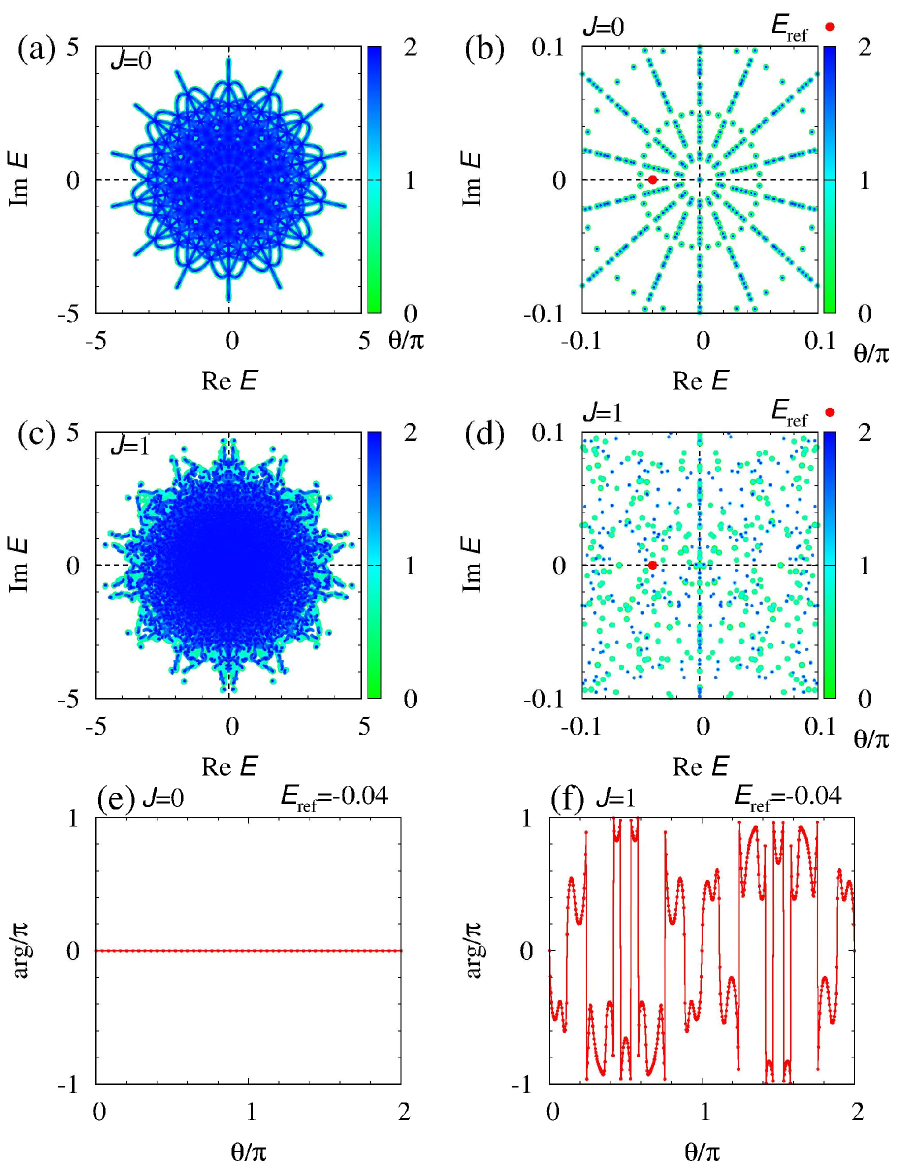
Namely, while the topology of the one-body Hamiltonian induces the extreme sensitivity of the energy spectrum and the expectation values to the boundary conditions, such extreme sensitivity is not observed in the interacting case [see Fig. S6].