Nonequilibrium steady-state picture of incoherent light-induced excitation harvesting
Abstract
We formulate a comprehensive theoretical description of excitation harvesting in molecular aggregates photoexcited by weak incoherent radiation. An efficient numerical scheme that respects the continuity equation for excitation fluxes is developed to compute the nonequilibrium steady state (NESS) arising from the interplay between excitation generation, excitation relaxation, dephasing, trapping at the load, and recombination. The NESS is most conveniently described in the so-called preferred basis, in which the steady-state excitonic density matrix is diagonal. The NESS properties are examined by relating the preferred-basis description to the descriptions in the site or excitonic bases. Focusing on a model photosynthetic dimer, we find that the NESS in the limit of long trapping time is quite similar to the excited-state equilibrium, in which the stationary coherences originate from the excitation–environment entanglement. For shorter trapping times, we demonstrate how the properties of the NESS can be extracted from the time-dependent description of an incoherently driven, but unloaded dimer. This relation between stationary and time-dependent pictures is valid provided that the trapping time is longer than the decay time of dynamic coherences accessible in femtosecond spectroscopy experiments.
I Introduction
The observation of unexpectedly long-lived oscillatory features of ultrafast spectroscopic signals measured on photosynthetic pigment–protein complexes Engel et al. (2007); Panitchayangkoon et al. (2010) has generated much excitement during the last decade. The idea that (dynamic) coherences modulating these signals may be directly relevant to natural light harvesting, Cheng and Fleming (2009); Ishizaki and Fleming (2011) which is triggered by stationary incoherent sunlight, Brumer and Shapiro (2012); Brumer (2018) has been driving intense research activities in the field. Mančal (2020); Cao et al. (2020) Insights from ultrafast spectroscopies are indispensable in determining the underlying Hamiltonian of the system under investigation, from which the dynamics of excitation energy transfer (EET) under any excitation condition may be inferred (as long as the excitation is sufficiently weak). Mančal and Valkunas (2010); Chenu, Malý, and Mančal (2014); Brumer (2018); Scholes (2018); Mančal (2020) A number of experimental Engel et al. (2007); Panitchayangkoon et al. (2010) and theoretical Ishizaki and Fleming (2009a); Sarovar et al. (2010) studies speculating about a positive impact of coherences and entanglement on the biological EET have been performed on the so-called unloaded systems. Such systems feature no transmission of photoinduced excitations to the reaction center (RC or load) from which the excitation energy is eventually harvested. Furthermore, the time scales addressed in these studies are generally much shorter than those representative of exciton recombination, either radiative or nonradiative. van Amerongen, Valkunas, and van Grondelle (2000) Direct experimental insights into the dynamics of molecular aggregates initiated by incoherent light are limited. Turner et al. (2013) Therefore, at present, the possible relevance of some sort of quantum coherence for EET under natural conditions is best examined within appropriate theoretical models.
Such models should contain a realistic description of photoexcitation by natural incoherent light, whose intensity is essentially constant from the molecular viewpoint. Thus, the physically plausible description of natural light harvesting should feature continuous generation of electronic excitations by light, their continuous delivery to the RC, and their continuous loss by recombination. Kassal, Yuen-Zhou, and Rahimi-Keshari (2013); Tscherbul and Brumer (2018); Manzano (2013); Brumer (2018); Jesenko and Žnidarič (2013); Chuang and Brumer (2020) The EET is then studied from the standpoint of nonequilibrium steady states (NESSs), which arise as a result of excitation photogeneration, phonon-induced relaxation, dephasing, trapping at the RC, and recombination. Furthermore, the coupling between the radiation and absorbing pigments is, in general, weak, so that its second-order treatment is reasonable. Then, the only information we need about the radiation is its first-order correlation function, Mančal and Valkunas (2010) which can be either modeled by appropriate expressions Mančal and Valkunas (2010); Sadeq and Brumer (2014) or obtained by a suitable ensemble average. Sadeq and Brumer (2014); Chenu and Brumer (2016) Excitation and deexcitation events can be treated within the Born–Markov approximation Breuer and Petruccione (2002) by Lindblad dissipators, Fassioli, Olaya-Castro, and Scholes (2012); Chan et al. (2018) or by establishing the Bloch–Redfield quantum master equation. Tscherbul and Brumer (2015, 2018) Approaching the problem from the perspective of open quantum systems, one can introduce an appropriate spectral density of light–matter coupling, Pachón and Brumer (2013); Pachón, Botero, and Brumer (2017); Olšina et al. (2014); Chuang and Brumer (2020) and possibly treat it even beyond the second order. Olšina et al. (2014)
Reasonable models of photosynthetic EET should not overlook the non-Markovian interplay between photoinduced electronic excitations and nuclear reorganization processes, Ishizaki and Fleming (2012); Chenu and Scholes (2015) whose relevance is emphasized by ultrafast spectroscopic studies. To that end, a number of studies attempt to combine an explicit treatment of the photoexcitation step with a nonperturbative approach to the excitation–environment coupling. Fassioli, Olaya-Castro, and Scholes (2012); Chan et al. (2018); Maguire, Iles-Smith, and Nazir (2019); Dijkstra and Beige (2019); Janković and Mančal (2020); Olšina et al. (2014) The method of choice for an exhaustive treatment of excitation–environment coupling are hierarchical equations of motion (HEOM). Ishizaki and Fleming (2009b); Tanimura (2020) In the accompanying paper, Janković and Mančal (2020) we combine HEOM with a second-order treatment of light–matter coupling for light of arbitrary properties. Our method correctly captures light-induced reorganization processes and nonequilibrium evolution of the bath between the two interactions with light.
Recently, a number of groups have suggested that stationary coherences in the energy basis (interexciton coherences) or local basis (intersite coherences) under incoherent illumination may improve the light-harvesting efficiency in photosynthetic systems. Tscherbul and Brumer (2018); Tomasi and Kassal (2020); Yang and Cao (2020); Jung and Brumer (2020) However, the majority of existing theoretical approaches to NESSs in photosynthetic light harvesting typically feature a simplified treatment of the photoexcitation Jesenko and Žnidarič (2013); León-Montiel, Kassal, and Torres (2014); Manzano (2013) or a simplified treatment of excitation relaxation and dephasing. León-Montiel, Kassal, and Torres (2014); Tscherbul and Brumer (2018); Manzano (2013); Yang and Cao (2020); Jung and Brumer (2020) Also, efficient algorithms that avoid the explicit temporal propagation in the computation of NESSs induced by natural incoherent light have just begun to be developed. Axelrod and Brumer (2018) Therefore, there is still an urge to construct theoretical methods that circumvent the disparity between the time scales of EET dynamics and incoherent excitation sources, and yet meet the two requirements outlined in the above text.
Another pertaining issue is the origin of stationary coherences, i.e., whether they are primarily induced by incoherent radiation or by the coupling to the protein environment. The authors of Ref. Olšina et al., 2014 argued that the NESS coherences ultimately stem from the entanglement of electronic excitations with the environment. This entanglement has been systematically studied both analytically Lee, Cao, and Gong (2012); Lee, Moix, and Cao (2012) and numerically Lee, Cao, and Gong (2012); Cerrillo and Cao (2014) within the undriven and unloaded spin–boson model. The two-level system displays noncanonical equilibrium statistics, Strümpfer and Schulten (2009); Moix, Zhao, and Cao (2012) whose deviation from the canonical equilibrium statistics can be conveniently measured by a single parameter. Lee, Cao, and Gong (2012) This parameter can be interpreted as the angle by which the basis in which the system’s Hamiltonian (or the system–bath interaction Hamiltonian) is diagonal should be rotated to obtain the diagonal reduced density matrix (RDM). The basis in which the RDM is diagonal is thus singled out by the environment, and the corresponding basis states are known as the preferred (or pointer) states within the framework of the decoherence theory. Zurek (2003); Schlosshauer (2007) The concept of preferred basis is useful whenever representation-dependent issues, such as the ones we are after in this study, arise.
In this paper, we extend the ideas developed in Ref. Lee, Cao, and Gong, 2012 to examine the properties of the NESS that arises in an incoherently driven and loaded excitonic aggregate. Adapting the algorithm presented in Ref. Zhang et al., 2017, we devise a procedure to find the NESS of our recently proposed HEOM that incorporates incoherent photoexcitation, Janković and Mančal (2020) and to properly define light-harvesting efficiency under incoherent illumination. Our theoretical approach fully respects the continuity equation for excitation fluxes. The NESS is most conveniently described in the so-called preferred basis, in which the steady-state RDM is diagonal. Such a description of a driven and loaded aggregate may be regarded as analogous to the normal-mode description of a system of coupled harmonic oscillators. While our theoretical method is quite general and applicable to arbitrary excitonic networks, we investigate the properties of the NESS using the appropriately parameterized model dimer. For realistic values of the load extraction time, we conclude that light-induced coherences are completely irrelevant in the NESS, which is then close to the noncanonical equilibrium of the undriven and unloaded dimer. We find that the NESS of the driven and loaded dimer is intimately related to the dynamics of the driven, but unloaded dimer, that takes place on the time scale of the excitation trapping at the load. This close connection between the dynamic and stationary picture is correct provided that the load extraction time is longer than the time scale of coherence dephasing, which is in principle accessible in ultrafast spectroscopies.
The paper is structured as follows: The model and method are presented in Secs. II and III, respectively. In Sec. IV, we discuss the relation of the preferred-basis description of the NESS to more standard descriptions conducted in the site or excitonic basis. Section V presents numerical results, with an emphasis on the relation between the time-dependent and stationary picture. In Sec. VI, we outline how the methodology presented in this work could be applied to multichromophoric systems. Section VII concludes the paper by summarizing its principal findings.
II Minimal Model
We consider the simplest EET system, a molecular aggregate composed of two mutually coupled chromophores (a dimer, a spin–boson-like model Zimanyi and Silbey (2012)). Although a similar model has been repeatedly used by many authors to gain insight into fundamentals of light harvesting under incoherent illumination, Jesenko and Žnidarič (2013); Olšina et al. (2014); Tscherbul and Brumer (2014, 2018); Tomasi et al. (2019); Yang and Cao (2020); Jung and Brumer (2020) our analysis uses a rigorous theoretical approach to investigate in greater detail certain properties of the NESS that have not received enough attention so far. The dimer system can be considered as the minimal model of a photosynthetic antenna with delocalization in which one can study the effects of energy relaxation, dephasing, and (static) disorder in local transition energies (the so-called asymmetric dimer, see Sec. V.1). To be specific, we speak about the model dimer, while we note that the model and the method to be presented are quite general and applicable to multichromophoric situations.
Electronic excitations of the model dimer are modeled within the Frenkel exciton model Valkunas, Abramavicius, and Mančal (2013); May and Kühn (2011) and the corresponding Hamiltonian reads as
| (1) |
In Eq. (1), is the singly excited state localized on chromophore , is its vertical excitation energy, while are resonance couplings (we take ). We limit our discussion to the manifold of singly excited states, which is justified under the assumption that the driving by the radiation is sufficiently weak. The aggregate is in contact with the thermal bath, which represents its protein environment, and which is modeled as a collection of independent oscillators labelled by site index and mode index
| (2) |
The phonon creation and annihilation operators and entering Eq. (2) satisfy Bose commutation relations. The aggregate is driven by weak radiation and the generation of excitations is described in the dipole and rotating-wave approximations
| (3) |
In Eq. (3), operators are the positive- and negative-frequency part of the (time-independent) operator of the (transversal) electric field, while the part of the dipole-moment operator reads as
| (4) |
We assume that transition dipole moment of chromophore does not depend on nuclear coordinates (Condon approximation). The interaction of photoinduced excitations with the environment is taken to be in Holstein form, i.e., it is local and linear in oscillator displacements
| (5) |
We assume there are two possible channels through which photogenerated excitons may decay. The first one is their transfer to the charge-separated state in the RC, in which case they are usefully harvested. On the other hand, exciton recombination, either radiative or nonradiative, is detrimental to the efficiency of EET. While our description of exciton photogeneration and the subsequent phonon-induced relaxation is exact, see Sec. III and Ref. Janković and Mančal, 2020, the description of exciton trapping by the RC and exciton recombination is only effective and relies on the results of more elaborate treatments performed in Refs. Kreisbeck et al., 2011; Shatokhin et al., 2018. There, it is realized that EET from one chromophore to another, as well as the radiative decay to the ground state, are actually mediated by the bath of environmental photons. Performing a second-order treatment of the appropriate interaction Hamiltonians, one ends up with effective Liouville superoperators and that describe respectively the excitation trapping and recombination on the level of reduced excitonic dynamics. In the following sections, we provide more details on the form of these effective Liouvillians and the manner in which they enter our description.
III Methods
We use our exact description of weak-light-induced exciton dynamics in molecular aggregates, which is developed in the accompanying paper. Janković and Mančal (2020) The radiation correlation function, which is the only property of the radiation entering our reduced description, is modeled by the following expression Loudon (2000)
| (6) |
where , , and are the intensity, central frequency, and coherence time of the radiation, respectively. The radiation is assumed to have well defined directions of propagation and polarization. The weak-light assumption underlying our theoretical approach is well satisfied in a wide variety of photosynthetically relevant situations. For example, for a bacteriochlorophyll molecule irradiated by ambient sunlight, the excitation–light interaction may be estimated to be of the order of , see the accompanying paper Janković and Mančal (2020) and Ref. Chan et al., 2018. This energy scale is much smaller than the typical energy scales () of resonance couplings or the excitation–environment coupling. Also, the number of photons per unit time incident on a single photosynthetic complex under sunlight at the surface of the Earth can be estimated to be of the order of , see the accompanying paper Janković and Mančal (2020) and Ref. Chuang and Brumer, 2020. In other words, the corresponding time scale is orders of magnitude longer than time scales typical for excitation transport, trapping at the load, and recombination.
For the sake of simplicity, we assume that the baths on both sites are identical, but uncorrelated. The bath correlation function, which is the only property of the bath entering the reduced description, can be decomposed into the optimized exponential series Zhang et al. (2018) ()
| (7) |
In Eq. (7), the collective bath coordinate is defined in Eq. (5), the expansion coefficients may be complex, while the corresponding decay rates , as well as the white-noise-residue strength , are assumed to be real and positive. The bath correlation function is usually expressed in terms of the environmental spectral density [, where is the temperature]
| (8) |
which conveniently combines information on the density of environmental-mode states and the respective coupling strengths to electronic excitations. May and Kühn (2011); Valkunas, Abramavicius, and Mančal (2013) We explicitly treat only terms in Eq. (7), where and are the numbers of explicitly treated poles of the Bose–Einstein function and the bath spectral density, respectively. We assume the environmental spectral density of the overdamped Brownian oscillator
| (9) |
where is the reorganization energy, while is the characteristic time scale for the decay of the bath correlation function .
The exponential decompositions embodied in Eqs. (6) and (7) enable us to formulate the problem as HEOM incorporating photoexcitation. As demonstrated in Ref. Janković and Mančal, 2020, the hierarchy consists of two parts, one in the sector, and another in the sector. Each density matrix is uniquely characterized by vector of non-negative integers , where index enumerates chromophores, while index counts terms in the decomposition of [Eq. (7)]. In order to describe excitation harvesting by the RC and recombination, we augment our formalism by effective Liouvillians describing these two processes. As demonstrated in Ref. Kreisbeck et al., 2011, these Liouvillians appear on each level of HEOM. Performing the appropriate rescalings, which ensure that auxiliary density operators are all dimensionless and consistently smaller in deeper levels of the hierarchy, Shi et al. (2009) we obtain the following equations describing the NESS we are interested in ()
| (10) |
| (11) |
In the NESS, the continuity equation for exciton currents should be valid, i.e., the number of generated excitons per unit time must balance the sum of the recombination and trapping exciton fluxes. This is physically clear, and it is seen from a more formal perspective by taking the trace (with respect to the electronic system of interest) of Eq. (11) in which . This results in
| (12) |
In the continuity equation [Eq. (12)], we define all currents to be positive, while the sign is determined by the ”direction” of the current ( if it leads to an increase/a decrease in the exciton number). In more detail, the definitions of currents, which are dimensionless in our description, are
| (13) |
| (14) |
| (15) |
Let us immediately note that currents and are written in a basis-invariant manner. This feature is quite appealing, since one can express currents in terms of populations and coherences in any particular basis. The light-harvesting efficiency is defined as
| (16) |
As discussed in the accompanying paper Janković and Mančal (2020) and in Sec. VI, for realistic values of the light coherence time , the expression for the generation current may be further simplified to
| (17) |
In other words, possible enhancements in due to coherences in any basis ultimately originate from the expression for the trapping Liouvillian , as detailed in the following section.
IV Different Bases
In the literature on the physics of photosynthetic light harvesting, two bases play special roles. The first one is basis of singly excited states localized on single chromophores (local or site basis). While Hamiltonian parameters are usually known in the local basis, the description of, e.g., absorption properties of a photosynthetic aggregate is usually performed in the excitonic basis , i.e., in the basis of stationary states of the isolated-aggregate Hamiltonian . Claims about possible impact of coherences on the efficiency of photosynthetic light harvesting are usually made with the coherences in the excitonic or local basis in mind.
Tomasi and Kassal have recently classified different types of possible coherent enhancements of light harvesting efficiency according to the basis in which the excitation decay mechanisms are defined. Tomasi and Kassal (2020) Let us now focus on the trapping at the RC. Two forms for the Liouvillian are widely used in the literature. Jesenko and Žnidarič (2013); León-Montiel, Kassal, and Torres (2014); Tscherbul and Brumer (2018); Yang and Cao (2020); Jung and Brumer (2020) If site is closest to the RC, so that it is essentially the sole site coupled to it, one uses the so-called localized-trapping Liouvillian Jesenko and Žnidarič (2013); León-Montiel, Kassal, and Torres (2014); Tscherbul and Brumer (2018)
| (18) |
where is the characteristic time scale on which the populations are delivered from site to the RC. However, even in such a situation, the excitation transfer to the RC should be regarded as the multichromophoric Förster transfer, Jang, Newton, and Silbey (2004); Ma and Cao (2015) so that it is more appropriate to employ the so-called delocalized-trapping Liouvillian León-Montiel, Kassal, and Torres (2014); Tscherbul and Brumer (2018)
| (19) |
The recombination occurs due to both radiative and nonradiative processes. In other words, the lifetime of chromophores’ excited states is determined not only by the fluorescent decay, but to a great extent also by the conversion of the singlet states to triplets. While the radiative recombination is most naturally described in the excitonic basis, the nonradiative processes likely occur in a different basis of states, depending on how the triplet states are coupled among themselves. Overall, it is not possible to say that recombination occurs straightforwardly in neither the delocalized, nor local basis. For definiteness, we assume that the (nonradiative) recombination may occur from each site with the same rate constant , and the appropriate Liouvillian reads as
| (20) |
The fact that we have chosen the local version of the recombination should not, however, influence our results, because the recombination process is generally much slower than every other process, it occurs on the time scale of nanoseconds, i.e., several orders of magnitude slower than trapping and other processes, see Sec. V. In situations in which the radiative decay is the major loss channel, it may be more appropriate to consider the recombination Liouvillian in the excitonic basis
| (21) |
where is the Weisskopf–Wigner spontaneous emission rate Chan et al. (2018) from excitonic state .
If we assume the trapping at the RC to be governed by Eq. (18), the trapping current in Eq. (14) depends only on site populations, and on both exciton populations and interexciton coherences. If, on the other hand, we assume that the trapping is governed by Eq. (19), is expressed in terms of exciton populations only, while its expression in the local basis contains both site populations and intersite coherences. This has been recognized in recent studies, which ascertain that possible coherent enhancements of the efficiency can be achieved only when the coherence occurs in a basis different from that in which the trapping or recombination are modeled. Tscherbul and Brumer (2018); Tomasi and Kassal (2020)
However, we feel that the notion of coherent efficiency enhancements is not defined well enough. The word ”enhancement” would suggest that there is a reference value of the efficiency (which should be smaller than unity), with respect to which we can expect to achieve an enhancement. Moreover, since the enhancement is supposed to be coherent, one could imagine that the aforementioned reference value should depend on populations only, so that the subsequent inclusion of coherences should enhance the efficiency above that reference value. Whatever the form of the effective trapping and recombination Liouvillians is, there will always be a basis in which is entirely expressed in terms of basis-state populations only. Such a basis will be denoted as and termed the preferred basis of the NESS under investigation. The trapping current is then expressed only in terms of the RDM diagonal elements, so that the efficiency value calculated in the preferred basis could be regarded as the reference value above which coherent enhancements due to non-zero values of coherences in some other basis (e.g., in the local or excitonic basis) may be possible. However, our definition of the efficiency [Eq. (16)] is basis-independent, and the fact that the coherences in the excitonic or local basis are non-zero should not be expected to bring about any efficiency enhancements.
While the above discussion about coherent efficiency enhancements is quite general, it will not be the main topic of our numerical investigations, which focus on a model photosynthetic dimer and in which the efficiency is close to unity, see Sec. V.1. Our central question is how the stationary state of the incoherently driven and loaded molecular system looks like, and how it relates to the two standard pictures, the picture of local states and the delocalized-states picture. Nevertheless, to the best of our knowledge, our work is among the first works that properly describe the principal physical features of excitation harvesting under incoherent light and properly define the light-harvesting efficiency. Chuang and Brumer (2020); Yang and Cao (2020); Jung and Brumer (2020) The arguments of the previous paragraph suggest that, once a proper description of the state in which the photosynthetic systems under incoherent illumination and load find themselves, the involvement of the coherences in the description of photosynthetic light harvesting should be critically reassessed. We thus believe that future applications of our NESS methodology to larger systems, see Sec. VI, could significantly contribute to the debate on possible coherent efficiency enhancements.
The excited-state sector of the steady-state RDM in the preferred basis reads as
| (22) |
The preferred basis is determined by the competition between excitation generation, pure dephasing, energy relaxation, excitation trapping at the RC and recombination.
The excitonic (or site) basis and the preferred basis of the NESS are connected through a unitary transformation. For our model dimer, the most general transformation of that kind can be parameterized by four real parameters, so that the basis vectors in the preferred basis are expressed in terms of the basis vectors in the excitonic basis as follows Murnaghan (1962)
| (23) |
Due to the phase freedom, we can immediately remove parameters and from further discussion, so that we are left with only two parameters, and . From our subsequent discussion, it will emerge that the rotation angle is closely related to the analogous rotation angle which measures the deviation from the non-canonical statistics in the undriven and unloaded system (no generation, trapping, and recombination). Lee, Cao, and Gong (2012) The phase is intimately connected to the rates of excitation trapping and recombination, which remove excitations from the system.
The parameters and can be related to the Bloch angles and that are commonly used to characterize the basis in which the RDM is diagonal. Lee, Cao, and Gong (2012); Cerrillo and Cao (2014) Namely, one can always normalize to obtain whose trace is unity and whose eigenvalues will be denoted as . For the model dimer, the operator can always be expressed as
| (24) |
where is the unity matrix, while are three Pauli matrices. Let be diagonal in the excitonic basis (i.e., ) and let be the corresponding vector in Eq. (24). Then it can be shown that the spherical angles and on the Bloch sphere are related to parameters and as follows
| (25) |
| (26) |
Equations (25) and (26) relate and to the Bloch angles and that are straightforwardly obtained from vector . In a similar manner, one obtains parameters and of the unitary transformation that connects the preferred and the local basis. The rotation angle can always be chosen in the range , while . This is discussed in greater detail in Sec. SI of the Supplementary Material.
V Numerical Results
V.1 Model Parameterization and Numerical Implementation
Numerical computations are performed on an asymmetric dimer, which is schematically presented in Fig. 1. The parameters of our model are summarized in Table 1.
| (cm-1) | 100 |
|---|---|
| (cm-1) | 100 |
| (fs) | 100 |
| (K) | 300 |
| (fs) | 1.3 |
| (ns) | 1.0 |
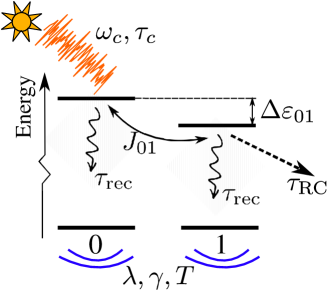
In brief, we selectively and resonantly () excite site 0 by weak-intensity radiation whose coherence time assumes the value representative of the natural Sunlight. Kano and Wolf (1962); Mehta (1963) Such a selective excitation of one site was also a feature of previous studies on model dimers. Jesenko and Žnidarič (2013); Tscherbul and Brumer (2018) As mentioned in Sec. III, the propagation direction and the polarization vector of the radiation are assumed to be well defined. While the transition dipole moment of site 1 is assumed to be orthogonal to the polarization vector, the magnitude of the projection of the transition dipole moment of site 0 onto the polarization vector will be further denoted as . While we assume the selective excitation of site 0, the excitation trapping at the load is modeled by Eq. (18) or Eq. (19) in which we assume that the load is coupled only to site 1, i.e., in Eqs. (18) and (19). Such an assumption is motivated by our wish to include the spatial transfer of excitations within our dimer model. The difference between the local energy levels, resonance coupling , and bath relaxation time assume values typical of the FMO complex. Jang and Mennucci (2018); Moix et al. (2011) The value of the recombination time constant is chosen on the basis of the measured exciton lifetime in the FMO complex, Zhou et al. (1994); Magdaong et al. (2017) and is similar to the value used in previous theoretical studies. Tscherbul and Brumer (2018); Plenio and Huelga (2008); León-Montiel, Kassal, and Torres (2014); Jesenko and Žnidarič (2013) Let us note that the recombination time scale is significantly longer than all other time scales in the problem. Also, in our model dimer, it is quite unlikely that an excitation would be prevented from reaching the RC during its lifetime. Therefore, the recombination is not probable, the precise value of is not important, and the light-harvesting efficiency will always be close to 1 in the model dimer. Jesenko and Žnidarič (2013); Yang and Cao (2020) The recombination is explicitly treated in order to formulate the continuity equation [Eq. (12)], and the efficiency is not of primary interest in this work, which will be focused on other relevant properties of the NESS. Within our simplified model, there is a certain level of arbitrariness in the choice of the appropriate value of . Namely, a more elaborate treatment of excitation harvesting should explicitly consider both the forward and backward excitation transfer from the absorbing aggregate to the state of primary electron donor in the RC, as well as the primary charge separation, after which the excitation may be considered as usefully harvested. Blankenship (2014); Damjanović, Ritz, and Schulten (2000); Caycedo-Soler et al. (2017) Previous theoretical works employing a simplified description of excitation harvesting, as we do here, typically assumed that is of the order of picoseconds. Plenio and Huelga (2008); Moix et al. (2011) On the other hand, experiments on various species of photosynthetic bacteria, as well as computational studies, suggest that the appropriate value of our parameter may be as large as a couple of tens of picoseconds. Timpmann, Freiberg, and Sundström (1995); Damjanović, Ritz, and Schulten (2000); Strümpfer and Schulten (2012); Adolphs and Renger (2006) The reported values of the reorganization energy in the FMO complex range from tens to hundreds of inverse centimeters. Adolphs and Renger (2006); Wang et al. (2015); Kim, Choi, and Rhee (2018) Having all these things considered, the values of and will be varied in wide, yet physically relevant ranges, in order to examine how they impact the properties of the NESS.
The NESS is obtained by solving coupled Eqs. (10) and (11) by adapting the algorithm that was introduced in Ref. Zhang et al., 2017. The computational approach of Ref. Zhang et al., 2017 was developed to compute the equilibrium RDM of an undriven and unloaded system and it relies on the Jacobi iterative procedure to solve a diagonally dominant system of linear algebraic equations. The procedure is repeated until a convergence criterion, which in Ref. Zhang et al., 2017 was related to the magnitude of the ADO elements, is satisfied. Here, we deal with a driven and loaded system, and our computations are terminated once the continuity equation [Eq. (12)] is satisfied with a desired numerical accuracy. More details on our numerical scheme to compute the NESS of a driven and loaded dimer can be found in Sec. SII of the Supplemental Material.
V.2 Results: Long Trapping Times
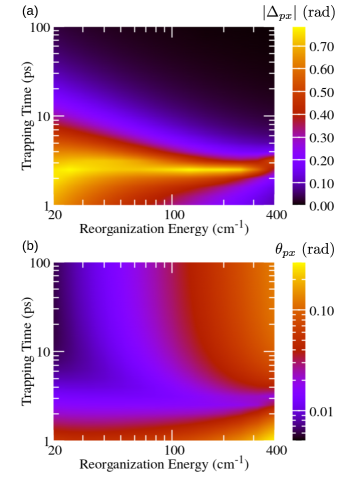
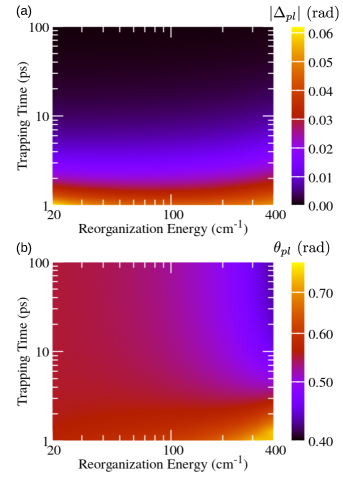
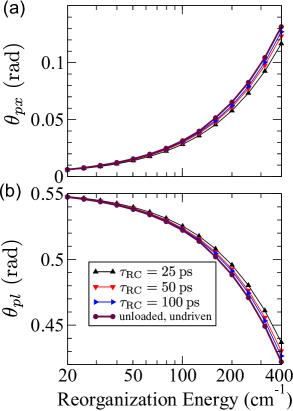
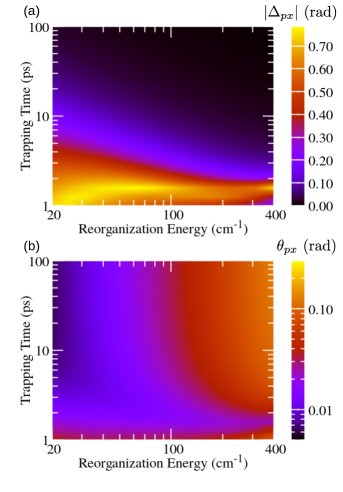
Figure 2 (Fig. 3) summarizes the dependence of the parameters of the unitary transformation between the preferred and excitonic (local) basis on the reorganization energy and the trapping time at the RC. The trapping is assumed to be governed by the localized Liouvillian, see Eq. (18), while the recombination is described by the Liouvillian in Eq. (20). When the trapping at the RC is so slow that is (much) longer than characteristic time scales for excitation dephasing and energy relaxation, phases and tend to zero, see Figs. 2(a) and 3(a) for ps. These time scales are still much shorter than those relevant for recombination. Therefore, the obtained NESS is expected to be quite similar to the equilibrium state of an undriven and unloaded aggregate. Olšina et al. (2014) To confirm this expectation, in Figs. 4(a) and 4(b) we plot angles and as functions of the reorganization energy for different values of . We conclude that, as is increased, angles and tend to the values specific of the thermal equilibrium of undriven and unloaded dimer (in which we may formally identify ). For small reorganization energies, angle tends to zero, see Fig. 4(a), and the preferred basis is close to the excitonic basis. At the same time, the limiting value reached by as the reorganization energy is decreased, see Fig. 4(b), corresponds to the angle of the rotation by which the excitonic basis is transformed into the local basis (the mixing angle is given as ). As the reorganization energy is increased, the preferred basis continuously changes from the excitonic basis [in which is diagonal, see Eq. (1)] towards the local basis [in which is diagonal, see Eq. (5)]. Lee, Cao, and Gong (2012) Therefore, the magnitude of increases, see Fig. 4(a), while the magnitude of decreases, see Fig. 4(b), with increasing reorganization energy.
V.3 Results: Short Trapping Times. Relation between Stationary and Time-Dependent Pictures
On the other hand, when the trapping at the RC is faster, phases and increase in magnitude, see Figs. 2(a) and 3(a), while the values of angles and start to deviate from the respective values in the thermal equilibrium, see Figs. 2(b) and 3(b). These deviations are more pronounced as the trapping time is decreased and the reorganization energy is increased, see the lower right corners of Figs. 2(b) and 3(b). At the same time, the magnitude of phase is large in the region of fast trapping and relatively small reorganization energy, see the lower left corner of Fig. 2(a), while the increase that displays as the trapping time is reduced is virtually the same for all considered values of reorganization energy, see Fig. 3(a). It was suggested that the trapping time practically determines the temporal frame in which the intrinsic dimer’s dynamics is interrogated. Olšina et al. (2014) It is therefore interesting to examine if the dependence of the transformation parameters between the excitonic (or site) and the preferred basis of the NESS on the trapping time (vertical cuts in Figs. 2 and 3) can be somehow recovered from the dynamics of the unloaded dimer initiated by suddenly turned-on incoherent light.
To gain some intuition on the relation between the stationary and time-dependent pictures, let us consider the differential equation
| (27) |
This equation models the system, characterized by quantity , that is subjected to continuous pumping (source term ) and continuous decay (decay rate ). It resembles the equation for the total excited-state population that may be obtained by taking the trace of the temporal counterpart of Eq. (11) for (on the level of the RDM). The stationary point of Eq. (27) is . If one considers the driven system in the absence of decay channels, its dynamics is governed by (the superscript ”ul” specifies that the system is unloaded). To solve the last equation, we assume that the driving is suddenly turned on at instant , and that the initial condition is (which would correspond to the initially unexcited system). One immediately realizes that , i.e., the steady-state solution under constant driving and decay can be obtained from the temporal evolution of the driven system without decay channels at instant corresponding to the characteristic decay time.
In the following, we develop the above-described simple argument in the situation of our interest. While the argument is quite formal, some of its parts will be specifically developed for the case of our model dimer, and in Sec. VI we discuss its validity in multichromophoric situations.
Let us first note that there is a hierarchy of temporal scales characteristic for the dimer’s dynamics under weak incoherent light. The shortest time scales (roughly speaking, tens to hundreds of femtoseconds) stem from the intrinsic dimer’s dynamics. The time scale of the trapping at the load is of the order of 1–10 ps in the photosynthetically relevant range of parameters. The longest time scale characterizes the excitation loss by recombination, and it is of the order of nanoseconds. The assumption of weak light actually means that the radiation is so weak that the number of incident photons per unit time is very small and the corresponding time scale is the longest time scale in the problem (we may loosely say that the light intensity is so low that the absorption of incident radiation is the rate-limiting process).
We now concentrate on the NESS Eqs. (10) and (11). Within our second-order treatment of the light-matter interaction, one can first solve Eq. (10) and obtain the steady-state in the sector, , and then use this solution to compute the source-term in the sector [the third term on the RHS of Eq. (11)]. Given the known source term in the sector, which will be denoted as , Eq. (11) can be recast as
| (28) |
where is the HEOM-space representation of the RDM and ADMs, is the HEOM-space representation of the hierarchical links between DMs [it comprises terms 1, 2, 5–7 on the RHS of Eq. (11)], while is the HEOM-space representation of the recombination and trapping at the load [term 4 on the RHS of Eq. (11)]. Eq. (28) is solved by
| (29) |
While the matrix is off-diagonal in the HEOM space and non-symmetric, the matrix is diagonal in the HEOM space. Whatever the form of the trapping and recombination Liouvillians is, all the matrix elements of are of the same magnitude, (due to the above-mentioned hierarchy of dimer’s temporal scales, the recombination rate, , is completely irrelevant). Moreover, the eigenvalues of matrix , which represent the rates of the internal dimer’s dynamics, are much larger than . Therefore, computing the inverse , we can regard as a small isotropic correction to . In the lowest-order approximation, the spectral decomposition of can be formulated as
| (30) |
where and are respectively the eigenvalues, right, and left eigenvectors of matrix , while are the elements of (they are all approximately the same).
Let us now focus on the temporal counterparts of Eqs. (10) and (11) in which the trapping and recombination Liouvillians are omitted. Within our second-order treatment of the light-matter interaction, one can first solve the dynamics in the sector [Eq. (10)], and then use this solution as a known time-dependent source term in Eq. (11), which describes the sector we are primarily interested in. However, as advocated in the accompanying paper, Janković and Mančal (2020) for realistic values of the light coherence time (of the order of 1 fs for natural sunlight), this source term is approximately time-independent (see also the expression for the generation current in the limit of short coherence time of light) and thus equal to introduced in the above discussion. The temporal counterpart of Eq. (11) without trapping and recombination then reads as
| (31) |
Assuming that the light is abruptly turned on at and that , the solution is
| (32) |
In the spectral representation of , the solution reads as
| (33) |
It is known from the literature Dunn, Tempelaar, and Reichman (2019) that, for the spin–boson model whose coupling to the environment has the overdamped Brownian oscillator spectral density [Eq. (9)], at least one of the eigenvalues of matrix is equal to zero, while non-zero eigenvalues appear in complex conjugate pairs and have positive real parts. Assuming that , the time-dependent solution for a driven but unloaded system [Eq. (32)] can be recast as
| (34) |
The same observations enable us to recast the spectral form of the NESS solution under driving and load as
| (35) |
Remembering that , and that is sufficiently smaller than 1 for all , we can approximate in Eq. (34) and in Eq. (35) to finally obtain that
| (36) |
We have just demonstrated that that the dimer’s NESS can be reconstructed from the time evolution of the initially unexcited, driven, but unloaded dimer at instant after a sudden turn-on of the driving. This result establishes an interesting relationship between the stationary (with load) and time-dependent (without load) pictures under incoherent driving.
While the physical relevance of the sudden turn-on of incoherent light may be questionable, see Ref. Brumer, 2018 and references therein, this formal argument demonstrates that there is a formal connection between this seemingly unphysical setting and the NESS picture. However unphysical the sudden turn-on may be, the final-value theorem from the theory of Laplace transforms ensures that Eq. (32), in which the load is added, i.e., , can be used to obtain the NESS in Eq. (29) by temporal propagation up to sufficiently long time .
In the following, we concentrate on a numerical demonstration of this relationship. First, we propagate the temporal counterparts of Eqs. (10) and (11), in which the trapping and recombination Liouvillians are omitted, see Figs. 5(a1)–5(d2). The RDM elements [in Figs. 5(a1)–5(d2), in the excitonic basis] are measured in units of , and not in absolute units, as is customarily done. Our reason for choosing this unit lies in our perturbative treatment of the interaction with light, which ensures that singly excited-state populations and intraband coherences are proportional to the light intensity , see Eq. (6), and to the excited-state oscillator strength , see the caption of Fig. 1. Analyzing Eqs. (10) and (11), one can readily conclude that is the natural unit in which to measure populations and coherences. The absolute value of this unit may be estimated by using the estimate for the magnitude of the excitation–light interaction given in Sec. III, so that for the value of given in Table 1 we obtain . Second, we use the RDM at to determine the transformation parameters and by virtue of Eqs. (24)–(26). The results emerging from these real-time computations are confronted with the results emerging from NESS computations in Figs. 5(a3)–5(d4) for a couple of values of reorganization energy. It is observed that the two methods predict quite similar values of transformation parameters and for all the examined values of the reorganization energy and trapping time. This result, together with the RDM dynamics initiated by a sudden turn-on of incoherent radiation, can help us better understand the dependence of and on for . The discontinuous change from to that phase undergoes at around [see the bright area in Fig. 2(a)] should be attributed to the fact that the real part of interexciton coherence in an incoherently driven, but unloaded dimer, becomes equal to zero at around , see solid curves in Figs. 5(a2)–5(d2). The imaginary part of the interexciton coherence saturates somewhat earlier, see dashed curves in Figs. 5(a2)–5(d2). As is further increased, decreases because the real part of the interexciton coherence is increasing, see also Eq. (26).
The relation between the NESS and RDM dynamics in real time leans on the above-mentioned hierarchy of time scales of the dimer’s dynamics, i.e., on the fact that the trapping time scale is long enough. Namely, in time traces of a driven and unloaded dimer for , one observes that the behavior of both exciton populations [Figs. 5(a1)–(d1)] and interexciton coherences [Figs. 5(a2)–(d2)] displays certain steadiness. In other words, populations, as well as the real part of the interexciton coherence, linearly increase in time, while the imaginary part of the interexciton coherence reaches a constant value, see also the accompanying paper. Janković and Mančal (2020) When the trapping time constant is , so that at the steadiness has already been established, the dynamical quantities of a driven, but unloaded dimer, may be used to quite accurately reconstruct the NESS. On the other hand, when , so that the steadiness has not been established yet, the reconstruction of the NESS from the quantities of a driven and unloaded dimer computed at would be less accurate. This is particularly clear for low values of the reorganization energy, see Figs. 5(a3) and 5(a4), when oscillations in the interexciton coherence are damped on a time scale of , see Fig. 5(a2). A similar situation can be expected for slow bath, when the bath correlation time is long enough. Ishizaki and Fleming (2009b) In such cases, the reconstruction of NESS from the dynamics of the incoherently driven and unloaded dimer is not accurate because is comparable to the time scales of the intrinsic dimer’s dynamics. At this point, it is useful to remember that the information extracted from ultrafast spectroscopic signals can be used to determine the Hamiltonian parameters of the system under consideration, i.e., to determine the rate constants and the frequencies of the oscillatory features of the intrinsic dimer’s dynamics that enter Eqs. (30) and (32)–(36). Brumer (2018); Mančal (2020); Mančal and Valkunas (2010); Chenu, Malý, and Mančal (2014); Scholes (2018) The dynamics of a driven, but unloaded, system, in which the driving is abruptly turned on at , see Eq. (32) and Figs. 5(a1)–5(d2), can be formally regarded as the interference of all possible outcomes of ultrafast experiments in which the delta-like excitation is centered at instant and which freely evolve for the time interval of length . The initial condition is set by the ratios (the initial condition has to be dimensionless!) of the components of the generation vector , which can be shown to be basically proportional to the square of the transition dipole moments and dependent on their mutual alignments, see Sec. VI. The oscillatory features stemming from the abruptly turned-on incoherent light in Figs. 5(a2) and 5(b2) are thus tightly connected to the oscillatory features characteristic for the excitation by very short pulses. The time scales on which the oscillatory features can be observed thus set the lower limit on for which the above-described relationship between the stationary and dynamic pictures is valid. Therefore, the decay time of dynamical coherences observed in spectroscopies may still be relevant in the natural setting, although the dynamical coherences themselves are absent in the NESS. Pelzer et al. (2014) In Sec. SIII of the Supplementary Material, we estimate the time scales characteristic of exciton decoherence by suitable fitting procedures. For the values of model parameters adopted in this work, we find that the characteristic decay times of exciton coherence are shorter than resonable values of .
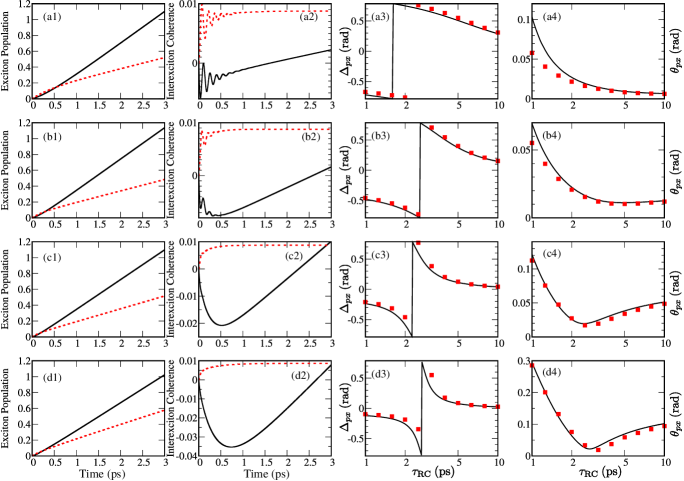
The previous discussion was conducted for interexciton coherences. Similar conclusions can be also reached in the site basis, see Fig. 3. While we have already discussed the limit of long trapping time in Fig. 4(b), the case of relatively short is analyzed in greater detail in Fig. S2 of the Supplementary Material. The analysis is completely analogous to that accompanying Figs. 5(a1)–5(d4).
The choice of instant at which time-dependent quantities are extracted to obtain the properties of the NESS is somewhat arbitrary because is not really the time, but the characteristic time scale of the trapping. This is also apparent from our formal demonstration of the relation between the stationary and time-dependent pictures, in which we only used the fact that all are of the order of , while their precise values were not important. Moreover, in the results presented so far, we used the trapping [Eq. (18)] and recombination [Eq. (20)] Liouvillians that are diagonal in the local basis. It is, therefore, not obvious if and how the above-discussed relation between the dynamic and stationary pictures under incoherent driving changes when the trapping or recombination Liouvillian that is diagonal in the excitonic basis, Eqs. (19) and (21), is employed. In Figs. 6(a) and 6(b), which are analogous to Figs. 2(a) and 2(b), respectively, we examine the dependence of the transformation parameters and on and under the assumption of delocalized trapping, while we retain the recombination Liouvillian in Eq. (20). The main features of Figs. 2(a) and 2(b) are clearly recognizable in Figs. 6(a) and 6(b). This is particularly true at long trapping times. However, at short trapping times, the maximum that reaches in Fig. 2(a) at is shifted towards in Fig. 6(a). A similar discussion applies to Fig. 6(b), where the decrease that exhibits as is increased from 1 ps is shifted to shorter trapping times with respect to Fig. 2(b). We believe that the maximum in , which occurs at for delocalized trapping, should still be interpreted to originate from the fact that the real part of the interexction coherence in the driven and unloaded dimer changes its sign on a picosecond time scale. If the real part of the interexciton coherence is equal to zero at instant , it is not guaranteed that the magnitude of (computed from the NESS) reaches it maximal value of exactly at [this is also observed in Figs. 5(a3)–5(d3)]. Our point here is that the magnitude of reaches at irrespective of the precise form of the trapping Liouvillian.
V.4 Further Results
In the following, we discuss how the variations in the electronic parameters of the model, in particular in the difference between local energy levels, affect the properties of the NESS. We fix the reorganization energy to 150 cm-1.
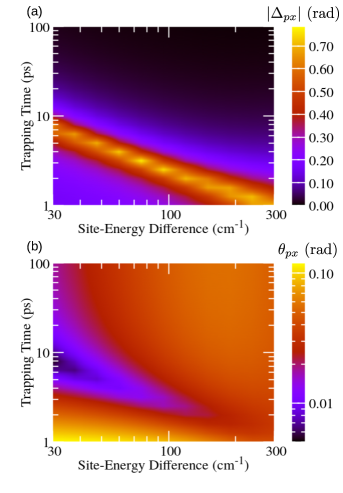
Figures 7(a) and 7(b) present the dependence of transformation parameters and on and . We varied from 30 to 300 cm-1 on the basis of the literature values of site-energy differences in the FMO complex. Adolphs and Renger (2006); Jang and Mennucci (2018) Let us first focus on the long trapping times, when the magnitude of the phase is small, see the upper half of Fig. 7(a), and the NESS obtained is quite similar to the excited-state equilibrium. For small values of , for which , exciton delocalization prevails over the localizing effect of the environment, which is reflected in relatively small values of the rotation angle , see the upper left part of Fig. 7(b). As the local energy levels become more off-resonant, the environment-induced localization becomes more pronounced than exciton delocalization, so that the rotation angle from the excitonic basis to the preferred basis of the NESS increases. However, when is large enough, so that , the excitonic basis is already localized enough and, for the chosen value of , the localizing effect of the environment is effectively suppressed. This leads to a decrease in the rotation angle . As the trapping time is shortened, the deviations from the above-established picture become more pronounced. The magnitude of reaches its maximum at depending on the particular value of , see Fig. 7(a), while angle exhibits a minimum in the very same region of the space, see Fig. 7(b).
VI Possible Applications to Multichromophoric Aggregates
So far, we have employed our novel theoretical approach to study in great detail the NESS of an incoherently driven and loaded dimer. The dimer represents the only model, in which one can represent the character of the NESS by only two parameters—the angle of the basis rotation with respect to a chosen basis, and the phase that is closely connected with the excitation decay rates—and the difference between different NESSs is easy to understand. In other words, the dimer is the only model, where relatively simple “understanding” of the role of different parameters in establishing the preferred basis can be derived. Whether our results can be translated to larger systems is, of course, an important question. This section aims at presenting the basic steps that have to be taken in order to apply our NESS formalism to multichromophoric situations.
We start from the fact that the realistic coherence time of light is much shorter than any other time scale in the problem. All our results for the dimer are obtained by solving Eqs. (10) and (11) and do not lean on any assumption about the coherence time entering Eq. (6). In the accompanying paper, Janković and Mančal (2020) we have argued that, in the limit of small , the first-order light correlation function defined in Eq. (6) may be replaced by
| (37) |
which represents the so-called white-noise model of the radiation. Olšina et al. (2014) Equation (10) is then omitted from further discussion, while the source term (the third term) of Eq. (11) reads as Janković and Mančal (2020)
| (38) |
We recall that the operator is the projection of the dipole-moment operator onto the radiation polarization vector . Using the definition of in Eq. (4), the matrix elements of the source term in the basis of the single-excitation manifold can be expressed as
| (39) |
The basis is in principle arbitrary; it can be the local basis (), the excitonic basis (), the preferred basis (), or any other basis in the single-excitation manifold. Equation (39) is in the form in which the rotational average can be straightforwardly performed with the final result Hein et al. (2012)
| (40) |
where is the transition dipole moment of state . In a typical situation, the system of interest consists of many photosynthetic complexes in solution, and the rotational average is performed over random orientation of individual chromophores’ dipole moments with respect to the polarization direction. The source term in Eq. (40) depends only on relative orientations of transition dipole moments, which are known, e.g., for the widely investigated -band excitations of the FMO complex. Read et al. (2008) Representing Eq. (11) in basis and using the rotationally averaged source term given in Eq. (40), we obtain a description of incoherent-light driven EET that exploits both the light incoherence (short ) and experimentally available data on relative orientations of transition dipole moments. Such a description features a much more realistic excitation condition than the one we have employed in the study of model dimer (the selective excitation of a local site).
Our NESS approach can also be used to follow the pathways of light-induced excitations, from the point of their generation, through the chromophore network, to the point of their extraction at the load. To elaborate on this, we compute the matrix element of Eq. (11) for the RDM () and obtain
| (41) |
In Eq. (41), is the excitation generation flux into the singly excited state
| (42) |
is the excitation trapping flux from
| (43) |
is the excitation recombination flux from
| (44) |
while (for ) is the net flux of excitations that are exchanged between states and
| (45) |
The last term in Eq. (41), , stems from the residual term in the decomposition of the bath correlation function into the optimized exponential series, see Eq. (7). This term can, therefore, be made arbitrarily small by explicitly treating a sufficient number of exponentially decaying terms in Eq. (7), and will not be considered in the further discussion. For the sake of completeness, let us also note that, if the light incoherence is exploited on the level of Eqs. (37)–(40), Eq. (42) for the generation flux should be replaced by
| (46) |
Similarly to Eq. (12), all the fluxes entering Eq. (41) are dimensionless and the sign in front of them is determined by the ”direction” of the flux ( if it leads to an increase/a decrease in the population of state ). One can prove that the (global) continuity equation [Eq. (12)] is obtained by adding Eq. (41) for different states . Therefore, Eq. (41) can be regarded as the local continuity equation in the basis . Here, the term ”local” is in no manner connected to the local basis because Eq. (41) is formulated in an arbitrary basis . The local continuity equation establishes the balance (on the level of a single-excitation state) between the excitation generation, trapping, and recombination on the one hand and the excitation flow from the considered state toward other states on the other hand.
We believe that the local continuity equation is a potentially interesting feature of our NESS picture because it enables us to track the steady-state excitation pathways. The excitation flux satisfies , it is positive when the net excitation flow is directed from to , while it is negative when the net excitation flow is directed from to . One may, therefore, identify the states in which the generation, trapping, and recombination predominantly occur, and then individuate the pathways along which the excitations travel. Such a discussion can be performed in an arbitrary basis of singly excited states, enabling one to follow the excitation pathways in, e.g., the local, excitonic, or preferred basis.
We conclude this section by discussing the generality of the relationship between the stationary and time-dependent pictures that we established for the model dimer in Sec. V.3. This relationship relies on the hierarchy of time scales of the dimer’s dynamics under incoherent light that is also introduced in Sec. V.3. On the other hand, in a multichromophoric aggregate, the rates of excitation transfer between various states can be of the different orders of magnitude, and the excitation may be trapped in certain states, so that the recombination time scale may also become important. As an example, let us take a single unit of the FMO complex which, despite the fact that its contribution to direct light harvesting is minor, has become a paradigmatic system to discuss quantum effects in biological systems. Pšenčík and Mančal (2017) It is known that the intraunit energy transfer predominantly proceeds on subpicosecond time scales. Thyrhaug et al. (2016) The excitation transfer from the considered unit of the FMO complex to the RC typically occurs on a time scale, while the decay time of the lowest FMO level is around . Dostál, Pšenčík, and Zigmantas (2016) We may thus speculate that the hierarchy of time scales introduced in Sec. V.3 is valid in this example and that the reconstruction of the NESS from the dynamics of the driven, but unloaded system is possible. However, in this multichromophoric example, the value of the efficiency genuinely depends on a complex interplay between the excitation generation, relaxation, dephasing, trapping at the RC and recombination and, as such, it cannot be predicted in advance (as is the case for the dimer model). This is even more pronounced in photosynthetically more relevant situations, in which the excitations initially created in antenna complexes reach the RC after many steps of relatively slow interpigment transfer. Chuang and Brumer (2020) The application of our NESS formalism to such situations is out of the scope of this paper.
VII Discussion and Conclusion
We have provided a detailed and rigorous theoretical description of the light harvesting by a molecular aggregate under conditions that are representative of photosynthetic light harvesting as it occurs in Nature. The picture established in this work takes into account the excitation generation by means of weak incoherent light, their subsequent relaxation and dephasing, as well as excitation trapping by a load (the RC) and recombination. While the generation, relaxation, and dephasing are described in a (numerically) exact manner, which we have reported in the accompanying paper, the excitation trapping and recombination are included on the level of effective Liouvillians.
This piece of research addresses a recurrent question of the possible relevance of quantum coherent effects (understood in a very broad sense) for the natural light harvesting. Our NESS approach provides a physically transparent definition of the light-harvesting efficiency [Eq. (16)] that is basis-invariant, so that we are in position to embark upon the study of possible coherent enhancements of the efficiency. Recent reports have suggested that these coherent enhancements strongly depend on the basis in which the effective trapping and/or recombination Liouvillians are diagonal. Here, we use the fact that the state of an incoherently driven and loaded aggregate is most naturally represented and studied in the so-called preferred basis of the NESS, in which the steady-state RDM is diagonal. This definition of the preferred basis implies that this basis sublimes the joint effect of excitation generation, relaxation, dephasing, trapping, and recombination. The preferred-basis description of the NESS under driving and load can be seen as an analogue of the description of a system of coupled harmonic oscillators in terms of normal modes. While finding the preferred basis is highly nontrivial, as demonstrated throughout this paper, this concept may shed new light on the debate on the role of coherences in the energy transfer under incoherent light.
We have examined the properties of the preferred basis of the NESS of an incoherently driven and loaded dimer by studying the manners in which it is connected to two widely used representations, namely those employing the excitonic and the local bases. The recombination time scale is, in general, significantly longer than any other time scale in the problem, so that, in the limit of long trapping time, the NESS is very similar to the previously studied excited-state equilibrium of an undriven and unloaded system. We also find that the NESS under driving and load carries information that is encoded in the temporal evolution of the unloaded system driven by suddenly turned-on incoherent light. If the radiation is abruptly turned on at , the properties of the NESS which arises due to the excitation trapping with time constant can be quite reliably extracted from the RDM at . We conclude that the trapping time scales for which such a relation between the NESS and the dynamics of the driven but unloaded system is sensible are basically determined by the time scales of decoherence and relaxation, which are accessible in ultrafast spectroscopy experiments. Since realistic trapping times are in general much longer than decoherence and relaxation time scales, the relation we found between the steady-state and time-dependent pictures is quite general.
We again note that our theoretical and computation approach to obtain the NESS under incoherent driving is general and not limited to the model dimer studied here. We opted for the dimer because the relationships between basis vectors of the preferred basis and the excitonic or local basis can be parameterized by only two real parameters, which have certain physical significance and whose dependence on model parameters can be studied in a systematic manner. In the case of a more complex excitonic aggregate, one should resort to more involved parameterizations of unitary matrices.
Supplementary Material
See supplementary material for: (a) the derivation of equations for the transformation parameters and ; (b) detailed numerical procedure to compute the nonequilibrium steady state; (c) analysis of the dynamics initiated by a delta-like photoexcitation; (d) the comparison of transformation parameters and obtained from stationary and time-dependent pictures in the case of fast trapping.
Acknowledgements.
The initial stages of this work were supported by Charles University Research Center program No. UNCE/SCI/010 and by Czech Science Foundation (GAČR) grant No. 18-18022S. The final stages of this work were supported by the Institute of Physics Belgrade, through the grant by the Ministry of Education, Science, and Technological Development of the Republic of Serbia. Computational resources were supplied by the project ”e-Infrastruktura CZ” (e-INFRA LM2018140) provided within the program Projects of Large Research, Development and Innovations Infrastructures. Numerical computations were also performed on the PARADOX supercomputing facility at the Scientific Computing Laboratory of the Institute of Physics Belgrade.Author’s Contributions
T.M. gave the initial impetus for this work, which started during V.J.’s stay in Prague. V.J. developed the methodology, conducted all numerical computations, analyzed their results, and prepared the initial version of the manuscript. Both authors contributed to the submitted version of the manuscript.
Data Availability Statement
The data that support the findings of this study are available from the corresponding authors upon reasonable request.
References
- Engel et al. (2007) G. S. Engel, T. R. Calhoun, E. L. Read, T.-K. Ahn, T. Mančal, Y.-C. Cheng, R. E. Blankenship, and G. R. Fleming, “Evidence for wavelike energy transfer through quantum coherence in photosynthetic systems,” Nature 446, 782–786 (2007).
- Panitchayangkoon et al. (2010) G. Panitchayangkoon, D. Hayes, K. A. Fransted, J. R. Caram, E. Harel, J. Wen, R. E. Blankenship, and G. S. Engel, “Long-lived quantum coherence in photosynthetic complexes at physiological temperature,” Proc. Natl. Acad. Sci. 107, 12766–12770 (2010).
- Cheng and Fleming (2009) Y.-C. Cheng and G. R. Fleming, “Dynamics of light harvesting in photosynthesis,” Annu. Rev. Phys. Chem. 60, 241–262 (2009).
- Ishizaki and Fleming (2011) A. Ishizaki and G. R. Fleming, “On the interpretation of quantum coherent beats observed in two-dimensional electronic spectra of photosynthetic light harvesting complexes,” J. Phys. Chem. B 115, 6227–6233 (2011).
- Brumer and Shapiro (2012) P. Brumer and M. Shapiro, “Molecular response in one-photon absorption via natural thermal light vs. pulsed laser excitation,” Proc. Natl. Acad. Sci. 109, 19575–19578 (2012).
- Brumer (2018) P. Brumer, “Shedding (incoherent) light on quantum effects in light-induced biological processes,” J. Phys. Chem. Lett. 9, 2946–2955 (2018).
- Mančal (2020) T. Mančal, “A decade with quantum coherence: How our past became classical and the future turned quantum,” Chem. Phys. 532, 110663 (2020).
- Cao et al. (2020) J. Cao, R. J. Cogdell, D. F. Coker, H.-G. Duan, J. Hauer, U. Kleinekathöfer, T. L. C. Jansen, T. Mančal, R. J. D. Miller, J. P. Ogilvie, V. I. Prokhorenko, T. Renger, H.-S. Tan, R. Tempelaar, M. Thorwart, E. Thyrhaug, S. Westenhoff, and D. Zigmantas, “Quantum biology revisited,” Sci. Adv. 6, eaaz4888 (2020).
- Mančal and Valkunas (2010) T. Mančal and L. Valkunas, “Exciton dynamics in photosynthetic complexes: excitation by coherent and incoherent light,” New J. Phys. 12, 065044 (2010).
- Chenu, Malý, and Mančal (2014) A. Chenu, P. Malý, and T. Mančal, “Dynamic coherence in excitonic molecular complexes under various excitation conditions,” Chem. Phys. 439, 100–110 (2014).
- Scholes (2018) G. D. Scholes, “Coherence from light harvesting to chemistry,” J. Phys. Chem. Lett. 9, 1568–1572 (2018).
- Ishizaki and Fleming (2009a) A. Ishizaki and G. R. Fleming, “Theoretical examination of quantum coherence in a photosynthetic system at physiological temperature,” Proc. Natl. Acad. Sci. 106, 17255–17260 (2009a).
- Sarovar et al. (2010) M. Sarovar, A. Ishizaki, G. R. Fleming, and K. B. Whaley, “Quantum entanglement in photosynthetic light-harvesting complexes,” Nat. Phys. 6, 462–467 (2010).
- van Amerongen, Valkunas, and van Grondelle (2000) V. van Amerongen, L. Valkunas, and R. van Grondelle, Photosynthetic Excitons (World Scientific Publishing Co. Pte. Ltd., 2000).
- Turner et al. (2013) D. B. Turner, D. J. Howey, E. J. Sutor, R. A. Hendrickson, M. W. Gealy, and D. J. Ulness, “Two-dimensional electronic spectroscopy using incoherent light: Theoretical analysis,” J. Phys. Chem. A 117, 5926–5954 (2013).
- Kassal, Yuen-Zhou, and Rahimi-Keshari (2013) I. Kassal, J. Yuen-Zhou, and S. Rahimi-Keshari, “Does coherence enhance transport in photosynthesis?” J. Phys. Chem. Lett. 4, 362–367 (2013).
- Tscherbul and Brumer (2018) T. V. Tscherbul and P. Brumer, “Non-equilibrium stationary coherences in photosynthetic energy transfer under weak-field incoherent illumination,” J. Chem. Phys. 148, 124114 (2018).
- Manzano (2013) D. Manzano, “Quantum transport in networks and photosynthetic complexes at the steady state,” PLOS ONE 8, 0057041 (2013).
- Jesenko and Žnidarič (2013) S. Jesenko and M. Žnidarič, “Excitation energy transfer efficiency: Equivalence of transient and stationary setting and the absence of non-Markovian effects,” J. Chem. Phys. 138, 174103 (2013).
- Chuang and Brumer (2020) C. Chuang and P. Brumer, “LH1–RC light-harvesting photocycle under realistic light–matter conditions,” J. Chem. Phys. 152, 154101 (2020).
- Sadeq and Brumer (2014) Z. S. Sadeq and P. Brumer, “Transient quantum coherent response to a partially coherent radiation field,” J. Chem. Phys. 140, 074104 (2014).
- Chenu and Brumer (2016) A. Chenu and P. Brumer, “Transform-limited-pulse representation of excitation with natural incoherent light,” J. Chem. Phys. 144, 044103 (2016).
- Breuer and Petruccione (2002) H.-P. Breuer and F. Petruccione, The Theory of Open Quantum Systems (Oxford University Press, 2002).
- Fassioli, Olaya-Castro, and Scholes (2012) F. Fassioli, A. Olaya-Castro, and G. D. Scholes, “Coherent energy transfer under incoherent light conditions,” J. Phys. Chem. Lett. 3, 3136–3142 (2012).
- Chan et al. (2018) H. C. H. Chan, O. E. Gamel, G. R. Fleming, and K. B. Whaley, “Single-photon absorption by single photosynthetic light-harvesting complexes,” J. Phys. B: At. Mol. Opt. Phys. 51, 054002 (2018).
- Tscherbul and Brumer (2015) T. V. Tscherbul and P. Brumer, “Partial secular Bloch-Redfield master equation for incoherent excitation of multilevel quantum systems,” J. Chem. Phys. 142, 104107 (2015).
- Pachón and Brumer (2013) L. A. Pachón and P. Brumer, “Incoherent excitation of thermally equilibrated open quantum systems,” Phys. Rev. A 87, 022106 (2013).
- Pachón, Botero, and Brumer (2017) L. A. Pachón, J. D. Botero, and P. Brumer, “Open system perspective on incoherent excitation of light-harvesting systems,” J. Phys. B: At. Mol. Opt. Phys. 50, 184003 (2017).
- Olšina et al. (2014) J. Olšina, A. G. Dijkstra, C. Wang, and J. Cao, “Can natural sunlight induce coherent exciton dynamics?” (2014), arXiv:1408.5385 [physics.chem-ph] .
- Ishizaki and Fleming (2012) A. Ishizaki and G. R. Fleming, “Quantum coherence in photosynthetic light harvesting,” Annu. Rev. Condens. Matter Phys. 3, 333–361 (2012).
- Chenu and Scholes (2015) A. Chenu and G. D. Scholes, “Coherence in energy transfer and photosynthesis,” Annu. Rev. Phys. Chem. 66, 69–96 (2015).
- Maguire, Iles-Smith, and Nazir (2019) H. Maguire, J. Iles-Smith, and A. Nazir, “Environmental nonadditivity and Franck-Condon physics in nonequilibrium quantum systems,” Phys. Rev. Lett. 123, 093601 (2019).
- Dijkstra and Beige (2019) A. G. Dijkstra and A. Beige, “Efficient long-distance energy transport in molecular systems through adiabatic passage,” J. Chem. Phys. 151, 034114 (2019).
- Janković and Mančal (2020) V. Janković and T. Mančal, “Exact description of excitonic dynamics in molecular aggregates weakly driven by light,” (2020), arXiv:2001.07180 [physics.chem-ph] .
- Ishizaki and Fleming (2009b) A. Ishizaki and G. R. Fleming, “Unified treatment of quantum coherent and incoherent hopping dynamics in electronic energy transfer: Reduced hierarchy equation approach,” J. Chem. Phys. 130, 234111 (2009b).
- Tanimura (2020) Y. Tanimura, “Numerically “exact” approach to open quantum dynamics: The hierarchical equations of motion (HEOM),” J. Chem. Phys. 153, 020901 (2020).
- Tomasi and Kassal (2020) S. Tomasi and I. Kassal, “Classification of coherent enhancements of light-harvesting processes,” J. Phys. Chem. Lett. 11, 2348–2355 (2020).
- Yang and Cao (2020) P.-Y. Yang and J. Cao, “Steady-state analysis of light-harvesting energy transfer driven by incoherent light: From dimers to networks,” J. Phys. Chem. Lett. 11, 7204–7211 (2020).
- Jung and Brumer (2020) K. A. Jung and P. Brumer, “Energy transfer under natural incoherent light: Effects of asymmetry on efficiency,” J. Chem. Phys. 153, 114102 (2020).
- León-Montiel, Kassal, and Torres (2014) R. d. J. León-Montiel, I. Kassal, and J. P. Torres, “Importance of excitation and trapping conditions in photosynthetic environment-assisted energy transport,” J. Phys. Chem. B 118, 10588–10594 (2014).
- Axelrod and Brumer (2018) S. Axelrod and P. Brumer, “An efficient approach to the quantum dynamics and rates of processes induced by natural incoherent light,” J. Chem. Phys. 149, 114104 (2018).
- Lee, Cao, and Gong (2012) C. K. Lee, J. Cao, and J. Gong, “Noncanonical statistics of a spin-boson model: Theory and exact Monte Carlo simulations,” Phys. Rev. E 86, 021109 (2012).
- Lee, Moix, and Cao (2012) C. K. Lee, J. Moix, and J. Cao, “Accuracy of second order perturbation theory in the polaron and variational polaron frames,” J. Chem. Phys. 136, 204120 (2012).
- Cerrillo and Cao (2014) J. Cerrillo and J. Cao, “Non-Markovian dynamical maps: Numerical processing of open quantum trajectories,” Phys. Rev. Lett. 112, 110401 (2014).
- Strümpfer and Schulten (2009) J. Strümpfer and K. Schulten, “Light harvesting complex II B850 excitation dynamics,” J. Chem. Phys. 131, 225101 (2009).
- Moix, Zhao, and Cao (2012) J. M. Moix, Y. Zhao, and J. Cao, “Equilibrium-reduced density matrix formulation: Influence of noise, disorder, and temperature on localization in excitonic systems,” Phys. Rev. B 85, 115412 (2012).
- Zurek (2003) W. H. Zurek, “Decoherence, einselection, and the quantum origins of the classical,” Rev. Mod. Phys. 75, 715–775 (2003).
- Schlosshauer (2007) M. Schlosshauer, Decoherence and the Quantum-To-Classical Transition (Springer-Verlag Berlin Heidelberg, 2007).
- Zhang et al. (2017) H.-D. Zhang, Q. Qiao, R.-X. Xu, X. Zheng, and Y. Yan, “Efficient steady-state solver for hierarchical quantum master equations,” J. Chem. Phys. 147, 044105 (2017).
- Zimanyi and Silbey (2012) E. N. Zimanyi and R. J. Silbey, “Theoretical description of quantum effects in multi-chromophoric aggregates,” Phil. Trans. R. Soc. A 370, 3620–3637 (2012).
- Tscherbul and Brumer (2014) T. V. Tscherbul and P. Brumer, “Long-lived quasistationary coherences in a -type system driven by incoherent light,” Phys. Rev. Lett. 113, 113601 (2014).
- Tomasi et al. (2019) S. Tomasi, S. Baghbanzadeh, S. Rahimi-Keshari, and I. Kassal, “Coherent and controllable enhancement of light-harvesting efficiency,” Phys. Rev. A 100, 043411 (2019).
- Valkunas, Abramavicius, and Mančal (2013) L. Valkunas, D. Abramavicius, and T. Mančal, Molecular Excitation Dynamics and Relaxation (WILEY-VCH Verlag GmbH & Co. KGaA, 2013).
- May and Kühn (2011) V. May and O. Kühn, Charge and Energy Transfer Dynamics in Molecular Systems, 3rd ed. (WILEY-VCH Verlag GmbH & Co. KGaA, Weinheim, 2011).
- Kreisbeck et al. (2011) C. Kreisbeck, T. Kramer, M. Rodríguez, and B. Hein, “High-performance solution of hierarchical equations of motion for studying energy transfer in light-harvesting complexes,” J. Chem. Theory Comput. 7, 2166–2174 (2011).
- Shatokhin et al. (2018) V. N. Shatokhin, M. Walschaers, F. Schlawin, and A. Buchleitner, “Coherence turned on by incoherent light,” New J. Phys. 20, 113040 (2018).
- Loudon (2000) R. Loudon, The Quantum Theory of Light (Oxford University Press, Oxford, 2000).
- Zhang et al. (2018) H.-D. Zhang, R.-X. Xu, X. Zheng, and Y. Yan, “Statistical quasi-particle theory for open quantum systems,” Mol. Phys. 116, 780–812 (2018).
- Shi et al. (2009) Q. Shi, L. Chen, G. Nan, R.-X. Xu, and Y. Yan, “Efficient hierarchical Liouville space propagator to quantum dissipative dynamics,” J. Chem. Phys. 130, 084105 (2009).
- Jang, Newton, and Silbey (2004) S. Jang, M. D. Newton, and R. J. Silbey, “Multichromophoric förster resonance energy transfer,” Phys. Rev. Lett. 92, 218301 (2004).
- Ma and Cao (2015) J. Ma and J. Cao, “Förster resonance energy transfer, absorption and emission spectra in multichromophoric systems. I. Full cumulant expansions and system-bath entanglement,” J. Chem. Phys. 142, 094106 (2015).
- Murnaghan (1962) F. Murnaghan, The Unitary and Rotation Groups (Spartan Books, Washington D.C., 1962).
- Kano and Wolf (1962) Y. Kano and E. Wolf, “Temporal coherence of black body radiation,” Proc. Phys. Soc. 80, 1273–1276 (1962).
- Mehta (1963) C. L. Mehta, “Coherence-time and effective bandwidth of blackbody radiation,” Il Nuovo Cimento (1955-1965) 28, 401–408 (1963).
- Jang and Mennucci (2018) S. J. Jang and B. Mennucci, “Delocalized excitons in natural light-harvesting complexes,” Rev. Mod. Phys. 90, 035003 (2018).
- Moix et al. (2011) J. Moix, J. Wu, P. Huo, D. Coker, and J. Cao, “Efficient energy transfer in light-harvesting systems, III: The influence of the eighth bacteriochlorophyll on the dynamics and efficiency in FMO,” J. Phys. Chem. Lett. 2, 3045–3052 (2011).
- Zhou et al. (1994) W. Zhou, R. LoBrutto, S. Lin, and R. E. Blankenship, “Redox effects on the bacteriochlorophyll -containing Fenna-Matthews-Olson protein from Chlorobium tepidum,” Photosynth. Res. 41, 89–96 (1994).
- Magdaong et al. (2017) N. C. M. Magdaong, G. Saer, R, D. M. Niedzwiedzki, and R. E. Blankenship, “Ultrafast spectroscopic investigation of energy transfer in site-directed mutants of the Fenna–Matthews–Olson (fmo) antenna complex from Chlorobaculum tepidum,” J. Phys. Chem. B 121, 4700–4712 (2017).
- Plenio and Huelga (2008) M. B. Plenio and S. F. Huelga, “Dephasing-assisted transport: quantum networks and biomolecules,” New J. Phys. 10, 113019 (2008).
- Blankenship (2014) R. E. Blankenship, Molecular Mechanisms of Photosynthesis (John Wiley & Sons, Ltd, 2014).
- Damjanović, Ritz, and Schulten (2000) A. Damjanović, T. Ritz, and K. Schulten, “Excitation energy trapping by the reaction center of Rhodobacter Sphaeroides,” Int. J. Quant. Chem. 77, 139–151 (2000).
- Caycedo-Soler et al. (2017) F. Caycedo-Soler, C. A. Schroeder, C. Autenrieth, A. Pick, R. Ghosh, S. F. Huelga, and M. B. Plenio, “Quantum redirection of antenna absorption to photosynthetic reaction centers,” J. Phys. Chem. Lett. 8, 6015–6021 (2017).
- Timpmann, Freiberg, and Sundström (1995) K. Timpmann, A. Freiberg, and V. Sundström, “Energy trapping and detrapping in the photosynthetic bacterium Rhodopseudomonas viridis: transfer-to-trap-limited dynamics,” Chem. Phys. 194, 275–283 (1995).
- Strümpfer and Schulten (2012) J. Strümpfer and K. Schulten, “Excited state dynamics in photosynthetic reaction center and light harvesting complex 1,” J. Chem. Phys. 137, 065101 (2012).
- Adolphs and Renger (2006) J. Adolphs and T. Renger, “How proteins trigger excitation energy transfer in the FMO complex of green sulfur bacteria,” Biophys. J. 91, 2778–2797 (2006).
- Wang et al. (2015) X. Wang, G. Ritschel, S. Wüster, and A. Eisfeld, “Open quantum system parameters for light harvesting complexes from molecular dynamics,” Phys. Chem. Chem. Phys. 17, 25629–25641 (2015).
- Kim, Choi, and Rhee (2018) C. W. Kim, B. Choi, and Y. M. Rhee, “Excited state energy fluctuations in the Fenna–Matthews–Olson complex from molecular dynamics simulations with interpolated chromophore potentials,” Phys. Chem. Chem. Phys. 20, 3310–3319 (2018).
- Dunn, Tempelaar, and Reichman (2019) I. S. Dunn, R. Tempelaar, and D. R. Reichman, “Removing instabilities in the hierarchical equations of motion: Exact and approximate projection approaches,” J. Chem. Phys. 150, 184109 (2019).
- Pelzer et al. (2014) K. M. Pelzer, T. Can, S. K. Gray, D. K. Morr, and G. S. Engel, “Coherent transport and energy flow patterns in photosynthesis under incoherent excitation,” J. Phys. Chem. B 118, 2693–2702 (2014).
- Hein et al. (2012) B. Hein, C. Kreisbeck, T. Kramer, and M. Rodríguez, “Modelling of oscillations in two-dimensional echo-spectra of the Fenna–Matthews–Olson complex,” New J. Phys. 14, 023018 (2012).
- Read et al. (2008) E. L. Read, G. S. Schlau-Cohen, G. S. Engel, J. Wen, R. E. Blankenship, and G. R. Fleming, “Visualization of excitonic structure in the Fenna-Matthews-Olson photosynthetic complex by polarization-dependent two-dimensional electronic spectroscopy,” Biophys. J. 95, 847 – 856 (2008).
- Pšenčík and Mančal (2017) J. Pšenčík and T. Mančal, “Light harvesting in green bacteria,” in Light Harvesting in Photosynthesis, edited by R. Croce, R. van Grondelle, H. van Amerongen, and I. van Stokkum (Taylor & Francis/CRC Press, 2017) Chap. 7, pp. 121–154.
- Thyrhaug et al. (2016) E. Thyrhaug, K. Žídek, J. Dostál, D. Bína, and D. Zigmantas, “Exciton structure and energy transfer in the Fenna–Matthews–Olson complex,” J. Phys. Chem. Lett. 7, 1653–1660 (2016).
- Dostál, Pšenčík, and Zigmantas (2016) J. Dostál, J. Pšenčík, and D. Zigmantas, “In situ mapping of the energy flow through the entire photosynthetic apparatus,” Nat. Chem. 8, 705–710 (2016).