Mechanism for Sequestering Magnetic Energy at Large Scales in Shear-Flow Turbulence
Abstract
Straining of magnetic fields by large-scale shear flow, generally assumed to lead to intensification and generation of small scales, is re-examined in light of the persistent observation of large-scale magnetic fields in astrophysics. It is shown that, in magnetohydrodynamic turbulence, unstable shear flows have the unexpected effect of sequestering magnetic energy at large scales, due to counteracting straining motion of nonlinearly excited large-scale stable eigenmodes. This effect is quantified via dissipation rates, energy transfer rates, and visualizations of magnetic field evolution by artificially removing the stable modes. These analyses show that predictions based upon physics of the linear instability alone miss substantial dynamics, including those of magnetic fluctuations.
Turbulence is a fundamentally multiscale process in which nonlinear dynamical motions carry energy across scales johnson2020 . One of the most robust and extensively studied mechanisms for cross-scale energy transfer is straining by shear flow batchelor1950 ; batchelor1954 , long recognized for its role in cascades to small scale townsend1976 . This process is especially active in magnetohydrodynamic (MHD) turbulence maron2004 . The stretching, squeezing, and folding of magnetic field lines by shear flow is readily discernible in visualizations, and leads to, for example, the enhancement of small-scale resistive dissipation and the intensification of magnetic energy at small scales beyond the viscous cutoff in turbulence with magnetic Prandtl number greater than unity schekochihin2002 ; maron2004 . The ubiquity of turbulence and turbulent straining is at odds with the well-established observations of magnetic fields at large scales in the universe (stellar, galactic, and beyond) neronov2010 ; kulsrud1999 and part of a conundrum as to how magnetic fields exist at such large scales brandenburg2005 ; tobias2013 ; squire2015 .
In this Letter we report the first observations that magnetic energy is, to a considerable degree, counterintuitively sequestered at large scales in straining by a paradigmatic turbulent shear flow. The result applies to a Kelvin-Helmholtz- (KH-)unstable flow maintained by an external force with a uniform magnetic field that is initially flow-aligned and therefore optimally configured to promote small-scale generation.
The process that counteracts the small scale generation of magnetic energy, which is quantified in this Letter, can be traced to the nonlinear excitation of large-scale stable linear eigenmodes hatch2011 . The dispersion relation that is associated with the shear-flow instability present in this study has a stable (damped) root, which acts to return energy and momentum to the mean profile, and which is excited to a high level by the nonlinearity fraser2017 ; fraser2021 . The nonlinear excitation of stable modes and its effect on turbulence levels and transport have been studied previously, particularly in the context of fusion-relevant gyroradius-scale turbulence terry2018 ; whelan2018 ; fraser2018 ; pueschel2016 ; makwana2014 ; hatch2013 ; makwana2012 ; hatch2011 ; makwana2011 ; terry2006 . Recent studies have shown that stable modes are excited in macroscopic shear-flow driven turbulence also and transiently affect momentum transport fraser2017 ; fraser2021 . However, critical features of counteracting straining motion of the stable modes, essential for large-scale sequestering of magnetic energy, have only come to light in the study described here.
To whit, stable-mode effects have been linked to small-scale dissipation hatch2013 , suggesting that they affect turbulence only if there is an increase in entropy. However, we show here that the reversible process by which stable modes return energy to the mean flow, in opposition to extraction by the instability, is quite effective at blunting the energy cascade to small scales. Stable modes have been connected to the shear-layer contraction (i.e., build up of mean flow) fraser2021 , but only as a transient process. Here we demonstrate that for a driven flow profile, the return of fluctuation energy to the mean profile occurs continuously at a rate that nearly matches the rate at which unstable modes attempt to flatten the flow profile.
The principal result of this Letter is that, in driven shear-flow MHD turbulence, the nonlinearly excited large-scale stable eigenmodes efficiently strain the magnetic fields, in a way that counteracts the effect of unstable modes, and thus greatly weakens the magnetic energy cascade to small scales.
We study an incompressible, two-dimensional (D) system with the initial fluid velocity given as and an initially uniform flow-aligned magnetic field as . The parameters represent the half-width of the flow-shear, the maximum fluid velocity, and the magnetic field strength, respectively, which are used to non-dimensionalize all the variables henceforth. Consequently, time and energy (per unit mass) have units of and . We describe the flow and the magnetic field by a stream function and a flux function , where and . We study their evolution using the momentum and induction equations of the standard MHD biskamp2003 ; remark_supplementary
| (1a) | ||||
| (1b) | ||||
where and are the vorticity and the current density; Poisson bracket ; the Alfvnic Mach number is ; the fluid and magnetic Reynolds numbers are defined as and . In this study, we take . Except for simulations where and (which will be stated explicitly), all others have . In Eq. (1), represents a body force that continuously regenerates the mean-flow shear. The expression in it indicates that the force acts only on the (-averaged) mean flow, where is the Fourier wavenumber. The forcing thus prevents gradual flattening of the mean flow as the instability extracts energy from it. A similar process occurs in astrophysical flows, where forces like gravitation replenish continually the shear profile, e.g., shear layers in accretion disks, stars, and planetary atmospheres. We represent this force by a Krook-like operator pueschel2014 ; marston2008 ; smith2021 , sometimes referred to as a profile relaxation term allawala2020 ,
| (2) |
where represents the rate at which the mean flow is forced towards the reference flow profile. We choose . Detailed analyses of a complete scan of different parameters will be reported elsewhere. The force balances the viscous diffusion of the mean shear layer, , in Eq. (1), to ensure an initial equilibrium state.
We add small-amplitude perturbations to the flow and the magnetic field remark_supplementary ; fraser2021 and perform time-integration of the above set of equations using the pseudospectral code Dedalus burns2020 for long times (; the -folding growth time of the instability is ). Apart from the simulation of that uses spectral resolution of Fourier (Chebyshev) modes along the -(-)axis for the box size of , all other simulations are performed at spectral resolution for the box size of , while convergence checks utilize resolutions as high as , with no substantial impact on dissipation rates and spectral energy densities. (All simulations additionally use dealiasing rule burns2020 ).
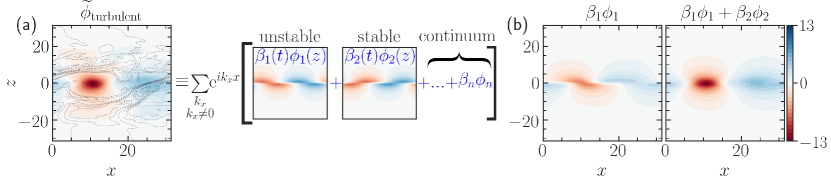
We also perform a corresponding eigenvalue calculation of the non-dissipative equations, derived from Eqs. (1) & (1b), by linearizing about the initial flow and magnetic field profiles fraser2021 . A priori, one does not know whether such an eigenmode basis is useful to understand turbulent features. Nevertheless, since the eigenmodes of this linear operator form a complete (albeit non-orthogonal) basis remark_B ; bender2019 , we can expand the time-evolving state space of nonlinear simulations in this basis after determining amplitude of each eigenmode at each as a function of time, where captures the turbulent state space and is the eigenmode fraser2021 ; tripathi2022_sherwood ; remark_energynorm ; remark_ECS_PCA . This procedure is illustrated in Fig. 1(a) where the eigenmodes of the flow fluctuations are shown. (The magnetic fluctuations have eigenmodes fraser2021 and parity ishizawa2019 ; sato2017 related to those of the flow fluctuations.)
At each wavenumber , linearly unstable to perturbations (i.e., for the present shear-flow instability), there is an unstable eigenmode and its conjugate stable eigenmode , with eigenvalues complex conjugate to each other chandrashekhar1961 ; fraser2017 . All the remaining eigenmodes have purely real eigenfrequencies tripathi2022_sherwood , belong to the continuum case1960 , and have narrow structures along the -axis.
Tracking the time evolution of each eigenmode amplitude in the nonlinear simulations, the initially exponentially decaying mode has been found to be nonlinearly excited to almost the same level as the unstable mode fraser2021 . The driving mechanism for this excitation is, at early times, the nonlinear interaction between the unstable modes, see, e.g., Refs. terry2006 ; makwana2011 ; fraser2021 . In the fully nonlinear phase, however, all modes that have large energy interact dominantly.
For a given KH-unstable wavenumber, it is found, in Fig. 1(b), that a combination of two eigenmodes from the above basis reconstructs the large-scale turbulent flow at that wavenumber. Figure 1(b) shows a reconstruction of the turbulent flow features using unstable eigenmodes at each unstable wavenumber (left column) and with their conjugate stable eigenmodes added (right column). Simply adding one additional mode drastically decreases the difference between the true stream function and its reduced representation compared to what is achieved with a reduced representation based on the unstable modes alone (such as those predicted by quasilinear models) remark_field_reconstruction .
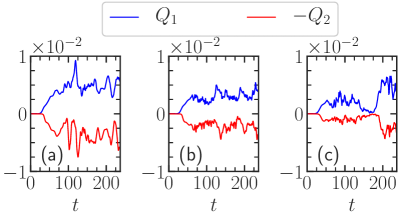
Note that the stable and unstable eigenmodes of the KH linear operator, employed above, are related to one another by a space-time-reversal symmetry operation bender2019 ; fu2020 . Thus the unstable and stable eigenmodes transfer energy in opposite directions—the former from the mean flow to the fluctuations, while the latter in the reverse direction. The energy that is available to cascade to small scales thus depends on this competition. The magnitude of the energy transfer rates between the background shear-flow and the fluctuation scale by the unstable and stable eigenmodes can be derived from the MHD equations remark_supplementary : and where is the linear growth rate of the unstable mode at that wavenumber .
Shown in Fig. 2 are the time traces of and for different (resistivity) over a challenging two orders of magnitude. Summation over the KH-unstable wavenumbers, , is used in determining and . Within each subplot, it is observed that and are nearly in equipartition in the nonlinear phase.
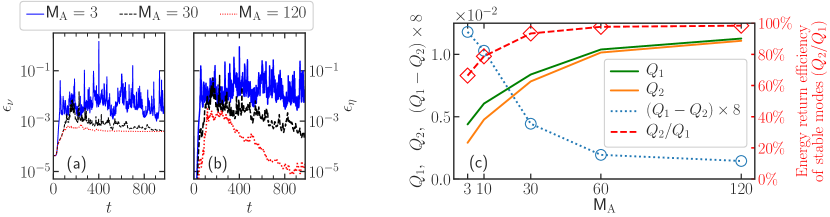
Stable-mode-excitation process may also be impacted by magnetic fields. To analyze such, we first observe the visco-resistive dissipation with varying field strengths in Figs. 3(a) and (b). The small-scale dissipation—a proxy of small-scale cascade—enhances with stronger fields, despite the fields reducing the linear growth rate of the instability, which sometimes is argued to lead to subdued turbulent fluctuations as the energy extraction rate by the unstable mode becomes lower.
To understand such enhanced dissipation rate, it may be instructive to compute the energy transfer rates between the background flow and the fluctuations due to both the unstable and stable eigenmodes. As seen in Fig. 3(c), the (time-averaged) energy injection rate by the instability, , decreases with stronger magnetic fields, as anticipated. However, the energy return rate by the stable modes, , is impacted more by the stronger fields, allowing larger energy cascade to small scales () that manifests as enhanced dissipation rate at such scales remark_instantaneousmean . Thus a stronger magnetic field, until it nearly stabilizes the system, allows more net energy extraction from the free-energy source in the nonlinear state. This questions models that assume the linear growth rate of an instability as a surrogate for the cascade rate or a proxy for nonlinear time, see, e.g., fuller2019 ; pessah2006 ; garaud2018 ; goodman1994 . Predictions based upon conventional wisdom of instability-saturation are thus challenged.
The ratio of the stable-eigenmode efficiency to the unstable-eigenmode efficiency in transferring energy between the fluctuations and the background flow is more than even for the strongest magnetic fields in Fig. 3(c), see the red dashed line. Such a strong Lorentz back-reaction is most potent in disrupting the vortex mak2017 and creating more small scales, leading to more reduced than the corresponding reduction in .
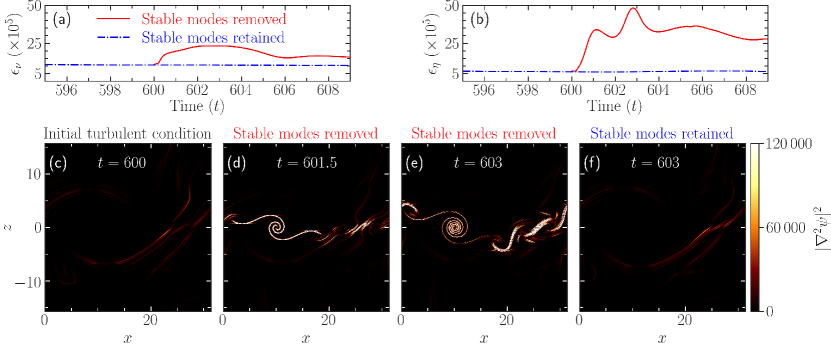
We now show visualizations of highly effective role of stable modes in structuring the magnetic fields. After carrying out an initial-value simulation up to a time when the dynamics are nonlinear and turbulent, we perform two distinct simulation continuations—one unchanged with the stable modes untouched, and another with the stable modes removed. For the latter, we set only the large-scale () stable-eigenmode amplitudes to zero at the instant when the simulation is restarted. The differences immediately afterward are significant: Removing stable eigenmodes rapidly enhances dissipation rates, see Figs. 4(a) and (b); (Multimedia view). To understand this rise in the dissipation, we plot the squared current density at small scales () in Figs. 4(c)–(f). Observe in Figs. 4(d) and (e) that the magnetic fields get rapidly distorted by the unstable modes of the flow, creating spirals as the counter-straining motion of the stable modes is removed. Note that the (un)stable modes have the largest strain around for the shown time, as can also be seen in the figure in the supplementary material. The stable modes here are deleted only at the instant when the simulation is paused; they are quickly nonlinearly driven back. In another simulation where the stable modes are untouched (retained), the spirals do not appear, however, and the time evolution immediately afterward remains almost identical to the initial stage [cf. Figs. 4(f) and Fig. 4(c)]. This exercise of instantaneous deletion of stable modes exposes the true nonlinear impact of the stable modes on magnetic field evolution, which remained hidden in Fig. 1(a).
This numerical experiment illustrates that the energy injected by the unstable modes into the large-scale fluctuations () via mean-fluctuation interactions, in the absence of stable modes, cascades in its entirety to smaller scales nonlinearly where it dissipates visco-resistively. This is a statement of the classical small-scale cascade by the advective nonlinearity, , as commonly understood. When is composed of comparable unstable and stable mode-amplitudes, though, the cascade is weakened by a factor of up to ten [Figs. 2, 3(c), and 4(b)]. Such a finding informs and can be used to improve reduced models of geo- and astrophysical instability-driven turbulence vandine2021 ; fuller2019 , where all the energy injected by the instability is assumed to pass through an inertial cascade, e.g., Refs. fuller2019 ; pessah2006 ; garaud2018 ; goodman1994 .
It may be noted that, although D and D turbulence have different conserved quantities and corresponding cascade processes, the stable modes of this study are the conjugate roots of the KH-instability, which exist in both D and D chandrashekhar1961 . Our preliminary studies of D turbulence have indeed shown that virtually identical stable mode excitation and its impact in turbulence occur in D as well. Details of such an investigation will be left for a future publication tripathi2022_testingQL .
This Letter demonstrates, for the first time, that stable modes via their counteracting straining motion of the flow, sequester magnetic energy at large scales, despite the conventional association of shear-flow with intensification and generation of small scales in MHD turbulence. Our analyses show that the stable modes greatly weaken the magnetic energy cascade to small scales and hence can thwart the magnetic-energy accumulation at sub-viscous scales in the high-magnetic-Prandtl-number regime, typically encountered in diffuse astrophysical plasmas; thus possibly allowing operation of a dynamo with magnetic fields concentrated at large scales, in accord with astrophysical observations schekochihin2002 ; tobias2013 . This work opens a new direction towards such studies.
Supplementary Material
See the supplementary material for the details of (I) simulation set-up, (II) modal energy evolution equation, (III) energy transfer rates between the background flow and the fluctuations, and (IV) residuals in low-order representation of the turbulent flow in Fig. 1(b). A simulation movie supplements Fig. 4 of this Letter.
Acknowledgements
Acknowledgements.
The authors thank K. Burns and Dedalus developers for assistance. Thanks also to N. Hurst for offering helpful discussions and a careful reading of the manuscript. This material is based upon work funded by the U.S. Department of Energy [DE-SC0022257] through the NSF/DOE Partnership in Basic Plasma Science and Engineering. We also gratefully acknowledge the Award no. DE-FG02-04ER54742, supported by the U.S. DOE, and Grant Nos. AST-1814327 and AST-1908338 supported by the U.S. NSF. All simulations reported herein were performed using the XSEDE supercomputing resources via Allocation No. TG-PHY130027. The data that support the findings of this study are available from the corresponding author upon reasonable request.References
- (1) P.L. Johnson, Energy Transfer from Large to Small Scales in Turbulence by Multiscale Nonlinear Strain and Vorticity Interactions, Phys. Rev. Lett. 124, 104501 (2020).
- (2) G.K. Batchelor, On the spontaneous magnetic field in a conducting liquid in turbulent motion, Proc. Roy. Soc. London, Ser. A 201, 405 (1950).
- (3) G.K. Batchelor and I. Proudman, The effects of rapid distortion of a fluid in turbulent motion, Q. J. Mech. Appl. Math 7, 83 (1954).
- (4) A.A. Townsend, The Structure of Turbulent Shear Flow, Ed. (Cambridge University Press, Cambridge, 1976).
- (5) J. Maron, S. Cowley, and J. McWilliams, The nonlinear magnetic cascade, Astrophys. J. 603, 569 (2004).
- (6) A.A. Schekochihin, J.L. Maron, S.C. Cowley, and J.C. McWilliams, The Small-Scale Structure of Magnetohydrodynamic Turbulence with Large Magnetic Prandtl Numbers, Astrophys. J. 576, 806 (2002).
- (7) A. Neronov and I. Vovk, Evidence for strong extragalactic magnetic fields from Fermi observations of TeV blazars, Science 328, 73 (2010).
- (8) R.M. Kulsrud, A critical review of galactic dynamos, Annu. Rev. Astron. Astrophys. 37, 37 (1999).
- (9) A. Brandenburg and K. Subramanian, Astrophysical magnetic fields and nonlinear dynamo theory, Phys. Rep. 417, 1 (2005).
- (10) S.M. Tobias, and F. Cattaneo, Shear-driven dynamo waves at high magnetic Reynolds number, Nature 497, 463 (2013).
- (11) J. Squire and A. Bhattacharjee, Generation of large-scale magnetic fields by small-scale dynamo in shear flows, Phys. Rev. Lett. 115, 175003 (2015).
- (12) D.R. Hatch, P.W. Terry, F. Jenko, F. Merz, and W.M. Nevins, Saturation of gyrokinetic turbulence through damped eigenmodes, Phys. Rev. Lett. 106, 115003 (2011).
- (13) A.E. Fraser, P.W. Terry, E.G. Zweibel, and M.J. Pueschel, Coupling of damped and growing modes in unstable shear flow, Phys. Plasmas 24, 062304 (2017).
- (14) A.E. Fraser, P.W. Terry, E.G. Zweibel, M.J. Pueschel, and J.M. Schroeder, The impact of magnetic fields on momentum transport and saturation of shear-flow instability by stable modes, Phys. Plasmas 28, 022309 (2021).
- (15) P.W. Terry, B.J. Faber, C.C. Hegna, V.V. Mirnov, M.J. Pueschel, and G.G. Whelan, Saturation scalings of toroidal ion temperature gradient turbulence, Phys. Plasmas 25, 012308 (2018).
- (16) G.G. Whelan, M.J. Pueschel, and P.W. Terry, Nonlinear Electromagnetic Stabilization of Plasma Microturbulence, Phys. Rev. Lett. 120, 175002 (2018).
- (17) A.E. Fraser, M.J. Pueschel, P.W. Terry, and E.G. Zweibel, Role of stable modes in driven shear-flow turbulence, Phys. Plasmas 25, 122303 (2018).
- (18) M.J. Pueschel, B.J. Faber, J. Citrin, C.C. Hegna, P.W. Terry, and D.R. Hatch, Stellarator Turbulence: Subdominant Eigenmodes and Quasilinear Modeling, Phys. Rev. Lett. 116, 085001 (2016).
- (19) K.D. Makwana, P.W. Terry, M.J. Pueschel, and D.R. Hatch, Subdominant Modes in Zonal-Flow-Regulated Turbulence, Phys. Rev. Lett. 112, 095002 (2014).
- (20) D.R. Hatch, F. Jenko, A.B. Navarro, and V. Bratanov, Transition between saturation regimes of gyrokinetic turbulence, Phys. Rev. Lett. 111, 175001 (2013).
- (21) K.D. Makwana, P.W. Terry, and J.-H. Kim, Role of stable modes in zonal flow regulated turbulence, Phys. Plasmas 19, 062310 (2012).
- (22) K.D. Makwana, P.W. Terry, J.-H. Kim, and D.R. Hatch, Damped eigenmode saturation in plasma fluid turbulence, Phys. Plasmas 18, 012302 (2011).
- (23) P.W. Terry, D.A. Baver, and S. Gupta, Role of stable eigenmodes in saturated local plasma turbulence, Phys. Plasmas 13, 022307 (2006).
- (24) D. Biskamp, Magnetohydrodynamic Turbulence (Cambridge University Press, 2003).
- (25) See supplementary material for (I) simulation set-up and details, (II) modal energy evolution equation, (III) energy transfer rates between the background flow and the fluctuations, and (IV) residuals in low-order representation of the turbulent flow in Fig. 1(b).
- (26) M.J. Pueschel, D. Told, P.W. Terry, F. Jenko, E.G. Zweibel, V. Zhdankin, and H. Lesch, Magnetic Reconnection Turbulence in Strong Guide Fields: Basic Properties and Application to Coronal Heating, Astrophys. J., Suppl. Ser. 213, 30 (2014).
- (27) J.B. Marston, E. Conover, and T. Schneider, Statistics of an Unstable Barotropic Jet from a Cumulant Expansion, J. Atmos. Sci. 65, 1955 (2008).
- (28) K.M. Smith, C.P. Caulfield, and J.R. Taylor, Turbulence in forced stratified shear flows, J. Fluid Mech. 910, A42 (2021).
- (29) A. Allawala, S.M. Tobias, and J.B. Marston, Dimensional reduction of direct statistical simulation, J. Fluid Mech. 898, A21 (2020).
- (30) K.J. Burns, G.M. Vasil, J.S. Oishi, D. Lecoanet, and B.P. Brown, Dedalus: A flexible framework for numerical simulations with spectral methods, Phys. Rev. Research 2, 023068 (2020).
- (31) This is because the dissipationless linear operator respects the Parity-Time-reversal symmetry operation, and hence its eigenmodes can be proven to form a complete basis. See Ref. bender2019 .
- (32) C.M. Bender, PT symmetry: In quantum and classical physics (World Scientific, 2019).
- (33) B. Tripathi, A.E. Fraser, P.W. Terry, E.G. Zweibel, and M.J. Pueschel, Near-cancellation of up- and down-gradient momentum transport in forced magnetized shear-flow turbulence, submitted to Phys. Plasmas (2022).
- (34) Energy norm is used to normalize the eigenmodes.
- (35) Some similarities can be identified between the state-space reconstruction via this method and other approaches of modeling turbulence, e.g., exact coherent structures suri2017 and proper orthogonal decomposition taira2017 .
- (36) B. Suri, J. Tithof, R.O. Grigoriev, and M.F. Schatz, Forecasting Fluid Flows Using the Geometry of Turbulence, Phys. Rev. Lett. 118, 114501 (2017).
- (37) K. Taira, S.L. Brunton, S.T.M. Dawson, C.W. Rowley, T. Colonius, B.J. McKeon, O.T. Schmidt, S. Gordeyev, V. Theofilis, and L.S. Ukeiley, Modal Analysis of Fluid Flows: An Overview, AIAA J 55, 4013 (2017).
- (38) A. Ishizawa, Y. Kishimoto, and Y. Nakamura, Multi-scale interactions between turbulence and magnetic islands and parity mixture—a review, Plasma Physics and Controlled Fusion 61, 054006 (2019).
- (39) M. Sato, and A. Ishizawa, Nonlinear parity mixtures controlling the propagation of interchange modes, Physics of Plasmas 24, 082501 (2017).
- (40) S. Chandrasekhar, Hydrodynamic and Hydromagnetic Stability (Clarendon Press, Oxford 1961).
- (41) K.M. Case, Stability of Inviscid Plane Couette Flow, Phys. Fluids 3, 143 (1960).
- (42) Thus reconstructed flow includes components that strain the magnetic field efficiently. The turbulent magnetic field, in contrast, requires many eigenmodes for each wavenumber. This is consistent with the fluid-straining-induced generation of small scales of the magnetic field, which when projected onto the eigenmode space covers a large number of marginally-stable continuum eigenmodes.
- (43) Y. Fu, and H. Qin, The physics of spontaneous parity-time symmetry breaking in the Kelvin-Helmholtz instability, New J. Phys 22, 083040 (2020).
- (44) Essentially the same conclusion has been found by recomputing and where they refer to the transfer rates between the fluctuations and the instantaneous mean profiles.
- (45) J. Fuller, A.L. Piro, and A.S. Jermyn, Slowing the spins of stellar cores, Mon. Not. R. Astron. Soc. 485, 3661 (2019).
- (46) M.E. Pessah, C.-K. Chan, and D. Psaltis, The signature of the magnetorotational instability in the Reynolds and Maxwell stress tensors in accretion discs, Mon. Not. R. Astron. Soc. 372, 183 (2006).
- (47) J. Goodman and G. Xu, Parasitic Instabilities in Magnetized, Differentially Rotating Disks, Astrophys. J. 432, 213 (1994).
- (48) P. Garaud, Double-Diffusive Convection at Low Prandtl Number, Annu. Rev. Fluid Mech. 50, 275 (2018).
- (49) J. Mak, S.D. Griffiths, and D.W. Hughes, Vortex disruption by magnetohydrodynamic feedback, Phys. Rev. Fluids 2, 113701 (2017).
- (50) B. Tripathi, A.E. Fraser, P.W. Terry, E.G. Zweibel, M.J. Pueschel, and E.H. Anders, Testing quasilinear theories in shear-flow turbulence, Manuscript in preparation.
- (51) A. VanDine, H.T. Pham, and S. Sarkar, Turbulent shear layers in a uniformly stratified background: DNS at high Reynolds number, J. Fluid Mech. 916, A42 (2021).