A theory of Stimulated and Spontaneous Axion Scattering

The propagation of electromagnetic (EM) fields through materials is governed by the celebrated Maxwell’s equations. In vacuum, these equations arise from the usual electromagnetic action $\mathcal{S}_{EM}=\frac{1}{2}\int d^{3}xdt\left(\epsilon_{0}\bm{E}^{2}-\bm{B}^{2}/\mu_{0}\right)$, where $\bm{E}$ and $\bm{B}$ are the electric and magnetic fields and $\epsilon_{0}$ and $\mu_{0}$ are the vacuum permittivity and permeability, respectively. For waves in a medium, it often suffices to promote these constants to material dependent values without changing the form of the action. However, in materials that break time reversal ($\mathcal{T}$) and inversion ($\mathcal{P}$) symmetries, a novel term becomes possible

with a pseudoscalar field $\theta$ (odd under both $\mathcal{T}$ and $\mathcal{P}$) coupled to electromangetic fields with strength $g_{a\gamma\gamma}$. This unusual term first appeared in quantum chromodynamics in the context of the strong charge conjugation-parity problem  [1, 2, 3]. The elementary excitations of the $\theta$ field, “axions”, are one of the prime candidates for cosmological dark matter  [4]. Despite significant efforts [5, 6, 7, 8, 9, 10, 11, 12, 13], they are yet to be detected experimentally.

The magnetoelectric coupling (1) has been known to exist in materials like Cr₂O₃ for quite some time [14, 15, 16]. The corresponding action, $\mathcal{S}_{\text{ME}}=\int d^{3}xdt\ \tilde{\alpha}_{ij}E_{i}B_{j}$, typically requires breaking both $\mathcal{P}$ and $\mathcal{T}$ symmetries. However, recently it has been recognized that even in the presence of $\mathcal{P}$ and/or $\mathcal{T}$, such a coupling can exist, if $\tilde{\alpha}_{ij}$ is given by $\alpha\epsilon_{0}c\theta\delta_{ij}/\pi$, with $c$ the speed of light, $\alpha$ the fine structure constant, and $\theta$ is an integer multiple of $\pi$. Having $\theta=n\pi$ with $n$ odd is the defining characteristic of topological insulators and is the origin of the topological magnetoelectric effect [17, 4, 2, 20].

When both $\mathcal{P}$ and $\mathcal{T}$ symmetries are broken, $\theta$ is no longer quantized. This is the case in Cr₂O₃, but also some magnetically doped topological insulators  [2, 6]. In the latter, finite antiferromagnetic (Neel) order leads to the deviation of $\theta$ from $\pi$, and longitudinal fluctuations of the Neel order parameter translate into fluctuations of $\theta$. To avoid confusion with the static part of $\theta$, these fluctuations are sometimes referred to as dynamical axions (DAs). DAs can hybridize with photons, forming axion-polaritons, and can manifest in a variety of dynamical responses  [3, 23, 24, 25, 26, 27]. Recent experimental work in thin film MnBi₂Te₄, which is predicted to host DAs, not only observed an axion-like coupling between the Neel order and $\bm{E}\cdot\bm{B}$  [28] but also directly probed DAs [29].

In this Letter, we focus on an inherently nonlinear phenomenon associated with light propagation in media supporting DAs, namely stimulated axion scattering (SAS). We show that the nonlinear excitation of DAs by two counterpropagating and orthogonally polarized fields leads to energy transfer between the two waves, with the intensity of the lower frequency (Stokes) mode growing exponentially in space. This can lead to reflection and transmission coefficients of the Stokes mode to be greater than $1$, and provides a sensitive probe of axion dynamics in the material. We also show that the Stokes mode can be generated spontaneously in the presence of a single applied electromagnetic wave when allowing for quantum or thermal fluctuations of the Neel order. Apart from providing a method for axion spectroscopy, this latter phenomenon can be employed to realize the phase conjugation effect  [30], which can be used in holography and image correction [31].

The exponential growth of the Stokes mode in SAS is similar to that seen in Stimulated Brillouin Scattering (SBS) [32] and Stimulated Raman Scattering (SRS) [33]. Instead of axions, the oscillator modes that enable SBS and SRS are atomic or molecular vibrations. Due to their high sensitivity, SBS and SRS have found applications in microscopy and spectroscopy both in inroganic materials and biological systems  [34, 35, 36, 37, 38]. Furthermore, the orthogonality of polarizations which maximizes SAS, concomitantly minimizes SBS and SRS; this sensitivity to the polarization can thus help isolate these different phenomena. Remarkably, even though the DA is driven by the product of the electric and magnetic fields, which one naively would expect to have weaker coupling to matter than the product of electric fields, the exponential amplification rate for SAS can be orders of magnitude larger than for SBS and SRS. This difference arises from the relatively low stiffness of the DA as compared to phonons, making DAs easier to excite. The strength of SAS and its tunability with external fields point towards possible practical importance of materials that host DAs for various optoelectronics applications where SBS and SRS are currently employed [39, 40].

Equations of Motion.— The minimal action for the coupled EM fields and DAs in a material is given by

Here we allow the permeability $\mu$ and permittivity $\epsilon$ to deviate from their vacuum values. $\tilde{\alpha}=\alpha\epsilon_{0}c/\pi$ is the coupling between the EM fields and the DA, $J,\,v$ and $m$ are the stiffness, velocity, and mass of the DA, respectively. For the remainder of this letter, we neglect the static part of $\theta$, as it only weakly affects SAS (see discussion in SI).

Minimizing the action leads to the coupled equations of motion

Here $c^{\prime}=1/\sqrt{\mu\epsilon}$ is the speed of light in the material and the current due to DAs is given by $\bm{J}_{\theta}=\partial_{t}\bm{P}_{\theta}+\bm{\nabla}\times\bm{M}_{\theta}$, where the polarization and magnetization induced by the DA are $\bm{P}_{\theta}=\tilde{\alpha}\theta\bm{B}$ and $\bm{M}_{\theta}=\tilde{\alpha}\theta\bm{E}$, respectively. A rate $\gamma$ has been introduced phenomenologically to describe the damping of axions (which in turn relates to the relaxation of appropriate spin waves in the material).

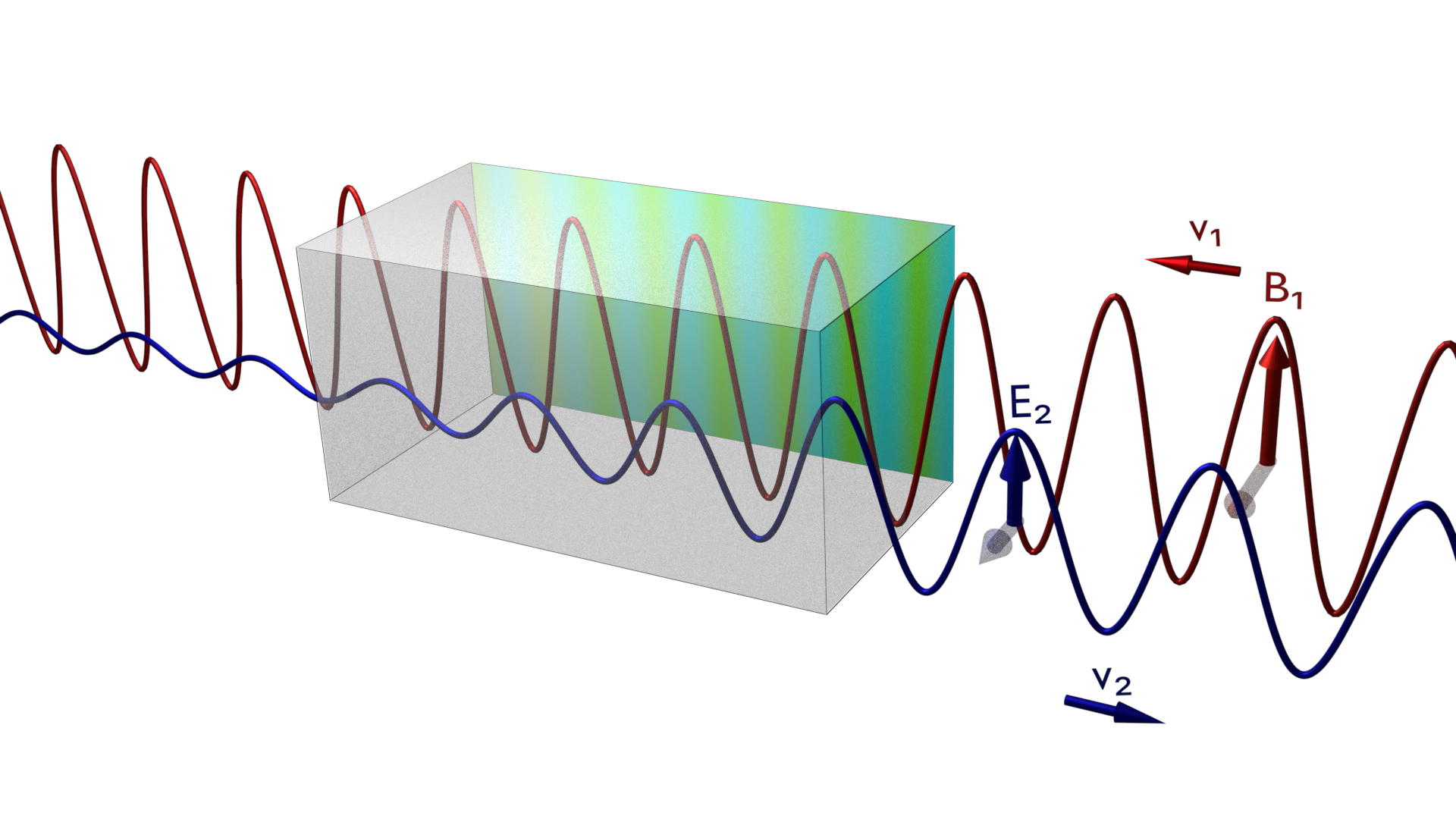
As depicted in Fig. 1, we consider two counter propagating electromagnetic waves, $\bm{E}=\bm{E}_{1}(z,t)e^{i(\omega_{1}t-k_{1}z)}+\bm{E}_{2}(z,t)e^{i(\omega_{2}t+k_{2}z)}$ + c.c., where $\bm{E}_{1(2)}$ are the slowly varying envelope functions and $\omega_{1(2)}$ and $k_{1(2)}$ their frequency and wavevector in the DA medium, respectively; the waves are oriented such that $\bm{E}_{1(2)}\parallel\bm{B}_{2(1)}$ and propagating along the $z$-axis. In the following we will ignore the spatial derivative part on the LHS of (3b), using the fact that the axion velocity is much smaller than the speed of light. Then, if the difference of the incoming light beam frequencies $\Omega=\omega_{1}-\omega_{2}$ matches the DA energy $m$, DAs will be resonantly excited. We refer to the higher frequency ($\omega_{1}$) mode as the pump, and the lower frequency ($\omega_{2}$) mode as the Stokes mode. We will also assume that $\Omega\ll\omega_{1,2}$, which allows us to use the rotating wave approximation. Making the ansatz $\theta=\theta_{12}(z,t)e^{i(\Omega t-Qz)}$ + c.c., with $Q=k_{1}+k_{2}$ and plugging in the solution for $\theta_{12}$ from Eq. (3b) into Eq. (3a), we find for the envelope functions $E_{1,2}$ in the slowly varying envelope approximation:

In the limit when $E_{1}$ can be assumed constant ($|E_{1}|\gg|E_{2}|$), the RHS of Eq. (4b) can be viewed as giving an imaginary contribution to the index of refraction, which on resonance, $\Omega=m$, goes as $-c^{\prime}g_{A}I_{1}/(2\omega_{1})$, where $g_{A}$ is the gain coefficient defined below in Eq. (6) and $I_{1}=2\epsilon_{0}c^{\prime}|E_{1}|^{2}$ is the intensity of the pump. This imaginary contribution to the index of refraction makes the amplitude of the Stokes mode $E_{2}$ grow exponentially as a function of $z$.

We can find time-independent solutions to the above equations for arbitrary incoming beam intensities and frequencies. Writing Eqs. (4) in terms of the intensites $I_{i}=2\epsilon_{0}c^{\prime}|E_{i}|^{2}$, their real parts yield

where

Here, $g_{A}$ is the gain coefficient for the SAS process mentioned above, $\xi_{12}=\omega_{2}/\omega_{1}$ and $n=\sqrt{\epsilon\mu/(\epsilon_{0}\mu_{0})}$ is the index of refraction of the DA medium. Note that Eqs. (5) imply $\nabla_{z}I_{1}/\omega_{1}=\nabla_{z}I_{2}/\omega_{2}$ which can be interpreted as the conservation of photon number between the Stokes and pump fields (single absorbed pump photon converts into single Stokes photon and DA). The pump beam enters the sample at $z=0$, while the Stokes beam at the opposite end at $z=L$. In the limit $\xi_{12}I_{1}(0)\gg I_{2}(0)$, the output Stokes intensity is readily obtained and found to grow exponentially in both the pump intensity and sample length:

The pump intensity $I_{1}(L)$ can be obtained using the photon conservation equation; a general solution for arbitrary pump and Stokes intensities is given in the SI.

Effect strength estimate. We now estimate $g_{A}$ using model parameters for Bi₂Se₃ doped with Fe [4]. The axion stiffness $J$ is given by (see SI for more details)

where $d_{i}$ are parameters in an effective four-band model for the system, $A_{1(2)}$ are velocities and $M_{0}$ is the gap at the $\Gamma$ point. Using $M_{0}=-.03$ eV, such that the band gap is trivial, $A_{1}=2.2$ eV$\cdot$Å , $A_{2}=4.1$ eV$\cdot$Å [4], an axion mass $m=2$ meV, magnon lifetime $\tau=0.1$ ns, pump frequency $\omega_{1}=1.481$ THz, index of refraction $n=1$, and assuming we are on resonance, we find $g_{A}=11.1\text{ m/GW}$.

Importantly, $g_{A}$ is proportional to the magnon lifetime and may be larger as magnon lifetimes can exceed $10-100$ ns [1]. Conversely, the gain bandwidth is protortional to the magnon relaxation rate; see Eq. (6). Thus, an increase of the bandwidth can be traded for a decrease in gain amplitude and vice versa. We also note that $g_{A}$ is inversely proportional to the Neel temperature, as $m\sim k_{B}T_{N}$ which can be modulated, for instance, by applying a magnetic field perpendicular to the AFM ordering direction. The controllability of gain parameters using external fields makes media supporting DAs potentially more versatile for non-linear amplification than materials where SBS, SRS or inversion breaking $\chi^{(2)}$ non-linearities [42] are employed.

The estimated gain $g_{A}$ is at least an order of magnitude larger than that obtained with SBS or SRS in materials typically used for nonlinear optics [5]. These processes are similar to SAS with the distinction that SBS and SRS involve lattice and molecular vibrations, respectively, in lieu of axions. The large SAS effect is at first surprising given that SBS and SRS proceed via a putatively stronger coupling $\sim\delta\epsilon\bm{E}\cdot\bm{E}$, where $\delta\epsilon$ is a change in the dielectric constant due to lattice or molecular vibrations while the former corresponds to a weaker coupling $\sim\theta\bm{E}\cdot\bm{B}$. In the SI, we argue that the reason for the strength of SAS in comparison to SBS (which in turn is generally stronger than SRS) is because of the lower stiffness of axions compared to phonons, making them easier to excite. Another process used for parameteric amplification when inversion symmetry is absent are $\chi^{(2)}$ non-linearities. Here, the gain coefficient scales as $\sim\sqrt{I}$ and is generically weaker than that for SAS, SBS, and SRS, but the gain bandwidth is much broader (for a quantiative comparison, see Ref. [42]). In short, SAS is a strong effect that could be used for parameteric amplification depending on the precise requirements of gain, bandwidth, and wavelength of interest.

Finally, we note that the maximum amplification obtainable is not without bounds. The DA amplitude is bounded due to the finite amplitude of the underlying Neel order. The deviation in the static $\theta$ from $\pi$ in AFM topological insulators is given by $\delta\theta=-m_{5}/M_{0}$ [3], where $m_{5}\sim k_{B}T_{N}$ is the contribution of the longitudinal AFM order to the electronic parameter $d_{5}$ introduced above. This limits the amplitude of DA to $\mathinner{\!\left\lvert\theta(z)\right\rvert}\leq\mathinner{\!\left\lvert m_{5}/M_{0}\right\rvert}$. Since $\theta(z)$ is excited by the product of the pump and Stokes fields, this puts a bound on the geometric mean of the local intensities, $\sqrt{I_{1}(z)I_{2}(z)}<2\alpha\omega_{1}/(\pi cg_{A})$. While this does not bound the relative gain ($I_{2}(0)/I_{2}(L)$), it asserts a bound on the maximum output Stokes intensity.

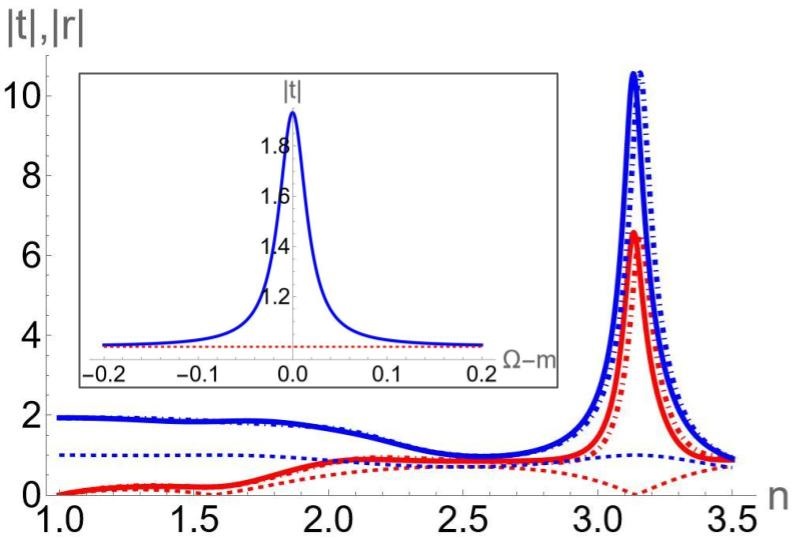
Boundary effects:— We now consider a more detailed treatment of the setup in Fig. 1 accounting for boundary conditions. The bulk equations of motion can be solved semi-analytically and a solution consistent with the boundary conditions can be found numerically by iteration; see SI for details. In Fig. 2, the numerically computed reflectance and transmittance coefficients of the Stokes mode are plotted as a function of index of refraction of the DA medium. We used a sample length $L=600$ $\mu$m, so that $2QL\gg 1$, which simplifies the treatment of the nonlinearity (see SI for details). The electric field amplitudes in the incident waves are $E_{1a}=5.5\times 10^{-3}$ V/nm and $E_{2a}=10^{-6}$ V/nm; this leads to a gain factor for the Stokes mode $G_{A}\approx 1.32$ at $n=1$. The transmittance being greater than 1 is a direct consequence of the exponential gain of the Stokes intensity governed by Eq. (7). Moreover, when the index of refraction $n\neq 1$, reflections at the boundaries occur, and a part of the Stokes beam can exit the sample in the direction opposite to its incidence, resulting in reflectance coefficients that can also exceed one.

Note that the Stokes and pump beam polarizations remain unchanged as they propagate through the medium if $\theta$ has no static part (A static contribution to $\theta$ due to the equilibrium AFM order is generally present; however, even $\theta=\pi$ in topological insulators produces only a very small Kerr rotation  [25, 16]. See SI for details). This simplification allows one to compute the reflection and transmission coefficients of the Stokes’ mode by geometrically adding up contributions from all possible paths of the beam (with an arbitrary number of internal reflections) accounting for the phase $e^{ik_{2}L}$ and gain $e^{G_{A}}$ factors accrued along the segments of the path. This analytical computation matches the exact result obtained by numerical iteration well, predicting a series of Fabry-Perot type resonances where the Stokes intensity can be greatly amplified (see an example in Fig. 2, and the analytical derivation in Eqs. (S74-77) of the SI).

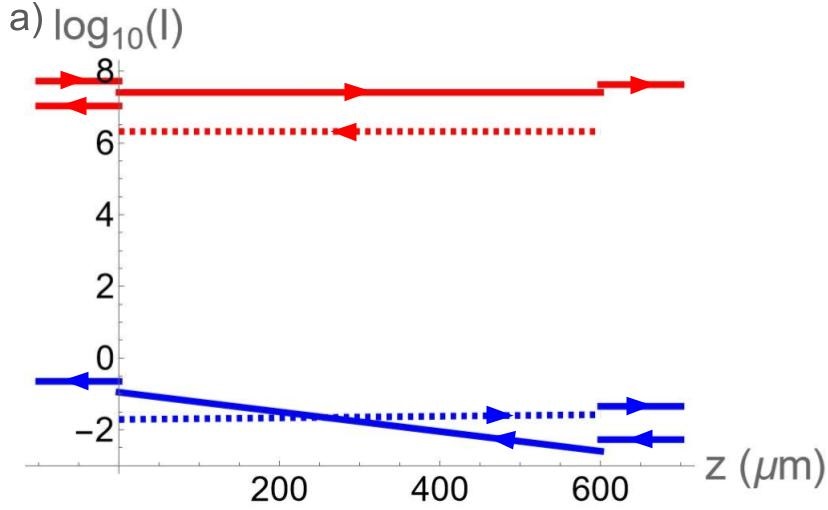
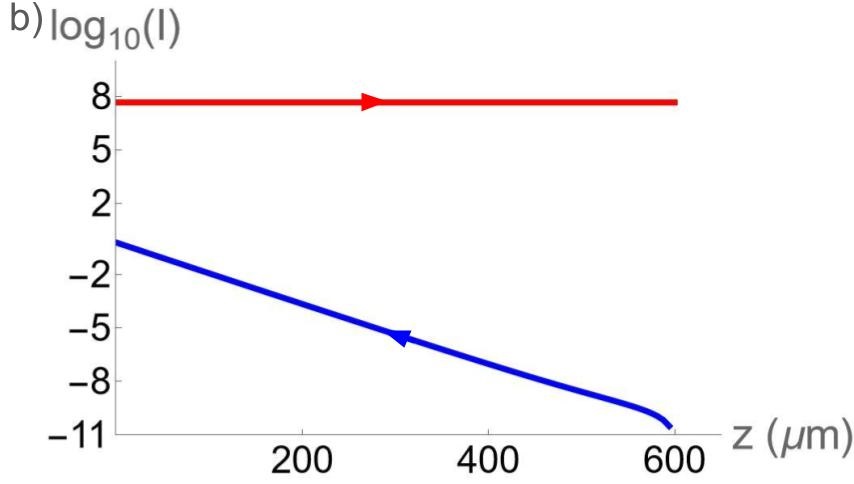
Spontaneous Emission:— We now explore the possibility of spontaneous generation of the Stokes mode due to the resonant amplification of quantum or thermal fluctuations in the DA medium in the presence of a single coherent source. Including Langevin fluctuations in Eq. (3b), and solving for $\theta$ and $E_{2}$ in the presence of a strong and thus approximately spatially constant $E_{1}$, we find the average Stokes intensity emitted at $z=0$ (See SI) to be given by [8, 9, 10]

where $I_{k}(x)$ is the $k^{\text{th}}$ modified Bessel function of the first kind, $G_{A}$ is the gain factor as defined above, and $I_{\text{Noise}}$ is an effective seed Stokes intensity,

We assume $\omega_{2}=1$ THz and $\gamma=0.04$ meV as above, sample cross-section $A\approx\lambda_{2}^{2}=3.55~{}\text{ mm}^{2}$ and $k_{B}T=1.3$ meV. The temperature $T$ and sample cross-section $A$ enter due to the average fluctuation strength of the dynamical axion $\langle|\theta|^{2}\rangle\propto T/A$ (in the classical limit) as discussed in the SI. In the large gain limit $G_{A}\gg 1$, Eq. (9) shows that the amplitude of the Stokes mode grows exponentially with the length of the sample, much like the stimulated case. We note again that the exponential growth is limited by the condition, $I_{2}\ll I_{1}$, saturating when intensities become comparable.

Conclusion:— SAS described in this paper is a qualitatively strong phenomenon that may be useful for spectroscopic studies of condensed matter axions, and could also have practical applications. For our estimates we considered Bi₂Se₃ which has well established electronic parameters, and is expected to harbor an AFM phase when doped with Fe (but has not yet been experimentally realized). The band gap and the putative Néel temperature are small—this limits the temperatures where the effect is present, $T<T_{N}$, as well as the frequencies of the electromagnetic sources, $\omega_{1,2}<|M_{0}|$. The above discussion also applies to thin films of $\text{MnBi}_{2}\text{Te}_{4}$, which likewise has a small band gap but a relatively large $T_{N}\sim 21$ K  [28]. Using Eq. (8), we find a similar gain coefficient $g_{A}$. We note here that bulk $\text{MnBi}_{2}\text{Te}_{4}$ possesses parity symmetry, and thus quantized $\theta$, which should make SAS vanish in bulk samples. SAS should also be present in known magnetoelectrics, such as $\text{Cr}_{2}\text{O}_{3}$. In $\text{Cr}_{2}\text{O}_{3}$, a significantly larger band gap (3 eV) allows application of much higher frequency pump and Stokes beams without deleterious bulk absorption, and the high Neel temperature (300 K) [47] should allow for observing SAS at room temperature. The gain coefficient benefits from higher frequency sources, though is likely suppressed by the larger band gap. An accurate estimate of $g_{A}$ for $\text{Cr}_{2}\text{O}_{3}$ is left for future work.

We would like to mention that the general framework of stimulated scattering, SAS, SBS, or SRS, thanks to its exquisite selectivity and sensitivity can have many applications in condensed matter systems. In particular, it may be useful for detecting other long sought excitations such as the Higgs mode [48, 49], or dark Josephson plasmons [50].

Finally, it is interesting to consider whether cosmic axions can be excited in a similar fashion by colliding sufficiently strong orthgonally polarized laser beams. The problem of light-by-light scattering has rich history, form the elastic Euler-Heisenberg scattering [51, 52, 53], to inelastic Beit-Wheeler electron-positron pair creation [54]. At low photon frequencies, elastic scattering is extremely weak and hard to detect except in some special cases [55]; inelastic scattering [54], on the other hand, requires very high frequency photons [56]. In this regard, SAS may be advantageous since the axion mass is expected to be in the sub eV range, and axions could be excited resonantly. Even if such experiments do not lead to the detection of cosmic axions, they should put new bounds on the mass and the coupling to this elusive particle.

The authors of Ref. [26] considered a similar set up where DAs were excited by counter-propagating electromagnetic waves. They do not include, however, the back-action of DAs on the driving fields crucial in SAS, instead studying the DA contribution to time-resolved Kerr rotations of a probe beam.

The code used to generate plots in Figs 2 and 3 can be obtained in the Harvard Dataverse with the identifier https://doi.org/10.7910/DVN/PZPUAW.

We thank V. Quito, P. Orth, S.-Y. Xu, R. McQueeney, J. Curtis, A. Bhattacharya, A. Burkov, A. Hoffman, and D. Basov for useful discussions. This work was supported by the Center for the Advancement of Topological Semimetals (CATS), an Energy Frontier Research Center funded by the U.S. Department of Energy (DOE) Office of Science (SC), Office of Basic Energy Sciences (BES), through the Ames National Laboratory under contract DE-AC02-07CH11358. The work of KA, particularly on the sample boundary effects, was supported by the US Department of Energy, Office of Science, Basic Energy Sciences, Materials Sciences and Engineering Division.