KM3NeT/ARCA expectations in view of a novel multimessenger study of starburst galaxies
Abstract
Starburst galaxies (SBGs) and more in general starforming galaxies represent a class of galaxies with a high star formation rate (up to 100 /year). Despite their low luminosity, they can be considered as guaranteed “factories” of high energy neutrinos, being “reservoirs” of accelerated cosmic rays and hosting a high density target gas in the central region. In this contribution we present a novel multimessenger study of these sources and the possibility of observing their neutrino signals with the KM3NeT/ARCA telescope. The differential sensitivity for different SBG scenarios is reported considering track-like neutrino events in the 100 GeV-100 PeV energy range.
1 Introduction
Star forming and Starburst Galaxies (SFGs and SBGs) are astrophysical sources which are characterised by a star formation rate which ranges from 10 to 100 times the one measured in normal galaxies like the Milky way [1]. The intense star-forming activity implies that a high density of interstellar gas is present in the source core which represents the target for inelastic collision of high-energy protons accelerated by supernova remnants. What is more, these sources are considered to be cosmic-ray reservoirs, the accelerated particles are likely to be confined within the astrophysical environment and therefore they have an enhanced probability of emitting gamma rays and neutrinos via hadronic collisions. Different analyses have been carried out about this source class (for instance see [3, 2]) and demonstrated that it cannot be the sole contribution for IceCube’s observations. Despite all the phenomenological constraints, SFGs and SBGs could still play an important role in high-energy neutrino production and explain a seizable part of these observations. In fact, recent works ([7, 4]) have renewed the analysis about this class. In particular, [7] has shown how their flux could be consistent with the one of through-going muon neutrinos, while [4] has performed a multi-component fit demonstrating that SBGs could explain up to of the high energy starting events (HESE) and their contribution is expected to peak at hundred of TeVs. The major obstacle encountered when obtaining their “calorimetric” parameters it is their low luminosity in the gamma-ray range. In fact, only a dozen of SBGs have been observed as point-like sources of gamma-rays by Fermi-LAT. However, this does not mean their diffuse contribution is negligible with respect to other sources when we consider their emission up to high redshifts as we highlight in this contribution. On the other hand, the IceCube collaboration ([5]) has reported a excess of neutrino events coming from NGC 1068, which is one of the SBG observed by the Fermi-LAT telescope. Moreover [6] has recently shown how the cores of SMC and Circinus galaxy could possibily be observed in six years of data taking by KM3NeT/ARCA, which would demonstrate that the star-forming activity is dominated by hadronic emissions. For this reason, the KM3NeT/ARCA telescope could be crucial in order to detect such sources both as point-like objects and to constrain their diffuse flux. In this context, we present a novel sensitivity study for the possible diffuse signal of this class of sources provided by [4] considering the phase 2.0 of the incoming KM3NeT/ARCA telescope. In this study, differentiating the energy range between 100 GeV and 10 PeV into 11 bins and only taking into account up-going track-like events, we calculate the KM3NeT/ARCA differential sensitivity for the expected diffuse SBG emission up to a .
2 Diffuse neutrino expectation from Starburst galaxies
In this section, we outline the theoretical model we used to estimate the Starburst Galaxies neutrino emissions. In particular, we make use of the semi-analytical approach put forward by [7, 4] . We suppose that the star-forming activity for these sources is concentrated on their central core. This particular region is usually called Starburst Nucleus (SBN) and we approximate it to be a spherical region of the order of pc (see [8] for the details ). As a result, we solve the leaky-box model equation in order to calculate the high-energy proton distribution within the SBN
| (2.1) |
where and are respectively the distribution function and the injection rate for protons. Eq. (2.1) physically represent the balance between the injection of the high-energy protons given by supernova remnants and the hadronic transport within the SBN itself. In fact, this phenonemon depends on the different timescales: the advection (), energy losses () and diffusion processes () (see [4] for the details). Assuming that protons are injected with a power-law spectrum in momentum space with spectral index and an exponential cutoff varying between , the flux is normalized by requiring that each supernova releases into protons of its total explosion kinetic energy (). We determine the neutrino flux of a single Starburst galaxy using the approach given by [9], which assumes that the pions carry a fixed energy portion of the high-energy protons . Assuming the calorimetric condition for the central part of these galaxies, their neutrino flux hardly depends on their structural details and physical parameters; but rather, it is mainly driven by the star formation rate (), the cut-off energy () of high-energy protons and the spectral index (). Hence, in order to constrain the number of SBGs in the Universe, we use the method of the star formation rate function. We consider the modified Shechter function SFR(z, ) reported in [7], which has been obtained by fitting in the redshift interval the IR+UV data of a Herschel Source sample after subtracting the AGN contamination. Furthermore, as assumed by [4], we do not assume every SBG to have the same spectral index, but instead, we exploit the variability of this parameter along the source class. In particular, we use a Gaussian distribution for these parameters employing the experimental catalogue provided by [10]. The formula for the diffuse flux is [4]
| (2.2) | ||||
where is the Hubble parameter with , and , and is the emitted neutrino fluxes averaged over the distribution of spectral indeces. The astrophysical flux provided by Eq. (2.2) peaks at hudrends of TeVs and it can explain up to of the IceCube’s observations without exceeding the gamma-ray constraints coming from the hadronic component of the extra-galactic background light (EGB). In fact, in Ref [4], a multi-component fit of the IceCube and Fermi-LAT datasets has been perfomed taking into account also Blazars and Radio Galaxies.The main result is highlighted in Fig. 1, which show the band and the maximum contribution accountable for SBGs; the left panel corresponds to the multi-messenger analysis with IceCube 7.5-year HESE, while the right one corresponds to the analysis with IceCube 6-year cascade.
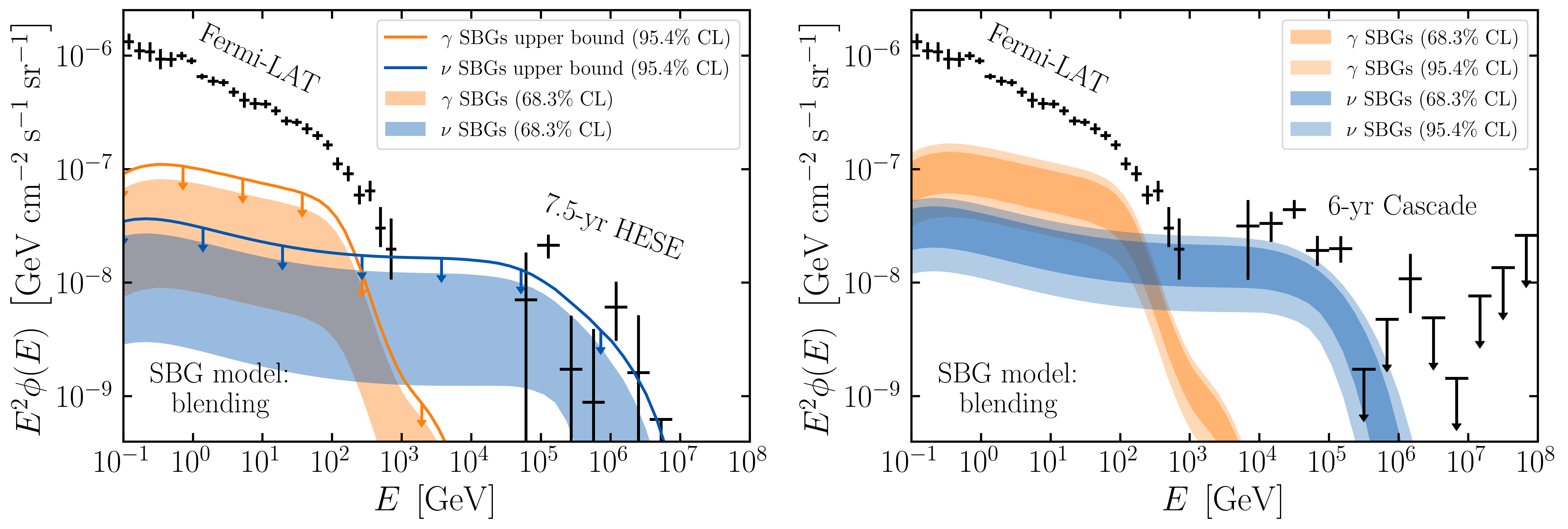
As a final remark, we wish to mention that the behaviour of the flux is a more complex function than a simple power-law due to the spectral index blending. However, for energies greater than 100 GeV, it can be accommodated by a simple power-law with an exponential cut-off (). In particular, for the forth-coming analysis, we use the expression
| (2.3) |
where GeV-1 cm-2 s-1 and for we use two different values: PeV and PeV which respectively correspond to 5 and 12 PeV for .
3 Differential sensitivity with KM3NeT/ARCA telescope
In order to estimate the probability for ARCA detector to observe the diffuse neutrino signal originated from SBGs was performed the calculation of an appropriate sensitivity. Given the model flux by 2.3, the sensitivity is calculated at 90 of Confidence Level (C.L.) obtained with the Neyman method:
| (3.1) |
The analysis was performed with the latest version of the KM3NeT-ARCA115 MC simulation: the background were defined as atmospheric muons and neutrinos, otherwise the signal was given by the neutrino SBGs (considering for both background and signal only via charge-current interactions). The calculation of the sensitivity involves several assumptions: an interval energy between 100 GeV - 10 PeV was divided in 11 bins in order to calculate a differential sensitivity, full sky scenario was considered (no selection in declination), two building blocks of KM3NeT/ARCA detector and five years of acquisition data were take into account. The optimization of this analysis was obtained by applying a bin-per-bin selection chain (Cut&Count method), considering the following variables: a pre-selection on the reconstructed zenith angle (only events with were considered) and a selection on the likelihood () from track reconstruction algorithm. Finally, once we applied all the selection chain we calculated bin-per-bin the sensitivity reported in eq. 3.1.
The differential KM3NeT/ARCA sensitivity for the described model of SBGs in eq. 2.3 is shown in fig. 2.
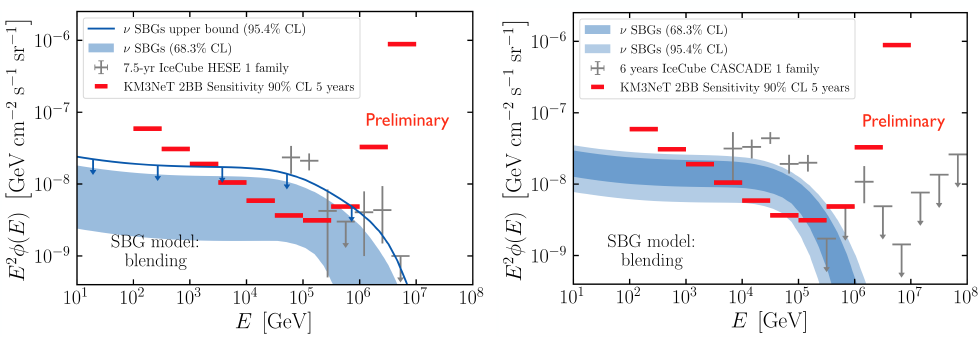
4 Discussion and conclusions
In this contribution we show how the observations of the incoming KM3NeT/ARCA telescope for the diffuse signal in the range of 100 TeV can be crucial to better constrain the physics of cosmic-ray “reservoir” sources. In particular we obtain the expected differential sensitivity of the incoming KM3NeT/ARCA for the diffuse contribution of starburst galaxies up to a redshift of . Even though differentiate single diffuse astrophysical components can be a challenging purpose, this study shows how few years of data taking of this new telescope can be crucial to select between different multicomponent fitting models.
References
- Thompson et al. [2006] Thompson T. A., Quataert E., Waxman E., Murray N., Martin C. L., 2006, ApJ, 645, 186. doi:10.1086/504035
- [2] I. Tamborra, S. Ando and K. Murase, JCAP 09 (2014), 043 doi:10.1088/1475-7516/2014/09/043 [arXiv:1404.1189 [astro-ph.HE]].
- [3] K. Murase, M. Ahlers and B. C. Lacki, Phys. Rev. D 88 (2013) no.12, 121301 doi:10.1103/PhysRevD.88.121301 [arXiv:1306.3417 [astro-ph.HE]].
- [4] A. Ambrosone, M. Chianese, D. F. G. Fiorillo, A. Marinelli, G. Miele and O. Pisanti, Mon. Not. Roy. Astron. Soc. 503 (2021) no.3, 4032-4049 doi:10.1093/mnras/stab659 [arXiv:2011.02483 [astro-ph.HE]].
- [5] M. G. Aartsen et al. [IceCube], Phys. Rev. Lett. 124 (2020) no.5, 051103 doi:10.1103/PhysRevLett.124.051103 [arXiv:1910.08488 [astro-ph.HE]].
- [6] A. Ambrosone, M. Chianese, D. F. G. Fiorillo, A. Marinelli and G. Miele, [arXiv:2106.13248 [astro-ph.HE]].
- [7] E. Peretti, P. Blasi, F. Aharonian, G. Morlino and P. Cristofari, Mon. Not. Roy. Astron. Soc. 493 (2020) no.4, 5880-5891 doi:10.1093/mnras/staa698 [arXiv:1911.06163 [astro-ph.HE]].
- [8] E. Peretti, P. Blasi, F. Aharonian and G. Morlino, Mon. Not. Roy. Astron. Soc. 487 (2019) no.1, 168-180 doi:10.1093/mnras/stz1161 [arXiv:1812.01996 [astro-ph.HE]].
- [9] S. R. Kelner, F. A. Aharonian and V. V. Bugayov, Phys. Rev. D 74 (2006), 034018 [erratum: Phys. Rev. D 79 (2009), 039901] doi:10.1103/PhysRevD.74.034018 [arXiv:astro-ph/0606058 [astro-ph]].
- [10] M. Ajello, M. Di Mauro, V. S. Paliya and S. Garrappa, Astrophys. J. 894 (2020) no.2, 88 doi:10.3847/1538-4357/ab86a6 [arXiv:2003.05493 [astro-ph.GA]].
The KM3NeT Collaboration author list (July 2021)
M. Ageron1,
S. Aiello2,
A. Albert3,55,
M. Alshamsi4,
S. Alves Garre5,
Z. Aly1,
A. Ambrosone6,7,
F. Ameli8,
M. Andre9,
G. Androulakis10,
M. Anghinolfi11,
M. Anguita12,
G. Anton13,
M. Ardid14,
S. Ardid14,
W. Assal1,
J. Aublin4,
C. Bagatelas10,
B. Baret4,
S. Basegmez du Pree15,
M. Bendahman4,16,
F. Benfenati17,18,
E. Berbee15,
A. M. van den Berg19,
V. Bertin1,
S. Beurthey1,
V. van Beveren15,
S. Biagi20,
M. Billault1,
M. Bissinger13,
M. Boettcher21,
M. Bou Cabo22,
J. Boumaaza16,
M. Bouta23,
C. Boutonnet4,
G. Bouvet24,
M. Bouwhuis15,
C. Bozza25,
H.Brânzaş26,
R. Bruijn15,27,
J. Brunner1,
R. Bruno2,
E. Buis28,
R. Buompane6,29,
J. Busto1,
B. Caiffi11,
L. Caillat1,
D. Calvo5,
S. Campion30,8,
A. Capone30,8,
H. Carduner24,
V. Carretero5,
P. Castaldi17,31,
S. Celli30,8,
R. Cereseto11,
M. Chabab32,
C. Champion4,
N. Chau4,
A. Chen33,
S. Cherubini20,34,
V. Chiarella35,
T. Chiarusi17,
M. Circella36,
R. Cocimano20,
J. A. B. Coelho4,
A. Coleiro4,
M. Colomer Molla4,5,
S. Colonges4,
R. Coniglione20,
A. Cosquer1,
P. Coyle1,
M. Cresta11,
A. Creusot4,
A. Cruz37,
G. Cuttone20,
A. D’Amico15,
R. Dallier24,
B. De Martino1,
M. De Palma36,38,
I. Di Palma30,8,
A. F. Díaz12,
D. Diego-Tortosa14,
C. Distefano20,
A. Domi15,27,
C. Donzaud4,
D. Dornic1,
M. Dörr39,
D. Drouhin3,55,
T. Eberl13,
A. Eddyamoui16,
T. van Eeden15,
D. van Eijk15,
I. El Bojaddaini23,
H. Eljarrari16,
D. Elsaesser39,
A. Enzenhöfer1,
V. Espinosa14,
P. Fermani30,8,
G. Ferrara20,34,
M. D. Filipović40,
F. Filippini17,18,
J. Fransen15,
L. A. Fusco1,
D. Gajanana15,
T. Gal13,
J. García Méndez14,
A. Garcia Soto5,
E. Garçon1,
F. Garufi6,7,
C. Gatius15,
N. Geißelbrecht13,
L. Gialanella6,29,
E. Giorgio20,
S. R. Gozzini5,
R. Gracia15,
K. Graf13,
G. Grella41,
D. Guderian56,
C. Guidi11,42,
B. Guillon43,
M. Gutiérrez44,
J. Haefner13,
S. Hallmann13,
H. Hamdaoui16,
H. van Haren45,
A. Heijboer15,
A. Hekalo39,
L. Hennig13,
S. Henry1,
J. J. Hernández-Rey5,
J. Hofestädt13,
F. Huang1,
W. Idrissi Ibnsalih6,29,
A. Ilioni4,
G. Illuminati17,18,4,
C. W. James37,
D. Janezashvili46,
P. Jansweijer15,
M. de Jong15,47,
P. de Jong15,27,
B. J. Jung15,
M. Kadler39,
P. Kalaczyński48,
O. Kalekin13,
U. F. Katz13,
F. Kayzel15,
P. Keller1,
N. R. Khan Chowdhury5,
G. Kistauri46,
F. van der Knaap28,
P. Kooijman27,57,
A. Kouchner4,49,
M. Kreter21,
V. Kulikovskiy11,
M. Labalme43,
P. Lagier1,
R. Lahmann13,
P. Lamare1,
M. Lamoureux222also at Dipartimento di Fisica, INFN Sezione di Padova and Università di Padova, I-35131, Padova, Italy4,
G. Larosa20,
C. Lastoria1,
J. Laurence1,
A. Lazo5,
R. Le Breton4,
E. Le Guirriec1,
S. Le Stum1,
G. Lehaut43,
O. Leonardi20,
F. Leone20,34,
E. Leonora2,
C. Lerouvillois1,
J. Lesrel4,
N. Lessing13,
G. Levi17,18,
M. Lincetto1,
M. Lindsey Clark4,
T. Lipreau24,
C. LLorens Alvarez14,
A. Lonardo8,
F. Longhitano2,
D. Lopez-Coto44,
N. Lumb1,
L. Maderer4,
J. Majumdar15,
J. Mańczak5,
A. Margiotta17,18,
A. Marinelli6,
A. Marini1,
C. Markou10,
L. Martin24,
J. A. Martínez-Mora14,
A. Martini35,
F. Marzaioli6,29,
S. Mastroianni6,
K. W. Melis15,
G. Miele6,7,
P. Migliozzi6,
E. Migneco20,
P. Mijakowski48,
L. S. Miranda50,
C. M. Mollo6,
M. Mongelli36,
A. Moussa23,
R. Muller15,
P. Musico11,
M. Musumeci20,
L. Nauta15,
S. Navas44,
C. A. Nicolau8,
B. Nkosi33,
B. Ó Fearraigh15,27,
M. O’Sullivan37,
A. Orlando20,
G. Ottonello11,
S. Ottonello11,
J. Palacios González5,
G. Papalashvili46,
R. Papaleo20,
C. Pastore36,
A. M. Păun26,
G. E. Păvălaş26,
G. Pellegrini17,
C. Pellegrino18,58,
M. Perrin-Terrin1,
V. Pestel15,
P. Piattelli20,
C. Pieterse5,
O. Pisanti6,7,
C. Poirè14,
V. Popa26,
T. Pradier3,
F. Pratolongo11,
I. Probst13,
G. Pühlhofer51,
S. Pulvirenti20,
G. Quéméner43,
N. Randazzo2,
A. Rapicavoli34,
S. Razzaque50,
D. Real5,
S. Reck13,
G. Riccobene20,
L. Rigalleau24,
A. Romanov11,42,
A. Rovelli20,
J. Royon1,
F. Salesa Greus5,
D. F. E. Samtleben15,47,
A. Sánchez Losa36,5,
M. Sanguineti11,42,
A. Santangelo51,
D. Santonocito20,
P. Sapienza20,
J. Schmelling15,
J. Schnabel13,
M. F. Schneider13,
J. Schumann13,
H. M. Schutte21,
J. Seneca15,
I. Sgura36,
R. Shanidze46,
A. Sharma52,
A. Sinopoulou10,
B. Spisso41,6,
M. Spurio17,18,
D. Stavropoulos10,
J. Steijger15,
S. M. Stellacci41,6,
M. Taiuti11,42,
F. Tatone36,
Y. Tayalati16,
E. Tenllado44,
D. Tézier1,
T. Thakore5,
S. Theraube1,
H. Thiersen21,
P. Timmer15,
S. Tingay37,
S. Tsagkli10,
V. Tsourapis10,
E. Tzamariudaki10,
D. Tzanetatos10,
C. Valieri17,
V. Van Elewyck4,49,
G. Vasileiadis53,
F. Versari17,18,
S. Viola20,
D. Vivolo6,29,
G. de Wasseige4,
J. Wilms54,
R. Wojaczyński48,
E. de Wolf15,27,
T. Yousfi23,
S. Zavatarelli11,
A. Zegarelli30,8,
D. Zito20,
J. D. Zornoza5,
J. Zúñiga5,
N. Zywucka21.
1Aix Marseille Univ, CNRS/IN2P3, CPPM, Marseille, France.
2INFN, Sezione di Catania, Via Santa Sofia 64, Catania, 95123 Italy.
3Université de Strasbourg, CNRS, IPHC UMR 7178, F-67000 Strasbourg, France.
4Université de Paris, CNRS, Astroparticule et Cosmologie, F-75013 Paris, France.
5IFIC - Instituto de Física Corpuscular (CSIC - Universitat de València), c/Catedrático José Beltrán, 2, 46980 Paterna, Valencia, Spain.
6INFN, Sezione di Napoli, Complesso Universitario di Monte S. Angelo, Via Cintia ed. G, Napoli, 80126 Italy.
7Università di Napoli “Federico II”, Dip. Scienze Fisiche “E. Pancini”, Complesso Universitario di Monte S. Angelo, Via Cintia ed. G, Napoli, 80126 Italy.
8INFN, Sezione di Roma, Piazzale Aldo Moro 2, Roma, 00185 Italy.
9Universitat Politècnica de Catalunya, Laboratori d’Aplicacions Bioacústiques, Centre Tecnològic de Vilanova i la Geltrú, Avda. Rambla Exposició, s/n, Vilanova i la Geltrú, 08800 Spain.
10NCSR Demokritos, Institute of Nuclear and Particle Physics, Ag. Paraskevi Attikis, Athens, 15310 Greece.
11INFN, Sezione di Genova, Via Dodecaneso 33, Genova, 16146 Italy.
12University of Granada, Dept. of Computer Architecture and Technology/CITIC, 18071 Granada, Spain.
13Friedrich-Alexander-Universität Erlangen-Nürnberg, Erlangen Centre for Astroparticle Physics, Erwin-Rommel-Straße 1, 91058 Erlangen, Germany.
14Universitat Politècnica de València, Instituto de Investigación para la Gestión Integrada de las Zonas Costeras, C/ Paranimf, 1, Gandia, 46730 Spain.
15Nikhef, National Institute for Subatomic Physics, PO Box 41882, Amsterdam, 1009 DB Netherlands.
16University Mohammed V in Rabat, Faculty of Sciences, 4 av. Ibn Battouta, B.P. 1014, R.P. 10000 Rabat, Morocco.
17INFN, Sezione di Bologna, v.le C. Berti-Pichat, 6/2, Bologna, 40127 Italy.
18Università di Bologna, Dipartimento di Fisica e Astronomia, v.le C. Berti-Pichat, 6/2, Bologna, 40127 Italy.
19KVI-CART University of Groningen, Groningen, the Netherlands.
20INFN, Laboratori Nazionali del Sud, Via S. Sofia 62, Catania, 95123 Italy.
21North-West University, Centre for Space Research, Private Bag X6001, Potchefstroom, 2520 South Africa.
22Instituto Español de Oceanografía, Unidad Mixta IEO-UPV, C/ Paranimf, 1, Gandia, 46730 Spain.
23University Mohammed I, Faculty of Sciences, BV Mohammed VI, B.P. 717, R.P. 60000 Oujda, Morocco.
24Subatech, IMT Atlantique, IN2P3-CNRS, Université de Nantes, 4 rue Alfred Kastler - La Chantrerie, Nantes, BP 20722 44307 France.
25Università di Salerno e INFN Gruppo Collegato di Salerno, Dipartimento di Matematica, Via Giovanni Paolo II 132, Fisciano, 84084 Italy.
26ISS, Atomistilor 409, Măgurele, RO-077125 Romania.
27University of Amsterdam, Institute of Physics/IHEF, PO Box 94216, Amsterdam, 1090 GE Netherlands.
28TNO, Technical Sciences, PO Box 155, Delft, 2600 AD Netherlands.
29Università degli Studi della Campania "Luigi Vanvitelli", Dipartimento di Matematica e Fisica, viale Lincoln 5, Caserta, 81100 Italy.
30Università La Sapienza, Dipartimento di Fisica, Piazzale Aldo Moro 2, Roma, 00185 Italy.
31Università di Bologna, Dipartimento di Ingegneria dell’Energia Elettrica e dell’Informazione "Guglielmo Marconi", Via dell’Università 50, Cesena, 47521 Italia.
32Cadi Ayyad University, Physics Department, Faculty of Science Semlalia, Av. My Abdellah, P.O.B. 2390, Marrakech, 40000 Morocco.
33University of the Witwatersrand, School of Physics, Private Bag 3, Johannesburg, Wits 2050 South Africa.
34Università di Catania, Dipartimento di Fisica e Astronomia "Ettore Majorana", Via Santa Sofia 64, Catania, 95123 Italy.
35INFN, LNF, Via Enrico Fermi, 40, Frascati, 00044 Italy.
36INFN, Sezione di Bari, via Orabona, 4, Bari, 70125 Italy.
37International Centre for Radio Astronomy Research, Curtin University, Bentley, WA 6102, Australia.
38University of Bari, Via Amendola 173, Bari, 70126 Italy.
39University Würzburg, Emil-Fischer-Straße 31, Würzburg, 97074 Germany.
40Western Sydney University, School of Computing, Engineering and Mathematics, Locked Bag 1797, Penrith, NSW 2751 Australia.
41Università di Salerno e INFN Gruppo Collegato di Salerno, Dipartimento di Fisica, Via Giovanni Paolo II 132, Fisciano, 84084 Italy.
42Università di Genova, Via Dodecaneso 33, Genova, 16146 Italy.
43Normandie Univ, ENSICAEN, UNICAEN, CNRS/IN2P3, LPC Caen, LPCCAEN, 6 boulevard Maréchal Juin, Caen, 14050 France.
44University of Granada, Dpto. de Física Teórica y del Cosmos & C.A.F.P.E., 18071 Granada, Spain.
45NIOZ (Royal Netherlands Institute for Sea Research), PO Box 59, Den Burg, Texel, 1790 AB, the Netherlands.
46Tbilisi State University, Department of Physics, 3, Chavchavadze Ave., Tbilisi, 0179 Georgia.
47Leiden University, Leiden Institute of Physics, PO Box 9504, Leiden, 2300 RA Netherlands.
48National Centre for Nuclear Research, 02-093 Warsaw, Poland.
49Institut Universitaire de France, 1 rue Descartes, Paris, 75005 France.
50University of Johannesburg, Department Physics, PO Box 524, Auckland Park, 2006 South Africa.
51Eberhard Karls Universität Tübingen, Institut für Astronomie und Astrophysik, Sand 1, Tübingen, 72076 Germany.
52Università di Pisa, Dipartimento di Fisica, Largo Bruno Pontecorvo 3, Pisa, 56127 Italy.
53Laboratoire Univers et Particules de Montpellier, Place Eugène Bataillon - CC 72, Montpellier Cédex 05, 34095 France.
54Friedrich-Alexander-Universität Erlangen-Nürnberg, Remeis Sternwarte, Sternwartstraße 7, 96049 Bamberg, Germany.
55Université de Haute Alsace, 68100 Mulhouse Cedex, France.
56University of Münster, Institut für Kernphysik, Wilhelm-Klemm-Str. 9, Münster, 48149 Germany.
57Utrecht University, Department of Physics and Astronomy, PO Box 80000, Utrecht, 3508 TA Netherlands.
58INFN, CNAF, v.le C. Berti-Pichat, 6/2, Bologna, 40127 Italy.