Field-induced dimer orders in quantum spin chains
Abstract
Field-induced excitation gaps in quantum spin chains are an interesting phenomenon related to confinements of topological excitations. In this paper, I present a novel type of this phenomenon. I show that an effective magnetic field with a fourfold screw symmetry induces the excitation gap accompanied by dimer orders. The dimer order parameter and the excitation gap exhibit characteristic power-law dependence on the fourfold screw-symmetric field. Moreover, the field-induced dimer order and the field-induced Néel order coexist when the external uniform magnetic field, the fourfold screw-symmetric field, and the twofold staggered field are applied. This situation is in close connection with a compound [J. Liu et al., Phys. Rev. Lett. 122, 057207 (2019)]. In this paper, I discuss a mechanism of field-induced dimer orders by using a density-matrix renormalization group method, a perturbation theory, and quantum field theories.
I Introduction
Quantum spin- chains do not have a unique gapped ground state in the presence of the time-reversal symmetry unless either the U(1) spin-rotation symmetry or the translation symmetry is broken Lieb et al. (1961); Affleck and Haldane (1987); Furuya and Oshikawa (2017). For example, the spin- Heisenberg antiferromagnetic (HAFM) chain has a unique gapless ground state called the Tomonaga-Luttinger (TL) liquid state Giamarchi (2004). Even when the time-reversal symmetry is broken by the external magnetic field, the TL liquid does not immediately acquire the gap, though it eventually does with the saturated magnetization Metlitski and Thorngren (2018). This is because the external magnetic field is uniform in the scale of spin chains. It breaks neither the U(1) rotation nor the translation symmetry. Interestingly, however, there are several spin- chain compounds where the magnetic field immediately opens the excitation gap Dender et al. (1997); Zvyagin et al. (2004); Umegaki et al. (2009).
This puzzle of the field-induced gap was found in Cu Benzoate Dender et al. (1997) and later solved with quantum field theories Oshikawa and Affleck (1997); Affleck and Oshikawa (1999); Furuya and Oshikawa (2012). Essentially, the field-induced excitation gap in those compounds comes from an absence of a bond-centered inversion symmetry. This low crystalline symmetry allows the tensor of electron spins to have a twofold staggered component. The magnetic field, when combined with the low symmetry, generates a twofold staggered magnetic field that breaks the translation symmetry. As a result, the uniform magnetic field induces the excitation gap and also the Néel order in the direction of the effectively generated twofold staggered magnetic field. Thanks to the dimensionality and strong interactions among elementary excitations, the excitation gap and the Néel order exhibit interesting power-law behaviors that deviate from spin-wave predictions Oshikawa and Affleck (1997); Affleck and Oshikawa (1999); Furuya et al. (2011). The phenomenon of the field-induced excitation gap has drawn attention for its connection with confinement of topological excitations Faure et al. (2018); Takayoshi et al. (2018).
In this paper, I discuss a novel field-induced excitation gap phenomenon. That is field-induced dimer orders in quantum spin chains.
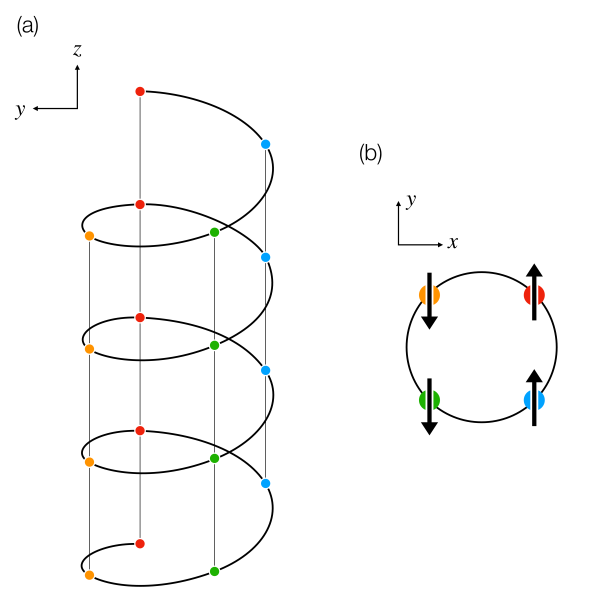
A key ingredient is a fourfold screw symmetry (Fig. 1). The screw structure can violate two kinds of inversion symmetries at the same time, namely, the bond-centered inversion symmetry and a site-centered inversion symmetry. Such a fourfold structure is indeed incorporated in the tensor of spin-chain compounds, Kimura et al. (2013); Faure et al. (2018) and Liu et al. (2019). When the uniform magnetic field is externally applied, the fourfold screw symmetry manifests itself as an effective fourfold screw-symmetric magnetic field. The fourfold screw field brings dimer orders to spin chains immediately. I discuss first a mechanism of the dimer-order generation. Next I take the twofold staggered field into account and discuss coexistent growth of the dimer and Néel orders with increase of the uniform magnetic field.
This paper is organized as follows. I define a spin-chain model and show numerical evidence of the field-induced dimer orders in the simplest case in Sec. II. A qualitative mechanism of field-induced dimer orders is discussed in Sec. III, where the spin-chain model is replaced to a spinless fermion model which is smoothly deformed from the original spin-chain model. Here, the low-energy effective Hamiltonian is systematically derived. In Sec. IV, on the basis of observations made in Sec. III, I develop a quantum field theory that explains quantitatively numerical results of Sec. II. The quantum field theory also predicts the coexistence of the dimer and Néel orders both of which grow with the uniform magnetic field. This coexistent growth of the dimer and Néel orders are discussed in Sec. V, which is supported by numerical calculations. I also discuss relevance of theoretical results to experiments in Sec. VI. Finally, I summarize the paper in Sec. VII.

II Screw field
II.1 Definition of the model
In this paper I discuss a quantum spin- chain with the following Hamiltonian:
| (1) |
where is the spin operator, is the antiferromagnetic exchange coupling, and for respectively, . Parameters , , and denote the uniform magnetic field, the twofold staggered field, and the fourfold screw field, respectively. Note that has a simple expression,
| (2) |
Throughout this paper, I employ the unit of unless otherwise stated, where is the lattice spacing.
The spin-chain model (1) is related to a model proposed for Liu et al. (2019) but differs from the latter in three points: field directions, a weak exchange anisotropy, and a uniform Dzyaloshinskii-Moriya (DM) interaction. In Ref. Liu et al. (2019), and are applied in the and the directions, respectively. The model used in Ref. Liu et al. (2019) contains a weak XXZ interaction and the uniform DM interaction. Those differences hardly affect the ground state of , which are to be clarified in Sec. VI.
In the compound Liu et al. (2019), the twofold staggered field and the fourfold screw field originate from the tensor of electrons and are thus proportional to the externally applied uniform magnetic field . In this section, I first deal with an unrealistic but simplest situation with and in Sec. II.2. I will discuss a more realistic situation with and later in Sec. V.
II.2 Fourfold screw field
The fourfold screw field can generate an excitation gap all by itself to the ground state of the spin chain. To show this, I set and discuss the dependence of the lowest-energy excitation gap from the ground state. The Hamiltonian is thus simplified as
| (3) |
When , the ground state of the model (3) is gapless Giamarchi (2004). Figure 2 shows numerical results on the excitation gap obtained by using the density-matrix renormalization group (DMRG) method with the ITensor C++ library ITe , where I used the bond dimension and the truncation error cutoff . Note that all the DMRG calculations in this paper were performed with the open boundary condition. The DMRG result implies that an infinitesimal immediately opens the excitation gap between the ground state and the lowest-energy excited state,
| (4) |
Similarly to the twofold staggered field, the fourfold screw field induces an excitation gap with a power law. However, the power differs from that, , of the twofold staggered field Oshikawa and Affleck (1997); Affleck and Oshikawa (1999).
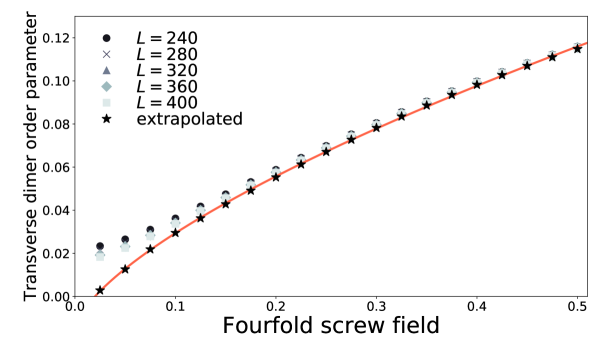
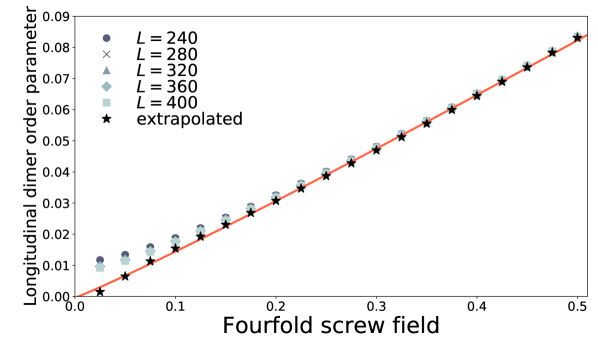
Unlike the twofold staggered field, the fourfold screw field induces no Néel order. Instead, induces dimer orders (Figs. 3 and 4),
| (5) | ||||
| (6) |
where is the length of the spin chain. The dimer order parameters (5) and (6) show different power-law dependence on . DMRG results (Figs. 3 and 4) imply
| (7) | ||||
| (8) |
Induction of by is easily understandable. Let us recall that is coupled to an operator,
| (9) |
The fourfold screw field induces the uniform order:
| (10) |
The longitudinal dimer order parameter is written in terms of as
| (11) |
Nonzero follows immediately from the uniform order (10). However, the induction of the transverse dimer order (7) is nontrivial.
III Free spinless fermion theory
This section is devoted to a qualitative explanation on a mechanism of the field-induced transverse dimer order (7). For this purpose, I rewrite the spin chain (3) in terms of spinless fermions with the aid of the Jordan-Wigner transformation Giamarchi (2004). Let and be creation and annihilation operators of the spinless fermion at the site , respectively. The spin-chain model (3) is equivalent to the following model of interacting spinless fermions:
| (12) |
where denotes the Hermitian conjugate.
The interaction of spinless fermions, the second line of Eq. (12), comes from the longitudinal component of the exchange interaction, . Even if this interaction is ignored, qualitative aspects of the ground state are kept intact since the HAFM chain and the XY chain belong to the same TL-liquid phase Giamarchi (2004). Therefore, I discuss in this section the free spinless fermion model,
| (13) |
instead of the model (12). In terms of spins, it is the XY chain in the fourfold screw field,
| (14) |
III.1 Particle-hole excitations
Performing the Fourier transformation on Eq. (14), I obtain
| (15) |
where and is the wave number. When , the spinless fermion is free and has the simple cosine dispersion. Since the total magnetization is zero in the XY chain, the cosine band is half occupied and the Fermi points are located at .
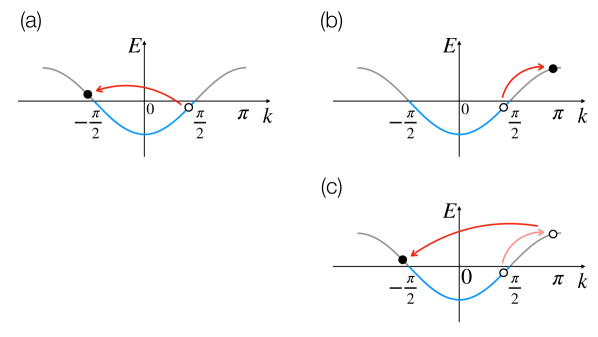
Let us make some observations on effects of the fourfold screw field on the free spinless fermion chain in the TL-liquid phase. Particle-hole excitations are the fundamental low-energy excitation in the TL-liquid phase. An operator , which creates a particle-hole excitation with a wave number , can be written as a superposition of bosonic creation and annihilation operators of the TL liquid Giamarchi (2004). When the second line of Eq. (15) acts on the ground state of the XY model, particle-hole excitations with wave numbers are generated [Fig. 5 (b)]. Apparently, such an term hardly affects the low-energy physics of the XY model because these particle-hole excitations have large excitation energies of . However, applying the term twice to the ground state, I can generate low-energy particle-hole excitations with the wave number [Figs. 5 (a,c)]. These observations show that though the fourfold screw field is highly irrelevant, it will generates a relevant interaction in a second-order perturbation process.
III.2 Low-energy effective Hamiltonian
To confirm the perturbative generation of the relevant interaction, I derive a simple effective Hamiltonian that governs the low-energy physics of the fermion chain (15). Among several options to derive such a low-energy effective Hamiltonian is to use the Schrieffer-Wolff canonical transformation Schrieffer and Wolff (1966); Slagle and Kim (2017). The generic theory is explained in Appendix A.1. Here, I briefly summarize the derivation. First, I perform a canonical transformation,
| (16) |
with an antiunitary operator . Two Hamiltonians and have one-to-one corresponding lists of eigenstates with exactly the same eigenenergies. Next, I perform a perturbative expanison by using a projection operator onto a low-energy subspace with and
| (17) |
where is a cutoff in the wave number and assumed as . Here, is an eigenstate of the unperturbed Hamiltonian, Eq. (15) for , with the total wave number . can explicitly be written as . The projection onto the low-energy subspace leads to the effective Hamiltonian
| (18) |
Choosing properly, I can simplify the perturbative expansion of the right hand side of Eq. (18). The effective Hamiltonian up to the second order of is then given by (see Appendix A.2)
| (19) |
Since the cutoff is small enough, the kinetic term of Eq. (19) can be linearized around the Fermi surface Giamarchi (2004). Creation operators at and are replaced to those of different species, which I denote as and , respectively. and refer to right movers and left movers of fermions. The low-energy Hamiltonian thus turns out to be
| (20) |
where is the Fermi velocity. Note that the last term of Eq. (19) was discarded in the linearized Hamiltonian (20) because the coefficient of is and thus negligibly small for . The Hamiltonian (20) is diagonalized in terms of Majorana fermions,
| (21) |
The second line of Eq. (20) becomes mass terms,
| (22) |
which indicate that these Majorana fermions have the excitation gap Shelton et al. (1996). Note that the gap does not reproduce the power law (4). This discrepancy of the power is attributed to interactions of fermions. I will come back to this point in Sec. IV.
The mass terms (22) are nothing but the bond alternation with Giamarchi (2004):
| (23) |
For comparison, the following is the twofold staggered field term in terms of fermions.
| (24) |
Here, I comment on effects of the canonical transformation (16) on observables. The canonical transformation also transforms an operator, say, , in the original model to
| (25) |
Note that one observes in experiments, not . Equations (23) and (24) refer to the latter. According to the generic framework of Appendix A.1, the operator can be expanded with ,
| (26) |
A relation is valid for . I can thus basically identify and but their small discrepancy, , would affect dynamics of spin chains (see Appendix. B).
III.3 Symmetries
I showed that the fourfold screw field yields the bond alternation instead of the twofold staggered field. Actually, the bond-centered inversion symmetry of the spin chain (3) forbids the twofold staggered field from emerging in the effective Hamiltonian (20).
The uniform spin chain is symmetric under two types of spatial inversions: the site-centered inversion and the bond-centered inversion . These spatial inversions act on spins as and . The twofold staggered field is invariant under but not under . On the other hand, the bond alternation and the fourfold screw field are invariant under but not under . In general, a low-energy effective Hamiltonian keeps the symmetries that the original Hamiltonian possesses. In this sense, the low-energy effective Hamiltonian of the spin chain (3) cannot have the twofold staggered field term that breaks the bond-centered inversion symmetry of the original Hamiltonian (14).
IV Interacting boson theory
The second-order perturbation turned out to give rise to the bond alternation in the low-energy effective Hamiltonian of the XY model in the screw field (14). However, the free spinless fermion theory does not explain the power-law behavior of the excitation gap. In this section, I present a simple theoretical explanation for the numerically found power law, incorporating the interaction of spinless fermions.
Discussions in the previous section prompt us to make an ansatz that the low-energy effective Hamiltonian of the HAFM model in the fourfold screw field (3) should be
| (27) |
Here, the effective bond alternation is characterized by the parameter .
Let us investigate whether the ansatz (27) explains numerical results. For small enough , one can bosonize the spin operator Affleck and Haldane (1987),
| (28) | ||||
| (29) |
where is the ladder operator. Coefficients , , depend on details of the lattice model and are thus nonuniversal. They are numerically estimated Hikihara and Furusaki (2004). The Hamiltonian is then bosonized as
| (30) |
Here, is the spinon velocity and the coefficient is a nonuniversal constant Takayoshi and Sato (2010); Hikihara et al. (2017). This bosonic field theory (30) is interacting but, fortunately, integrable Essler and Konik (2005).
The lowest-energy excitation gap of the sine-Gordon model (30) is exactly given by Lukyanov and Zamolodchikov (1997); Zamolodchikov (1995); Eßler (1999)
| (31) |
I thus find
| (32) |
The power shows an excellent agreement with the numerical estimation (4).
The sine-Gordon theory also explains the power-law behavior of the transverse dimer order (7). In terms of the sine-Gordon theory, the transverse dimer order is an average of the vertex operator Lukyanov and Zamolodchikov (1997),
| (33) |
It immediately follows from Eq. (32) that
| (34) |
The power also agrees excellenetly with the numerical estimation (7).
The bosonization approach predicts the same power law for the longitudinal dimer order. When I naively bosonizes the operator , I obtain
| (35) |
An operator-product expansion on the right hand side Cardy (1996) yields a more relevant interaction Starykh et al. (2005),
| (36) |
Here, is a nonuniversal constant and precisely estimated Takayoshi and Sato (2010); Hikihara et al. (2017). Note that and satisfy the following relation for small Takayoshi and Sato (2010); Hikihara et al. (2017),
| (37) |
which reflects the SU(2) symmetry of the exchange interaction. The bosonization formula (36) indicates
| (38) |
Nevertheless, the DMRG result (Fig. 4) implies Eq. (8). This discrepancy remains unclear unfortunately. This will be because the low-energy Hamiltonian fails to capture the uniform order (10) properly.
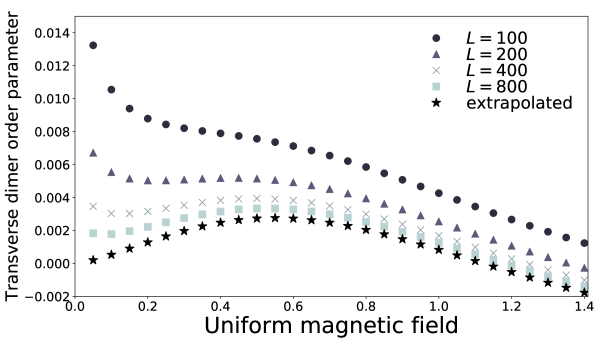
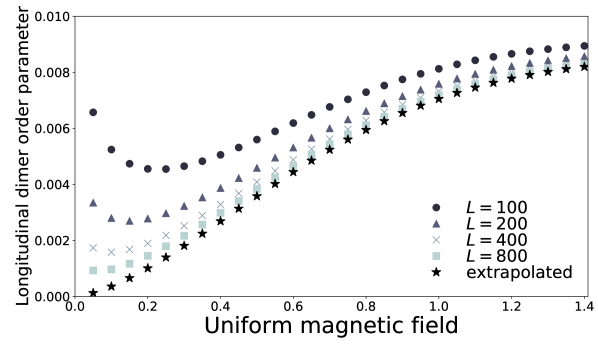
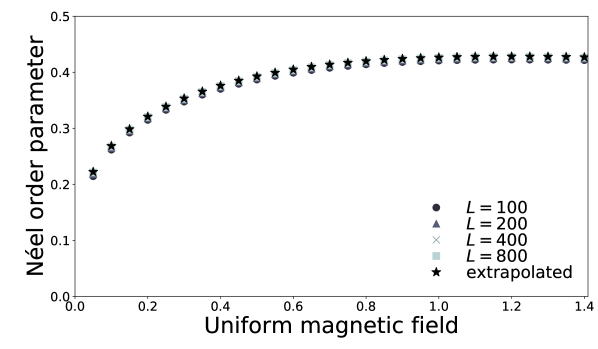
V Coexistence of Néel and dimer orders
V.1 Renormalization groups
On the basis of the fact that the fourfold screw field induces the transverse dimer order (34), here, I investigate the realistic case with and proportional to the uniform field . I assume two proportional coefficients
| (39) |
are both constant. DMRG results for the dimer order parameters and the Néel order parameter are shown in Figs. 6, 7, and 8 for .
To understand DMRG results, I replace the Hamiltonian (1) to the low-energy effective Hamiltonian,
| (40) |
with . I can immediately bosonize it.
| (41) |
where and . This complex Hamiltonian consists of two parts. The first line of Eq. (41) favors the gapless TL-liquid ground state for small . The second line represents potential terms of and that give rise to an excitation gap. In general, the scaling dimensions of and are and , respectively. Here, is a parameter called the Luttinger parameter that signifies strength of interactions Giamarchi (2004). The XY and the Heisenberg chains have and , respectively. In the latter case, two cosine interactions are equally relevant. Therefore, Néel and dimer orders can coexist in the ground state from the viewpoint of the renormalization group (RG).
The coupling constant of , whose bare value is , is increasing in the course of iterative RG transformations. follows the RG equation,
| (42) |
Here, characterizes the effective short-distance cutoff . Note that is the lattice spacing which was set to be unity. The RG transformation of Eq. (42) is terminated when reaches a correlation length of the lowest-energy excitation, .
Despite the same value of scaling dimensions, behaviors of the RG transformation of differ from that of . This is due to the Zeeman energy which competes with the transverse bond alternation . I can absorb the Zeeman energy in Eq. (41) into the kinetic term by shifting . The shift introduces an incommensurate oscillation to the transverse bond alternation term, . When the wave length is much longer than the short-distance cutoff , the incommensurate oscillation is negligible and then the RG equation of is simply
| (43) |
Otherwise, the incommensurate oscillation is rapid enough to eliminate :
| (44) |
The same argument can be found in Ref. Affleck and Oshikawa (1999).
Now I can classify into two cases the strong-coupling limit that the RG flow eventually reaches. (i) When , the coupling constants and grow equally following Eqs. (42) and (43) and eventually reach . Then the Néel and the dimer orders coexist in the ground state. (ii) When , the coupling constant vanishes because of the rapid incommensurate oscillation [Eq. (44)]. Then, the ground state has only the Néel order.
The correlation length and the wave length are easily compared. If , the gap becomes . When , the gap never exceeds , in other words, . Then the ground state does not have the dimer order. On the other hand, if is finite, the gap is a complex function of . Still, in the limit , the gap is reduced to the simple form of , which is much larger than . In other words, is valid and the first scenario comes true. Therefore, finite is necessary for the coexistence of the Néel and the dimer orders.
V.2 Non-Abelian bosonization
There is one remaining problem in the RG analysis on the coexistence of the Néel and the dimer orders. The transverse Néel order and the transverse dimer order seem to compete with each other since and are noncommutative. However, this competition is an artifact of the Abelian bosonization and these orders are cooperative Garate and Affleck (2010); Chan et al. (2017); Jin and Starykh (2017).
To avoid the artifact, I rewrite the Hamiltonian (40) as
| (45) |
where I assume finite . According to the RG analysis, the uniform Zeeman energy is negligible for finite . Here, I simply put from the beginning. Note that the second line of Eq. (45) yields only irrelevant interactions for the relation (37) and is discarded hereafter.
Instead of the Abelian bosonization, I employ the non-Abelian bosonization approach Affleck and Haldane (1987); Gogolin et al. (2004). In the non-Abelian bosonization language, the effective Hamiltonian (41) for is written as
| (46) |
Here, the spin operator is represented as
| (47) |
with currents and , a fundamental field , the Pauli matrices Affleck and Haldane (1987); Gogolin et al. (2004). The matrix is simply related to the U(1) bosons,
| (48) |
Since global rotations keep the excitation spectrum unchanged, I perform a global rotation in the spin space as . The rotation transforms the Hamiltonian (46) into
| (49) |
Translating it to the Abelian bosonizaion language, I can express this Hamiltonian as
| (50) |
with the coupling constant and the phase shift . The complex model (40) is thus reduced to the simple sine-Gordon model (50).
The incommensurate phase shift realizes the coexistence of the Néel order,
| (51) |
and the transverse dimer order (5). Their ground-state averages are given by
| (52) | ||||
| (53) |
For , the angle approaches . Therefore, at low fields , and are expected to follow power laws:
| (54) |
The power law (54) is qualitatively consistent with Figs. 6 and 8 at low fields. For weak magnetic fields, the longitudinal and the transverse dimer order parameters are almost equal. On the other hand, they are much smaller than the Néel order parameter . If we assume , the bosonized theory (50) predicts a ratio given by
| (55) |
The right hand side is approximately estimated as for the parameters used in DMRG. If , the ratio (55) is roughly consistent with the DMRG data of Figs. 6 and 8 for .
VI Experimental relevance
VI.1 [Cu(pym)(H2O)4]SiF H2O
The model (1) that I have dealt with so far is similar to a model proposed for the spin-chain compound with the following Hamiltonian: Liu et al. (2019)
| (56) |
where . There are three differences in two models (1) and (56): field directions, the weak exchange anisotropy, and the uniform DM interaction. In this section, I investigate effects of these differences one by one and discuss an experimental feasibility of the field-induced transverse dimer order.
VI.2 Field directions
In the model (56), the magnetic field , the twofold staggered field , and the fourfold screw field are applied in different directions. On the other hand, the model (1) has the uniform field and the fourfold screw field in the same direction. This difference in field directions is actually insignificant in low-energy physics for small . When the uniform DM interaction is absent (), the model (56) has the following low-energy effective Hamiltonian:
| (57) |
with . Relabeling the spins , I rewrite this Hamiltonian as
| (58) |
Since the last term of Eq. (58) is irrelevant, the bosonized Hamiltonian of Eq. (58) is given by
| (59) |
with . Except for the last term that comes from the exchange anisotropy, the Hamiltonian (59) is identical to the one (41) investigated in Sec. V.
VI.3 Exchange anisotropy
The bosonized effective Hamiltonian (59) shows that the small exchange anisotropy gives rise to the interaction. Though this interaction iteself can be marginally relevant at most in the RG sense, it is negligible in the presence of the much more relevant interaction .
VI.4 Uniform DM interaction
After all, the uniform DM interaction is the only significant difference in the models (1) and (56). The major effect of the uniform DM interaction is a chiral rotation. Let us resurrect the uniform DM intearction in the rotated effective Hamiltonian (58):
| (60) |
Here, the irrelevant term is already dropped. The uniform DM interaction itself is bosonized as Gangadharaiah et al. (2008)
| (61) |
with a nonuniversal constant . I employed the non-Abelian bosonization language (see Sec. V.2). The right hand side of Eq. (61) resembles the Zeeman energy,
| (62) |
While the Zeeman energy (62) is non-chiral (i.e. symmetric in the permutation of ), but the uniform DM interaction (61) is chiral. As far as only either the part or the part is concerned, I cannot distinguish the Zeeman energy and the uniform DM interaction.
A chiral rotation can combine the uniform DM interaction (61) and the Zeeman energy (62) Garate and Affleck (2010); Jin and Starykh (2017); Chan et al. (2017).
| (63) |
for . Here, the rotation is defined as
| (64) |
I assume that and are both positive. Then, the rotation leads to
| (65) |
with
| (66) |
if and take the following values,
| (67) | ||||
| (68) |
The chiral rotation transforms into
| (69) |
Effects of this chiral rotation on the low-energy Hamiltonian are explained in Appendix. B. Here, I show results only. The chirally rotated Hamiltonian then becomes
| (70) |
where and . Note that the chiral rotation (69) mixes the Néel and dimer orders. Though the right hand side of Eq. (70) is complex, its basic structure is the same as that of Eq. (41) in a sense that coupling constants and in the former follow the same RG equations as those for and in the latter. Following the argument in Sec. V.2, I obtain the Néel and dimer orders in the ground state (see Appendix B for details):
| (71) | ||||
| (72) |
where the coupling constant and the angle are defined in Eqs. (133) and (134). In analogy with Eq. (54), I obtain
| (73) |
at low fields .
In short, the uniform DM interaction causes the chiral rotation that mixes the Néel and the transverse dimer orders if the following condition is met.
| (74) |
When , the condition (74) is trivially satisfied for small . However, the inequality (74) can be violated at extremely small magnetic fields .
Let me comment on effects of the uniform DM interaction on electron spin resonance (ESR). In one-dimensional quantum spin systems, the uniform DM interaction splits the ESR peak that corresponds to the Zeeman energy [Eqs. (61) and (62)] Povarov et al. (2011); Furuya (2017). In some cases, the DM interaction changes selection rules of ESR and yields an additional resonance that occurs at a frequency away from the Zeeman energy Oshikawa and Affleck (2002); Furuya and Oshikawa (2012); Ozerov et al. (2015); Zhao et al. (2003); Lou et al. (2005).
The experiment Liu et al. (2019) on found that ESR peaks of this compound exhibit unconventional power-law dependence on the magnetic field. A part of this unconventional behavior is attributed to the chiral rotation and the complex dependence of the coupling constant on the magnetic field. A derivation of ESR selection rules is described in Appendix. B. Here, I simply summarize the result. Elementary excitations of the sine-Gordon theory are a soliton, an antisoliton, and their bound states, breathers. Let us represent these excitation gaps by , where can be the soliton mass or the breather mass. ESR in the model (60) occurs when the frequency of the applied microwave satisfies
| (75) |
or
| (76) |
These resonance frequencies are close to neither the Zeeman energy nor the typical gap, , in quantum spin chains with the twofold staggered field Dender et al. (1997); Zvyagin et al. (2004); Umegaki et al. (2009). In particular, the latter resonance frequency (76) approaches in the limit Povarov et al. (2011); Furuya (2017).
VI.5 (Ba/Sr)Co2V2O8
I can find other quantum spin chain compounds with the fourfold screw symmetry such as Faure et al. (2018); Kimura et al. (2007) and Wang et al. (2015); Bera et al. (2017). Unlike , these compounds have Ising-like exchange interactions. As I already showed, the SU(2) symmetry of the exchange interaction is essential for the coexistence of the Néel and the transverse dimer orders. The strong enough Ising anisotropy ruins the coexistence and thus makes the fourfold screw field insignificant. Thus far, most experimental results on these compounds are well understood with models without the fourfold screw field Faure et al. (2018); Kimura et al. (2007); Wang et al. (2015); Bera et al. (2017) though some ESR peaks can be attributed to the presence of the fourfold screw field Kimura et al. (2007).
VII Summary
I discussed the novel type of field-induced gap phenomena, field-induced dimer orders in quantum spin chains. The fourfold screw field with the bond-centered inversion symmetry introduces perturbatively the effective bond alternation to the spin chain. In analogy with the twofold staggered field, the fourfold screw field, which breaks the one-site translation symmetry, gives rise to the excitation gap from the ground state to the excited states.
In the first part of the paper, I applied the fourfold screw field solely to quantum spin chains. The field-induced excitation gap by turned out to show a distinctive power law from that by the twofold staggered field . The gap is proportional to for the fourfold screw field instead of for the twofold staggered field Oshikawa and Affleck (1997); Affleck and Oshikawa (1999). The power law was predicted from the quantum field theory and consistent with the numerical results (Fig. 2). The field theory also gave the explanation on the power law of the transverse dimer order (7), though it failed for the longitudinal one (8) somehow.
Next, I applied the uniform field, the twofold staggered field, and the fourfold screw field simultaneously to HAFM chains. The SU(2) symmetry of the exchange interaction turned out to make the coexistence of the Néel and dimer order possible in the ground state. The coexistence of these orders are nontrivial and already interesting Garate and Affleck (2010); Jin and Starykh (2017); Chan et al. (2017). More interestingly, the dimer order grows in association with the uniform magnetic field (Figs. 6, 7, and 8). The coexistent growth of the Néel and the dimer orders were numerically found and supported by the effective field theory.
Last but not least, I discussed the relevance of my model to experimental studies, in particular, Ref. Liu et al. (2019) on . There are three differences in the model for and the model Hamiltonian (1), or equivalently Eq. (40), that I dealt with in this paper. They are field directions, the weak exchange anisotropy, and the uniform DM interaction. In Sec. VI, I discussed that all the three differences do not interfere with the field-induced growth of the Néel and the dimer orders. However, the uniform DM interaction may cause nontrivial effects on dynamics of the spin chain such as ESR. In the presence of the uniform DM interaction, increase of the magnetic field rotates chirally the spin chain. This chiral rotation affects selection rules of the electron spin resonance. It will be interesting to test experimentally the coexistence of the Néel and the dimer orders in spin-chain compounds with the fourfold screw symmetry.
Acknowledgments
I thank Akira Furusaki, Yusuke Horinouchi, and Tsutomu Momoi for useful discussions.
Appendix A Derivation of effective Hamiltonian
This section is devoted to derivation of the low-energy effective Hamiltonian discussed in Sec. III.2 as generically as possible.
A.1 Framework
I consider a Hamiltonian whose eigenstates are exactly known.
| (77) |
I can assume for without loss of generality. Adding a perturbation , I modify the Hamiltonian to
| (78) |
where is a small parameter that controls the perturbation expansion. At low energies, effects of the perturbation can be taken into account as a form of the effective Hamiltonian . The effective Hamiltonian can be easily obtained up to the second order.
To derive the low-energy effective Hamiltonian , I focus on the low-energy eigenstates with for . One can take or . The latter case is useful for later application to the free spinless fermion chain. When applying to the free spinless fermion chain, I assume for , where is the Fermi energy and is an energy cutoff.
Each eigenstate defines a projection operator into that eigenstate. satisfies . An operator ,
| (79) |
then projects an arbitrary state into the subspace spanned by the eigenstates. projects any state into the supplementary space.
The key idea is to perform a canonical transformation of the Schrieffer-Wolff type on the Hamiltonian Schrieffer and Wolff (1966); Bravyi et al. (2011),
| (80) |
where is anti-Hermitian so that is unitary. The Schrieffer-Wolff formulation is useful in quantum spin systems Slagle and Kim (2017). Here, I briefly review the derivation of the effective Hamiltonian based on the Schrieffer-Wolff formulation to make the paper self-contained.
Two Hamiltonians and have one-to-one corresponding lists of eigenstates with exactly the same eigenenergies. An appropriate choice of simplifies the transformed Hamiltonian . I expand and determine .
| (81) |
In the last line, I expanded around :
| (82) |
is determined so that Slagle and Kim (2017)
| (83) |
I solve Eq. (83) at each order of . At the first order, Eq. (83) leads to
| (84) |
The anti-Hermitian that satisfies Eq. (84) is given by Slagle and Kim (2017)
| (85) |
The second order of Eq. (83),
| (86) |
with being
| (87) |
is similar to the first-order equation (84). The solution is immediately obtained.
| (88) |
I am now ready to write down the low-energy effective Hamiltonian,
| (89) |
up to the second order of . First three terms for are shown below.
| (90) | ||||
| (91) |
where holds true for . The second-order term is given by
| (92) |
One can simplify the last line:
| (93) |
This leads to the following effective Hamiltonian of the second order of :
| (94) |
A.2 Application to spinless fermion chains
Here I apply the generic formalism of the effective Hamiltonian to the spinless fermion chain (15). The low-energy region is defined as Eq. (17). The operator is redefined as a projection operator onto the subspace (17) of the reciprocal space [Eq. (17)]. acts on as follows. for and otherwise. acts on in the same manner. acts on and as . In applying the generic Schrieffer-Wolff formulation to the XY chain (15), I regard and of Eq. (78) as
| (95) | ||||
| (96) |
The effective Hamiltonian (94) is then given by
| (97) |
where is the second-order term. Note that the first-order term vanishes trivially because for any or thanks to the assumption . The second-order correction is calculated as follows.
| (98) |
. One can simplify these projections. Since the Fermi surface is located at , the projection gives back itself for and zero otherwise. In the end, I obtain
| (99) |
The first line of Eq. (99) is the the bond alternation for Giamarchi (2004) and the second line is a small correction to the Zeeman energy.
Appendix B Electron spin resonance
Here, I describe how the uniform DM interaction affects the ESR spectrum. In this Appendix, I start with the spin chain model (60) with . Namely, I consider the spin chain with the following Hamiltonian:
| (100) |
The ESR spectrum is obtained from the part of the dynamical correlation function for . I can obtain selection rules of the ESR spectrum by relating the part of the spin, , where
| (101) |
to the boson fields of the effective field theory.
Let us bosonize the spin chain by using the non-Abelian bosonization formula Gogolin et al. (2004); Affleck and Haldane (1987),
| (102) |
where , , and are defined as
| (103) | ||||
| (104) | ||||
| (105) | ||||
| (106) | ||||
| (107) |
Here and at a position and a time are related to and through
| (108) | ||||
| (109) |
The derivatives and are abbreviations of the following derivatives.
| (110) | ||||
| (111) |
Boson fields and are subject to equal-time commutation relations,
| (112) |
with a step function,
| (116) |
for are thus bosonized as Mudry et al. (2019)
| (117) | ||||
| (118) | ||||
| (119) |
In the non-Abelian bosonization laugage, the Hamiltonian (100) is expressed as
| (120) |
In order to combine terms on the second line, I perform the chiral rotation (63). The chiral rotation turns the Hamiltonian into
| (121) |
, , and are related to U(1) bosons and in analogy with , , and . I can eliminate the Zeeman energy by shifting
| (122) | ||||
| (123) |
This shift affect , , and as follows.
| (124) | ||||
| (125) | ||||
| (126) | ||||
| (127) | ||||
| (128) |
The shift introduces incommensurate oscillations to the Hamiltonian (121). Here, I assume an inequality,
| (129) |
This condition guarantees that the incommensurate oscillation is negligible (cf. Secs. V.1 and V.2). At low fields , this inequality reads
| (130) |
Under this condition (130), I can safely discard the incommensurate oscillation with the wave number in the Hamiltonian. Note that must be kept in the relations between operators and quantum fields. The Hamiltonian is thus given by
| (131) |
Here, as I did in Sec. V.2, I rotate the system by around the axis: . The -rotated Hamiltonian finally becomes simple.
| (132) |
with
| (133) | ||||
| (134) |
Let us relate the spin in the original coordinate frame to the and fields in Eq. (132), recalling all the chiral and nonchiral rotations performed.
| (135) | ||||
| (136) | ||||
| (137) |
Note that the rotation was performed on the rightmost hand sides of Eqs. (135), (136), and (137). The transverse dimer order is expressed as
| (138) |
I can confirm that the spin chain model (100) has the Néel and dimer orders.
| (139) | ||||
| (140) |
A list of low-energy excitations created by operators on the rightmost hand sides of Eqs (135), (136), and (137) is available Lukyanov (1997); Lukyanov and Zamolodchikov (2001); Babujian and Karowski (2002). Vertex operator operators create solitons and antisolitons with an excitation energy,
| (141) | ||||
| (142) |
Other vertex operators create breathers, bound states of a soliton and an antisoliton, with an excitation energy,
| (143) | ||||
| (144) |
Here, . The index takes values of .
I can now predict the ESR frequency caused by for . For example, in yields the resonance peak at
| (145) |
Though in the limit, this resonance frequency follows a simple power law , it will be a complicated function of in general. Another interesting term is in . This term yields resonance peaks at
| (146) |
where can be or for .
The selection rule is also affected by the canonical transformation (80). Precisely speaking, the left hand sides of Eqs. (135), (136), and (137) should be denoted as for . Here, is defined as
| (147) |
and create particle-hole excitations with and , respectively, when applied to the TL-liquid ground state. Such a mixing of different wave numbers will allow ESR to detect and excitations. Excitations with can be read from the staggered terms of Eqs. (135), (136), and (137), which are similar to those with .
References
- Lieb et al. (1961) Elliott Lieb, Theodore Schultz, and Daniel Mattis, “Two soluble models of an antiferromagnetic chain,” Annals of Physics 16, 407 – 466 (1961).
- Affleck and Haldane (1987) Ian Affleck and F. D. M. Haldane, “Critical theory of quantum spin chains,” Phys. Rev. B 36, 5291–5300 (1987).
- Furuya and Oshikawa (2017) Shunsuke C. Furuya and Masaki Oshikawa, “Symmetry Protection of Critical Phases and a Global Anomaly in Dimensions,” Phys. Rev. Lett. 118, 021601 (2017).
- Giamarchi (2004) T. Giamarchi, Quantum Physics in One Dimension (Oxford University Press, Oxford, 2004).
- Metlitski and Thorngren (2018) Max A. Metlitski and Ryan Thorngren, “Intrinsic and emergent anomalies at deconfined critical points,” Phys. Rev. B 98, 085140 (2018).
- Dender et al. (1997) D. C. Dender, P. R. Hammar, Daniel H. Reich, C. Broholm, and G. Aeppli, “Direct Observation of Field-Induced Incommensurate Fluctuations in a One-Dimensional Antiferromagnet,” Phys. Rev. Lett. 79, 1750–1753 (1997).
- Zvyagin et al. (2004) S. A. Zvyagin, A. K. Kolezhuk, J. Krzystek, and R. Feyerherm, “Excitation Hierarchy of the Quantum Sine-Gordon Spin Chain in a Strong Magnetic Field,” Phys. Rev. Lett. 93, 027201 (2004).
- Umegaki et al. (2009) Izumi Umegaki, Hidekazu Tanaka, Toshio Ono, Hidehiro Uekusa, and Hiroyuki Nojiri, “Elementary excitations of the one-dimensional antiferromagnet in a magnetic field and quantum sine-Gordon model,” Phys. Rev. B 79, 184401 (2009).
- Oshikawa and Affleck (1997) Masaki Oshikawa and Ian Affleck, “Field-Induced Gap in Antiferromagnetic Chains,” Phys. Rev. Lett. 79, 2883–2886 (1997).
- Affleck and Oshikawa (1999) Ian Affleck and Masaki Oshikawa, “Field-induced gap in Cu benzoate and other antiferromagnetic chains,” Phys. Rev. B 60, 1038–1056 (1999).
- Furuya and Oshikawa (2012) Shunsuke C. Furuya and Masaki Oshikawa, “Boundary Resonances in Antiferromagnetic Chains Under a Staggered Field,” Phys. Rev. Lett. 109, 247603 (2012).
- Furuya et al. (2011) Shunsuke C. Furuya, Masaki Oshikawa, and Ian Affleck, “Semiclassical approach to electron spin resonance in quantum spin systems,” Phys. Rev. B 83, 224417 (2011).
- Faure et al. (2018) Quentin Faure, Shintaro Takayoshi, Sylvain Petit, Virginie Simonet, Stéphane Raymond, Louis-Pierre Regnault, Martin Boehm, Jonathan S White, Martin Månsson, Christian Rüegg, et al., “Topological quantum phase transition in the Ising-like antiferromagnetic spin chain BaCo2V2O8,” Nature Physics 14, 716 (2018).
- Takayoshi et al. (2018) Shintaro Takayoshi, Shunsuke C. Furuya, and Thierry Giamarchi, “Topological transition between competing orders in quantum spin chains,” Phys. Rev. B 98, 184429 (2018).
- Kimura et al. (2013) Shojiro Kimura, Koichi Okunishi, Masayuki Hagiwara, Koichi Kindo, Zhangzhen He, Tomoyasu Taniyama, Mitsuru Itoh, Keiichi Koyama, and Kazuo Watanabe, “Collapse of Magnetic Order of the Quasi One-Dimensional Ising-Like Antiferromagnet BaCo2V2O8 in Transverse Fields,” Journal of the Physical Society of Japan 82, 033706 (2013).
- Liu et al. (2019) J. Liu, S. Kittaka, R. D. Johnson, T. Lancaster, J. Singleton, T. Sakakibara, Y. Kohama, J. van Tol, A. Ardavan, B. H. Williams, S. J. Blundell, Z. E. Manson, J. L. Manson, and P. A. Goddard, “Unconventional Field-Induced Spin Gap in an Chiral Staggered Chain,” Phys. Rev. Lett. 122, 057207 (2019).
- (17) ITensor Library (version 2.0.11) http://itensor.org .
- Schrieffer and Wolff (1966) J. R. Schrieffer and P. A. Wolff, “Relation between the Anderson and Kondo Hamiltonians,” Phys. Rev. 149, 491–492 (1966).
- Slagle and Kim (2017) Kevin Slagle and Yong Baek Kim, “Fracton topological order from nearest-neighbor two-spin interactions and dualities,” Phys. Rev. B 96, 165106 (2017).
- Shelton et al. (1996) D. G. Shelton, A. A. Nersesyan, and A. M. Tsvelik, “Antiferromagnetic spin ladders: Crossover between spin S=1/2 and S=1 chains,” Phys. Rev. B 53, 8521–8532 (1996).
- Hikihara and Furusaki (2004) T. Hikihara and A. Furusaki, “Correlation amplitudes for the spin- chain in a magnetic field,” Phys. Rev. B 69, 064427 (2004).
- Takayoshi and Sato (2010) Shintaro Takayoshi and Masahiro Sato, “Coefficients of bosonized dimer operators in spin- chains and their applications,” Phys. Rev. B 82, 214420 (2010).
- Hikihara et al. (2017) Toshiya Hikihara, Akira Furusaki, and Sergei Lukyanov, “Dimer correlation amplitudes and dimer excitation gap in spin- XXZ and Heisenberg chains,” Phys. Rev. B 96, 134429 (2017).
- Essler and Konik (2005) Fabian H. L. Essler and Robert M. Konik, “Application of massive integrable quantum field theories to problems in condensed matter physics,” in From Fields to Strings: Circumnavigating Theoretical Physics: Ian Kogan Memorial Collection (In 3 Volumes) (World Scientific, 2005) pp. 684–830.
- Lukyanov and Zamolodchikov (1997) Sergei Lukyanov and Alexander Zamolodchikov, “Exact expectation values of local fields in the quantum sine-gordon model,” Nuclear Physics B 493, 571 – 587 (1997).
- Zamolodchikov (1995) Al. B. Zamolodchikov, “Mass scale in the sine–gordon model and its reductions,” International Journal of Modern Physics A 10, 1125–1150 (1995).
- Eßler (1999) Fabian H. L. Eßler, “Sine-gordon low-energy effective theory for copper benzoate,” Phys. Rev. B 59, 14376–14383 (1999).
- Cardy (1996) John Cardy, Scaling and renormalization in statistical physics, Vol. 5 (Cambridge university press, 1996).
- Starykh et al. (2005) Oleg A. Starykh, Akira Furusaki, and Leon Balents, “Anisotropic pyrochlores and the global phase diagram of the checkerboard antiferromagnet,” Phys. Rev. B 72, 094416 (2005).
- Garate and Affleck (2010) Ion Garate and Ian Affleck, “Interplay between symmetric exchange anisotropy, uniform Dzyaloshinskii-Moriya interaction, and magnetic fields in the phase diagram of quantum magnets and superconductors,” Phys. Rev. B 81, 144419 (2010).
- Chan et al. (2017) Yang-Hao Chan, Wen Jin, Hong-Chen Jiang, and Oleg A. Starykh, “Ising order in a magnetized Heisenberg chain subject to a uniform Dzyaloshinskii-Moriya interaction,” Phys. Rev. B 96, 214441 (2017).
- Jin and Starykh (2017) Wen Jin and Oleg A. Starykh, “Phase diagram of weakly coupled Heisenberg spin chains subject to a uniform Dzyaloshinskii-Moriya interaction,” Phys. Rev. B 95, 214404 (2017).
- Gogolin et al. (2004) Alexander O Gogolin, Alexander A Nersesyan, and Alexei M Tsvelik, Bosonization and strongly correlated systems (Cambridge university press, 2004).
- Gangadharaiah et al. (2008) Suhas Gangadharaiah, Jianmin Sun, and Oleg A. Starykh, “Spin-orbital effects in magnetized quantum wires and spin chains,” Phys. Rev. B 78, 054436 (2008).
- Povarov et al. (2011) K. Yu. Povarov, A. I. Smirnov, O. A. Starykh, S. V. Petrov, and A. Ya. Shapiro, “Modes of magnetic resonance in the spin-liquid phase of ,” Phys. Rev. Lett. 107, 037204 (2011).
- Furuya (2017) Shunsuke C. Furuya, “Angular dependence of electron spin resonance for detecting the quadrupolar liquid state of frustrated spin chains,” Phys. Rev. B 95, 014416 (2017).
- Oshikawa and Affleck (2002) Masaki Oshikawa and Ian Affleck, “Electron spin resonance in antiferromagnetic chains,” Phys. Rev. B 65, 134410 (2002).
- Ozerov et al. (2015) M. Ozerov, M. Maksymenko, J. Wosnitza, A. Honecker, C. P. Landee, M. M. Turnbull, S. C. Furuya, T. Giamarchi, and S. A. Zvyagin, “Electron spin resonance modes in a strong-leg ladder in the tomonaga-luttinger liquid phase,” Phys. Rev. B 92, 241113 (2015).
- Zhao et al. (2003) J. Z. Zhao, X. Q. Wang, T. Xiang, Z. B. Su, and L. Yu, “Effects of the Dzyaloshinskii-Moriya Interaction on Low-Energy Magnetic Excitations in Copper Benzoate,” Phys. Rev. Lett. 90, 207204 (2003).
- Lou et al. (2005) Jizhong Lou, Changfeng Chen, Jize Zhao, Xiaoqun Wang, Tao Xiang, Zhaobin Su, and Lu Yu, “Midgap States in Antiferromagnetic Heisenberg Chains with a Staggered Field,” Phys. Rev. Lett. 94, 217207 (2005).
- Kimura et al. (2007) S. Kimura, H. Yashiro, K. Okunishi, M. Hagiwara, Z. He, K. Kindo, T. Taniyama, and M. Itoh, “Field-Induced Order-Disorder Transition in Antiferromagnetic Driven by a Softening of Spinon Excitation,” Phys. Rev. Lett. 99, 087602 (2007).
- Wang et al. (2015) Zhe Wang, M. Schmidt, A. K. Bera, A. T. M. N. Islam, B. Lake, A. Loidl, and J. Deisenhofer, “Spinon confinement in the one-dimensional Ising-like antiferromagnet ,” Phys. Rev. B 91, 140404 (2015).
- Bera et al. (2017) A. K. Bera, B. Lake, F. H. L. Essler, L. Vanderstraeten, C. Hubig, U. Schollwöck, A. T. M. N. Islam, A. Schneidewind, and D. L. Quintero-Castro, “Spinon confinement in a quasi-one-dimensional anisotropic heisenberg magnet,” Phys. Rev. B 96, 054423 (2017).
- Bravyi et al. (2011) Sergey Bravyi, David P. DiVincenzo, and Daniel Loss, “Schrieffer–wolff transformation for quantum many-body systems,” Annals of Physics 326, 2793 – 2826 (2011).
- Mudry et al. (2019) Christopher Mudry, Akira Furusaki, Takahiro Morimoto, and Toshiya Hikihara, “Quantum phase transitions beyond landau-ginzburg theory in one-dimensional space revisited,” Phys. Rev. B 99, 205153 (2019).
- Lukyanov (1997) Sergei Lukyanov, “Form Factors of Exponential Fields in the Sine–Gordon Model,” Modern Physics Letters A 12, 2543–2550 (1997).
- Lukyanov and Zamolodchikov (2001) Sergei Lukyanov and Alexander Zamolodchikov, “Form factors of soliton-creating operators in the sine-Gordon model,” Nuclear Physics B 607, 437 – 455 (2001).
- Babujian and Karowski (2002) H Babujian and M Karowski, “Sine-Gordon breather form factors and quantum field equations,” Journal of Physics A: Mathematical and General 35, 9081–9104 (2002).