Fermi Velocity Dependent Critical Current in Ballistic Bilayer Graphene Josephson Junctions

Ballistic graphene Josephson junctions (GJJs) have been widely utilized as a platform to study various interesting physics that arise at low temperatures from interactions between superconductors and normal metals [1, 2, 3, 4, 5, 6]. The ballistic superconductor-normal metal-superconductor Josephson junction (SNSJJ) hosts Andreev bound states (ABS) which carry supercurrents across the normal region of the JJ; a disorder-free weak link and high transparency at the SN interface are necessary. Hexagonal Boron-Nitride (hBN) encapsulated graphene as the weak link enables highly transparent contacts at the interface whilst keeping graphene clean throughout the fabrication process [7]. Here, we study proximitized, ballistic, bilayer graphene Josephson junctions (BGJJs). Bilayer graphene devices (in contrast to monolayer) allow extra potential tunability via a non-linear dispersion relation, applied displacement field, or lattice rotation [6].
The critical current ($I_{C}$) of SNSJJ in the intermediate-to-long regime, where the junction length (L) $\geq$ superconducting coherence length ($\xi_{0}$), scales with temperature (T) as $I_{C}=exp(-k_{B}T/\delta E)$. Here, $\delta E=\hbar\nu_{F}/2\pi L$, an energy scale related to ABS level spacing [8, 9, 10, 11, 12]. Note that in the intermediate regime ($L\approx\xi_{0}$) $\delta E$ is found to be suppressed [4]. A previous study of GJJs found that in this regime, the relation is held more precisely when $\xi$ was taken into account along with L, that is: $\delta E=\hbar\nu_{F}/2\pi(L+\xi)$ [8]. Monolayer graphene displays a linear dispersion relation, which results in a constant fermi velocity ($\nu_{F0}$). Thus, in ballistic GJJs, $\delta E$ remains independent of the carrier density. In comparison, bilayer graphene displays a quadratic dispersion relation at low energies. In BGJJs we studied, a back-gate voltage ($V_{G}$) controls the carrier density; and $\delta E$ dependence on $V_{G}$ is observed. Using $\delta E$, we extract Fermi velocity in bilayer graphene: it is seen that $\nu_{F}$ increases with $V_{G}$, and saturates to the constant value, $\nu_{F0}$, of the monolayer graphene.
Our device consists of a series of four terminal Josephson junctions (on $\mathrm{S}iO_{2}/Si$ substrate) made with hBN encapsulated bilayer graphene contacted by Molybdenum-Rhenium (MoRe) electrodes. Bilayer graphene is obtained via the standard exfoliation method. It is then encapsulated in hexagonal Boron-Nitride using the dry transfer method [13]. MoRe of $80$ nm thickness is deposited via DC magnetron sputtering. The resulting device has four junctions of lengths $400$ nm, $500$ nm, $600$ nm, and $700$ nm. The width of the junctions is $4~{}\mu$m. The device is cooled in a Leiden cryogenics dilution refrigerator operated at temperatures above $1$ K, and the measurements were performed using the standard four-probe lock-in method. A gate voltage $V_{G}$ is applied to the $Si$ substrate with the oxide layer acting as a dielectric, which allows modulation of the carrier density.[14, 15, 4, 8, 16, 17].

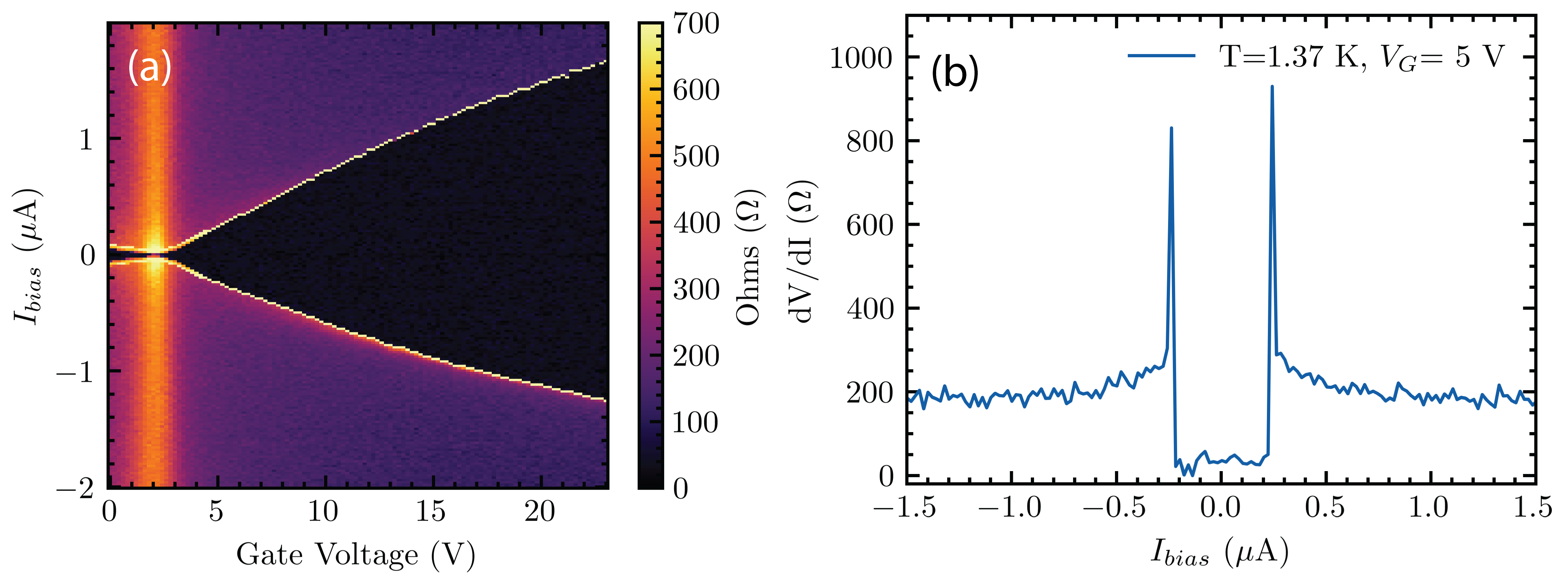
Fig. 1(a) displays the differential resistance ($dV/dI$) map of the $400$ nm junction at $T=1.37$ K; we see zero resistance (black region) across all applied $V_{G}$ indicating the presence of supercurrent. As the bias current $I_{bias}$ is swept from negative to positive values, the junction first reaches its superconducting state at a value $|I_{bias}|=I_{R}$, known as re-trapping current. Then, as $|I_{bias}|$ is increased to higher positive values, the junction transitions to the normal state at $|I_{bias}|=I_{S}$, known as switching current. Fig. 1(a) shows that the junction can sustain larger region of critical current as we modulate the carrier density to higher values via $V_{G}$. Fig. 1(b) displays a line plot extracted from the $dV/dI$ map which shows hysteresis in $I_{R}$ and $I_{S}$. This is a commonly observed phenomenon in underdamped junctions [18, 14], or can also be attributed self-heating [15, 19, 16]. The critical current $I_{C}$ of the junction is approximately $I_{S}$ as found in switching statistics measurements, discussed in previous publications [8, 20, 21, 22].
Extracting the critical current $I_{C}$ from the differential maps for different temperatures, we can see that $I_{C}$ falls exponentially with inverse $T$ (Fig. 2c) We also extract conductance of the junction in the normal regime ($I_{Bias}\gg I_{C}$). Fig. 2(b) shows this conductance ($G$) for $400$ nm junction device. We find that conductance $G$ scales as the square-root of $V_{G}$ as seen from the fit (blue curve). However, due to large contact resistance ($R_{C}$) of the device, the measured conductance $G$ is suppressed compared to the ballistic limit expectation. Therefore, to demonstrate the ballistic nature of the device, we present normal resistances ($R_{N}$) of junctions of length $500$ nm, $600$ nm, and $700$ nm (Fig. 2(c) inset). Taking into account $R_{C}$, we plot extracted values versus $V_{G}$ from the Dirac point. The inset plot shows that the $R_{N}-R_{C}$ values are independent of junction length, which demonstrates the ballistic nature of the devices.

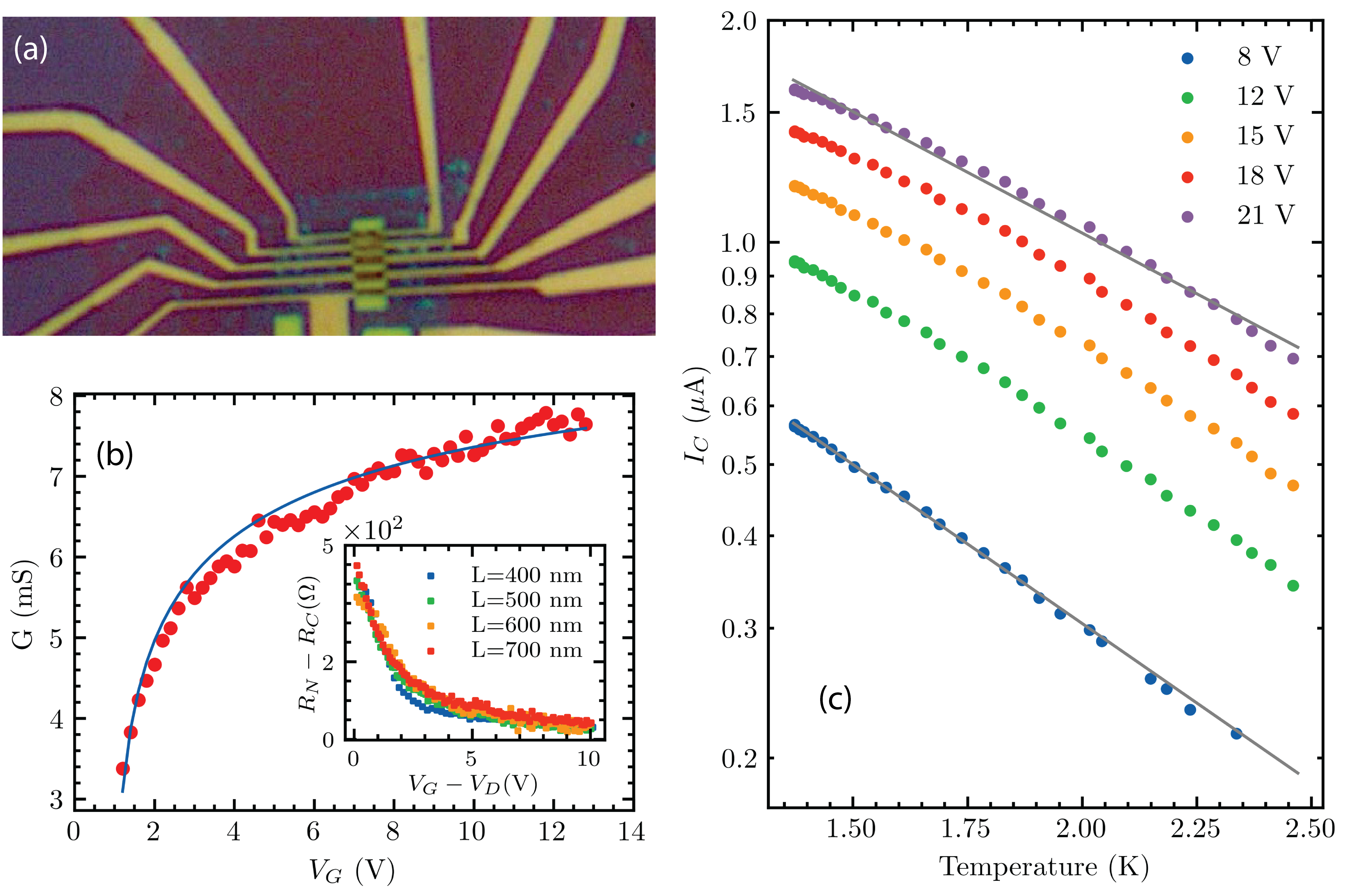
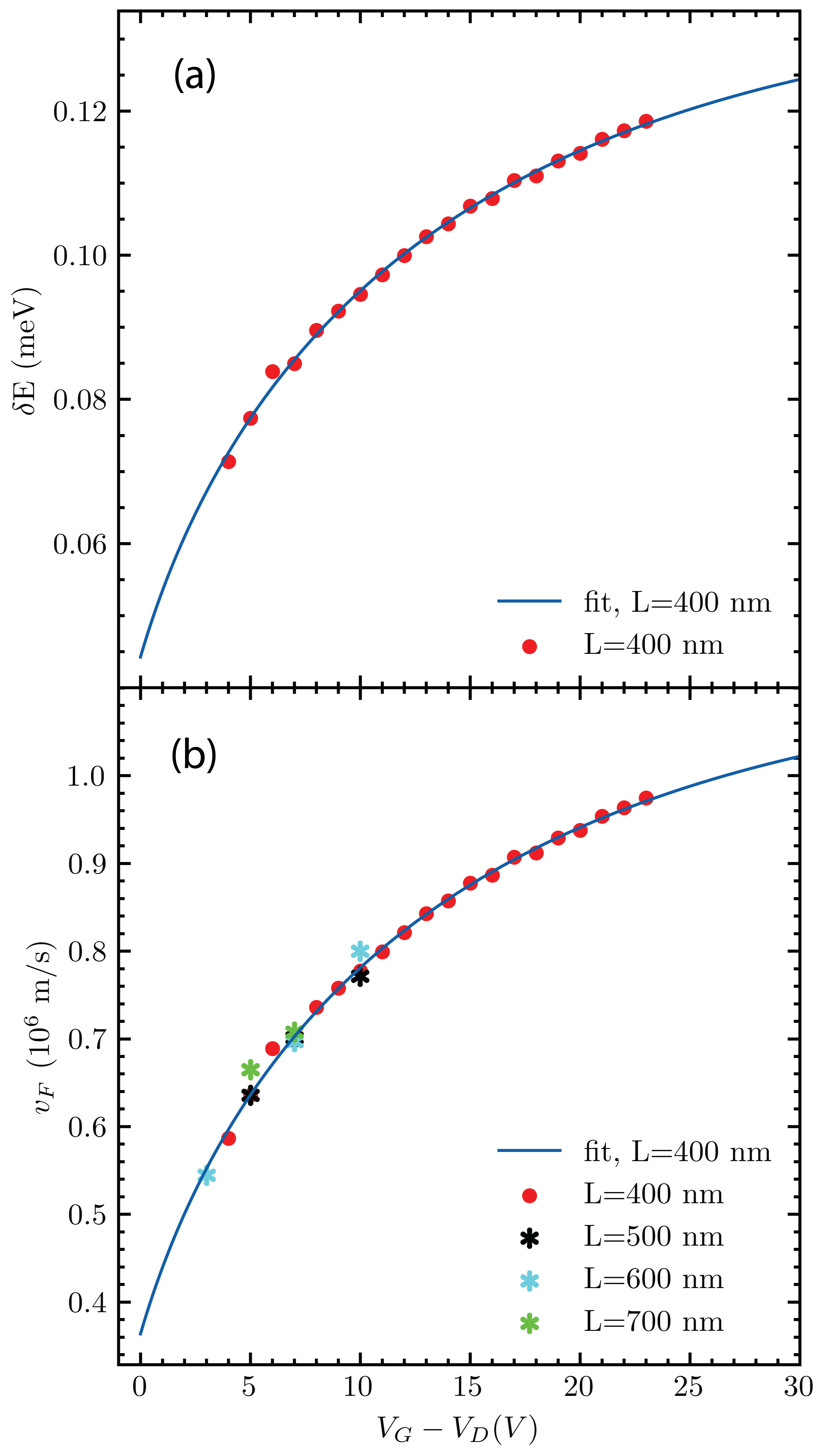
To extract $\delta E$ of the junction, we go to the discussion of $I_{C}$ vs temperature trends in Fig. 2(c). Here the y-axis is plotted in log scale. From the slope of curves $\mathrm{Log}(I_{C})=-(k_{B}/\delta E)T$ for each gate, one can extract $\delta E$ versus $V_{G}$ (plotted in Fig. 3a). Unlike for the case of monolayer graphene, a clear dependence on $V_{G}$ is seen. The energy $\delta E$ scales linearly with Fermi Velocity $v_{F}$ (Fig. 3b). Note that calculating $v_{F}$ from $\delta E$ for junctions in the intermediate regime requires knowledge of the superconducting coherence length $\xi$. In our case $\xi$ is obtained from the fit discussed below.
We now compare the experimentally obtained $\delta E$ (and $v_{F}$) to the theoretical expectation. Assuming a quadratic dispersion relation $\mathcal{E}_{F}=\frac{\hbar^{2}k^{2}_{F}}{2m^{*}}$, it follows that the expression for the Fermi velocity is: $v_{F}=\sqrt{\frac{2E_{F}}{m^{*}}}$ [23, 24, 25]. The Fermi Energy $\mathcal{E}_{F}$ for bilayer graphene scales as: $\mathcal{E}_{F}=\frac{\hbar^{2}\pi|n|}{2m^{*}}$. With $m^{*}$ being the effective mass of electrons in graphene. The carrier concentration $n$, controlled by the applied gate voltage $V_{G}$, is given by $n=\frac{V_{G}-V_{d}}{e}C_{Total}$ with $V_{d}$ as the gate voltage at the Dirac point. The total capacitance $C_{Total}$ is a combination of quantum capacitance $C_{q}$ and gate oxide capacitance $C_{ox}$: $C_{Total}=\left[\frac{1}{C_{ox}}+\frac{1}{C{q}}\right]^{-1}$. The quantum capacitance $C_{q}$ for bilayer graphene is determined by $C_{q}=\frac{2e^{2}m^{*}}{\pi\hbar^{2}}$. The gate oxide capacitance per unit area is $C_{ox}=\frac{\epsilon_{0}\epsilon_{r}}{d}$, where $\epsilon_{0}$ is vacuum permittivity, $\epsilon_{r}$ is the relative permittivity of the oxide, and $d$ is oxide layer thickness. For Silicon oxide gate with $d=300$ nm we get $C_{ox}\approx 115\mu F/m^{2}$. Thus, the full expression for the Fermi velocity $v_{F}$ is:

$\displaystyle v_{F}=\hbar\sqrt{\frac{2\pi\epsilon_{0}\epsilon_{r}e|V_{G}-V_{d}|}{m^{*}(2de^{2}m^{*}+\pi\epsilon_{0}\epsilon_{r}\hbar^{2})}}$

(1)

Note that the effective mass $m*$ typically ranges from $0.024~{}m_{e}$ to $0.058~{}m_{e}$ for $1*10^{12}\sim 4*10^{12}$ carriers/$cm^{2}$ [26], where $m_{e}$ is the electron rest mass. Moreover, since $m*$ has a carrier concentration dependence, we assume a linear shift of $m*$ with the gate voltage as: $m^{*}=m_{i}+dm(V_{G}-V_{d})$ [26].
Experimental data provides us with the following: $\delta E(V_{G})=\frac{\hbar}{2\pi(L+\xi)}v_{F}$. To fit this data, the model is set as :$\delta E(V_{G})=\mathcal{F}(m_{i},dm,\xi,V_{d},d)$ where $m_{i},dm,\xi,V_{d},d$ are the fitting parameters, and $V_{G}$ is the independent variable. (We use the as-designed length of the device $L$, and take $\epsilon_{r}=3.9$ for $SiO_{2}$.)
The resulting fit of the data from the $400~{}nm$ junction for both $\delta E$ and $v_{F}$ is plotted as solid lines in Fig. 3(a) and Fig. 3(b) respectively. Moreover, taking the fitted $\xi$, we calculate the Fermi velocity $v_{F}$ for all other junctions on the same substrate. As seen from Fig. 3(b), the calculated $v_{F}$ of all devices is in good agreement with the fit obtained from the $400$ nm junction. The fitted parameters are summarized in Table 1. All fall within the range of expected values, with $\xi$ being consistent with previously measured values for graphene/MoRe junctions.

In conclusion, we study the evolution of critical current with respect to gate in bilayer graphene Josephson Junctions (BGJJs). Using the critical current-temperature relation expected for intermediate-to-long junctions, we extract the relevant energy scale $\delta E$ and find that it has a clear gate dependence. As $\delta E$ is proportional to the Fermi velocity $v_{F}$ in the bilayer graphene, we are able to match the observed gate dependence to the theoretical expectation. Our observation is contrasted with monolayer graphene JJs, that do not have a gate dependent $\delta E$. This result showcases the greater tunability of BGJJs, and offers additional avenues for device characterization.