Exposing the hidden influence of selection rules on phonon-phonon scattering by pressure and temperature tuning

The thermal conductivity, $\kappa$, is a fundamental transport parameter that governs the efficiency of heat conduction through solids. In insulating crystals, the heat is transported by phonons, and intrinsic thermal resistance comes from phonon-phonon scattering processes, which arise from the anharmonicity of the interatomic bonding potential peierls_zur_1929 ; peierls_quantum_1955 ; ziman_electrons_2001 . Lowest-order processes involving the mutual interaction among three phonons typically dominate this intrinsic resistance.
For a given phonon mode, the collection of all such scattering processes that conserve energy and momentum - the scattering phase space - is dictated entirely by a material’s phonon dispersions. Specific features in these dispersions activate selection rules that can severely restrict the phase space of certain phonon-phonon scattering channels peierls_zur_1929 ; peierls_quantum_1955 ; ziman_electrons_2001 ; herpin_contribution_1952 ; klemens_thermal_1958 ; klemens_decay_1967 ; orbach_attenuation_1964 ; lax_spontaneous_1981 ; lindsay_first-principles_2013 ; broido_ab_2013 ; mukhopadhyay_optic_2016 ; ravichandran_phonon-phonon_2020 . While several of these selection rules have recently been identified lindsay_first-principles_2013 ; broido_ab_2013 ; mukhopadhyay_optic_2016 ; ravichandran_phonon-phonon_2020 , the most well-known of them dictates that an acoustic phonon cannot decay anharmonically into a set of others that are in the same dispersive phonon branch peierls_zur_1929 ; peierls_quantum_1955 ; ziman_electrons_2001 ; herpin_contribution_1952 ; klemens_thermal_1958 ; klemens_decay_1967 ; orbach_attenuation_1964 ; lax_spontaneous_1981 . It explains the long lifetimes of large wave vector transverse acoustic (TA) phonons, observed at low temperatures grill_excitation_1975 ; ulbrich_propagation_1980 . Exceptions exist tamura_spontaneous_1985 , but the phase space for those scattering events is small fNote1 .
The influence of this selection rule on $\kappa$ was long thought to be negligible since inter-branch phonon scattering processes dominate thermal transport, particularly at higher temperatures. However, it was recently pointed out that, even around and beyond room temperature (RT; 300 K), this selection rule can be activated for high frequency phonons in materials where the three acoustic phonon branches come close to each other in some region of the Brillouin zone (BZ), thereby mimicking the behavior of phonon decay within a single branch lindsay_first-principles_2013 ; broido_ab_2013 ; ravichandran_phonon-phonon_2020 . The phase space available to a heat carrying, high frequency acoustic (A) phonon in such a region of the BZ, decaying into two others (AAA process) can then become quite small, thus resulting in its long lifetime and large contributions to $\kappa$.
Perhaps the most interesting example of the impact of this AAA selection rule is cubic boron arsenide (BAs). First principles calculations lindsay_first-principles_2013 predicted that unusually weak AAA scattering, resulting from activation of the AAA selection rule, contributes to a $\kappa$ for BAs that should be comparable to that of diamond, the crystal having the highest measured $\kappa$ of all materials at RT. While inclusion of higher-order four-phonon scattering was found to reduce the BAs $\kappa$  feng_four-phonon_2017 ; tian_unusual_2018 , it still achieved a predicted RT $\kappa$ value of around 1300 Wm^-1K^-1, by far the highest value of any naturally occurring material, behind only the carbon crystals (e.g. diamond and graphite). These theoretical findings have been confirmed by measurements tian_unusual_2018 ; kang_experimental_2018 ; li_high_2018 , adding strong support to the impact of selection rules and corresponding reductions in the three-phonon scattering phase space on $\kappa$.
Recently, we have identified several other compounds, such as cubic boron phosphide (BP) and silicon carbide (SiC), where the AAA selection rule is activated, giving anomalously weak AAA scattering channels ravichandran_phonon-phonon_2020 . However, unlike in BAs, for these compounds, the weak AAA scattering is masked by another strong three-phonon scattering channel involving two acoustic phonons and an optic (O) phonon (AAO scattering channel), thus drastically reducing its impact on $\kappa$. In this letter, we show that the otherwise-hidden weak AAA scattering in BP and SiC can be exposed by hydrostatic pressure ($P$) and temperature ($T$) tuning of $\kappa$. We demonstrate that the evolving interplay between AAA and AAO scattering channels with varying $P$ and $T$ gives rise to two remarkable features in the $\kappa$ of BP around and above RT - an unusually sharp rise in $\kappa$ as $P$ increases from ambient pressure and, a peak and subsequent drop in $\kappa$ at high $P$. These features are contrary to the measured $\kappa$($P$, $T$) of many other insulating crystals that instead show a roughly linear increase with $P$ far away from phase transitions hakansson_thermal_1986 ; gerlich_temperature_1982 ; hakansson_thermal_1986 ; dalton_effect_2013 ; ohta_lattice_2012 ; manthilake_lattice_2011 ; hofmeister_pressure_2007 ; hofmeister_mantle_1999 ; hakansson_thermal_1986 ; andersson_thermal_1985 , consistent with simple theories hofmeister_pressure_2007 ; slack_thermal_1979 .
The $\kappa$($P$, $T$) of BP is calculated using a recently developed unified ab initio theoretical framework, which combines the use of density functional theory to obtain phonon dispersions and phonon scattering rates, with a numerical solution of the phonon Boltzmann transport equation ravichandran_unified_2018 . The theory has no adjustable parameters, and it has demonstrated good agreement with the measured $\kappa$ of several materials over a broad temperature and pressure range ravichandran_unified_2018 ; tian_unusual_2018 ; ravichandran_non-monotonic_2019 ; chen_ultrahigh_2020 ; ravichandran_phonon-phonon_2020 , including BP and SiC. Both three-phonon and higher-order four-phonon scattering processes are included in the calculations along with phonon scattering by mass disorder arising from the natural isotope mixture on the constituent atoms fNote2 . The details of this first principles approach have been published in Ref. ravichandran_unified_2018 and are summarized in the Supplemental Material (SM) section S1 fNote3 . We present the results for BP here, while those for SiC can be found in the SM section S4 fNote3 .

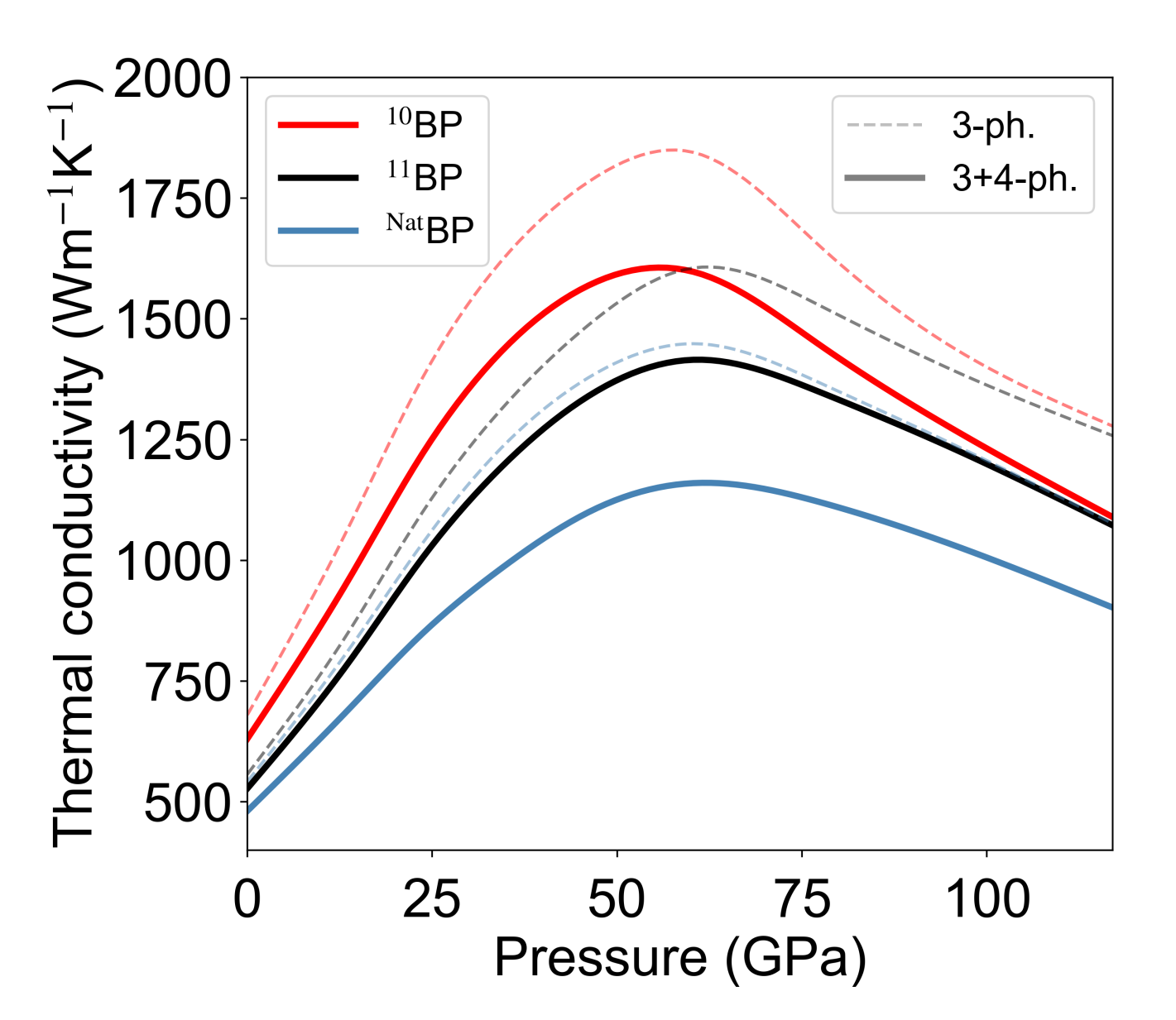
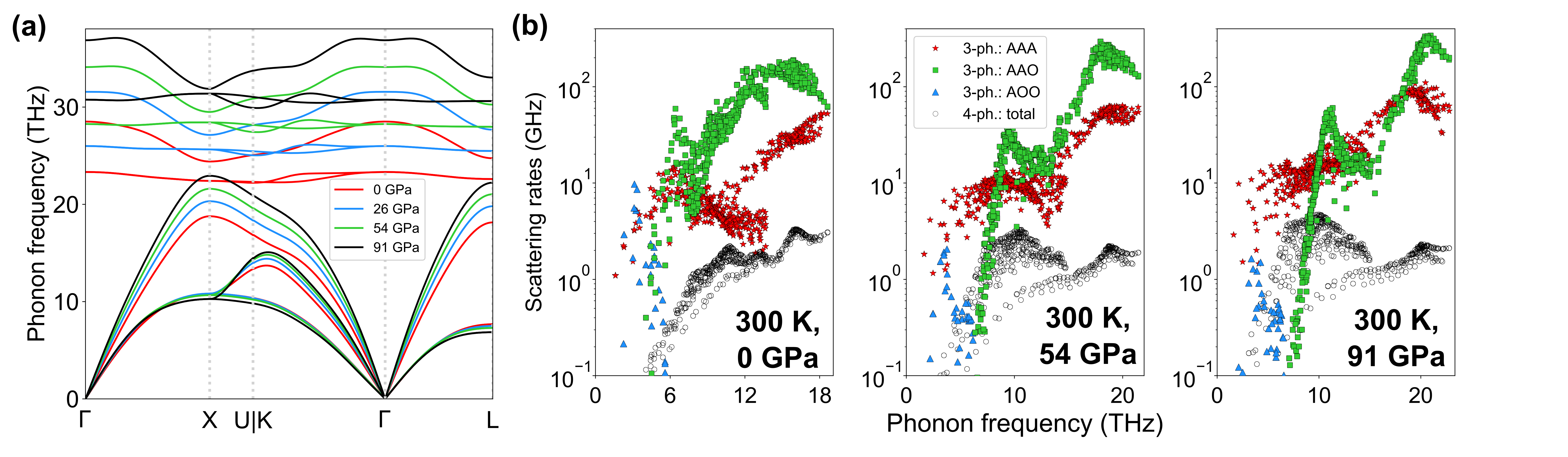
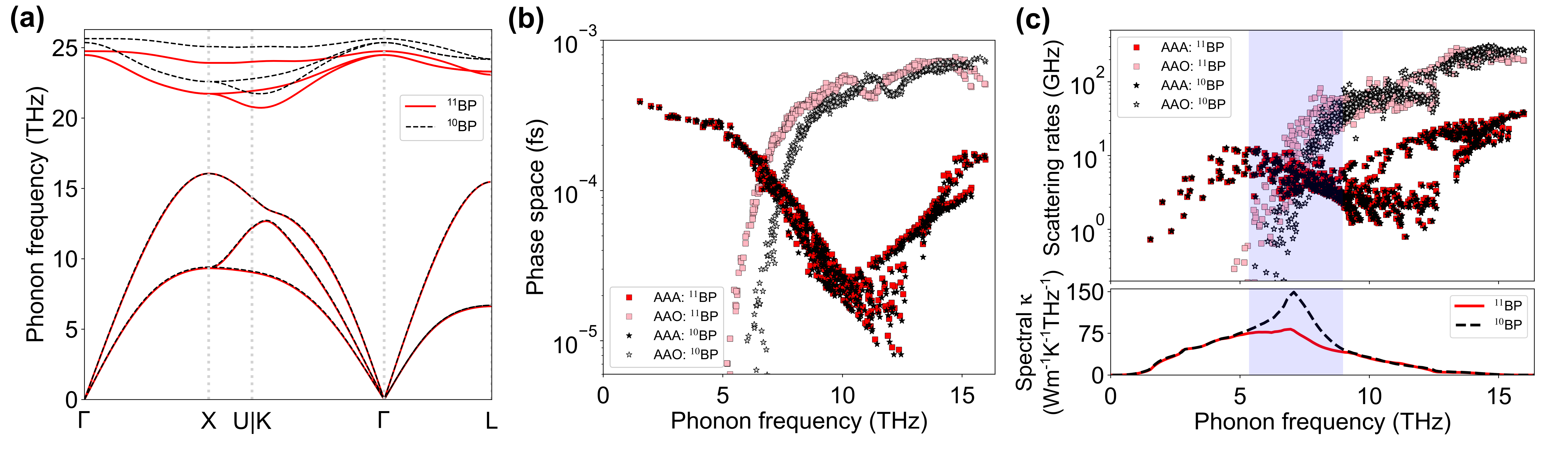
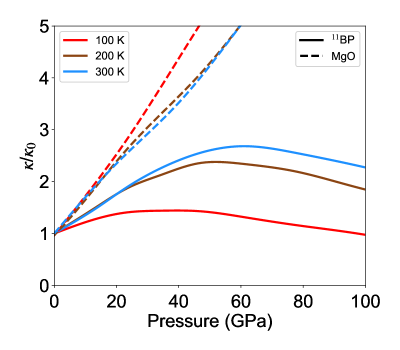
Figure 1 shows the calculated RT $\kappa$($P$) of BP, with and without four-phonon scattering, for three cases: BP with (1) naturally occurring B isotope mix (^NatBP), (2) B atoms isotopically enriched to 100 % ¹¹B (¹¹BP) and (3) B atoms isotopically enriched to 100 % ¹⁰B (¹⁰BP). All curves peak at around 55-60 GPa and subsequently decrease with increasing $P$, contrary to the typically measured linearly increasing behavior for many other materials. In addition, the $\kappa$($P$) curves rise rapidly with increasing $P$, and there is a significant increase in the slopes, $\mathrm{d}\kappa/\mathrm{d}P$, going from ^NatBP to ¹¹BP to ¹⁰BP. The peak values of $\kappa$ are extremely large, around 1150 Wm^-1K^-1 (^NatBP), 1400 Wm^-1K^-1 (¹¹BP) and 1600 Wm^-1K^-1 (¹⁰BP), about 2.5 times higher than their values at ambient pressure. It is important to note that, the effect of four-phonon scattering on $\kappa$ is relatively weak at all pressures in BP. Thus, the non-monotonic $\kappa$($P$) behavior for BP is unrelated to that predicted for BAs ravichandran_non-monotonic_2019 , as discussed below.
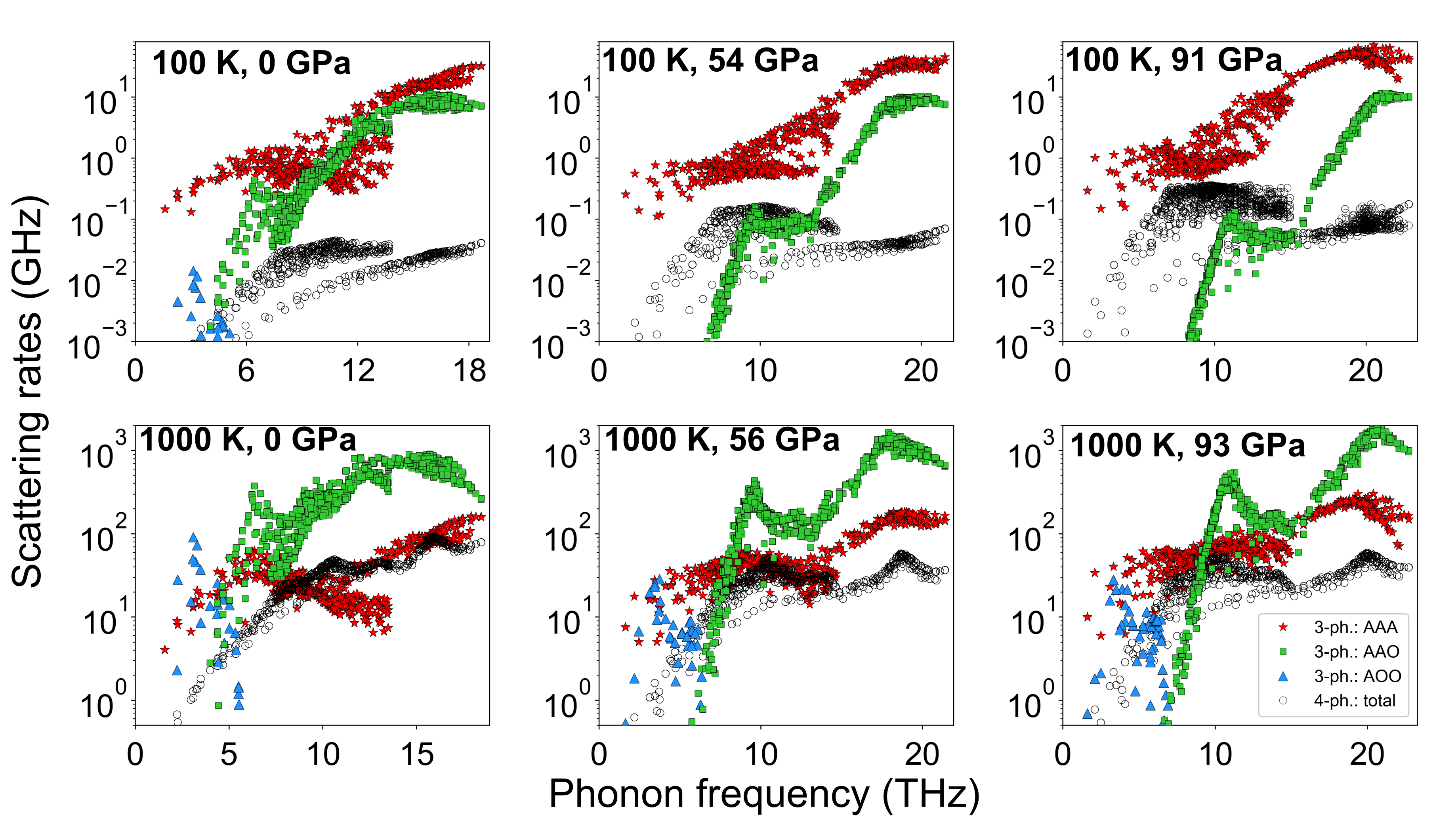
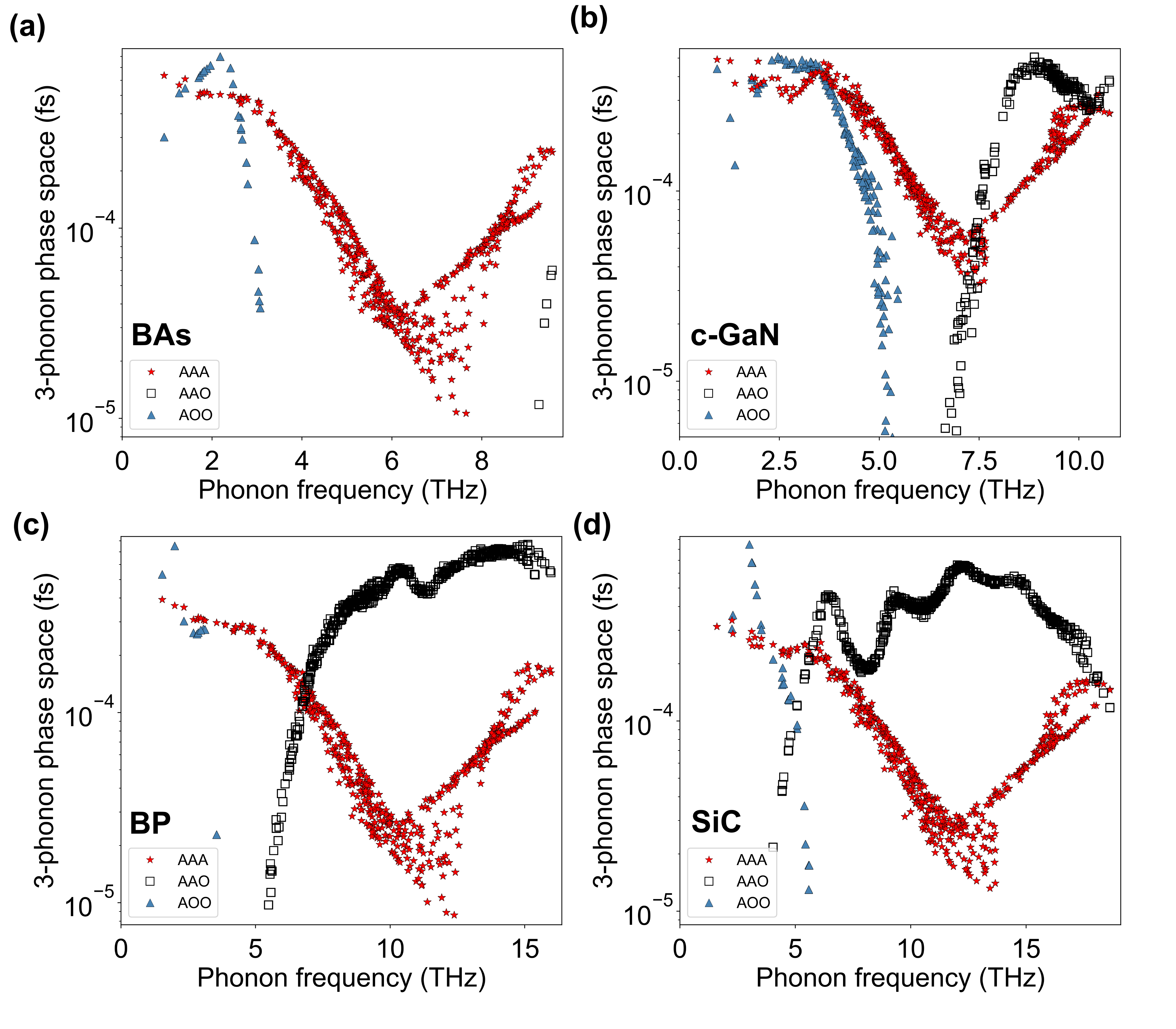
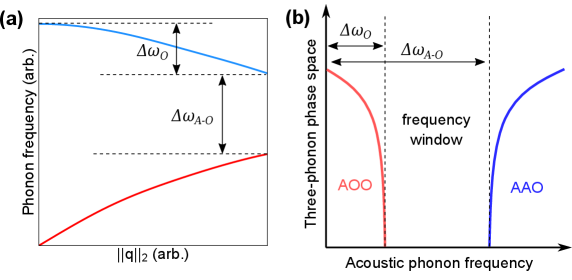
To understand these pressure-dependencies of $\kappa$, we first examine the phonon-phonon scattering processes in BP at ambient pressure. The light atoms and stiff bonding of BP result in a high $\kappa$ of around 500-600 Wm^-1K^-1 at RT and ambient pressure zheng_high_2018 ; chen_ultrahigh_2020 ; dames_ultrahigh_2018 . BP crystallizes in the zinc blende structure and has two atoms in each unit cell, so the phonon dispersions consist of three A and three O phonon branches. Only three types of three-phonon scattering processes can occur, which involve combinations of A and O phonons: AAA, AAO, and AOO. Energy conservation forbids all other processes (e.g. OOO) ravichandran_phonon-phonon_2020 . Figure 2 (b) shows the RT AAA, AAO and AOO scattering rates for BP at various pressures, along with those for four-phonon scattering. The sharp dip in the AAA scattering rates at $P$=0 in Fig. 2 (b) results from the AAA selection rule driven by the close proximity of the three acoustic phonon branches in a small region of the BZ, as seen in Fig. 2 (a), along $\Gamma\to K$ direction. Similar dips driven by the same selection rule occur in other cubic compounds such as BAs, BSb, SiC, diamond, c-BN, and GaN broido_ab_2013 ; ravichandran_phonon-phonon_2020 and certain transition metal carbides li_fermi_2018 . Some examples are shown in Fig. S1 in the SM.

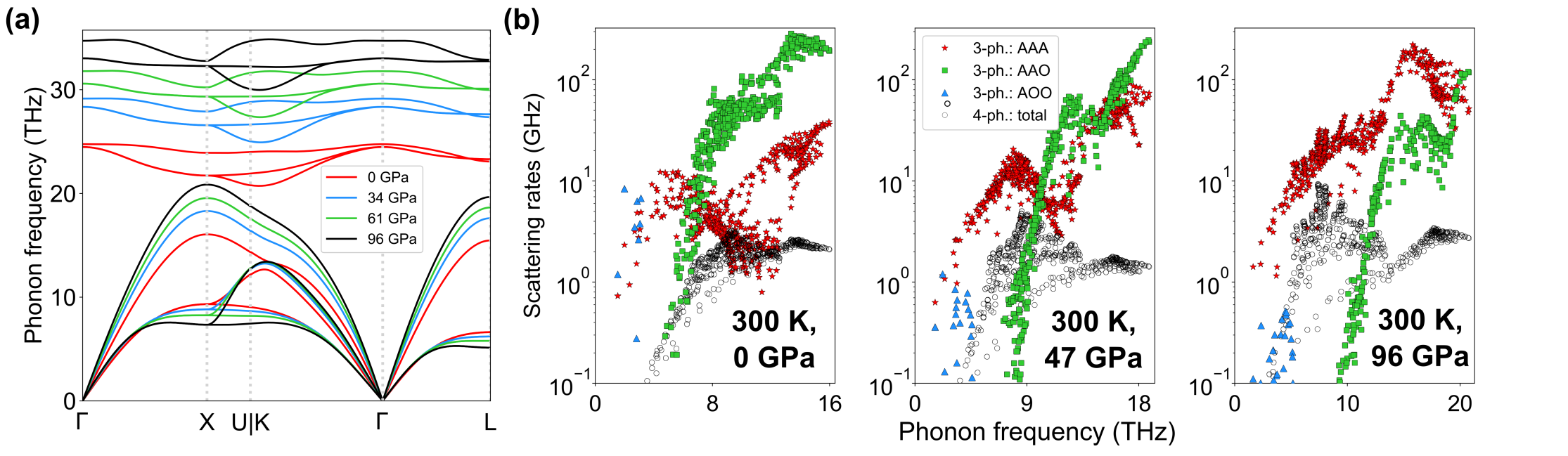
Apart from the close proximity of the acoustic phonons, other features in the phonon dispersions of BP and SiC activate additional selection rules. The mass difference between constituent atoms of these compound semiconductors causes a frequency gap, $\Delta\omega_{A-O}$, between acoustic and optic phonons (A-O gap). Energy conservation mandates that only those acoustic phonons with frequencies greater than $\Delta\omega_{A-O}$ can participate in AAO scattering processes lindsay_first-principles_2013 . Similarly, energy conservation restricts the participation of acoustic phonons in AOO scattering processes to those with frequencies less than the optic phonon bandwidth, $\Delta\omega_{O}$ mukhopadhyay_optic_2016 ; ravichandran_phonon-phonon_2020 . These restrictions are illustrated in Fig. S2 in the SM.
As shown in Fig. 2 (b), the AAO scattering rates in BP dominate over almost the entire frequency region of the dip in AAA scattering rates. The relatively small mass difference between boron and phosphorus atoms ($M_{P}/M_{B}=2.6$) gives a $\Delta\omega_{A-O}$ that is not large enough to freeze out the AAO scattering channel for acoustic phonons above $\sim$6 THz. This behavior is in stark contrast to large mass ratio compounds like BAs ($M_{As}/M_{B}=7$), where $\Delta\omega_{A-O}$ is large enough to almost completely remove AAO processes for acoustic phonons, thereby fully exposing the sharp dip in the AAA scattering rates, and resulting in significantly enhanced three-phonon limited $\kappa$ lindsay_first-principles_2013 ; fNote4 . To illustrate the importance of the AAO processes in BP, we perform calculations by artificially turning them off, and find that the RT $\kappa$ of ¹¹BP at ambient pressure jumps from 530 Wm^-1K^-1 (560 Wm^-1K^-1) to over 2600 Wm^-1K^-1 (6000 Wm^-1K^-1), including three-phonon and four-phonon (three-phonon only) scattering. These values are about twice the corresponding values in BAs.
In BP, application of hydrostatic pressure introduces three intertwined behaviors that affect $\kappa$: (i) the longitudinal acoustic (LA) and optic phonons shift to higher frequencies, which increases LA phonon group velocities and weakens the AAO scattering rates due to decreased optic phonon occupations. Both of these changes contribute to the increasing $\kappa$ with $P$ found in many materials. In BP, two additional features are critical to explain the anomalous behavior seen in Fig. 1 - (ii) optic phonons stiffen faster than do acoustic phonons [see Fig. 2 (a)], which increases $\Delta\omega_{A-O}$ and shifts the onset of AAO processes to higher frequencies, thereby exposing increasingly large portions of the AAA dip. This behavior significantly increases the rate of rise in the $\kappa(P)$ of BP in the low $P$ range, as discussed below; and (iii) with increasing $P$, the LA phonons stiffen, while the transverse acoustic (TA) phonons weakly soften. The resulting increased separation between LA and TA phonon branches gradually removes the impact of the AAA selection rule, and so, increases the AAA scattering rates, relative to those at ambient pressure [see Fig. 2 (b)]. This behavior acts to drive $\kappa$ lower, as pointed out previously lindsay_anomalous_2015 ; ravichandran_non-monotonic_2019 .
Peak and decrease in $\kappa$ - At RT and low $P$, the influence of trends (i) and (ii) is stronger than trend (iii) resulting in an increasing $\kappa(P)$. Beyond 50 GPa, the continued shift of AAO processes towards higher phonon frequencies almost fully exposes the AAA dip, and the rising AAA scattering rates from trend (iii) eventually dominate the behavior causing $\kappa$ to decrease with increasing $P$. As a result of this evolving interplay between AAA and AAO processes, the RT $\kappa(P)$ achieves a peak value at around 60 GPa, which is around 2.5 times that of its value at $P=0$.
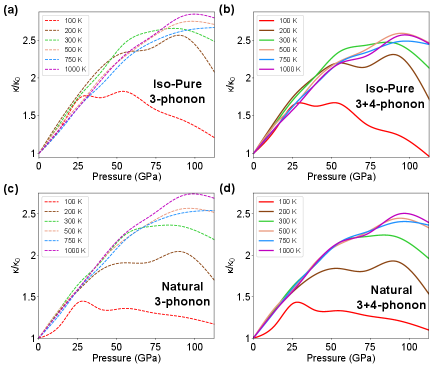
Figure 3 (a) shows the $\kappa(P)$ for ¹¹BP scaled by its zero pressure value, $\kappa_{0}$, for different $T$. Around and above RT, the curves for each $T$ roughly overlap, indicating that the $P$ and $T$ dependences are separable. Such separability is also predicted from empirical theory manthilake_lattice_2011 ; hofmeister_pressure_2007 and is found in conventionally behaving compounds such as MgO (see Fig. S4 in the SM). That it also occurs in BP with its anomalous non-monotonic $\kappa(P)$, is a consequence of the independent changes in AAA and AAO scattering rates induced by changing $T$ or $P$. Increasing $T$ strengthens AAO processes relative to AAA processes, while increasing $P$ shifts the AAO processes to higher frequencies relative to AAA processes. Above RT, AAO scattering rates in BP dominate in magnitude over AAA scattering rates until very high $P$ [Fig. 3 (b)], just as they do at RT [Fig. 2 (b)]. Thus, the evolution of $\kappa(P)$ above RT is qualitatively the same as that at RT. However, for $T$ well below RT, as AAO scattering rates weaken relative to AAA scattering rates [see Fig. 3 (b)], the rise in $\kappa$ from trends (i) and (ii) is overcome by trend (iii) at lower $P$, resulting in a weaker $P$ dependence to $\kappa(P)$, which eventually decreases below $\kappa_{0}$, as shown in Fig. 3 (a) for $T$ = 100 K. Similar behavior of $\kappa(P)$ also occurs in SiC (see SM section S4).

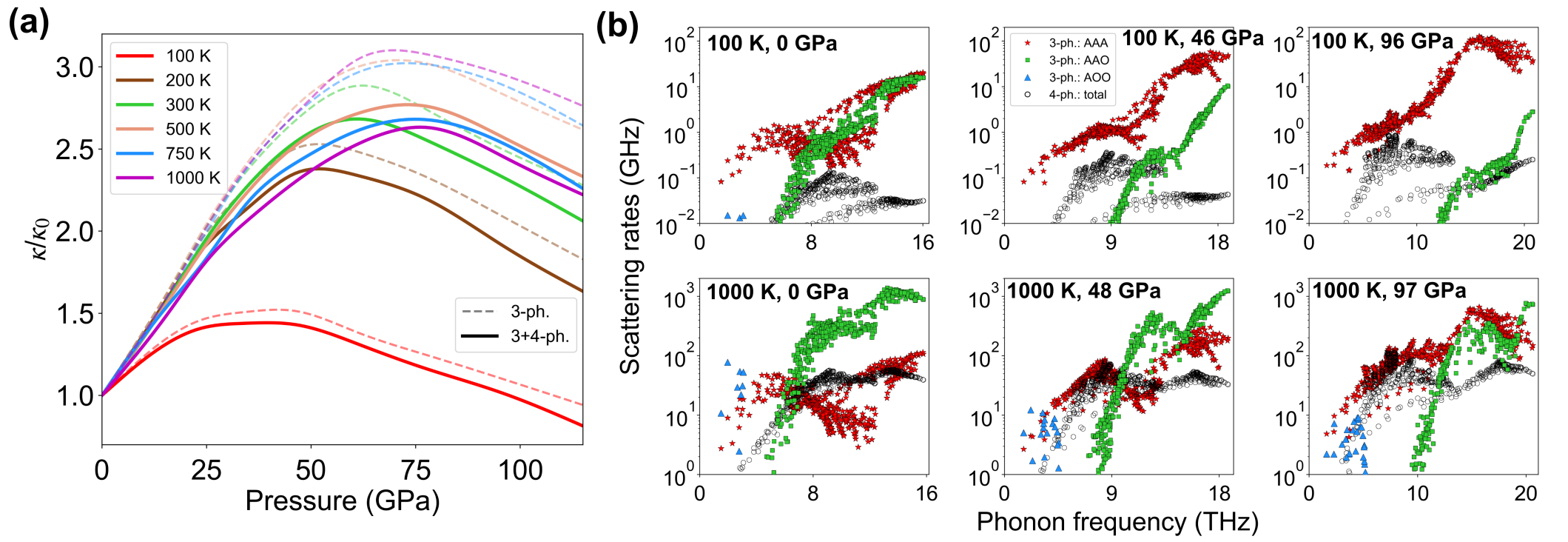
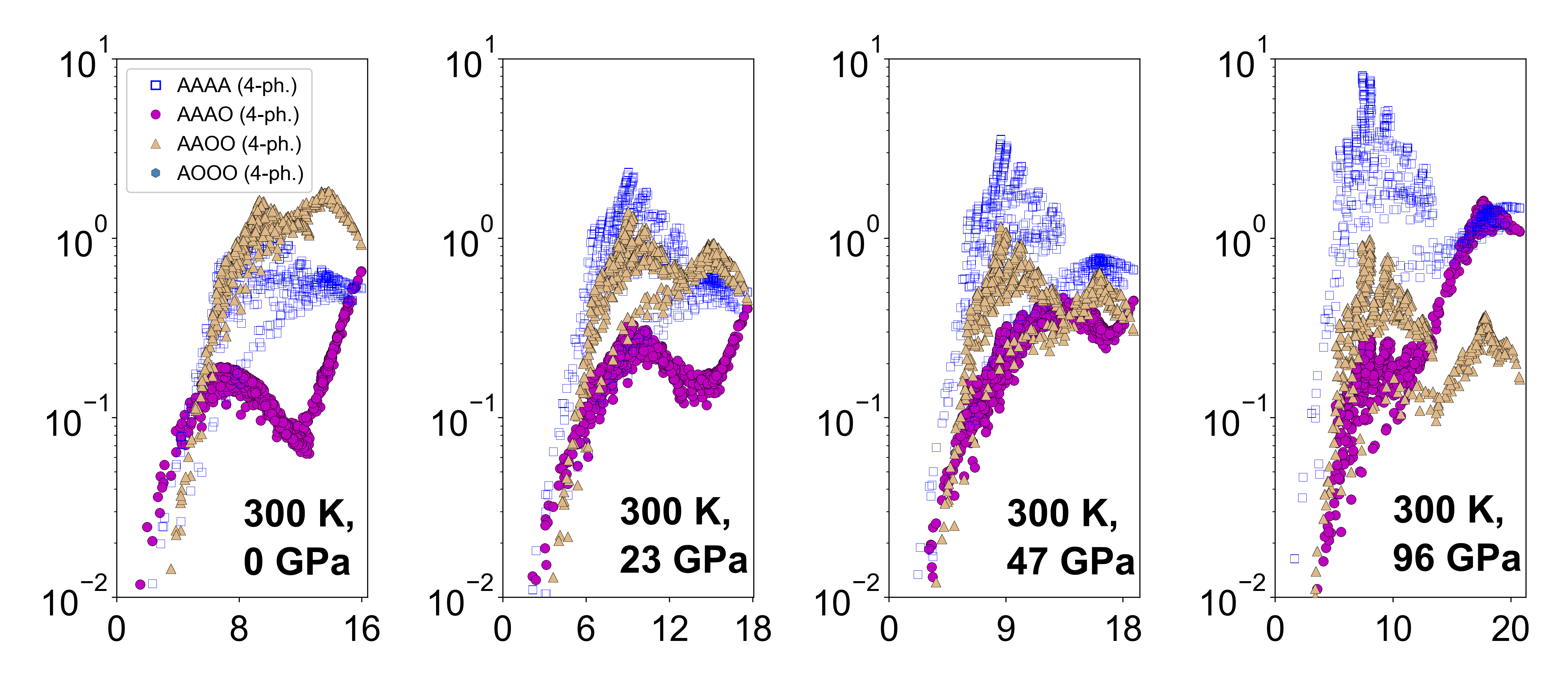
Previously, we predicted a non-monotonic pressure dependence of $\kappa$ for BAs ravichandran_non-monotonic_2019 , which was connected to an evolving competition between three-phonon and four-phonon scattering processes with increasing $P$. This competition is responsible for a significant suppression of the measured BAs $\kappa$-values compared with the lowest-order three-phonon prediction feng_four-phonon_2017 ; tian_unusual_2018 . In stark contrast, however, the non-monotonic behavior of $\kappa(P)$ in BP arises from fundamentally different physics involving competition only among three-phonon scattering channels. This competition contributes to a significantly larger peak-$\kappa$ value in BP than in BAs (2.5x in BP vs. 1.1x in BAs at RT), and a much sharper increase in the BP $\kappa$ at low $P$ (discussed next), thus making it more accessible for experimental validation. We note that the effect of four-phonon scattering on the $\kappa(P)$ of BP and SiC is dominated by the increasing strength of the AAAA scattering channel with increasing $P$ (see SM sections S3 and S4), but it is generally weak compared with the dominant three-phonon scattering channels, and so does not cause qualitative differences in the observed $\kappa(P)$ trends [see Figs. 1 and 3 (a)].
Unusually sharp rise in $\kappa(P)$ above P=0 - The calculated slopes, $\mathrm{d}\kappa/\mathrm{d}P$, of the $\kappa(P)$ curves in Fig. 1 around ambient pressure are exceptionally large. Significant contributions to these large values come from the rapid increase in the intrinsic lifetimes of acoustic phonons whose frequencies lie just below the onset of the AAO scattering. With increasing $P$, this onset shifts to higher frequencies, exposing more of the AAA dip. Phonons in the newly exposed region of the AAA dip have reduced scattering rates and so give correspondingly larger contributions to $\kappa$. This behavior is illustrated in Fig. 4, which shows, $\kappa(\nu)$, the spectral contributions to $\kappa$ as a function of phonon frequency, $\nu$, for ¹⁰BP and ¹¹BP at different pressures and RT. The effect is enhanced in ¹⁰BP compared with ¹¹BP because the lighter ¹⁰B atom shifts the onset of AAO scattering processes to higher frequencies even at $P=0$ (Fig. S3). This difference helps explain the much larger calculated RT and ambient pressure $\kappa$ of ¹⁰BP (630 Wm^-1K^-1) compared with that for ¹¹BP (530 Wm^-1K^-1), consistent with measured results zheng_high_2018 ; chen_ultrahigh_2020 .

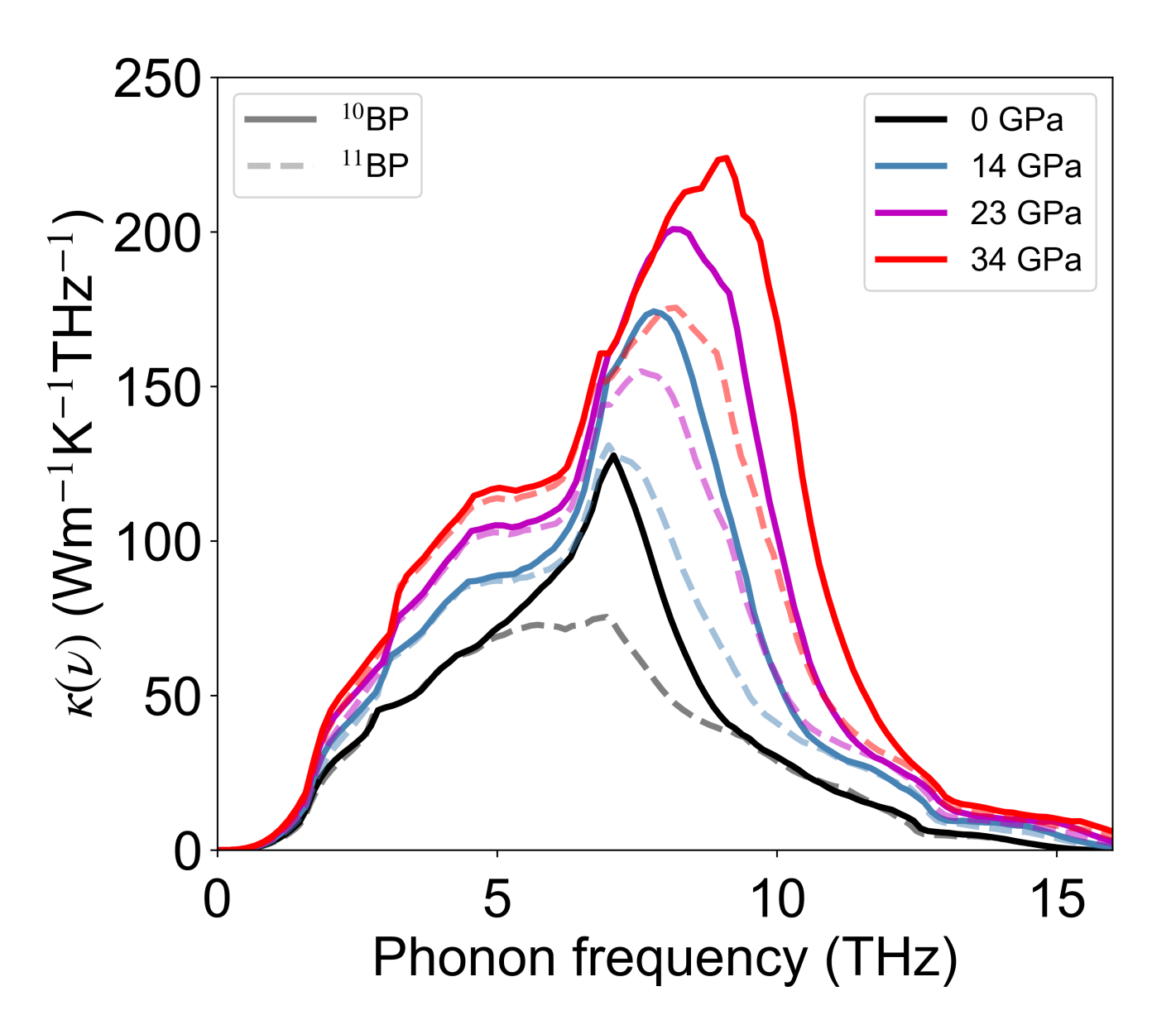
We calculate the RT $\mathrm{d}\kappa/\mathrm{d}P$ values of 15.4 Wm^-1K^-1GPa^-1 (^NatBP), 19.1 Wm^-1K^-1GPa^-1 (¹¹BP) and 24.1 Wm^-1K^-1GPa^-1 (¹⁰BP) at low $P$. Measurements and calculations of $\mathrm{d}\kappa/\mathrm{d}P$ given in the literature are only for materials with much lower $\kappa$, such as those of interest for geophysical studies dalton_effect_2013 ; ohta_lattice_2012 ; manthilake_lattice_2011 ; hofmeister_mantle_1999 ; hofmeister_pressure_2007 , e.g. MgO, as well as alkali halides andersson_thermal_1985 ; hakansson_thermal_1986 ; gerlich_temperature_1982 . For these materials, $\mathrm{d}\kappa/\mathrm{d}P$ is less than 3 Wm^-1K^-1GPa^-1, far smaller than our calculated values for BP. Therefore, to put the BP values in better context, we have calculated the RT $\mathrm{d}\kappa/\mathrm{d}P$ for diamond, whose $\kappa$ is higher than that of BP. We find RT $\mathrm{d}\kappa/\mathrm{d}P$ values of 21 Wm^-1K^-1GPa^-1 and 17 Wm^-1K^-1GPa^-1 for isotopically pure and naturally occurring diamond respectively. Remarkably, the calculated $\mathrm{d}\kappa/\mathrm{d}P$ value for ¹⁰BP is higher than that for diamond, even though the absolute RT $\kappa$ is five times lower. The present findings suggest that the $\mathrm{d}\kappa/\mathrm{d}P$ for ¹⁰BP may be the highest value achievable for any material, a striking signature of the influence of the AAA selection rule.
In summary, through ab initio calculations for BP, we have shown that a hidden weak three-phonon scattering channel arising from a selection rule on anharmonic decay of acoustic phonons can be revealed through examination of the pressure dependence of the thermal conductivity, which displays anomalous features including an exceptionally fast rise and a peak with increasing pressure. We predict that such anomalous behavior will also occur in other materials similarly affected by the same selection rule, which we have demonstrated for cubic silicon carbide (SiC) in the SM (see Figs. S6-S9). Other recently identified phase space selection rules should also give rise to unusual pressure dependence of phonon lifetimes and $\kappa$ in the affected materials ravichandran_phonon-phonon_2020 . The present work gives new insights into the impact of selection rules on phonon-phonon scattering and demonstrates novel behavior of phonon thermal transport in solids under pressure.
This work was supported by the Office of Naval Research under a Multidisciplinary University Research Initiative, Grant No. N00014-16-1-2436.