Experimental quantum simulation of superradiant phase transition beyond no-go theorem via antisqueezing

Superradiant phase transition, driven by the singularity of quantum fluctuation at the critical point, has undergone tremendous developments in recent years [1]. It was proposed in the Dicke model, describing the collective interaction between $N$ two-level systems and a quantum field, in the thermodynamics limit $N\rightarrow\infty$ [2, 3]. When $N$ = 1, the Dicke model is reduced to a Rabi model, in which the SPT has also been predicted theoretically by replacing the thermodynamics limit with the classical oscillator limit $\Omega/\omega\rightarrow\infty$ ($\Omega$ and $\omega$ being the frequency of spin and field, respectively) [4]. Above the quantum critical point, the vacuum (ground state) of cavity field is macroscopically occupied, and becomes twofold degenerate, corresponding to a spontaneously $\mathbb{Z}_{2}$ symmetry breaking. This leads to the appearance of important quantum effects in the supperradiant phase, including spin-field entanglement, distinguishable quantum superposition with large-amplitude, and so on [5].

Cavity (including circuit) QED systems [6, 7], allowing to manipulate the light-matter interaction at the quantum level, offer an important platform of implementing SPT. However the required critical parameter regime and ultralow-temperature ground state preparation are normally hard to be satisfied with current technologies of cavity QED. More importantly, the existence of the equilibrium SPT in the cavity QED systems is still challenged by the no-go theorem [8, 9, 10, 11, 12, 13]. Specifically, for describing the dipole atom-field interactions in the cavity QED system, the standard Dicke and Rabi Hamiltonians have neglected the squared term of electromagnetic vector potential (i.e., $A^{2}$ term), which will forbid the occurrence of equilibrium SPT. This is because the $A^{2}$ term, via adding a coupling-dependent potential of the cavity field, makes the disappearance of the singularity of quantum fluctuation during the whole parameter space. Until now, the SPT has not been realized experimentally in thermal equilibrium, while the nonequilibrium SPT has been observed in the simulations of the DM with a Bose-Einstein condensate in an open cavity [14, 15, 16] or a trapped ion setup [17].

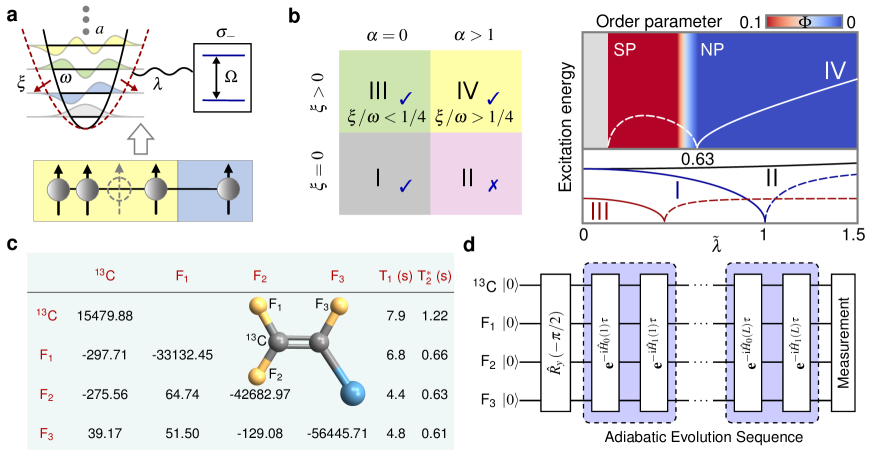
Here we employ the quantum simulation technology [18, 19, 20] to experimentally demonstrate the realization of equilibrium SPT beyond no-go theorem induced by an added antisqueezing of field. We use liquid-state NMR molecules [21] to simulate the quantum Rabi model including the $A^{2}$ and antisqueezing terms (approaching the classical oscillator limit $\Omega/\omega\rightarrow\infty$) by a well-defined spin-to-oscillator mapping scheme. Note that the unaccessible parameter conditions for SPT in the actual cavity QED system can be attained in the platform of NMR. Based on the excellent controllability, the NMR system has been successfully used to simulate topological orders [22] and Lee-Yang zeros [23].

Interestingly, we experimentally show that the antisqueezing effect not only makes the appearance of SPT in the case of including $A^{2}$ term, but also makes the SPT to be reversed, i.e., transition from normal phase (NP) to superradiant phase (SP) along with decreasing spin-field coupling strength. This originally comes from the exponentially enhanced ZPF induced by the antisqueezing effect, that recovers the singularity of the ground state of system. The optimized parameter condition including the necessary antisqueezing strength for phase transition is identified by presenting experimentally the antisqueezing-dependent phase diagram of ground state. In the SP, we experimentally obtain the strong spin-oscillator entanglement and the squeezed Schrödinger cat states of spins exhibiting a negative Wigner distribution, large-amplitude separation of peaks, and distinct interference fringes. These states could be used to fault-tolerant quantum computation [24, 25] and quantum metrology [26] approaching Heisenberg limit with actual NMR systems, aside from providing fundamental insights into the nature of decoherence and the quantum–classical transition [27]. Moreover, we show good theory-experiment agreement due to the antisqueezing-enhanced SNR of NMR spectrum, which applies to the general NMR experiments. Our work also provides the important family of antisqueezing with a new type of applications, besides its widely applications in quantum precision measurement [28] and enhancing light-matter interaction [29, 30, 31, 32, 33, 34, 35, 36].

The Rabi model with Hamiltonian

describes a two-level system with frequency $\Omega$ interacting with an oscillator mode with frequency $\omega$, and $\lambda$ denotes the coupling strength. Here $\hat{a}$ ($\hat{a}^{\dagger}$) is the annihilation (creation) operator of the oscillator mode, and $\hat{\sigma}_{z}$, $\hat{\sigma}_{x}$ are the Pauli operators for the two-level system. This model has the $\mathbb{Z}_{2}$ (or parity) symmetry associating with a well-defined parity operator $\hat{\Pi}=e^{i\pi\hat{\mathbb{N}}}$, where $\hat{\mathbb{N}}=\hat{a}^{\dagger}\hat{a}+(1/2)(\hat{\sigma}_{z}+1)$ is the total excitation number of the system. As shown in the regime ${\rm I}$ of FIG. 1b, the ground-state SPT is predicted theoretically in the classical oscillator limit $\Omega/\omega\rightarrow\infty$, characterized by a vanishing of the lowest excitation energy [4]. However, this SPT will disappear when the $A^{2}$ term $\hat{H}_{A}=(\alpha\lambda^{2}/\Omega)(\hat{a}+\hat{a}^{\dagger})^{2}$ ($\alpha\geq 1$ decided by the Thomas-Reiche-Kuhn sum rule) is included in the actual cavity QED systems, corresponding to the regime ${\rm II}$ of FIG. 1b. This is the no-go theorem of SPT [10], and the corresponding debate continues to today from 1970s. Here we will demonstrate experimentally the above no-go theorem can be broken through by introducing an antisqueezing effect, i.e., $\hat{H}_{\rm As}=-\xi(\hat{a}+\hat{a}^{\dagger})^{2}$ in the platform of NMR. The regime ${\rm IV}$ of FIG. 1b theoretically shows the reappearance of SPT via the singularity of the excitation energy and the sudden change of the order parameter $\Phi=(\omega/\Omega)\langle\hat{a}^{\dagger}\hat{a}\rangle$ at the critical point $\tilde{\lambda}=\sqrt{1+\alpha\tilde{\lambda}^{2}-4\xi/\omega}$ with $\tilde{\lambda}=2\lambda/\sqrt{\Omega\omega}$. Specifically, the rescaled ground-state occupation of oscillator $\Phi=(1/4)(\tilde{\lambda}_{s}^{2}-\tilde{\lambda}_{s}^{-2})$ becomes non-zero from $\Phi=0$ at the critical point [37]. The regime ${\rm III}$ of FIG. 1b demonstrates that the antisqueezing effects could dramatically reduce the critical point of SPT in the case of $\alpha=0$.

To experimentally demonstrate the ground-state SPT in the platform of NMR, we simulate the Rabi model including the $A^{2}$ and antisqueezing terms by using $N$+1 spins, as shown in Fig. 1a. Based on the generators of SU(2), the mapping process is defined as

where $\hat{\Sigma}_{z}=-\sum^{N}_{i=1}2^{i-2}\hat{\sigma}^{i}_{z}+(2^{N}-1)/2$, and $\mathds{1}^{\otimes N}=\mathds{1}\otimes\cdots\otimes\mathds{1}$ (with $N$ spin identity operator $\mathds{1}$) is the identity matrix of $2^{N}\times 2^{N}$ dimensions. Here the definitions of operators $\hat{A}_{\pm}$ and the well-defined spin-to-oscillator mapping process are shown in the Methods. This mapping process has some similarities to Holstein-Primakoff transformation, and it is exact in the limit of $N\rightarrow\infty$. We employ ¹³C-iodotriuroethylene dissolved in d-chloroform as 4-qubits system and the experiments are conducted on Bruker Avance III 400 MHz spectrometer at room temperature. The molecule consists of one ¹³C and three ¹⁹F nuclear spins, as shown in FIG. 1c. Now the base vectors of 4-qubits span a 16 dimensional Hilbert space $\{|n\rangle\}$ $\left(n=0,1,2....15\right)$, defined in the Methods. In the weak-coupling approximation, the natural Hamiltonian of the sample molecule is described as

where $\omega_{i}$ represents the chemical shift of the $i$-th spin, and $J_{ij}$ is the scalar coupling strength between two spins. The values of parameters $\omega_{i}$ and $J_{ij}$ are given in Fig. 1c. At the beginning, our system, initially at the thermal equilibrium state, is prepared to a pseudo-pure state (PPS) $\hat{\rho}_{\rm pps}=[(1-\varepsilon)/16]\mathds{1}^{\otimes 4}+\varepsilon\outerproduct{0}{0}$ by using the selective-transition approach [38]. Here $\varepsilon\approx 10^{-5}$ is the polarization, and the fidelity between the experimental initial state and the pure state $|0\rangle\langle 0|$ is 0.991. The detail initialization process is shown in the Supplementary [37].

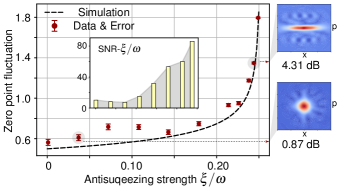
Let us first experimentally demonstrate the antisqueezing-enhanced ZPF in our 4-spins system, which is the key of recovering ground-state SPT in the case of including the $A^{2}$ term. It is also the nature of antisqueezing enhanced light-matter interaction explored in recent theoretical [29, 30, 31, 32, 33, 34, 35] and experimental [36] works. Theoretically, the antisqueezing term $\hat{H}_{\rm As}$ will make the ground state of an oscillator from a vacuum state $|0\rangle$ to the squeezed vacuum state $\hat{S}(r)|0\rangle$ with $\hat{S}(r)=\exp[r(\hat{a}^{2}-\hat{a}^{\dagger 2})/2]$ and the squeezing parameter $r=(1/4)\ln(1-4\xi/\omega)$. Then the ZPF, defined as ${\rm ZPF}=\sqrt{\langle\hat{x}^{2}\rangle-\langle\hat{x}\rangle^{2}}$ with nondimensional quadrature $\hat{x}=(\hat{a}+\hat{a}^{\dagger})/2$, will be exponentially enhanced with increasing the antisqueezing strength $\xi$ (see the dashed line of FIG. 2). In our experiment, the truncated squeezing operator in the Hilbert space $\{|n\rangle\}$ is implemented with the gradient ascent pulse engineering (GRAPE) pulses with time 15ms [39]. The pulse sequences are sequentially applied into the PPS for preparing the squeezing vacuum state. Then, we implement two steps measurement to obtain the ZPF of the squeezing vacuum state from the readout spectra of NMR. (i) Apply four $\pi/2$ readout pulses, i.e., $\hat{R}^{(i)}_{y}(\pi/2)=\exp(-i\pi/4\hat{\sigma}^{(i)}_{y})$, to reconstruct the diagonal elements [40] of the squeezing vacuum density matrix, corresponding to the expectation $\langle\hat{a}^{\dagger}\hat{a}\rangle$. (ii) Apply identity operator and unitary operator $\hat{U}_{1}$ (or $\hat{U}_{2}$) to the fourth (or third) spin, followed by measuring the NMR spectra of the fourth (or third) spin to obtain the expectation $\langle\hat{a}\rangle$ (or $\langle\hat{a}^{2}\rangle$). Here the operators $\hat{U}_{1}=\sum^{14}_{n=0}|n\rangle\langle n+1|+|15\rangle\langle 0|$ and $\hat{U}_{2}=\sum^{13}_{n=0}|{n}\rangle\langle{n+2}|+|14\rangle\langle{0}|+|15\rangle\langle{1}|$ are used to transfer the concerned parts of $\langle\hat{a}\rangle$ and $\langle\hat{a}^{2}\rangle$ to the single quantum coherence terms, and they can be implemented by the quantum circuits shown in Fig. S4 of Supplementary [37] or the equivalent GRAPE pulse sequences. To clearly show the antisqueezing effects, we also present the Wigner functions of two experimentally reconstructed states by the state tomography [40]. The experimental data shown in FIG. 2 are in excellent agreement with the theoretical prediction, and the error bars, coming from the statistical fluctuation of the NMR spectra, become smaller along with increasing the antisqueezing effect. This originally comes from the antisqueezing-enhanced SNR of the NMR spectra, as shown in the inset of FIG. 2 and FIG. S5(a) of Supplementary [37]. Our work offers a general method to enhance the measurement precision in the NMR experiments, where the signal is encoded in the $\hat{x}$ quadrature of the detector.

Next we experimentally show the equilibrium SPT modulated by the antisqueezing effects. The key point is the recovering of ground-state SPT of Rabi model including the $A^{2}$ term due to the antisqueezing-enhanced ZPF shown above. With the exact squeezing transformation, the ground state of the total system Hamiltonian $\hat{\mathds{H}}=\hat{H}_{R}+\hat{H}_{A}+\hat{H}_{As}$ is equivalent to apply a squeezing operation $\hat{S}(\tilde{r})$ on the ground state of the transformed Hamiltonian $\hat{\mathds{H}}_{s}=\hat{S}^{\dagger}(\tilde{r})\hat{\mathds{H}}\hat{S}(\tilde{r})=(\Omega/2)\hat{\sigma}_{z}+\omega_{s}\hat{a}^{\dagger}\hat{a}+\lambda_{s}(\hat{a}^{\dagger}+\hat{a})\hat{\sigma}_{x}$ [37]. Here $\omega_{s}=e^{2\tilde{r}}\omega$, $\lambda_{s}=e^{-\tilde{r}}\lambda$, $\tilde{r}=(1/4)\ln(1+\alpha\tilde{\lambda}^{2}-4\xi/\omega)$, and the constant term in Hamiltonian $\hat{\mathds{H}}_{s}$ has been ignored. Now the problem is transferred to experimentally preparing the ground state of $\hat{\mathds{H}}_{s}$. In our sample molecule, ¹³C spin is labeled as the two-level system, and three ¹⁹F nuclear spins are used to map the truncated boson mode $\hat{a}$ with the defined mapping process Eq. (2). In the experiments, we employ the widely used adiabatic method [41] to prepare the ground state of $\hat{\mathds{H}}_{s}$. According to the quantum circuit shown in FIG. 1d, the 4-spins sample is firstly prepared into the ground state of Hamiltonian $\hat{\mathds{H}}_{0}=\sum_{i=1}^{4}\hat{\sigma}^{(i)}_{y}$ by applying $\pi/2$ pulses along $y$ axis on four spins simultaneously. Then the quantum system is controlled to adiabatically evolve under the instantaneous Hamiltonian $\hat{\mathds{H}}(l)=[1-s(l)]\hat{\mathds{H}}_{0}+s(l)\hat{\mathds{H}}_{s}$, with $l=1,2,...L$ and $s\left(l\right)$ changing slowly from 0 to 1. The system will finally evolve to the ground state of $\hat{\mathds{H}}_{s}$, denoted by $|G\rangle_{s}$, after the above adiabatic evolution sequence. The experimental adiabatic evolution is implemented by the GRAPE pulse with time 26ms. Based on the prepared ground state $|G\rangle_{s}$, the order parameters of SPT, expressed as $\Phi=(\omega/\Omega)(\cosh(2\tilde{r})\langle\hat{a}^{\dagger}\hat{a}\rangle_{s}-(1/2)\sinh(2\tilde{r})(\langle\hat{a}^{\dagger 2}\rangle_{s}+\langle\hat{a}^{2}\rangle_{s})+\sinh^{2}\tilde{r})$, can be obtained by measuring the corresponding expectations defined with $|G\rangle_{s}$. The detail measurement processes are shown in the Methods.

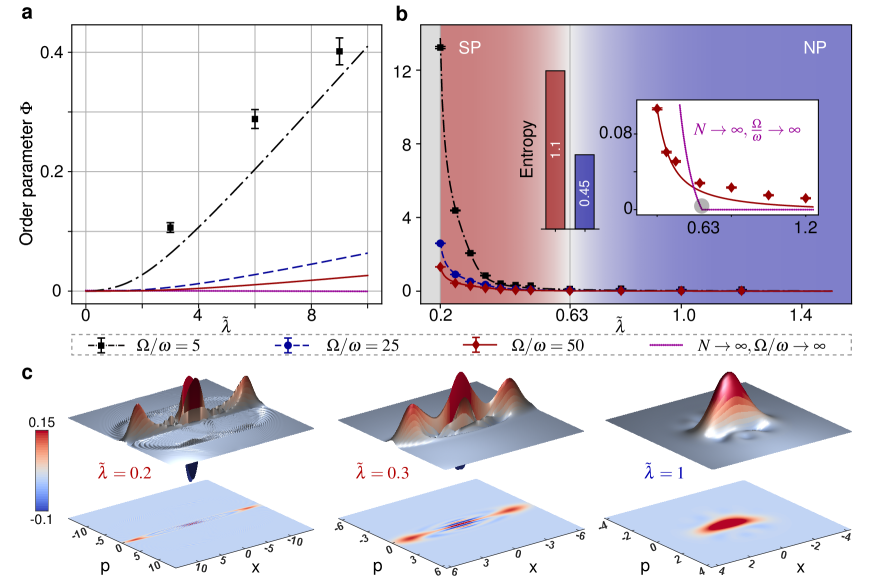
To show the realization of SPT, we present the dependence of the order parameter $\Phi$ (including the theoretical results and experimental data) on $\tilde{\lambda}$ for different frequency ratio $\Omega/\omega$ in FIGs. 3a and 3b. It is shown from FIG. 3a that, without antisqueezing effects ($\xi$ = 0), the phase transition is forbidden by the $A^{2}$ term, i.e., the no-go theorem. However, the SPT is recovered by introducing a fixed antisqueezing effect ($\xi/\omega=0.26$) in FIG. 3b. Specifically, the experimental order parameter $\Phi$ in FIG. 3b changes from almost zero to finite number at $\tilde{\lambda}\approx 0.63$ with decreasing $\tilde{\lambda}$, which indicates a reversed SPT approximately. Along with increasing $\Omega/\omega$, this tendency approaches the case of $\Omega/\omega\rightarrow\infty$, where the reversed SPT occurs exactly at the critical point $\tilde{\lambda}=\sqrt{1+\alpha\tilde{\lambda}^{2}-4\xi/\omega}$ (see the inset of FIG. 3b) [37]. Physically, the occurrence of SPT in our experiment originally comes from the recovering of the singularity of ground state fluctuations due to the antisqueezing-enhanced ZPF of system, as shown in Fig. S2 of the Supplementary [37]. Moreover, the comparison between FIGs. 3a and 3b shows that the experimental data agree highly with the theoretical results in the case of introducing the antisqueezing effects, which demonstrates again the property obtained from FIG. 2, i.e., the antisqueezing effects can significantly enhance the SNR of the NMR spectra.

Rich quantum resources can be obtained in the superradiant phase, such as the quantum entanglement and quantum superposition of coherent states, i.e., Schrödinger cat states. They are significant for quantum metrology and quantum computation, aside from their fundamental nature. For example, Schrödinger cat states can enhance the measurement precision by separating the two superposed coherent states with a large distance in phase space, which offers the complementary sensitivity to environmental influences. In the limit $\Omega/\omega\rightarrow\infty$, the ground state of our system (including the antisqueezing term) is theoretically predicted as a squeezed state $|G\rangle_{\rm np}=\hat{S}(\tilde{r}_{\rm np})|0\rangle_{a}|\!\downarrow\rangle$ in the NP and a spin-oscillator entangled state $|G\rangle_{\rm sp}\approx(1/\sqrt{2})\hat{S}(\tilde{r})[\hat{D}(\beta)|0\rangle_{a}|\!\downarrow\rangle_{+}+\hat{D}(-\beta)|0\rangle_{a}|\!\downarrow\rangle_{-}]$ in the SP with a defined displaced operator $\hat{D}(\beta)$ [37]. By the state tomography, we experimentally reconstruct the ground states of system, when it is in the NP ($\tilde{\lambda}=1$) and SP ($\tilde{\lambda}=0.2,\,0.3$). The bar graph in FIG. 3b clearly demonstrate that the strong spin entanglement is obtained in the SP via the von Neumann entropy $S=-\tr(\hat{\rho}_{a}\log_{2}\hat{\rho}_{a})$ ($\hat{\rho}_{a}$ is the reduced density matrix of oscillator). Moreover, in the SP, the entanglement state $|G\rangle_{\rm sp}$ becomes a squeezed cat state, when we measure the ¹³C spin in the $(1/\sqrt{2})(|\!\downarrow\rangle_{+}\pm|\!\downarrow\rangle_{-})$ basis [37]. We plot the corresponding Wigner functions of three experimentally reconstructed ground states in FIG. 3c, which clearly demonstrate the appearance of squeezed cat states in the SP. They have a negative Wigner distribution with distinct interference fringes and large size for $\tilde{\lambda}=0.3$ and $0.2$, which are the key for implementing super-resolution metrology with high probability and fault-tolerant quantum computing. Note that, the Schrödinger cat state also can be experimentally prepared via the homodyne detection on the number state[42, 43], photon subtraction on the squeezed state[44, 45], and high-order nonlinear atom-field interaction[46]. Here the realization of quantum superposition state indicates a spontaneously $\mathbb{Z}_{2}$ breaking, which is evidenced by the nonzero ground-state coherence $\langle\hat{a}\rangle_{\rm sp}$ [37].

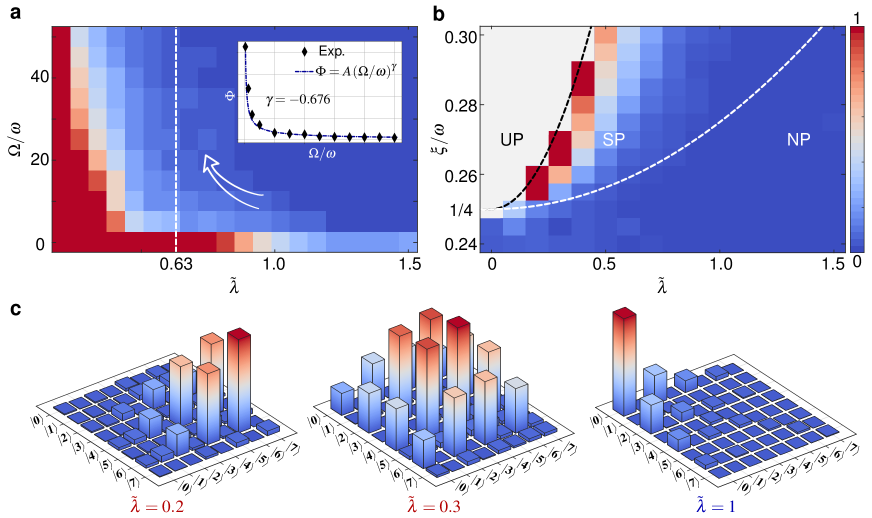
To fully demonstrate the rich equilibrium dynamics induced by the antisqueezing effect, in FIGs. 4a and 4b, we present the experimental ground-state phase diagram characterized by the rescaled ground-state excitation $\Phi$. The realization of reversed SPT are shown again, and it also can be seen from the reduced density matrix $\hat{\rho}_{a}^{s}$ reconstructed experimentally by state tomography. As shown in FIG. 4c, the main contributions to $\Phi$, i.e., $\langle\hat{a}^{\dagger}\hat{a}\rangle_{s}$ (diagonal elements) and $\langle\hat{a}^{2}\rangle_{s}$ (sub-sub diagonal elements), approximately change from zero to finite value along with decreasing $\tilde{\lambda}$. Figure 4a again shows that the dependence of $\Phi$ on $\tilde{\lambda}$ approaches to the case of exactly occurring SPT at the quantum critical point along with increasing $\Omega/\omega$. Furthermore, we measure a series of order parameters $\Phi$ at the critical point for different values of $\Omega/\omega$, showing the finite-frequency scaling for the observable $\Phi$ in the inset of FIG. 4a. The order parameter $\Phi$ vanishes with a power-law scaling, and the fitted finite-frequency scaling exponent $\gamma=-0.676$ is very close to the universal exponent $-2/3$ of the Rabi and Dicke model, which verifies the experimental realization of SPT in finite-frequency regime again. By fixing the value of $\Omega/\omega$, Figure 4b indicates that $\xi/\omega>1/4$ is required for the occurrence of phase transition, which is consistent with the analytical parameter condition $\tilde{\lambda}\geqslant\sqrt{1+\alpha\tilde{\lambda}^{2}-4\xi/\omega}$ of SPT. However, too large antisqueezing strength will lead the system enter into the unstable phase (UP), when the rescaled ground-state excitation becomes an imaginary number. With increasing the spin-oscillator coupling $\tilde{\lambda}$, the competition between the $A^{2}$ and antisqueezing effects will push the system to enter into SP when $\tilde{\lambda}>\sqrt{1+\alpha\tilde{\lambda}^{2}-4\xi/\omega}$, and then enter into NP when $\tilde{\lambda}<\sqrt{1+\alpha\tilde{\lambda}^{2}-4\xi/\omega}$. Our experimental results are approximately agreement with the exact boundaries of different phases, and this consistency becomes better and better along with increasing $\Omega/\omega$ (see FIG. S1 of the Supplementary [37]), which again predicts the occurring of exact SPT in the classical oscillator limit.

In summary, we have presented the first proof-in-principle experimental demonstration of equilibrium SPT beyond no-go theorem induced by the antisqueezing effects. To understand the reappearance of SPT, we experimentally shown the enhanced ZPF by antisqueezing, which ultimately recovers the singularity of the ground state of system. The antisqueezing modulated ground-state phase diagram are presented by experimentally preparing the ground state of system with the adiabatic method. Associating with the SPT, we also experimentally realize the strong entanglement and the squeezed cat state of spins, which provides the new possibilities both for quantum metrology and quantum information processing. Our work is fundamentally interesting in demonstrating that the $A^{2}$ term is not the ultimate limit for experimental observation of equilibrium SPT in the cavity QED system.

Aside from the above results, the observed antisqueezing-enhanced SNR could be used to the general NMR experiments. The defined spin-to-oscillator mapping process is suitable not only for NMR systems, but will also works well in other spin systems, such as trapped ions [47] and NV centers [48]. They opens the new routes for experimentally exploring the novel quantum optical effects with the platform of NMR or other spin systems.