Double free boundary problem for defaultable corporate bond with credit rating migration risks and their asymptotic behaviors
Abstract
In this work, a pricing model for a defaultable corporate bond with credit rating migration risk is established. The model turns out to be a free boundary problem with two free boundaries. The latter are the level sets of the solution but of different kinds. One is from the discontinuous second order term, the other from the obstacle. Existence, uniqueness, and regularity of the solution are obtained. We also prove that two free boundaries are . The asymptotic behavior of the solution is also considered: we show that it converges to a traveling wave solution when time goes to infinity. Moreover, numerical results are presented.
keywords:
Traveling wave; Free boundary problem; PDE with discontinuous leading order coefficient; Asymptotic behavior; Credit rating migration risk modelmyclipboard_main \affiliation[1]organization=School of Mathematical Sciences, Tongji University, city=Shanghai 200092, country=China \affiliation[2]organization=Institut de Mathématiques de Bordeaux, Université de Bordeaux, city=33405 Talence, country=France
1 Introduction
Due to the globalization and complexity of financial markets, the credit risks become more and more important and an unstable factor impacting the market, which might cause a crucial crisis. For example, in the 2008 financial tsunami and the 2010 European debt crisis, credit rating migration risk played a key role. The first step to managing the risks is modeling and measuring them. Thus, it has attracted more and more attention both in academics and in industry to understand these risks, especially default risk and credit rating migration risk.
Most credit risk research falls into two kinds of framework, namely structure model and intensity one. The intensity model assumes that the risk is due to some exogenous factors, which are usually modeled by Markov chains, see [36]. In this way, the default and/or migration times are determined by an exogenous transition intensity; see Jarrow, Lando, and Turnbull [22, 21], Duffie and Singleton [12], to mention a few. In the implementation, intensity transition matrices are usually obtained from historical statistical data. However, it is well-known that companies’ current financial status plays a crucial role in default and credit rating migrations. For example, the main reason which caused the 2010 European debt crisis was that the sovereign debts of several European countries reached an unsustainable level due to their poor economical situation. The crisis happened in these countries because of the downgrading of their credit ratings and the subsequent chain reactions. Therefore, Markov chain model alone cannot fully capture the credit risks.
To include the endogenous factor, the structural model comes into consideration for credit risk modeling, which could be traced back to Merton [35] in 1974. In such a kind of models, the reason for credit rating migration and default is related to the firm’s asset value and its obligation. For example, in Merton’s model, it is assumed that the company’s asset value follows a geometric Brownian motion and a default would happen if the asset value drops below the debt at maturity. Thus, the corporate bond, representing the company’s obligation, is a contingent claim of the asset value. Later, Black and Cox [2] extended Merton’s model to the so-called first passage-time model, where the default would happen whenever the asset value reached a given boundary; see also [26, 33, 27, 6, 39] for related works. Dai et al. [10] considered an optimal control problem in the case where a bank’s asset is opaque.
Using the structural model, Liang and Zeng [32] studied the pricing problem of the corporate bond with credit rating migration risk, where a predetermined migration threshold is given to divide asset value into high and low rating regions, in which the asset value follows different stochastic processes. Hu, Liang, and Wu [19] further developed this model, where the migration boundary is a free boundary governed by a ratio of the firm’s asset value and debt. Some theoretical results and traveling wave properties are also obtained in [30]. Li, Zhang, and Hu [28] studied the numerical method for solving related variational inequality. Later, Fu, Chen, and Liang [17] provided more mathematical analysis and detailed description of the free migration boundary. More extension of this model is considered in [31, 43, 40, 41]. Recently, Chen and Liang [9] also considered the case where upgrade and downgrade boundaries are different. The readers can also refer to the survey paper [8] for a summary.
However, the reason behind the credit rating migration is the default possibility; hence, it is natural to consider a model involving both the credit rating migration and default risks. In [41], as the first step, a predetermined default boundary of asset level is considered. In this paper, we will let the default boundary also depend on the ratio between the stock price and bond value. Therefore, the model will contain two free boundaries. Both of these boundaries are the level sets of the solution but of different types. One is from discontinuous leading second order term as in previous credit rating migration works (for example, see [30]); the other is from a more traditional free boundary problem, i.e. obstacle problem. Using PDE techniques, existence, uniqueness, regularity, and asymptotic behavior of the solution are obtained, which from a theoretical perspective insure the rationality of the model. Numerical results support our theoretical approach. The stability of traveling wave equation will be studied in our future work [3] using the techniques of [1, 4, 5].
This paper is organized as follows. In Section 2, the model is established and the pricing problem is reduced to a system of two parabolic PDEs with two free boundaries. In Section 3, for the sake of both uniform estimates and asymptotic behavior, we consider a traveling wave solution to the original problem. In Section 4, we use a penalization method and simultaneously a regularization of the coefficient of the 2nd order term to approximate the free boundary problem by a smooth Cauchy problem depending on a small parameter . A series of lemmas are proved in order to establish estimates which are independent of . The key point is that the two approximating free boundaries can be separated by a positive distance independent of . In Section 5, the main results are stated, including the existence, uniqueness, and regularity of the solution. In particular, we prove that two free boundaries are . The asymptotic behavior of the solution as time tends to infinity is examined in Section 6. Finally, a numerical method and some computational results are presented in Section 7.
2 The Model
2.1 Assumptions
Let be a complete probability space. We assume that the firm issues a corporate bond, which is a contingent claim of its value. The stock price of the firm admits different dynamics for different credit ratings.
Assumption 2.1 (the firm asset with credit rating migration).
Let denote the firm’s value in the risk neutral world. It satisfies
where is the risk free interest rate, which is positive constant, and
| (2.1) |
represent volatilities of the firm under the high and low credit grades respectively. They are also assumed to be positive constants. is the Brownian motion which generates the filtration .
It is reasonable to assume (2.1), namely that the volatility in high rating region is lower than the one in the low rating region. The firm issues only one zero coupon corporate bond with face value . Let denote the discount value of the bond at time . Therefore, at the maturity time , an investor can get . For simplicity, we assume in the following sections . The rating criterion is based on the ratio between the stock price and liability.
Assumption 2.2 (the credit rating migration time).
High and low rating regions are determined by the proportion between the debt and asset value. The credit rating migration time and are the first moments when the firm’s grade is downgraded and upgraded respectively as follows:
where is a contingent claim with respect to and
| (2.2) |
is a positive constant representing the threshold proportion of the debt and value of the firm’s rating. Also
is the so-called credit discount rate. In this paper, we also make the assumption that
| (2.3) |
Further, we assume that the bond will default when the stock price is too low, compared with the debt.
Assumption 2.3 (the defaultable corporate bond).
The default time is also determined by the proportion of the debt and asset value. Here, we assume that the default happens whenever
The default time is defined as
At the default time, the contract is closed and the investor obtains the cash .
Remark 2.4.
Condition (2.3) is also assumed in [30] to ensure the existence of the travelling wave equation. In finance, if is too small or too large, it is possible that the company will always be low rating or high rating. To see this, assume that the stock price is
where is the volatility of the stock taking values in depending on whether the stock is low rating or high rating. The present value of the bond is . Then, the company’s discounted debt-to-asset ratio is
If , the right hand side will go to as with probability . This implies that the company will always be low rating in the end. On the other hand, if , the right hand side will go to and, hence, the company will always be high rating.
2.2 The Cash Flow
If the bond does not default, once the credit rating migrates before the maturity , a virtual substitute termination happens, i.e., the bond is virtually terminated and substituted by a new one with a new credit rating. There is a virtual cash flow of the bond. We denote by and the values of the bond in high and low grades respectively, which are functions of and . Then, they are conditional expectations of the following
| (2.4) | ||||
| (2.5) |
where
2.3 The PDE problem
In the life time of the bond, by Feynman-Kac formula (see, e.g. [11]), it is not difficult to derive that the letting values and satisfy the following system of partial differential equations in their respective life regions:
| (2.6) | |||
| (2.7) |
If the bond life last to maturity, and satisfy the terminal conditions:
Define the function as
Then, it satisfies the following variational form
with
First, we make some transformation. Let . Then, satisfies
As already indicated in [30], it is more convenient to work in the moving coordinate frame
Then, the equation reads
| (2.8) |
Let us introduce the weight and make the further transformation ; we define
Thus, satisfies the following problem:
| (2.9) |
with
Let us finally define the free boundaries which will play a crucial role throughout the paper, respectively the default boundary
and the transit boundary
Our goal is not only to solve (2.9) but also to study the properties of these boundaries. If the solution is smooth enough, system (2.9) can be rewritten as the free boundary problem
| (2.10) |
For convenience, we set
It follows from (2.3) that and .
3 Traveling Wave Solution
In this section, we will consider the steady state of (2.9), i.e. the traveling wave solution for the original problem. In addition to giving the asymptotic behavior of (2.9), the traveling wave equation is also useful for constructing sub-solutions. The traveling wave solution satisfies
| (3.1) |
Denoting the two free boundaries respectively by and , and assuming that the solution is sufficiently smooth, we may reformulate Equation (3.1) as the following free boundary problem
| (3.2) |
Note that we also add a growth condition at due to the financial nature of our problem.
Theorem 3.1.
System (3.2) has a unique solution such that belongs to and the respective restrictions of to and are .
Proof.
It is elementary to solve the second order system in (3.2):
| (3.3) |
From the boundary conditions at , it comes
This implies that and . Then, from , we have that
| (3.4) |
Define the mapping
| (3.5) |
hence . Since , we have that the mapping is decreasing on . Since and , the transcendental equation (3.4) admits a unique positive solution
| (3.6) |
The interface condition yields that
where the last equality is due to (3.6). Combining with the condition , we have that
This implies that
| (3.7) |
Thus, , and are determined. Summarizing, it comes
| (3.10) |
∎
Some properties of are needed in the sections below. We list them in the following proposition.
Proposition 3.2.
(i) for , , , and if ;
(ii) if and if ;
(iii) for , ;
(iv) is a decreasing function of . Moreover, and .
Proof.
(i) It is straightforward to compute
Since , it holds that for . For , we rewrite
With the notation from Theorem 3.1, we have that
and
Since , it holds that for . Next, it comes
and
Noting that , and , we achieve the desired results.
(ii) It follows immediately from (i).
(iii) We know from (ii) that . On the one hand, thanks to (3.7), hence if (see (3.10)). On the other hand, note that implies that is increasing, which indicates that for .
(iv) Since is decreasing with respect to and , it follows from (3.7) that is decreasing with respect to . It also holds that and , hence the result. ∎
4 Penalized and Regularized Cauchy Problem
Problem (2.9) has singularities: at due to the indicator function in the definition of ; at as in a usual obstacle problem; and at because of the lack of regularity of the initial condition. To address these issues, we introduce , and which depend upon a small positive parameter . These smooth functions are chosen as the following. Let be the Heaviside function, i.e., for and for . Then, in (2.9) reads
First, we approximate by a function such that
Second, let be a smooth penalty function satisfying the following condition:
Let be the unique solution of . It is easy to see that when . Finally, let us define , where , for ; for and for .
From the construction of , we have the following lemma.
Lemma 4.1.
(i) For , ; (ii) .
Proof.
(i) It is easy to see that , hence positive for . Note that for and for . Then, we shall have the second inequality.
(ii) Differentiating , we have that
| (4.1) |
This implies that the minimum is achieved at . Thus,
It is easy to verify that for or . From (4.1), we see that for any . ∎
Now, for small, we consider the following approximated Cauchy problem:
| (4.2) |
where , , and
| (4.3) |
together with the initial condition
| (4.4) |
Hence, from the definition of in the previous, we have that for ; for . We have the following existence result:
Proof.
First, we turn Equation (4.2) into a quasilinear equation whose principal part is in divergence form:
| (4.5) |
with
One can check that and satisfy the assumptions of [25, Chapter V, Theorem 8.1]. Thus, there exists a unique bounded solution for any .444For usual parabolic Hölder spaces, see, e.g., [25, Chapter 1, Section 1],[34, Section 5.1]). Then, and belong to the same function class. Further Hölder regularity follows from classical results for linear problems (see [25, Chapter IV, Theorem 5.1], [34, Theorem 5.1.10]), which yields that . The result follows by bootstrapping. ∎
Remark 4.3.
From the definition of and , it is easy to see that when and when . Thus, when is small enough, at least one of these two equations holds.
4.1 Estimates on the approximating solution
We now proceed to derive necessary estimates on independent of , via the the maximum principle for parabolic equations in unbounded domains (see, e.g., [16, Chapter 2], [37, Chapter 7]). These properties will be inherited by the limit when taking and, thus, are crucial for the analysis of the bond value and free boundaries.
Lemma 4.4.
For sufficiently small, it holds in :
Proof.
Recall that we have introduced a smooth cut-off function in the beginning of this section. Define a function as . Then, we see that for ; for and for . Thus, it holds that if and only if . Furthermore, one can directly check that is bounded. Let and we have that
which can be rewritten as
Since when and when , we see that if is sufficiently small. Noting that , it holds that . As the coefficient of zeroth order term is bounded, one can apply maximum principle to get that , which is equivalent to .
Next, set . Then, verifies
From the definition of , it holds that . Hence, this leads to according again to the maximum principle.
Finally, Let . Then, it holds that
Noting that and , we deduce that according to the maximum principle.
∎
Lemma 4.5.
It holds in :
Proof.
Lemma 4.6.
It holds in :
Proof.
Lemma 4.7.
For sufficiently small , it holds in :
Proof.
At
is non-positive. Now, consider the function . From the definition of and , it is easy to see that when and when . Thus, we divide the space into two parts and
Case 1: .
In this case, we see that . Then, it holds that
| (4.7) |
Case 2: . For sufficiently small , we have that . Then, it holds that
and
where we denote , and for simplicity of the notations. Combining above equations, we have that
where the last inequality is due to the fact that is convex.
Combining these two cases, it holds that
Then, by the maximum principle, .
∎
Lemma 4.8.
It holds in :
Proof.
Set . Then, we see that
| (4.8) |
Because , we have that
| (4.9) |
Since , the first term is negative. Then, it is easy to check that when , the second term is zero. Hence, in this case. Now, it remains only to check the case . From Lemma 4.1 and monotonicity of , we have that
According to our choice of , we see that the above term is non-positive. Thus, we proved that , which yields the desired result thanks to the strong maximum principle. ∎
Lemma 4.9.
There are positive constants and , independent of , such that it holds in
Proof.
Since , and by Hölder continuity of the solution (see Theorem 4.2), there exists a , independent of , such that
where
Thus, for small enough such that , in . We observe that, in , the problem is reminiscent of a vanilla American option, which has a lower estimate (see, e.g., [18])
| (4.10) |
Let us refer to Lemma 4.8 for the notation . From (4.9), it is easy to verify that is uniformly bounded from below on . Combining with (4.10), there exists such that on . The Maximum Principle (see Lemma 4.8) yields that in . Together with (4.10), we get the desired result. ∎
As an immediate corollary, we have
Lemma 4.10.
There are positive constants and , independent of , such that it holds in
4.2 The approximating transit boundary
Let us denote by the approximating transit boundary, which is implicitely defined by the equation
| (4.11) |
We will construct the curve via the Implicit Function Theorem. To begin with, we give a lower bound for . Fom Lemma 4.4, it holds that when . This implies that when and . Then, we give a lower bound for .
Lemma 4.11.
Proof.
From Proposition 3.2 (iv), is well defined. We can rewrite that
with . Let . Then, it holds that
Since we choose , it holds that . Combining with the fact that , we see that the last term on the right hand side is non-positive. Since (see Proposition 3.2 (iii)), . Thus,
At , . It is easy to see that for and for . For both cases, we have . The desired result follows from the maximum principle. ∎
Theorem 4.12.
Proof.
To begin with, we compute
Because , it is clear that if small enough, hence and . Therefore, . We remind that the function is smooth and non-increasing; however, in some neighborhood of such that , the function is decreasing which yields that the initial position of is well-defined by (4.12).
Next, we compute
and (see the proof of Lemma 4.8)
It is now an exercise to apply the Implicit Function Theorem, which shows that there exist , and a unique function such that, if verifies , then . Note that is a decreasing function because
As by product, taking the restriction of to , we have constructed a (small) branch of the curve , of class , such that (4.11) holds for all , . Lemma 4.11 implies that for . Combining with the fact that , we see that .
Lemma 4.13.
For any , there exists a constant , independent of , such that .
Proof.
From the Implicit Function Theorem, it holds:
Note that Lemmas 4.8 and 4.9 implies that is bounded. To prove the desired results, we only need to show that , for some positive . In Lemma 4.7, we proved that , which implies that is non-increasing in . Since is smooth, there exists a point such that
We have shown that and . This yields that
Since is non-increasing, it holds that
This completes the proof. ∎
From Theorem 4.12 and Lemma 4.13, we see that the sequence is bounded in , therefore, extracting a subsequence if necessary, it converges uniformly to a function .
Corollary 4.14.
Extracting a subsequence if necessary, the sequence converges uniformly to a limit .
5 Main Results
5.1 Existence and Uniqueness
Lemmas 4.4-4.7 provide estimates on the approximated solution . By taking a limit as , we are able to derive the existence of a solution to (2.9)-(2.10).
Theorem 5.1.
(i) For any , there exists a sequence such that a.e. in , a.e. in , in weak- and weak-, for any , where . Moreover, extracting a subsequence if necessary, converges uniformly to ;555For , , , is the space of elements of whose derivatives are also in , respectively up to second order in and to first order in . is the space of bounded functions whose derivatives are bounded, respectively up to second order in and first order in . denotes the space of bounded functions whose derivative w.r.t. is also bounded.
(ii) is a solution of the original free boundary problem (2.9);
(iii) satisfies the estimates of Lemmas 4.4-4.7, and the inequality
| (5.1) |
as well as the following growth condition: there exists a constant such that when and when , .
Proof.
Let be a sequence converging to when and consider the corresponding solutions of (4.2) and (4.4). For simplicity, we denote by . According to Lemmas 4.4-4.10, we first observe that the sequence is bounded in the spaces . Second, the sequence is bounded in the space , .
Next, let be a sequence of positive numbers such that as . Let us consider the restriction of to the interval . At fixed , the sequence is bounded in the space for any . One can extract a subsequence, denoted by , which converges a.e. in and weakly in , , as . By a standard diagonal extraction procedure, one can eventually extract a subsequence, say , such that and converge respectively to and almost everywhere in as . After a new extraction, in weak- and weak-.
It is not difficult to see that satisfies the properties of Lemmas 4.4-4.7. Set , (see Lemma 4.7). According to the above results, in weak- which is non-negative in the distribution sense, and, hence, (5.1) holds.
Since the sequence is bounded in (see Lemma 4.13), a subsequence converges to some in . More specifically, in the proof of Lemma 4.13, we showed that , where the constant is independent of . With the estimate of in Lemma 4.10, we deduce that, for any , there exists a small constant independent of such that, for ,
and
Taking the limit as and combining with non-increasing in , we see that if and if . This yields that (see Corollary 4.14).
Moreover, the convergence of to implies the almost everywhere convergence of to . Hence, we have that converges to in weak-. This implies that . It is also easy to verify that whenever . Thus, is a solution to (2.9).
Then, the uniqueness of given by Theorem 5.1 is a direct consequence of the following theorem:
Theorem 5.2.
Let be a solution to (2.9) satisfying
for . Suppose that there exists such that for and for , . Then, it holds .
Proof.
Denote by and . We rewrite that
Let . We prove that . Due to the growth condition on and , it holds that for . Therefore if this conclusion is not true, will achieve a negative minimum at some point . By the parabolic version of Bony’s maximum principle, it holds that
This is equivalent to
By the continuity of , we derive in a small parabolic neighborhood of . It follows that
In this neighborhood, we also have that
Therefore,
which is a contradiction. Thus, we proved that . Similarly, the reverse inequality holds, which yields the uniqueness result. ∎
5.2 Properties of the free boundaries
For the original problem (2.9), we already introduced formally the default boundary and the transit boundary , see System (2.10). The goal of this subsection is to to define the free boundaries rigorously and prove some basic properties.
The default boundary
Let us remind that , see Lemma 4.11. Taking the limit as , this implies that . Since for , it holds that the set is bounded from below. Now, we are in position to define
| (5.2) |
Then, indicates that is also bounded from above. Thus, we will have the following result.
Theorem 5.3.
For each , is well-defined, i.e. we have . Moreover, for and whenever .
The transit boundary
We remind that is the limit of (see Theorem 5.1). Thus, we will have the following theorem.
Theorem 5.4.
The initial positions of the free boundaries are as follows:
Furthermore, and are non-increasing with respect to .
Proof.
In the following, we will prove the smoothness of the free boundaries. Note that the uniform lower bound in Lemma 4.5 implies that there exists a constant such that whenever . Then, one can choose a smooth function such that and for sufficiently small . Therefore, separates the default boundary and the transit boundary . So, we can discuss them one by one with cut-off functions being applied when necessary.
We first study the default boundary. The proof is essentially the same as that in [42], where the authors proved the smoothness of free boundary in American option problem. Thus, we just give a sketch of the proof for readers’ convenience. We make the change of variable and set . For suitable , we have that . It holds that
which implies the continuity of at . From the definition of , one can prove that is continuous in . Applying a result from Cannon et al. [7], we will have that . Then, we may use the theory of parabolic equations to improve the regularity of by bootstrapping. Repeating the procedure yields the following result.
Theorem 5.5.
.
Next, we consider the smoothness of the transit free boundary . For this purpose, we need the following lemma of the parabolic diffraction problem. The proof is essentially similar to that in [29]; hence, we just give a sketch.
Lemma 5.6.
In the domain , where are some constants, consider the following initial boundary problem
| (5.3) |
where , , , for , ,, for , and are positive constants. Then, the problem (5.3) admits a solution, and
Moreover, there exists a positive constant and depend only on the given data such that
Proof.
Make the transformation , , then problem (5.3) satisfies
| (5.4) |
where if , if . By well-known estimates for linear parabolic PDEs with discontinuous coefficients whose principal part is in divergence form (see [25, Chapter III, 5]), and the proof of [29, Theorem 1.1], the claim of this lemma follows. ∎
Now, we are in position to prove the smoothness of .
Theorem 5.7.
.
Proof.
In a neighborhood of , satisfies the system
| (5.5) |
Thus, it holds that
| (5.6) |
which means that
| (5.7) |
Set . From (5.5) and (5.7), it turns out that verifies the system
| (5.8) |
According to the free boundary condition, satisfies a typical Verigin problem, see [29, 38]. In particular, the regularity of the free boundary was proved in [29]. Therefore, we may obtain the same result for our problem in a similar manner. To see this, note that the free boundary is Lipschitz continuous and satisfies
| (5.9) | |||||
which is a kind of Stefan condition, see [23, 24, 14] for references. Applying Lemma 5.6 to problem (5.8) (up to some simple transformation), up to the free boundary. Furthermore, by Lemma 4.6, has a negative upperbound. Then, the right hand side of (5.9) belongs to . This implies in turn . In this way, by an iteration process, one can further improve the regularity of and shows eventually that it belongs to ∎
6 Asymptotic Convergence
In this section, we will prove that converges to the traveling wave solution as goes to . Since is non-positive, we see that, for any ,
Note that for , and which implies the integrability of over . Thus, we have that
Letting tend to infinity, we get that there exists a constant such that
| (6.1) |
Now let and consider as a sequence of functions defined on . Lemmas 4.4-4.10 indicate that it is a bounded sequence in . As in the proof of Theorem 5.1, via a standard diagonal extraction procedure there exists a function and a subsequence such that such that and converge respectively to and almost everywhere in . After a new extraction if necessary,
Since non-positivity is preserved under weak- convergence and , one can deduce that . Since (6.1) implies that as , we have that . Combining with the non-positivity of , it follows that which means that is only a function of . Then, the following properties pass from to ,
Since and are also non-increasing with respect to , they also admit limits at , which are denoted as and respectively. Then, one can verify that and . For any interval such that , there exists such that for any . In , it holds that
Taking subsequence , we derive that
Since is arbitrary, it holds that
Similarly, we can also show that
Note that implies that . Combining with the fact that , we see that it is a solution to (3.2), i.e.
Then, interior estimate implies that is smooth in and . Now, from the uniqueness of the solution, we derive that . Since any sub-sequential limit must be same, the full sequence must converge as goes to . We have proved the local convergence of . But, noting that for and , the convergence is also uniform over . Finally, we prove the following result.
Theorem 6.1.
As goes to , converges uniformly to .
7 Numerical Results
In this section, we will give some numerical results for illustration. As represents the value of the bond, we will come back to (2.8) instead of (2.9) which will give us more clear financial meaning.
7.1 Numerical Scheme
As our problem is non-standard, we will introduce the numerical scheme first. To solve the free boundary problem, we use an explicit-implicit finite difference scheme combined with Newton iteration to solve the penalized equation. The first step is to discretize the equation. Let , and . will be the approximation of the solution of (2.8) at mesh point . Consider the approximating penalized equation
Here with be a proper smooth function. For numerical convenience, we use the penalty function . In the numerical experiment, we choose
as proposed in [28]. Note that the left hand side is a nonlinear operator since coefficients depend on . In the numerical implement, we determine these coefficients with function value from previous time step. For illustration, let us perform discretization at . Denote by . The first order term is discretized by the upwind scheme, i.e.
We use the fully implicit approximation to the temporal term
and the usual discretization for the second order term
Thus, given function value at previous time step, current value is obtained by solving the following equation
| (7.1) |
for . Here the matrix is determined by and is a sparse -matrix due to our discretization scheme.
Now, we have to solve the nonlinear equation (7.1). We adopt the method used by [13] to value American options. For illustration, let us recall the classical Newton iteration for finding the root of a convex function . Given an initial guess, the point is updated as
which is equivalent to say that solves
It is easy to see that the left hand side of above equation is an first order approximation of at . Similarly, we can solve (7.1) with Newton iteration. Denote as the approximation at for th iteration. Then, solves the linearized equation
| (7.2) |
When the difference between and is small enough, we stop the iteration and set equals . Moreover, the initial guess is chosen to be .
In summary, we have the following iterative algorithm.
Algorithm 1 Explicit-Implicit Finite-difference Iterative Algorithm
7.2 Numerical Results
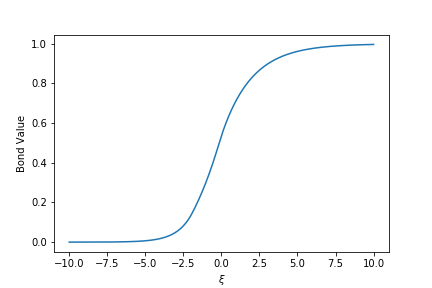
In the numerical experiment, we set the model parameters as and . For discretization, we have and . We also choose , . Having numerically solved (3.6), we are able to plot the traveling equation for (2.8), which is .
Next, we plot the numerical solution for (2.8) and compared it with the traveling wave equation in Figure 2.
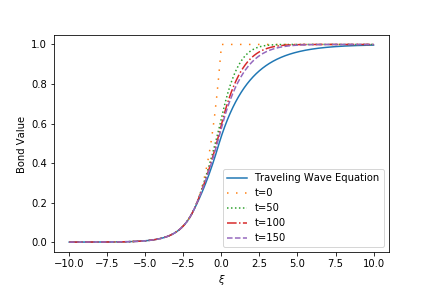
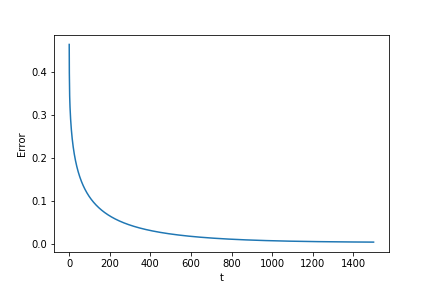
It seems that the solution will converge to the traveling wave equation as goes to infinity as the theoretical result indicates. To numerically check this, we compute the solution for large time and plot the error between the solution and the traveling wave equation. The result is shown in Figure 3. The error is defined as the supreme norm between the traveling wave equation and the value function at time . We see that the error is monotone decreasing with respect to . The final error is about at time .
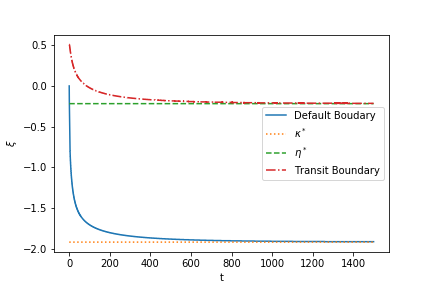
Finally, we plot the default and transit boundaries as a function of and compare them with those of traveling wave equation. The result is shown in Figure 4. It is clear that the boundaries are decreasing with respect to which is consistent with our previous theoretical analysis. We also see the convergence of two boundaries. \Copyr1major2
References
- [1] D. Addona, C.-M. Brauner, L. Lorenzi and W. Zhang, Instabilities in a combustion model with two free interfaces, J. Differ. Equ. 268 (2020), 396–4016.
- [2] F. Black and J. Cox, Some Effects of Bond Indenture Provisions, Journal of Finance, 1976, 31:351-367.
- [3] C.-M. Brauner, Y. Dong, J. Liang and L. Lorenzi, Stability of a free boundary problem arising from credit rating migration problem, in preparation.
- [4] C.-M. Brauner, J. Hulshof and A. Lunardi, A general approach to stability in free boundary problems, J. Differential Equations 164 (2000), 16-48.
- [5] C.-M. Brauner, L. Lorenzi and M. Zhang, Stability analysis and Hopf bifurcation for large Lewis number in a combustion model with free interface, Ann. Inst. H. Poincaré Anal. Non Linéaire 37 (2020), 581-604.
- [6] E. Briys and F. de Varenne, Valuing Risky Fixed Rate Debt: An Extension, Journal of Financial and Quantitative Analysis, 1997, 32: 239-249.
- [7] J. Cannon, D. Henry and D. Kotlow Continuous differentiability of the free boundary for weak solutions of the Stefan problem, Bulletin of the American Mathematical Society 80 (1974),45-48.
- [8] X. F. Chen, B. Hu, J. Liang, and H. M. Yin, The free boundary problem for measuring credit rating migration risks, Scientia Sinica Mathematica, 54, (2024), 1-28
- [9] X.F. Chen and J. Liang, A Free Boundary Problem for Corporate Bond Pricing and Credit Rating under Different Upgrade and Downgrade Thresholds, SIAM Financial Mathematics. Vol 12 (2021), 941-966
- [10] M. Dai, S. Huang & J. Keppo, Opaque bank assets and optimal equity capital, Journal of Economic Dynamics and Control 100 (2019), 369-394.
- [11] A. K. Dixit, S. Pindyck, Investment under Uncertainty, Princeton University Press, 1994.
- [12] D. Duffe and K. J. Singleton, Modeling Term Structures of Defaultable Bonds, The Review of Financial Studies 1999, 12: 687-720.
- [13] P. A. Forsyth and K. R. Vetzal Quadratic convergence for valuing American options using a penalty method SIAM Journal on Scientific Computing 23:6(2002), 2095–2122.
- [14] A. Friedman, Variational Principles and Free Boundary Problems, John Wiley and Sons, New York 1982.
- [15] A. Friedman, Parabolic variational inequalities in one space dimension and smoothness of the free boundary, J. Funct. Anal. 18 (1975) 151-176.
- [16] A. Friedman, Partial differential equations of parabolic type, Courier Dover Publications, 2008.
- [17] W. Fu, X. F. Chen and J. Liang, Pricing bond under the consideration of variable credit rating, to appear in Interfaces and Free Boundaries, 2019.
- [18] M.G. Garrori, J.L. Menaldi, Green Functions for Second Order Parabolic Integro-Differential Problems, Longman Scientific & Technical, New York, 1992.
- [19] B. Hu, J. Liang and Y. Wu, A Free Boundary Problem for Corporate Bond with Credit Rating Migration, J. Math. Anal. Appl. 428 (2015) 896-909.
- [20] B. Hu, Blow-up theories for semilinear parabolic equations, Springer, 2011.
- [21] R. A. Jarrow, D. Lando and S. M.Turnbull, A Markov model for the term structure of credit risk spreads, Review of Financial studies 10(2) (1997), 481-523.
- [22] R. Jarrow and S. Turnbull, Pricing Derivatives on Financial Securities Subject to Credit Risk, Journal of Finance 50(1995), 53-86.
- [23] L.Jiang, Stefan Problem (II) Acta Mathematica Sinica Vol 13, No.4 (1964) 33-49.
- [24] L.Jiang, Existence and Differentiation of the Two-phase Stefan Problem for Quasilinear Parabolic Equations Acta Mathematica Sinica Vol 15, No.6 (1965) 749-764.
- [25] O.A. Ladyzenskaja, V.A. Solonnikov and N.N. Uralceva, Linear and Quesilinear Equations of Parabolic Type, Nauka Moscow, 1967.
- [26] H. Leland, Corporate debt value,bond covenants,and optimal capital structure, Journal of Finance 49 (1994), 1213-1252.
- [27] H. Leland and K.B. Toft, Optimal capital structure,endogenous bankruptcy and the term structure of credit spreads, Journal of Finance 51(3) (1996), 987-1019.
- [28] Y. Li, Z. Zhang and B. Hu Convergence Rate of an Explicit Finite Difference Scheme for a Credit Rating Migration Problem SIAM Journal on Numerical Analysis 56(4)(2018),2430-2460.
- [29] J. Liang, The One-Dimensional Quasilinear Verigin Problem, J. Partial Differential Equations, 4, No.2 (1991) 74-96.
- [30] J. Liang, Y. Wu, B. Hu, Asymptotic traveling wave solution for a credit rating migration problem, J. Differ. Equations 261, 1017-1045.
- [31] J. Liang, H. M. Yin, , X. F. Chen and Y. Wu, On a Corporate Bond Pricing Model with Credit Rating Migration Risks and Stochastic Interest Rate, Quantitative Finance and Economics,2017, 1(3): 300-319.
- [32] J. Liang and Z.K. Zeng, Pricing on Defaultable and Callable bonds with credit rating migration risks under structure framework, Applied Mathematics A Journal of Chinese Universities(Ser.A), 2015, 61-70.
- [33] F. Longstaff and E. Schwartz, A Simple Approach to Valuing Risky Fixed and Floating Rate Debt, Journal of Finance, 1995, 50: 789-819.
- [34] A. Lunardi, Analytic Semigroups and Optimal Regularity in Parabolic Problems, Birkhäuser, Basel, 1996.
- [35] R.C. Merton, On the Pricing of Corporate Debt: The Risk Structure of Interest Rates, Journal of Finance, 1974, 29:449-470.
- [36] A.A. Markov, Rasprostranenie zakona bol’shih chisel na velichiny, zavisyaschie drug ot druga, Izvestiya Fiziko-matematicheskogo obschestva pri Kazanskom universitete, 2-ya seriya, 15, 1906, 135-156.
- [37] C. V. Pao, Nonlinear parabolic and elliptic equations. Springer Science & Business Media, 2012.
- [38] Y. Tao and F. Yi, Classical Verigin Problem as a Limit Case of Verigin Problem with Surface Tension at Free Boundary, Appl. Math. -JCU, 11B(1996) 307-322.
- [39] K. Tsiveriotis and C. Fernandes, Valuing convertible bonds with credit risk, The Journal of Fixed Income 1998 8(2): 95-102.
- [40] Y. Wu and J. Liang, Free boundaries of credit rating migration in switching macro regions, Mathematical Control and Related Fields 10 (2020) 257-274.
- [41] Y. Wu , J. Liang, and B. Hu, A free boundary problem for defaultable corporate bond with credit rating migration risk and its asymptotic, Discrete and Continuous Dynamical System Series B, 25, (2020) 1043-1058.
- [42] C. Yang, L. Jiang and B. Bian Free boundary and American options in a jump-diffusion model European Journal of Applied Mathematics. 17:1(2006), 95–127.
- [43] H. M. Yin, J. Liang and Y. Wu, On a New Corporate Bond Pricing Model with Potential Credit Rating Change and Stochastic Interest Rate, accepted by Journal of Risk and Financial Management, 11(4) (2018), 87.