Control of a spin qubit in a lateral GaAs quantum dot based on symmetry of gating potential

Every quantum two-level system can act as the quantum bit, a basic unit of quantum information processing NC10 ; BdV . Among different solid-state implementations of the qubit system LdV98 ; OTW+99 ; NPT99 ; YTB+12 , single-electron spin in a semiconductor quantum dot (QD) can be used to achieve the task. In order to manipulate spins of charge carriers embedded inside a semiconductor material electrically, through electric dipole spin resonance (EDSR) R60 , the presence of spin-orbit interaction (SOI) is obligatory.

Besides its positive effect in EDSR based schemes GBL06 ; BL07 ; NKN+07 ; R08 ; BSO+11 ; KSW+14 ; TYO+18 ; KLS19 ; SKT+19 , SOI enables the electron-phonon coupling mediated transitions between the qubit states KN00 ; PF05 ; S17 ; LLH+18 , affecting the spin qubit lifetime. To suppress the coupling to phonons, different approaches like the optimization of the QD design CBG+07 ; MSL16 or control of the system size CM07 were suggested. The observed anisotropy of the spin relaxation rate on the in-plane magnetic field orientation FAT05 offered another playground for fine-tuning of the spin qubit’s desired properties. In circular QDs, this is the only degree of freedom accessible in the optimization of the spin qubit, while for the elliptical confining potential SKS+14 ; MSL16 ; OS07 ; AMR+08 orientation of the QD potential with respect to the crystallographic frame can be used as the tuning parameter.

Evidently, different symmetry of the gating potential PRC15 is the main reason for the observed behavior. But to what extent can the potential symmetry alter the basic properties of the electrically controlled spin qubit? To address this question, we have performed a general analysis valid for the lateral GaAs QD system with ${\bf C}_{n{\rm v}}$ or ${\bf C}_{n}$ symmetry of the gating potential. Besides the expected anisotropy on the magnetic field orientation, we were able to find potential symmetries for which the QD orientation with respect to the crystallographic frame can act as another control parameter of the spin qubit characteristics. With our theory, we offer a simple and efficient way to determine the impact of the gating potential on the Rabi frequency and spin relaxation rate. This is shown in the example of anisotropic and isotropic harmonic potential, as well as for the infinite-wall equilateral triangle, square, and rectangular potential.

This paper is organized as follows. In Section II we define a single-electron GaAs spin qubit model. In Section III we define the dipole moment of the electrically controlled spin qubit that describes both the Rabi frequency and SOI-induced spin relaxation rate mediated by acoustic phonons. In Section IV we present the main results of the paper: analytical expressions for the dipole moment in the case of the gating potential with ${\bf C}_{n{\rm v}}$ or ${\bf C}_{n}$ symmetry. In Section V, to illustrate the impact of the gating potential on the spin qubit lifetime we use the obtained expressions to compare the influence of the harmonic confinement with an infinite-wall equilateral triangle, square, and rectangular potential. In Section VI we give our conclusions.

We start with the Hamiltonian describing the lateral dynamics of a single-electron in the GaAs material

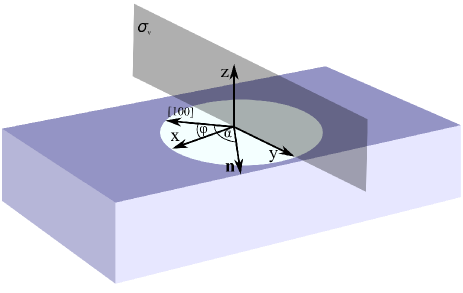
where $p_{x}$ and $p_{y}$ are the momentum operators, $m^{*}$ is the effective mass ($m^{*}=0.067m_{e}$ for GaAs, $m_{e}$ is the electron mass), while $V(x,y)$ is the gating potential used to localize the electron in a QD. In the lateral system, symmetries that can be present are the $n$-fold rotational symmetry and the vertical mirror plane symmetry $\sigma_{\rm v}$. For simplicity, we assume that $\sigma_{\rm v}$ coincides with the $yz$ plane of the QD coordinate frame (see FIG. 1). Thus, we assume a general form of the orbital Hamiltonian $H_{0}$ that has a ${\bf C}_{n{\rm v}}$ or ${\bf C}_{n}$ ($n=\infty$ also) symmetry. Due to the symmetry, eigenenergies and eigenvectors of $H_{0}$ can be classified according to the irreducible representations (IRs) of a given point-group symmetry.

Besides $H_{0}$, in Eq. (1) the Zeeman term $H_{\rm z}$ appears, describing the coupling of spin and magnetic field:

where $g$ is the effective Landé factor ($g\approx-0.44$ for GaAs), $\mu_{B}$ is the Bohr magneton, ${\bm{s}}=1/2{\bm{\sigma}}$ is the electron’s spin, and ${\bm{B}}=B{\bm{n}}$ is the in-plane magnetic field forming an angle $\alpha$ with the crystallographic [100] axis. In Eq. (1) we have neglected the orbital effects of the in-plane magnetic field. This is a reasonable assumption for the magnetic field strength weaker than a few ${\rm T}$ RSF11 . In the case of the magnetic field applied in the $z$ direction, orbital effects would be much more pronounced RSF11 .

Eigenstates of $H_{0}+H_{\rm z}$ can be written in a direct product form $|{\Psi_{i}\pm}\rangle=|{\Psi_{i}}\rangle\otimes{|{\pm}\rangle}$, where $|{\Psi_{i}}\rangle$ corresponds to the eigenvectors of the Hamiltonian $H_{0}$ with an energy $\epsilon_{i}$, while $|{\pm}\rangle$ represents eigenvectors of $H_{\rm z}$ with spin projection parallel or antiparallel to the magnetic field direction and an eigenenergy $\pm g\mu_{B}B/2$, respectively. The effect of $H_{\rm z}$ on the eigenspectra of $H_{0}$ can be seen as the splitting of $H_{0}$ eigenenergies into two branches with an energy difference $|g|\mu_{B}B$. In this work, we assume that $|g|\mu_{B}B$ is much weaker than the energy difference between the ground and the first excited state of the orbital Hamiltonian $H_{0}$.

Besides $H_{0}$ ($H_{\rm z}$) that acts trivially in the spin (orbital) space, the SOI Hamiltonian does not commute with $H_{0}+H_{\rm z}$. It consists of two terms, Dresselhaus DR and Rashba RA : the Dresselhaus term exists due to the bulk inversion asymmetry of the structure, while the Rashba term is present when an electric field perpendicular to the growth direction is applied. The form of spin-orbit coupling is dependent on the structure’s symmetry. For GaAs, having the zincblende structure, the SOI Hamiltonian is equal to

where $\alpha_{\rm r}$ and $\alpha_{\rm d}$ are Rashba and Dresselhaus coupling constants, while $p_{x}^{\rm c}$ and $p_{y}^{\rm c}$ are momentum operators in the [100] and [010] crystallographic directions, respectively. The electron spin is locked to the crystal momentum, since the potential trap confines electron of the crystal. Thus, an electron in a QD inherits the features of the crystal for which the crystal momentum is only appropriately defined. However, we have the choice to define the $x$ axis of our coordinate frame independently on the crystallographic [100] direction. Assuming that the angle between them is $\varphi$, $p_{x}^{\rm c}$ and $p_{y}^{\rm c}$ should be written in terms of momentum operators in the chosen frame: $p_{x}^{\rm c}=p_{x}\cos{\varphi}-p_{y}\sin{\varphi}$, $p_{y}^{\rm c}=p_{x}\sin{\varphi}+p_{y}\cos{\varphi}$.

The spin-orbit Hamiltonian can be written in a different form using the Rashba $l_{\rm r}=\hbar^{2}/2m\alpha_{r}$ and Dresselhaus $l_{\rm d}=\hbar^{2}/2m\alpha_{d}$ precession lengths,

To compare the ratio of the spin-orbit precession length and the orbital confinement length $l$, we redefine $l_{\rm r}$ and $l_{\rm d}$ in terms of the overall spin-orbit length $l_{\rm so}$ and the spin-orbit angle $\nu$:

Since we assume no doping of the GaAs material SL05 , $l_{\rm so}$ can be considered constant. Moreover, the relation $l_{\rm so}\gg l$ RSF11 ; RPS+14 is satisfied in GaAs QDs, meaning that SOI can be treated as a perturbation.

Without SOI, qubit states can be defined as $|{\Psi_{0}\pm}\rangle=|{\Psi_{0}}\rangle\otimes|{\pm}\rangle$, where $|{\Psi_{0}}\rangle$ corresponds to the ground state of the spin-independent Hamiltonian $H_{0}$. Because SOI can be treated on the level of a perturbation, we calculate first-order corrections of the qubit states due to spin-orbit coupling. Since it is known that the standard perturbation technique badly incorporates the spin-orbit-induced corrections CWL04 ; BSF10 , we follow the procedure explained in Ref. MSL16 : the Hamiltonian $H$ is transformed using the unitary operator $U={\rm exp}({\rm i}{\bf n}_{\rm so}\cdot{\bm{s}})$, defined with the help of the position-dependent spin-orbit vector ${\bf n}_{\rm so}=l_{\rm so}^{-1}(r_{1}\sin{\nu}+r_{2}\cos{\nu},-r_{1}\cos{\nu}-r_{2}\sin{\nu},0)$:

The unitary operator $U$ does not change the orbital and Zeeman Hamiltonian. On the other hand, the SOI Hamiltonian $H_{\rm so}$ is transformed into

where $l_{z}=-{\rm i}(r_{1}\partial_{r_{2}}-r_{2}\partial_{r_{1}})$ is the orbital angular momentum. Using $H_{\rm so}^{\rm eff}$, the first-order correction of the qubit states can be written as

where the sum over $i\neq 0$ corresponds to all orbital eigenvectors $|{\Psi_{i}}\rangle$ different from the ground state $|{\Psi_{0}}\rangle$, while $\sigma^{\prime\prime}=\pm$.

The lateral QD model is valid if the electron dynamics in the $z$ direction is suppressed; i.e., an electron is always in the ground state. Thus, we assume that confinement length in the $z$ direction is much stronger than in the $xy$ plane. The Hamiltonian describing the quantum confinement in the $z$ direction is equal to $H(z)=p_{z}^{2}/2m^{*}+V(z)$, where $V(z)=eE_{0}z$ for $z\geq 0$ and $V(z)=\infty$ for $z<0$. To this Hamiltonian corresponds the following ground state (for $z>0$) SS03

where ${\rm Ai}$ is the Airy function, while $\chi=(2m^{*}eE_{0}/\hbar^{2})^{1/3}$ is the inverse of the characteristic length $z_{0}=1.5587/\chi$ in the $z$ direction.

In order to simplify the notation, in the rest of the paper we assume that $|{\uparrow}\rangle$ and $|{\downarrow}\rangle$ represent SOI corrected qubit states in the $xy$-plane, while $|{\Psi_{\uparrow}}\rangle=|{\uparrow}\rangle\Psi_{0}(z)$ and $|{\Psi_{\downarrow}}\rangle=|{\downarrow}\rangle\Psi_{0}(z)$ correspond to wavefunctions of the qubit states in three dimensions.

Electrical control of the spin qubit is possible by applying the in-plane oscillating electric field ${\bf E}\cos{\omega t}$, resulting in the Rabi Hamiltonian $H_{\rm R}=e{\bf E}\cdot{\bf r}\cos(\omega t)$. The Rabi frequency, measuring the speed of the single-qubit rotations, is equal to $\Omega=e/\hbar|{\bf E}\cdot\langle{\uparrow}|{\bf r}|{\downarrow}\rangle|$, where

is the dipole moment (in $e$ units), present due to the SOI induced spin mixing mechanism. Misalignment of the applied field direction and the dipole moment leads to a trivial suppression of the Rabi frequency. Since it is beneficial to increase the Rabi frequency as much as possible, the electric field should be applied in the direction of the dipole moment. Thus, for fixed $|{\bf E}|$, the maximal value $\max(\Omega)=\Omega_{\uparrow\downarrow}$ of the Rabi frequency

is completely dependent on the strength of the dipole moment.

Since spin-phonon interaction in semiconductor QDs is irrelevant KN00 , unlike donor-bound electrons in direct band-gap semiconductors LKD+16 , only electron-phonon-induced transition between the qubit states should be considered in the study of spin relaxation. Electron-phonon coupling is triggered by the SOI-induced admixture mechanism, being highly dependent on the symmetry of the gating potential LKD+16 . We determine the rate of spin relaxation at $T=0$ from the Fermi golden rule,

assuming the dominant contribution of acoustic phonons, having an energy $\hbar\omega_{\nu{\bf q}}$, equal to the level separation between the qubit states, $\epsilon_{\uparrow\downarrow}=|g|\mu_{B}B$. For magnetic field strengths up to a few T, relevant for this work, the linear dependence of phonon frequencies on the crystal wave vector length can be used, $\omega_{\nu{\bf q}}=c_{\nu}|{\bf q}|$, giving us $|{\bf q}|=|g|\mu_{B}B/\hbar c_{\nu}$ coorframe .

The geometric factor $|M_{\nu}({\bf q})|^{2}$ is dependent on the phonon mode, longitudinal (LA) or transverse (TA). The longitudinal geometric factor CBG+06

depends on both $D$ and $h_{14}$, representing the deformation and piezoelectric constant, respectively. On the other hand, the transverse geometric factor CBG+06

is dependent on the piezoelectric constant solely. Other parameters for the GaAs material are CWL04 ; MSL16 $c_{\rm LA}=5290{\rm m}/{\rm s}$, $c_{\rm TA}=2480{\rm m}/{\rm s}$, $\rho=5300{\rm kg}/{\rm m^{3}}$, $D=7{\rm eV}$, $eh_{14}=1.4\times 10^{9}{\rm eV}/{\rm m}$, and $\epsilon=12.9$.

Finally, in Eq. (12) both the lateral and the $z$-direction confinement enter the relaxation rate through the scattering matrix element $|\langle{\Psi_{\uparrow}}|{\rm e}^{{\rm i}{\bf q}\cdot{\bf r}_{\rm c}}|{\Psi_{\downarrow}}\rangle|^{2}$. We employ the dipole approximation ${\rm e}^{{\rm i}{\bf q}\cdot{\bf r}_{c}}\approx 1+{\rm i}{\bf q}\cdot{\bf r}_{c}$, justified for magnetic field strengths below a few T.

To summarize, the phonon-induced relaxation rate can be divided into three separate channels: the deformation phonons $\Gamma_{\uparrow\downarrow}^{\rm def}$, the longitudinal piezoelectric phonons $\Gamma_{\uparrow\downarrow}^{\rm piez,LA}$, and the transverse piezoelectric phonons $\Gamma_{\uparrow\downarrow}^{\rm piez,TA}$. In GaAs QDs, $\Gamma_{\uparrow\downarrow}^{\rm piez,TA}$ is the dominant relaxation channel, being two orders of magnitude stronger than $\Gamma_{\uparrow\downarrow}^{\rm piez,LA}+\Gamma_{\uparrow\downarrow}^{\rm def}$ in the dipole approximation regime. Thus, we can identify the total relaxation rate with $\Gamma_{\uparrow\downarrow}^{\rm piez,TA}$ CSZ+18 :

where $K_{\rm TA}=|g|\mu_{B}B/\hbar c_{\rm TA}$. We assume a typical confinement length $l=10$nm RSF11 ; RPS+14 of the GaAs QD in an experimental setup and magnetic field up to a few T (see Section II). Since confinement in the $z$ direction is much stronger than in the $xy$ plane, $z_{0}\ll l$, we conclude that $7K_{\rm TA}^{2}z_{0}^{2}/33$ is much weaker than 1. In other words, the influence of the confinement in the $z$ direction can be neglected.

Note that $\Gamma_{\uparrow\downarrow}$ is squarely dependent on the absolute value of the dipole moment, meaning that the knowledge of the dipole moment is sufficient to fully explain the behavior of both the Rabi frequency and the spin relaxation rate.

Based on the previous conclusion, we come to the main objective: to derive symmetry allowed expression for the dipole moment. The results can be divided into three cases, according to the system’s group symmetry: (1) ${\bf C}_{n{\rm v}}$ ($n\geq 3$) and ${\bf C}_{\infty{\rm v}}$, (2) ${\bf C}_{2{\rm v}}$ and ${\bf C}_{1{\rm v}}$, and (3) ${\bf C}_{n}$ and ${\bf C}_{\infty}$.

The results presented in the previous Section fully explain the dependence of the Rabi frequency and spin relaxation rate on the spin-orbit angle, magnetic field direction, and the relative orientation of the gating potential with respect to the crystallographic frame.

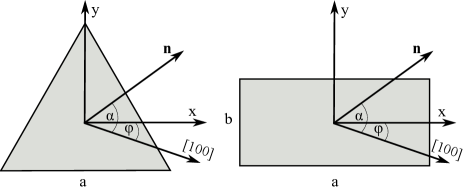
However, symmetry arguments alone cannot provide us with a qualitative estimation of the spin relaxation rate, corresponding to the phonon-allowed spin qubit lifetime. Since $\Gamma_{\uparrow\downarrow}$ is known for the harmonic gating MSL16 , we wish to compare the phonon-induced spin relaxation rate of other confinement potentials with the known values. To this end, we analyze the spin qubit confined inside the infinite-wall equilateral triangle, square, and rectangular gating potential (see FIG. 3):

In the first case, Eq. (38), the potential has ${\bf C}_{3{\rm v}}$ symmetry and the corresponding eigenvectors of the spin-independent Hamiltonian $H_{0}$ transform according to the one-dimensional IRs $A_{0}$ and $B_{0}$ and two-dimensional $E_{1}$ IR of the ${\bf C}_{3{\rm v}}$ group. The set of eigenenergies $\epsilon_{p,q}^{\rm tqd}$ and eigenvectors $\psi^{A_{0}}_{p,q}$, $\psi^{B_{0}}_{p,q}$, and $\psi^{E_{1}\pm}_{p,q}$ LB85 are dependent on two parameters $p$ and $q$ that have different sets of allowed values for each IR. Their concrete form is given in Appendix A.

In the second case, Eq. (41), the symmetry of the potential is dependent on the ratio $k=b/a\in(0,1]$: if $k=1$, the symmetry of the problem is ${\bf C}_{4{\rm v}}$; otherwise, ${\bf C}_{2{\rm v}}$ is the symmetry of the spin-independent Hamiltonian $H_{0}$. In both situations, eigenenergies and eigenvalues can be found by using the separation of variables. The set of eigenenergies $\epsilon_{p,q}^{\rm rqd}$ and eigenvectors $\psi_{p,q}^{\rm rqd}$ in this case is