Compact embeddings for fractional super and sub harmonic functions with radial symmetry

Jacopo Bellazzini Jacopo Bellazzini
Dipartimento di Matematica
Università di Pisa
Largo B. Pontecorvo 5, 56100 Pisa, Italy and Vladimir Georgiev V. Georgiev
Dipartimento di Matematica Università di Pisa Largo B. Pontecorvo 5, 56100 Pisa, Italy
and
Faculty of Science and Engineering
Waseda University
3-4-1, Okubo, Shinjuku-ku, Tokyo 169-8555
Japan and Institute of Mathematics and Informatics, Bulgarian Academy of Sciences, Acad. Georgi Bonchev Str., Block 8, 1113 Sofia, Bulgaria

Abstract.

We prove compactness of the embeddings in Sobolev spaces for fractional super and sub harmonic functions with radial symmetry. The main tool is a pointwise decay for radially symmetric functions belonging to a function space defined by finite homogeneous Sobolev norm together with finite $L^{2}$ norm of the Riesz potentials. As a byproduct we prove also existence of maximizers for the interpolation inequalities in Sobolev spaces for radially symmetric fractional super and sub harmonic functions.

Key words and phrases:

Interpolation inequalities, fractional Sobolev inequality, Riesz potential, radial symmetry, compact embeddings

2010 Mathematics Subject Classification:

Primary 46E35; Secondary 39B62

The authors thank N. Visciglia and L. Forcella for the reading of a preliminary version of the paper. J.B. and V.G. were partially supported by “Problemi stazionari e di evoluzione nelle equazioni di campo non-lineari dispersive” of GNAMPA 2020 and by the project PRIN 2020XB3EFL by the Italian Ministry of Universities and Research. V.G. was partially supported by by the Top Global University Project, Waseda University, by the University of Pisa, Project PRA 2018 49 and by Institute of Mathematics and Informatics, Bulgarian Academy of Sciences.

1. Introduction

The classical embedding in Sobolev spaces $H^{S}(\mathbb{R}^{d})\subset\dot{H}^{r}(\mathbb{R}^{d})$ for $0\leq r\leq S$ follows from the interpolation inequality in homogeneous Sobolev spaces

(1.1)

\|D^{r}\varphi\|_{L^{p}(\mathbb{R}^{d})}\leq C(r,S,p,d)\,\|\varphi\|_{L^{2}(\mathbb{R}^{d})}^{1-\theta}\,\|D^{S}\varphi\|_{L^{2}(\mathbb{R}^{d})}^{\theta},\,,

where $\varphi\in H^{S}(\mathbb{R}^{d})$ and $D^{s}\varphi$ is defined by

(\widehat{D^{s}\varphi})(\xi)=|\xi|^{s}\widehat{\varphi}(\xi).

The inequality (1.1) holds, see [5], Corollary 1.5 in [11], [6] or Theorem 2.44 in [1] provided that

•

$\frac{1}{p}=\frac{1}{2}+\frac{r-\theta S}{d},$
•

$\frac{r}{S}\leq\theta\leq 1$ ,
•

$0<r\leq S$ , $p>1$ .

We notice that at the endpoint case $p=2$ , corresponding to $\theta=\frac{r}{S}$ , we have

(1.2)

\|D^{r}\varphi\|_{L^{2}(\mathbb{R}^{d})}\leq C(r,S,2,d)\|\varphi\|_{L^{2}(\mathbb{R}^{d})}^{1-\frac{r}{S}}\,\|D^{S}\varphi\|_{L^{2}(\mathbb{R}^{d})}^{\frac{r}{S}},\qquad\forall\varphi\in H^{S}(\mathbb{R}^{d}),

and hence the embedding $H^{S}\subset\dot{H}^{r}$ for $0\leq r\leq S$ is just a consequence of (1.2). If we look at the endpoint cases $\theta=\frac{r}{S}$ and $\theta=1$ in (1.1) we obtain that the range of exponents $p$ without any symmetry and positivity assumption fulfills

(1.3)			$\displaystyle p\in[2,\frac{2d}{d-2(S-r)}]$	$\displaystyle\text{if }S-r<\frac{d}{2},$
(1.3)			$\displaystyle p\in[2,\infty)$	$\displaystyle\text{if }S-r\geq\frac{d}{2}.$

We remark that the lower endpoint does not depend on dimension $d$ .

Moreover, looking at (1.2), it is easy to prove that the best constant in (1.2) is $C(r,S,2,d)=1$ . Indeed from Hölder’s inequality in frequency applied to l.h.s. of (1.2) we get $C(r,S,2,d)\leq 1$ and calling $A_{n}=\left\{\xi\in\mathbb{R}^{d}\text{ s.t. }1-\frac{1}{n}<|\xi|<1+\frac{1}{n}\right\}$ it suffices to consider a sequence $\varphi_{n}$ such that $\hat{\varphi}_{n}(\xi)=\mathbbm{1}_{A_{n}}(\xi)$ to prove that $C(r,S,2,d)=1$ .

In the sequel we consider $r,S,d$ as fixed quantities and we aim to study the range of $p$ such that (1.1) holds in case we restrict to radially symmetric functions $\varphi$ in $H^{S}(\mathbb{R}^{d})$ such that $D^{r}\varphi$ is not only radially symmetric but also either positive or negative.

We introduce the notation for $0<r<s$

(1.4)

\dot{H}^{s}_{rad}(\mathbb{R}^{d}):=\{\varphi\in\dot{H}^{s}(\mathbb{R}^{d}),\ \ \varphi=\varphi(|x|)\},

(1.5)

H^{s}_{rad}(\mathbb{R}^{d}):=\{\varphi\in H^{s}(\mathbb{R}^{d}),\ \ \varphi=\varphi(|x|)\},

(1.6)

H^{s,r}_{rad,+}(\mathbb{R}^{d}):=\{\varphi\in H^{s}_{rad}(\mathbb{R}^{d}),\ \ \ D^{r}\varphi\geq 0\},

(1.7)

H^{s,r}_{rad,-}(\mathbb{R}^{d}):=\{\varphi\in H^{s}_{rad}(\mathbb{R}^{d}),\ \ \ D^{r}\varphi\leq 0\}.

By the relation $(\widehat{-\Delta\varphi})(\xi)=4\pi^{2}|\xi|^{2}\widehat{\varphi}(\xi)=4\pi^{2}(\widehat{D^{2}\varphi})(\xi)$ we shall emphasize that $H^{s,2}_{rad,+}(\mathbb{R}^{d})$ corresponds to the set of superharmonic radially symmetric functions belonging to $H^{s}(\mathbb{R}^{d})$ while $H^{s,2}_{rad,-}(\mathbb{R}^{d})$ corresponds to the set of subharmonic radially symmetric functions belonging to $H^{s}(\mathbb{R}^{d})$ . In the sequel we will call when $r\neq 2$ fractional superharmonic radially symmetric functions belonging to $H^{s}(\mathbb{R}^{d})$ the functions belonging to $H^{s,r}_{rad,+}(\mathbb{R}^{d})$ and fractional subharmonic radially symmetric functions belonging to $H^{s}(\mathbb{R}^{d})$ the functions belonging to $H^{s,r}_{rad,-}(\mathbb{R}^{d})$ .

The main questions we are interesting in are the following ones:

Question A: Can we find appropriate values of $(r,S)$ such that $p$ can be chosen below $2$ in (1.1) for fractional superharmonic (resp. subharmonic) functions belonging to $H^{S,r}_{rad,+}(\mathbb{R}^{d})$ ?

Question B: If the answer of question A is positive, then can we expect a compact embedding of type

(1.8)

H^{S,r}_{rad,+}(\mathbb{R}^{d})\subset\subset\dot{H}^{r}(\mathbb{R}^{d})?

In the sequel we will consider the case $\varphi\in H^{S,r}_{rad,+}(\mathbb{R}^{d})$ but all the results are still valid if we consider $\varphi\in H^{S,r}_{rad,-}(\mathbb{R}^{d})$ . The first result of the paper gives a positive answer to Question A.

Theorem 1.1.

Let $d\geq 2$ and $\frac{1}{2}<r<\min(\frac{d}{2},S-\frac{1}{2})$ , then

(1.9)			$\displaystyle\\|D^{r}\varphi\\|_{L^{p}(\mathbb{R}^{d})}\leq C_{rad,+}(r,S,p,d)\,\\|\varphi\\|_{L^{2}(\mathbb{R}^{d})}^{1-\theta}\,\\|D^{S}\varphi\\|_{L^{2}(\mathbb{R}^{d})}^{\theta},$
(1.9)			$\displaystyle\forall\varphi\ \in H^{S,r}_{rad,+}(\mathbb{R}^{d})\,,$

with

(1.10)		$\displaystyle p\in(p_{0},\frac{2d}{d-2(S-r)}]$	$\displaystyle\text{if }S-r<\frac{d}{2},$
(1.11)		$\displaystyle p\in(p_{0},\infty)$	$\displaystyle\text{if }S-r\geq\frac{d}{2},$

with $\theta$ fixed by the scaling equation

\frac{1}{p}=\frac{1}{2}+\frac{r-\theta S}{d},

and $p_{0}<2$ is given by

p_{0}=\frac{d-2r+2(S-r)(d-1)}{-((S-r)-\frac{1}{2})(d-2r)+2(S-r)(d-1)}.

Remark 1.1.

Theorem 1.1 holds also for $\varphi\ \in H^{S,r}_{rad,-}(\mathbb{R}^{d})$ . The crucial condition is that $D^{r}\varphi$ does not change sign.

The constant $C_{rad,+}(r,S,p,d)$ in (1.9) is defined as best constant in case of functions belonging to $H^{S,r}_{rad,+}(\mathbb{R}^{d})$ .

The fact that $p_{0}<2$ in the above Theorem implies $D^{r}\varphi\in L^{p}$ with $p\in(p_{0},2)$ and this allows us to obtain also a positive answer to Question B.

Theorem 1.2.

Let $d\geq 2$ and $\frac{1}{2}<r_{0}<\min(\frac{d}{2},S-\frac{1}{2})$ , then the embedding

H^{S,r_{0}}_{rad,+}(\mathbb{R}^{d})\subset\subset\dot{H}^{r}_{rad}(\mathbb{R}^{d})

is compact for any $0<r<S.$

Remark 1.2.

Theorem 1.2 holds also in $H^{S,r_{0}}_{rad,-}(\mathbb{R}^{d})$ . Clearly the main difficult in Theorem 1.2 is to prove that the embedding $H^{S,r_{0}}_{rad,+}(\mathbb{R}^{d})\subset\subset\dot{H}^{r_{0}}_{rad}(\mathbb{R}^{d})$ is compact, the compactness for $r\neq r_{0}$ will follow by interpolation.

As a second byproduct we have also the following result concerning the existence of maximizers for the interpolation inequality (1.9) in case $p=2$ .

Theorem 1.3.

Let $d\geq 2$ and $\frac{1}{2}<r<\min(\frac{d}{2},S-\frac{1}{2})$ then

\|D^{r}\varphi\|_{L^{2}(\mathbb{R}^{d})}\leq C_{rad,+}(r,S,2,d)\|\varphi\|_{L^{2}(\mathbb{R}^{d})}^{1-\frac{r}{S}}\,\|D^{S}\varphi\|_{L^{2}(\mathbb{R}^{d})}^{\frac{r}{S}},

\forall\varphi\ \in H^{S,r}_{rad,+}(\mathbb{R}^{d}),

and the best constant $C_{rad,+}(r,S,2,d)$ is attained and $C_{rad,+}(r,S,2,d)<1.$

The strategy to prove Theorem 1.1 and as a byproduct, the compactness result given in Theorem 1.2, it to rewrite (1.1) involving $L^{2}$ norms of Riesz potentials when $0<r<d$ . By defining $u=D^{r}\varphi$ we obtain

(1.12)

\|u\|_{L^{p}(\mathbb{R}^{d})}\leq C(\alpha,s,p,d)\,\|\frac{1}{|x|^{\alpha}}\star u\|_{L^{2}(\mathbb{R}^{d})}^{1-\theta}\,\|D^{s}u\|_{L^{2}(\mathbb{R}^{d})}^{\theta}

where $\alpha=d-r$ , $s=S-r$ . With respect to the new variables $\alpha,s$ we get without any symmetry or positivity assumption

(1.13)			$\displaystyle p\in[2,\frac{2d}{d-2s}]$		$\displaystyle\text{if }s<\frac{d}{2},$
(1.13)			$\displaystyle p\in[2,\infty)$		$\displaystyle\text{if }s\geq\frac{d}{2}.$

If one considers functions fulfilling $D^{r}\varphi=u\geq 0$ , inequality (1.12) is hence equivalent to the following inequality

(1.14)

\|u\|_{L^{p}(\mathbb{R}^{d})}\leq C(\alpha,s,p,d)\,\|\frac{1}{|x|^{\alpha}}\star|u|\|_{L^{2}(\mathbb{R}^{d})}^{1-\theta}\,\|D^{s}u\|_{L^{2}(\mathbb{R}^{d})}^{\theta}

considering $|u|$ instead of $u$ in the Riesz potential. The strategy is hence to prove that the radial symmetry increases the range of $p$ for which (1.14) holds and therefore as byproduct the range of $p$ for which (1.12) holds when $D^{r}\varphi=u$ is positive and radially symmetric (resp. negative). In particular we will show that the lower endpoint is allowed to be below $p=2$ . A reasonable idea to prove that the lower endpoint exponent in (1.14) decreases with radial symmetry is to look at a suitable pointwise decay in the spirit of the Strauss lemma [17] (see also [15, 16] for Besov and Lizorkin-Triebel classes). In our context where two terms are present, the Sobolev norm and the Riesz potential involving $|u|$ , we have been inspired by [13] where the case $s=1$ in (1.14) has been studied (see also [4] and [3]). For our purposes the fact that $s$ is in general not integer makes however the strategy completetly different from the one in [13] and we need to estimate the decay of the high/low frequency part of the function to compute the decay. To this aim we compute the high frequency part using the explicit formula for the Fourier transform for radially symmetric function involving Bessel functions, in the spirit of [7], while we use a weighted $L^{1}$ norm to compute the decay for the low frequency part. The importance of a pointwise decay for the low frequency part involving weighted $L^{p}$ norms goes back to [8] and we need to adapt it to our case in order to involve the Riesz potential. Here is the step where positivity is crucial. Indeed if one is interested to show a scaling invariant weighted inequality as

(1.15)

\int_{\mathbb{R}^{d}}\frac{|u(x)|}{|x|^{\gamma}}dx\leq C\|\frac{1}{|x|^{\alpha}}\star|u|\|_{L^{2}(\mathbb{R}^{d})}

a scaling argument forces the exponent $\gamma$ to verify the relation $\gamma=\alpha-\frac{d}{2}$ . Unfortunately (1.15) cannot hold in the whole Euclidean space following a general argument that goes back to [13] and [14]. However a scaling invariant inequality like (1.15) restricted on balls and on complementary of balls is enough for our purposes. Eventually, using all these tools, we are able to compute a pointwise decay that allows the lower endpoint for (1.14) to be below the threshold $p=2$ . Computed the pointwise decay we will follow the argument in [4] to estimate the lower endpoint for fractional superharmonic (resp. subharmonic) radially symmetric functions.

Concerning the compactness we prove that taking a bounded sequence $\varphi_{n}\in H^{S,r}_{rad,+}$ then $\varphi_{n}\to\varphi$ $\dot{H}^{r}$ with $r>0$ . Our strategy is to prove the smallness of $\|D^{r}(\varphi_{n}-\varphi)\|_{L^{2}(B_{\rho})}$ and $\|D^{r}(\varphi_{n}-\varphi)\|_{L^{2}(B_{\rho}^{c})}$ for suitable choice of the ball $B_{\rho}.$ For the first term we use Rellich-Kondrachov argument combined with commutator estimates, while for the exterior domain we use the crucial fact that $D^{r}(\varphi_{n}-\varphi)$ is in $L^{p}(|x|>\rho)$ for some $p\in(1,2).$

Looking at the case $r=0$ , by Rellich-Kondrachov we have $\|\varphi_{n}-\varphi\|_{L^{2}(B_{\rho})}=o(1)$ , however we can not obtain the smallness in the complementary $B_{\rho}^{c}$ of the ball so the requirement $r>0$ seems to be optimal.

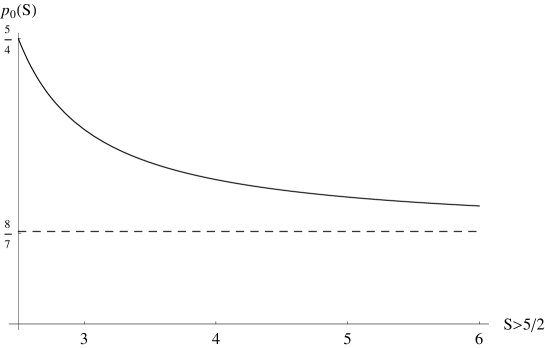
It is interesting to look at the lower endpoint exponent $p_{0}$ given in Theorem 1.1 in case we consider radially symmetric superharmonic (or subharmonic), namely when $r=2$ . In this case the condition $\frac{1}{2}<r<\min(\frac{d}{2},S-\frac{1}{2})$ , imposes to consider the case $d\geq 5$ and $S>\frac{5}{2}$ . As an example we show on Figure 1 the graph of the function $p_{0}(S)$ , that now is only a function of $S$ , in lowest dimensional case $d=5$ that is a branch of hyperbola with asymptote $p_{\infty}=\lim_{S\rightarrow\infty}p_{0}(S)=8/7.$ It is interesting how the regularity improves the lower endpoint $p_{0}(S)$ .

As a final comment we notice that for $d\geq 2$ if $D^{2}\varphi\geq 0$ then $D^{\frac{3}{4}}\varphi=D^{-{\frac{5}{4}}}\left(D^{2}\ \varphi\right)\geq 0$ then, taking $r_{0}=3/4$ and using the positivity of the Riesz kernel of $D^{-{\frac{5}{4}}},$ we apply Theorem 1.2 and we get the following corollary.

Corollary 1.1.

Let $\varphi_{n}$ be a sequence of radially symmetric superharmonic functions uniformly bounded in $H^{2}(\mathbb{R}^{d})$ , $d\geq 2$ . Then for any $0<r<2$ , up to subsequence $\varphi_{n}\to\varphi$ in $\dot{H}^{r}(\mathbb{R}^{d})$ .

Refer to caption — Figure 1. The graph of the function $p_{0}(S)=(16S-30)/(14S-27)$ in the case of superharmonic or subharmonic functions. Here $r=2,d=5$ and $S>5/2.$

2. Interpolation inequalities for radial functions involving Riesz potentials.

Let $d\geq 2$ , $0<\alpha<d$ , $\frac{1}{2}<s,$ we define

X=X_{s,\alpha,d}=\left\{u\in\dot{H}^{s}_{rad}(\mathbb{R}^{d}),\ \ \left\|\frac{1}{|x|^{\alpha}}\star|u|\right\|_{L^{2}}<+\infty\ \right\}.

The aim of this section is to prove the following

Theorem 2.1.

Let $u\in X$ with $d\geq 2$ , $s>\frac{1}{2}$ , $\frac{d}{2}<\alpha<d-\frac{1}{2}$ , then $u\in L^{p}(\mathbb{R}^{d})$ with

	$\displaystyle p\in(p_{rad},\frac{2d}{d-2s}]$	$\displaystyle\text{if }s<\frac{d}{2},$
	$\displaystyle p\in(p_{rad},\infty)$	$\displaystyle\text{if }s\geq\frac{d}{2}.$

where $p_{rad}<2$ with

p_{rad}=\frac{2(\alpha-\frac{d}{2})+2s(d-1)}{-(2s-1)(\alpha-\frac{d}{2})+2s(d-1)}.

Moreover, we have the scaling invariant inequality for $u\in X$

\|u\|_{L^{p}(\mathbb{R}^{d})}\leq C(\alpha,s,p,d)\,\|\frac{1}{|x|^{\alpha}}\star|u|\|_{L^{2}(\mathbb{R}^{d})}^{1-\theta}\,\|D^{s}u\|_{L^{2}(\mathbb{R}^{d})}^{\theta},

with $p\in(p_{rad},\frac{2d}{d-2s}]$ if $s<\frac{d}{2}$ and $p\in(p_{rad},\infty)$ if $s\geq\frac{d}{2}$ . Here $\theta$ is fixed by the scaling invariance

\frac{d}{p}=(1-\theta)((d-\alpha)+\frac{d}{2})+\theta(-s+\frac{d}{2}).

In order to show Theorem 2.1 we need to prove some preliminary results.

Proposition 2.1.

Let $d\geq 1$ , $q>1$ , $\frac{d}{q}<\alpha<d$ , $\delta>0$ , then there exists $C>0$ such that

(2.1)

\int_{B_{R}(0)^{c}}\frac{|u(x)|}{|x|^{\alpha-\frac{d}{q}+\delta}}dx\leq\frac{C}{R^{\delta}}||\frac{1}{|x|^{\alpha}}\star|u|||_{L^{q}(\mathbb{R}^{d})}

(2.2)

\int_{B_{R}(0)}\frac{|u(x)|}{|x|^{\alpha-\frac{d}{q}-\delta}}dx\leq CR^{\delta}||\frac{1}{|x|^{\alpha}}\star|u|||_{L^{q}(\mathbb{R}^{d})}.

The proposition for $q=2$ has been proved in [13], we follow the same argument for $q>1$ . In order to prove Proposition 2.1 two crucial lemmas are necessary. The case $q=2$ has been proved in [13] and we follow the same argument.

Lemma 2.1.

Let $d\geq 1$ , $q\geq 1$ , $0<\alpha<d$ , then there exists $C>0$ such that for any $a\in\mathbb{R}^{d}$

\int_{0}^{\infty}\left(\fint_{B_{\rho}(a)}|u(y)|dy\right)^{q}\rho^{(d-\alpha)q+d-1}d\rho\leq C||\frac{1}{|x|^{\alpha}}\star|u|||_{L^{q}(\mathbb{R}^{d})}^{q}.

Proof.

Let us take $x\in\mathcal{A_{\rho}}=B_{\rho}(a)\setminus B_{\frac{\rho}{2}}(a)$ , then

\frac{1}{|x|^{\alpha}}\star|u|(x)=\int_{\mathbb{R}^{d}}\frac{|u(y)|}{|x-y|^{\alpha}}dy\geq

\geq\int_{B_{\rho}(a)}\frac{|u(y)|}{|x-y|^{\alpha}}dy\geq C\rho^{d-\alpha}\fint_{B_{\rho}(a)}|u(y)|dy.

Thus we obtain for $x\in\mathcal{A_{\rho}}$

\left(\frac{1}{|x|^{\alpha}}\star|u|(x)\right)^{q}\geq C\rho^{(d-\alpha)q}\left(\fint_{B_{\rho}(a)}|u(y)|dy\right)^{q}

and hence

\int_{\mathcal{A_{\rho}}}\left(\frac{1}{|x|^{\alpha}}\star|u|(x)\right)^{q}dx\geq C\rho^{(d-\alpha)q+d}\left(\fint_{B_{\rho}(a)}|u(y)|dy\right)^{q}.

By integration we conclude that

\int_{0}^{\infty}\rho^{(d-\alpha)q+d-1}\left(\fint_{B_{\rho}(a)}|u(y)|dy\right)^{q}d\rho\leq

\leq C\int_{0}^{\infty}\left(\int_{\mathcal{A_{\rho}}}\left(\frac{1}{|x|^{\alpha}}\star|u|(x)\right)^{q}dx\right)\frac{d\rho}{\rho}=C||\frac{1}{|x|^{\alpha}}\star|u|||_{L^{q}(\mathbb{R}^{d})}^{q}.

∎

Let us call $W(\rho)=\int_{\rho}^{\infty}w(s)ds$ where $w:(0,\infty)\rightarrow\mathbb{R}$ is a measurable function such that

(2.3)

\int_{0}^{\infty}|w(\rho)|^{\frac{q}{q-1}}\rho^{\frac{\alpha q+1-d}{q-1}}d\rho<+\infty.

Lemma 2.2.

Let $d\geq 1$ , $q>1$ , $0<\alpha<d$ , then

|\int_{\mathbb{R}^{d}}|u(x)|W(|x|)dx|\lesssim

\left(\int_{0}^{\infty}|w(\rho)|^{\frac{q}{q-1}}\rho^{\frac{\alpha q+1-d}{q-1}}d\rho\right)^{\frac{q-1}{q}}\left(\int_{0}^{\infty}\left(\fint_{B_{\rho}(a)}|u(y)|dy\right)^{q}\rho^{\alpha q+d-1}d\rho\right)^{\frac{1}{q}},

and hence

(2.4)

|\int_{\mathbb{R}^{d}}|u(x)|W(|x|)dx|\leq C||\frac{1}{|x|^{\alpha}}\star|u|||_{L^{q}(\mathbb{R}^{d})}.

Proof.

We have, thanks to Fubini Theorem,

\int_{\mathbb{R}^{d}}|u(x)|W(|x|)dx=\int_{\mathbb{R}^{d}}|u(x)|\left(\int_{|x|}^{\infty}w(\rho)d\rho\right)dx=

=C\int_{0}^{\infty}w(\rho)\rho^{d}\left(\fint_{B_{\rho}(0)}|u(y)|dy\right)d\rho

such that by Hölder’s inequality we obtain

|\int_{\mathbb{R}^{d}}|u(x)|W(|x|)dx|=C|\int_{0}^{\infty}w(\rho)\rho^{d-\beta}\left(\fint_{B_{\rho}(0)}|u(y)|dy\right)\rho^{\beta}d\rho|\lesssim

\left(\int_{0}^{\infty}|w(\rho)|^{\frac{q}{q-1}}\rho^{\frac{\alpha q+1-d}{q-1}}d\rho\right)^{\frac{q-1}{q}}\left(\int_{0}^{\infty}\left(\fint_{B_{\rho}(0)}|u(y)|dy\right)^{q}\rho^{\alpha q+d-1}d\rho\right)^{\frac{1}{q}},

choosing $\beta$ such that $\beta q=(d-\alpha)q+d-1.$ Eq. (2.4) comes from Lemma 2.1. ∎

Proof of Proposition 2.1.

If we choose

w(\rho)=\left\{\begin{array}[]{ll}0,&\hbox{if $0<\rho<R$;}\\ \frac{1}{\rho^{\alpha-\frac{d}{q}+1+\delta}},&\hbox{if $\rho>R$.}\end{array}\right.

thanks to Lemma 2.2 we get (2.1). In order to get (2.2) it is enough to choose

w(\rho)=\left\{\begin{array}[]{ll}0,&\hbox{if $\rho>R$;}\\ \frac{1}{\rho^{\alpha-\frac{d}{q}+1-\delta}},&\hbox{if $0<\rho<R$.}\end{array}\right.

∎

Lemma 2.3.

Let $d\geq 1$ , $\frac{d}{2}<\alpha<d$ and $||D^{s}u||_{L^{2}(\mathbb{R}^{d})}=||\frac{1}{|x|^{\alpha}}\star|u|||_{L^{2}(\mathbb{R}^{d})}=1$ , then for any $\delta>0$ such that $0<\delta<d-\alpha$ ,

\int_{\mathbb{R}^{d}}\frac{|u(x)|}{|x|^{\alpha-\frac{d}{2}+\delta}}dx\leq C(\alpha,s,\delta,d).

Proof.

Let $0<\epsilon<\frac{d}{2}$ be a number to be fixed later. We have

\int_{B(0,1)}\frac{|u(x)|}{|x|^{\alpha-\frac{d}{2}+\delta}}dx=\int_{B(0,1)}\frac{|u(x)|}{|x|^{\alpha-\frac{d}{2}+\delta-\epsilon}}\frac{1}{|x|^{\epsilon}}dx\leq

\leq c_{d,\epsilon}\left(\int_{B(0,1)}\frac{|u(x)|^{2}}{|x|^{2(\alpha-\frac{d}{2}+\delta-\epsilon)}}\right)^{\frac{1}{2}},

where $c_{d,\epsilon}=\left(\int_{B(0,1)}\frac{1}{|x|^{2\epsilon}}dx\right)^{\frac{1}{2}}$ . Now choose $\epsilon=\alpha-\frac{d}{2}+\delta$ . Notice that $\epsilon<\frac{d}{2}$ such that

\int_{B(0,1)}\frac{|u(x)|}{|x|^{\alpha-\frac{d}{2}+\delta}}dx\leq c_{d,\epsilon}\left(\int_{B(0,1)}|u(x)|^{2}dx\right)^{\frac{1}{2}}.

which implies

\int_{B(0,1)}\frac{|u(x)|}{|x|^{\alpha-\frac{d}{2}+\delta}}dx\lesssim 1.

On the other hand by Proposition 2.1, when $\frac{d}{2}<\alpha<d$

\int_{B(0,1)^{c}}\frac{|u(x)|}{|x|^{\alpha-\frac{d}{2}+\delta}}dx\leq C||\frac{1}{|x|^{\alpha}}\star|u|||_{L^{2}(\mathbb{R}^{d})}

and hence we obtain the claim.∎

The next Proposition concerning pointwise decay for radial functions in $X$ follows the strategy of Theorem 3.1 in [8]. We will decompose the function in high/low frequency part, estimating the high frequency part involving the Sobolev norm while we control the low frequency part involving the Riesz norm.

Proposition 2.2.

Let $d\geq 2,$ $u$ be a radial function in $X$ with $s>\frac{1}{2}$ , $\frac{d}{2}<\alpha<d$ , and

(2.5)

||D^{s}u||_{L^{2}(\mathbb{R}^{d})}=||\frac{1}{|x|^{\alpha}}\star|u|||_{L^{2}(\mathbb{R}^{d})}=1.

Then for any $\sigma$ satisfying

(2.6)

\frac{2s\left(\frac{d}{2}-1\right)+\left(\frac{d}{2}\right)}{2s+1}<\sigma<\frac{2s(d-1)-(2s-1)\left(\alpha-\frac{d}{2}\right)}{2s+1}

we have

|u(x)|\leq C(\alpha,s,\sigma,d)|x|^{-\sigma}.

Remark 2.1.

It is easy to see that the above Proposition is equivalent to the following statement.

Let $u$ be a radial function in $X$ with $s>\frac{1}{2}$ , $\frac{d}{2}<\alpha<d$ , and

(2.7)

||D^{s}u||_{L^{2}(\mathbb{R}^{d})}=||\frac{1}{|x|^{\alpha}}\star|u|||_{L^{2}(\mathbb{R}^{d})}=1

then for any $\delta>0$ such that $0<\delta<d-\alpha$ ,

|u(x)|\leq C(\alpha,s,\delta,d)|x|^{-\sigma}

with

(2.8)

\sigma=\frac{-(2s-1)(\alpha-\frac{d}{2}+\delta)+2s(d-1)}{2s+1}.

Proof.

For any $R>1$ we can take a function $\psi_{R}(x)=R^{-d}\psi(x/R)$ such that $\widehat{\psi}(\xi)$ is a radial nonnegative function with support in $|\xi|\leq 2$ and $\widehat{\psi}(\xi)=1$ for $|\xi|\leq 1$ and then we make the decomposition of $u$ into low and high frequency part as follows

u(x)=\psi_{R}\star u(x)+h(x)

where $\hat{h}(\xi)=(1-\hat{\psi}(R|\xi|))\hat{u}(\xi)$ . For the high frequency part we will use Fourier representation for radial functions in $\mathbb{R}^{d}$ (identifying the function with its profile)

(2.9)

|h(x)|=(2\pi)^{\frac{d}{2}}|x|^{-\frac{d-2}{2}}\int_{0}^{\infty}J_{\frac{d-2}{2}}(|x|\rho)(1-\psi(R\rho))\hat{u}(\rho)\rho^{\frac{d}{2}}d\rho

where $J_{\frac{d-2}{2}}$ is the Bessel function of order $\frac{d-2}{2}.$ Applying the results in [7] and [8], we find

(2.10)

|h(x)|\leq cR^{s-\frac{1}{2}}|x|^{-\frac{1}{2}(d-1)}||u||_{\dot{H}^{s}(\mathbb{R}^{d})},\ s>\frac{1}{2}.

Indeed, using the uniform bound

|J_{\frac{d-2}{2}}(\rho)|\lesssim(1+\rho)^{-1/2},

we get

|h(x)|\lesssim|x|^{-\frac{d-2}{2}}\int_{0}^{\infty}|(J_{\frac{d-2}{2}})(|x|\rho)||(1-\psi(R\rho))||\hat{u}(\rho)|\rho^{\frac{d}{2}}d\rho\lesssim

|x|^{-\frac{d-2}{2}}\left(\int_{1/R}^{\infty}|J_{\frac{d-2}{2}}(|x|\rho)|^{2}\frac{d\rho}{\rho^{2s-1}}\right)^{1/2}\left(\int_{0}^{\infty}|\hat{u}(\rho)|^{2}\rho^{2s+d-1}d\rho\right)^{1/2}\lesssim

|x|^{-\frac{d-2}{2}}R^{s-1}\left(\int_{1}^{\infty}(1+|x|\rho/R)^{-1}\frac{d\rho}{\rho^{2s-1}}\right)^{1/2}\|u\|_{\dot{H}^{s}(\mathbb{R}^{d})}\lesssim

\lesssim R^{s-1/2}|x|^{-\frac{d-1}{2}}\|u\|_{\dot{H}^{s}(\mathbb{R}^{d})}

and this gives (2.10).

For low frequency term $\psi_{R}\star u(x)$ , since $\psi\in\mathcal{S}\left(\mathbb{R}^{d}\right)$ , we can take any $\gamma>1$ so that there exists $C>0$ such that

|\psi(x)|\leq C\left(1+|x|^{2}\right)^{-\gamma/2}.

We shall need the following estimate that can be found also in [12] and [8]. For sake of completeness we give an alternative proof of the Lemma in the Appendix.

Lemma 2.4.

If $b\in(-d+1,0),\gamma>d-1,$ then for any radially symmetric function $f(|y|)$ we have

(2.11)

\left|\int_{\mathbb{R}^{d}}\frac{f(|y|)dy}{(1+|x-y|^{2})^{\gamma/2}}\right|\lesssim\frac{1}{|x|^{d-1+b}}\left\||y|^{b}f\right\|_{L^{1}(\mathbb{R}^{d})}.

Then we estimate $\psi_{R}\star u(x)$ as follows,

	$\displaystyle\|\psi_{R}\star u(x)\|$	$\displaystyle\leq\left\|\psi_{R}(x)\right\|*\|u(x)\|\leq C\int_{\mathbb{R}^{d}}\frac{1}{R^{d}}\frac{\|u(y)\|}{\left(1+\left\|\frac{x-y}{R}\right\|\right)^{\gamma/2}}dy$
		$\displaystyle\leq C\int_{\mathbb{R}^{d}}\frac{\|u(Rz)\|}{\left(1+\left\|\frac{x}{R}-z\right\|^{2}dz\right)^{\gamma/2}}dz\quad(y=Rz).$

To this end we plan to apply Lemma 2.4 assuming $b=-(\alpha-d/2+\delta)$ . To check the assumption of the Lemma we use the inequalities

\alpha-\frac{d}{2}+\delta<\frac{d}{2}\leq d-1

for $d\geq 2.$ Applying the Lemma 2.4 we deduce

		$\displaystyle\|\psi_{R}\star u(x)\|$
		$\displaystyle\leq C\left\|\frac{x}{R}\right\|^{-(d-1+b)}\int_{\mathbb{R}^{d}}\|u(Rz)\|\|z\|^{b}dz$
		$\displaystyle\leq CR^{(d-1+b)}\|x\|^{-(d-1+b)}\int_{\mathbb{R}^{d}}\|u(y)\|\left\|\frac{y}{R}\right\|^{b}\frac{dy}{R^{d}}$
		$\displaystyle\leq CR^{-1}\|x\|^{-(d-1+b)}\\|\|y\|^{b}u\\|_{L^{1}(\mathbb{R}^{d})}.$

Therefore, collecting our estimates and using the condition (2.5), we find

\displaystyle|u(x)|

\displaystyle\leq C\left[|x|^{-(d-1)/2}R^{s-1/2}+|x|^{-(d-1+b)}R^{-1}\||y|^{b}u\|_{L^{1}(\mathbb{R}^{d})}\right].

We use Lemma 2.3 and we get

\displaystyle|u(x)|

\displaystyle\leq C\left[|x|^{-(d-1)/2}R^{s-1/2}+|x|^{-(d-1+b)}R^{-1}\right].

Minimizing in $R$ or equivalently choosing $R>0$ so that

|x|^{-(d-1)/2}R^{s-1/2}=|x|^{-(d-1+b)}R^{-1},

i.e.

R^{s+1/2}=|x|^{-b-(d-1)/2},

we find

|u(x)|\leq C(d,s,\alpha,\delta)|x|^{-\sigma}.

where $\sigma$ is defined in (2.8).

This completes the proof.

∎

With all these preliminary results we are now ready to prove Theorem 2.1.

Proof.

Let $u\in X$ with $||D^{s}u||_{L^{2}(\mathbb{R}^{d})}=||\frac{1}{|x|^{\alpha}}\star|u|||_{L^{2}(\mathbb{R}^{d})}=1$ , then by Proposition 2.2

|u(x)|\leq C(d,s,\alpha,\delta)|x|^{-\sigma}

with

\sigma=\frac{-(2s-1)(\alpha-\frac{d}{2}+\delta)+2s(d-1)}{2s+1}.

We aim to show that $p_{rad}<2$ , where $p=2$ is the lower endpoint for (1.12). Therefore it sufficies to show that $\int_{|x|>1}|u|^{p}dx<+\infty$ provided that $u\in X$ and $p_{rad}<p$ (indeed $\int_{|x|\leq 1}|u|^{p}dx<+\infty$ for all $0<p<2$ by interpolation).
We have, thanks to Proposition 2.2 and Lemma 2.3,

(2.12)

\int_{|x|>1}|u||u|^{p-1}dx\lesssim\int_{|x|>1}\frac{|u|}{|x|^{\sigma(p-1)}}dx\lesssim 1

provided that $\sigma(p-1)>\alpha-\frac{d}{2}$ . This condition is equivalent, $\sigma$ is defined in (2.8) and letting $\delta\rightarrow 0$ , to

p>\frac{\sigma+\alpha-\frac{d}{2}}{\sigma}=\frac{2(\alpha-\frac{d}{2})+2s(d-1)}{-(2s-1)(\alpha-\frac{d}{2})+2s(d-1)}:=p_{rad}.

An elementary computation shows that $p_{rad}<2$ provided that $\frac{d}{2}<\alpha<{d-\frac{1}{2}}$ .

Now consider an arbitrary $v\in X$ and let us call $u=\lambda v(\mu x)$ where the parameters $\lambda,\mu>0$ are chosen such that $||D^{s}u||_{L^{2}(\mathbb{R}^{d})}=||\frac{1}{|x|^{\alpha}}\star|u|||_{L^{2}(\mathbb{R}^{d})}=1$ . By scaling we have

1=||D^{s}u||_{L^{2}(\mathbb{R}^{d})}=\lambda\mu^{s-\frac{d}{2}}||D^{s}v||_{L^{2}(\mathbb{R}^{d})}

1=||\frac{1}{|x|^{\alpha}}\star|u|||_{L^{2}(\mathbb{R}^{d})}=\lambda\mu^{\alpha-\frac{3}{2}d}||\frac{1}{|x|^{\alpha}}\star|v|||_{L^{2}(\mathbb{R}^{d})}

and hence we obtain the relations

\mu=\left(\frac{||D^{s}v||_{L^{2}(\mathbb{R}^{d})}}{||\frac{1}{|x|^{\alpha}}\star|v|||_{L^{2}(\mathbb{R}^{d})}}\right)^{\frac{1}{\alpha-s-d}},\ \ \lambda=\frac{||\frac{1}{|x|^{\alpha}}\star|v|||_{L^{2}(\mathbb{R}^{d})}^{\frac{s-\frac{d}{2}}{\alpha-d-s}}}{||D^{s}v||_{L^{2}(\mathbb{R}^{d})}^{\frac{\alpha-\frac{3}{2}d}{\alpha-d-s}}}.

By the previous estimates we have

||u||_{L^{p}(\mathbb{R}^{d})}=\lambda\mu^{-\frac{d}{p}}||v||_{L^{p}(\mathbb{R}^{d})}\lesssim 1

which implies

||v||_{L^{p}(\mathbb{R}^{d})}\lesssim\lambda^{-1}\mu^{\frac{d}{p}}=||D^{s}v||_{L^{2}(\mathbb{R}^{d})}^{\theta}\|\frac{1}{|x|^{\alpha}}\star|v|\|_{L^{2}(\mathbb{R}^{d})}^{1-\theta},

where

\theta=\frac{d^{2}-2\alpha p+3dp-2ds}{2p(d+s-\alpha)},\ \ 1-\theta=\frac{(2s-d)(d+p)}{2p(d+s-\alpha)}.

It is easy to see that $\theta$ is fixed by the scaling invariance

\frac{d}{p}=(1-\theta)((d-\alpha)+\frac{d}{2})+\theta(-s+\frac{d}{2}).

∎

3. Proof of Theorem 1.1

Our goal is to represent $\varphi$ in the form $\varphi=\frac{1}{|x|^{\alpha}}\star u=cD^{-r}u,$ with $\alpha=d-r,$ $c=\frac{\pi^{d/2}\Gamma((d-\alpha)/2)}{\Gamma(\alpha/2)}$ and apply Theorem 2.1. Therefore, we choose (modulo constant) $u=D^{r}\varphi.$

Then the estimate of Theorem 2.1 gives

\|D^{r}\varphi\|_{L^{p}(\mathbb{R}^{d})}=\|u\|_{L^{p}(\mathbb{R}^{d})}\lesssim\,\|\frac{1}{|x|^{\alpha}}\star|u|\|_{L^{2}(\mathbb{R}^{d})}^{1-\theta}\,\|D^{s}u\|_{L^{2}(\mathbb{R}^{d})}^{\theta}=

=\left\|D^{-r}|D^{r}\varphi|\right\|_{L^{2}(\mathbb{R}^{d})}^{1-\theta}\|D^{S}\varphi|\|_{L^{2}(\mathbb{R}^{d})}^{\theta}.

By the assumption

(3.1)

D^{r}\varphi(x)\geq 0

for almost every $x\in\mathbb{R}^{d},$ then we deduce

\left\|D^{-r}|D^{r}\varphi|\right\|_{L^{2}(\mathbb{R}^{d})}^{1-\theta}\|D^{S}\varphi|\|_{L^{2}(\mathbb{R}^{d})}^{\theta}=\left\|D^{-r}D^{r}\varphi\right\|_{L^{2}(\mathbb{R}^{d})}^{1-\theta}\|D^{S}\varphi|\|_{L^{2}(\mathbb{R}^{d})}^{\theta}

and we obtain (1.9). The lower endpoint $p_{0}$ is hence nothing but $p_{rad}$ of Theorem 2.1 substituting $\alpha$ with $d-r$ and $s$ with $S-r$ . The condition $\frac{1}{2}<r<\min(\frac{d}{2},S-\frac{1}{2})$ is equivalent to the conditions $\frac{d}{2}<\alpha<d-\frac{1}{2}$ , $s>\frac{1}{2}$ of Theorem 2.1. All these estimates remain valid if we consider $D^{r}\varphi(x)\leq 0$ , i.e if $\varphi\in H^{s,r}_{rad,-}(\mathbb{R}^{d})$ . Indeed if $\varphi\in H^{s,r}_{rad,-}(\mathbb{R}^{d})$

\left\|D^{-r}|D^{r}\varphi|\right\|_{L^{2}(\mathbb{R}^{d})}^{1-\theta}\|D^{S}\varphi|\|_{L^{2}(\mathbb{R}^{d})}^{\theta}=

=\left\|-D^{-r}D^{r}\varphi\right\|_{L^{2}(\mathbb{R}^{d})}^{1-\theta}\|D^{S}\varphi|\|_{L^{2}(\mathbb{R}^{d})}^{\theta}=\left\|\varphi\right\|_{L^{2}(\mathbb{R}^{d})}^{1-\theta}\|D^{S}\varphi|\|_{L^{2}(\mathbb{R}^{d})}^{\theta}.

4. Proof of Theorem 1.2

We prove that under the assumption of Theorem 1.2, the embedding

H^{S,r_{0}}_{rad,+}(\mathbb{R}^{d})\subset\subset\dot{H}^{r_{0}}_{rad}(\mathbb{R}^{d})

is compact. As a byproduct the embedding

(4.1)

H^{S,r_{0}}_{rad,+}(\mathbb{R}^{d})\subset\subset\dot{H}^{r}_{rad}(\mathbb{R}^{d})

is compact for any $0<r<S$ . The embedding (4.1) follows noticing that if $\varphi_{n}$ converges weakly to some $\varphi$ in $H^{S}_{rad}(\mathbb{R}^{d})$ then $\varphi_{n}$ converges weakly to the same $\varphi$ in $H^{r_{0}}_{rad}(\mathbb{R}^{d})$ . Now if we prove that (taking a subsequence)

(4.2)

\|D^{r_{0}}(\varphi_{n}-\varphi)\|_{L^{2}}=o(1)

as $n\to\infty,$ then by the following interpolation inequalities

\|D^{r}(\varphi_{n}-\varphi)\|_{L^{2}}\lesssim\|D^{r_{0}}(\varphi_{n}-\varphi)\|_{L^{2}}^{1-\frac{r-r_{0}}{S-r_{0}}}\,\|D^{S}(\varphi_{n}-\varphi)\|_{L^{2}}^{\frac{r-r_{0}}{S-r_{0}}}=o(1)

if $0<r_{0}<r<S$ and

\|D^{r}(\varphi_{n}-\varphi)\|_{L^{2}}\lesssim\|(\varphi_{n}-\varphi)\|_{L^{2}}^{1-\frac{r}{r_{0}}}\,\|D^{r_{0}}(\varphi_{n}-\varphi)\|_{L^{2}}^{\frac{r}{r_{0}}}=o(1)

if $0<r<r_{0}$ , we get (4.1).

To prove (4.2) we recall that $(\varphi_{n})_{n\in\mathbb{N}}$ is a bounded sequence in $H^{S,r_{0}}_{rad,+}(\mathbb{R}^{d})$ and we can assume that $\varphi_{n}$ converges weakly to some $\varphi$ in $H^{S}(\mathbb{R}^{d})$ . To simplify the notation we will use $r$ instead of $r_{0}$ in the proof of (4.2). We choose a bump function $\theta\in C_{0}^{\infty}(\mathbb{R}^{d})$ , such that $\theta=1$ on $B_{1}$ and $\theta=0$ in $\mathbb{R}^{d}\setminus B_{2}$ and for any $\rho>1$ we define $\theta_{\rho}(x)=\theta(x/\rho).$ Clearly the multiplication by $\theta_{\rho}\in\mathcal{S}(\mathbb{R}^{d})$ is a continuous mapping $H^{S}(\mathbb{R}^{d})\rightarrow H^{S}(\mathbb{R}^{d})$ . Now setting $v_{n}=\theta_{\rho}\varphi_{n}$ and $v=\theta_{\rho}\varphi$ we aim to show that

(4.3)

\lim_{n\to\infty}\|D^{r}(v_{n}-v)\|_{L^{2}(\mathbb{R}^{d})}^{2}=\lim_{n\to\infty}\|D^{r}(\theta_{\rho}(\varphi_{n}-\varphi))\|_{L^{2}(\mathbb{R}^{d})}^{2}=0.

for any $r\in[0,S).$

Indeed, by Plancharel’s identity we have

\|D^{r}(v_{n}-v)\|_{L^{2}(\mathbb{R}^{d})}^{2}=\underbrace{\int_{|\xi|\leq R}|\xi|^{2r}|\widehat{v}_{n}(\xi)-\widehat{v}(\xi)|^{2}d\xi}_{=I}+\underbrace{\int_{|\xi|>R}|\xi|^{2r}|\widehat{v}_{n}(\xi)-\widehat{v}(\xi)|^{2}d\xi}_{=II}.

Clearly

II\leq\frac{1}{R^{2(S-r)}}\int_{|\xi|>R}|\xi|^{2S}|\widehat{v}_{n}(\xi)-\widehat{v}(\xi)|^{2}d\xi

and then we can choose $R>0$ such that $II\leq\frac{\epsilon}{2}$ .
Since $e^{-2\pi ix\cdot\xi}\in L^{2}_{x}(B_{2\rho})$ , by weak convergence in $L^{2}(B_{2\rho})$ we have $\widehat{v}_{n}(\xi)\rightarrow\widehat{v}(\xi)$ almost everywhere. Notice that $||\widehat{v}_{n}||_{L^{\infty}}\leq||v_{n}||_{L^{1}(B_{2\rho})}\leq\mu(B_{2\rho})^{\frac{1}{2}}||v_{n}||_{L^{2}(B_{2\rho})}\leq\mu(B_{2\rho})^{\frac{1}{2}}||v_{n}||_{H^{S}(\mathbb{R}^{d})}$ and hence $|\widehat{v}_{n}(\xi)-\widehat{v}(\xi)|^{2}$ is estimated by a uniform constant so that by Lebesgue’s dominated convergence theorem

I=\int_{|\xi|\leq R}|\xi|^{2r}|\widehat{v}_{n}(\xi)-\widehat{v}(\xi)|^{2}d\xi<\frac{\epsilon}{2},

for $n$ sufficiently large. This proves (4.3).

Our next step is to show that for a given $\varepsilon>0$ one can find $\rho_{0}=\rho_{0}(\varepsilon)$ sufficiently large and $n_{0}(\varepsilon)$ sufficiently large so that

(4.4)

\|\theta_{\rho}D^{r}(\varphi_{n}-\varphi)\|_{L^{2}(\mathbb{R}^{d})}^{2}\leq\frac{\varepsilon}{2}

for $n\geq n_{0},\rho\geq\rho_{0}$ and any $r\in[0,S).$

We consider first the case $0\leq r\leq 2,r<S.$ The cases $r=0$ and $r=2$ are trivial, for this we assume $0<r<\min(2,S).$ We shall use the following statement (see Corollary 1.1 in [10]).

Proposition 4.1.

Let $p,p_{1},p_{2}$ satisfy $1<p,p_{1},p_{2}<\infty$ and $1/p=1/p_{1}+1/p_{2}$ . Let $r,r_{1},r_{2}$ satisfy $0\leq r_{1},r_{2}\leq 1$ , and $r=r_{1}+r_{2}$ . Then the following bilinear estimate

\|\underbrace{D^{r}(fg)-fD^{r}g}_{=[D^{r},f]g}-gD^{r}f\|_{L^{p}}\leq C\|D^{r_{1}}f\|_{L^{p_{1}}}\|D^{r_{2}}g\|_{L^{p_{2}}}

holds for all $f,g\in\mathcal{S}$ .

By a density argument the statement holds for $f,g\in H^{S}(\mathbb{R}^{d}).$ We choose $f=\theta_{\rho},$ $g=\varphi_{n}-\varphi$ and $r_{1}=r_{2}=r/2$ and therefore we aim to use (4.3) and prove that

(4.5)			$\displaystyle\\|[\theta_{\rho},D^{r}](\varphi_{n}-\varphi)\\|_{L^{2}(\mathbb{R}^{d})}\leq$
(4.5)			$\displaystyle\leq O(\rho^{-r})\\|\varphi_{n}-\varphi\\|_{L^{2}(\mathbb{R}^{d})}+O(\rho^{-r/4})\\|\varphi_{n}-\varphi\\|_{H^{r}(\mathbb{R}^{d})}.$

Indeed from the Proposition 4.1 we have

\|[\theta_{\rho},D^{r}](\varphi_{n}-\varphi)\|_{L^{2}(\mathbb{R}^{d})}\lesssim\underbrace{\|D^{r}\theta_{\rho}\|_{L^{\infty}(\mathbb{R}^{d})}\|\varphi_{n}-\varphi\|_{L^{2}(\mathbb{R}^{d})}}_{=O(\rho^{-r})}+

+\|D^{r/2}\theta_{\rho}\|_{L^{p_{1}}(\mathbb{R}^{d})}\|D^{r/2}(\varphi_{n}-\varphi))\|_{L^{p_{2}}(\mathbb{R}^{d})}.

It is easy to check the estimate

\|D^{r/2}\theta_{\rho}\|_{L^{p_{1}}(\mathbb{R}^{d})}=O(\rho^{-r/4}),

as $\rho\to\infty,$ and this is obviously fulfilled if $\frac{d}{p_{1}}<\frac{r}{4}$ . To control $\|D^{r/2}(\varphi_{n}-\varphi))\|_{L^{p_{2}}(\mathbb{R}^{d})}$ we use Sobolev inequality

\|D^{r/2}(\varphi_{n}-\varphi))\|_{L^{p_{2}}(\mathbb{R}^{d})}\lesssim\|\varphi_{n}-\varphi\|_{H^{r}(\mathbb{R}^{d})}

so we need

\frac{1}{p_{2}}>\frac{1}{2}-\frac{r-r/2}{d}.

Summing up we have the following restrictions for $1/p_{1},1/p_{2}$

(4.6)			$\displaystyle\frac{1}{p_{1}}+\frac{1}{p_{2}}=\frac{1}{2}$
(4.6)			$\displaystyle\frac{1}{p_{1}}<\frac{r}{4d},\ \frac{1}{p_{2}}>\frac{1}{2}-\frac{r-r/2}{d}.$

Choosing $p_{2}=2+\kappa,p_{1}=2(2+\kappa)/\kappa$ with $\kappa>0$ sufficiently small we see that (4.6) is nonempty. Now notice that

(4.7)

\|\theta_{\rho}D^{r}(\varphi_{n}-\varphi)\|_{L^{2}(\mathbb{R}^{d})}\leq\|D^{r}(\theta_{\rho}(\varphi_{n}-\varphi)\|_{L^{2}(\mathbb{R}^{d})}+\|[\theta_{\rho},D^{r}](\varphi_{n}-\varphi)\|_{L^{2}(\mathbb{R}^{d})}

and we conclude that (4.4) is true for $0\leq r<\min(2,S)$ thanks to (4.3) and (4.5).

Now we consider the case $2\leq r<S.$ We have $D^{r}=D^{r_{1}}(-\Delta)^{\ell},$ where $\ell\geq 1$ is integer and $0<r_{1}<2.$ Then the commutator relation

[A,BC]=[A,B]C+B[A,C]

implies

[\theta_{\rho},D^{r}]=[\theta_{\rho},D^{r_{1}}](-\Delta)^{\ell}+D^{r_{1}}[\theta_{\rho},(-\Delta)^{\ell}].

In fact, we have the relation

\theta_{\rho}D^{r}(\varphi_{n}-\varphi)=[\theta_{\rho},D^{r_{1}}]((-\Delta)^{\ell}(\varphi_{n}-\varphi))+D^{r_{1}}[\theta_{\rho},(-\Delta)^{\ell}](\varphi_{n}-\varphi)

and we use (4.5) so that

\|[\theta_{\rho},D^{r_{1}}](-\Delta)^{\ell}(\varphi_{n}-\varphi)\|_{L^{2}(\mathbb{R}^{d})}\leq

\leq O(\rho^{-r_{1}})\|(-\Delta)^{\ell}(\varphi_{n}-\varphi)\|_{L^{2}(\mathbb{R}^{d})}+

+O(\rho^{-r_{1}/4})\|D^{r_{1}+2\ell}(\varphi_{n}-\varphi)\|_{L^{2}(\mathbb{R}^{d})}=o(1)

for $\rho\to\infty.$

The term

D^{r_{1}}[\theta_{\rho},(-\Delta)^{\ell}](\varphi_{n}-\varphi)

can be evaluated pointwise via the classical Leibnitz rule and then via the fractional Leibnitz rule as follows

\|D^{r_{1}}[\theta_{\rho},(-\Delta)^{\ell}](\varphi_{n}-\varphi)\|_{L^{2}(\mathbb{R}^{d})}\lesssim

\lesssim\sum_{1\leq|\alpha|,|\alpha|+|\beta|=2\ell}\|D^{r_{1}}(\partial_{x}^{\alpha}\theta_{\rho})\partial_{x}^{\beta}(\varphi_{n}-\varphi)\|_{L^{2}(\mathbb{R}^{d})}\lesssim O(\rho^{-1})\|\varphi_{n}-\varphi\|_{H^{r}(\mathbb{R}^{d})}.

Summing up, we conclude that (4.4) holds in case $r\in[0,S).$

To conclude that the embedding is compact it remains to show that also $\|D^{r}(\varphi_{n}-\varphi)\|_{L^{2}(B_{\rho}^{c})}^{2}\leq\epsilon$ . To this purpose we first use the pointwise decay in terms of homogeneous Sobolev norm, see [7]. Given $r$ there exists $0<\delta<\frac{d-1}{2}$ with $r+\frac{1}{2}+\delta<S$ such that

(4.8)

|D^{r}(\varphi_{n}-\varphi)(x)|\leq\frac{C}{|x|^{\gamma}}||\varphi_{n}-\varphi||_{\dot{H}^{r+\frac{1}{2}+\delta}(\mathbb{R}^{d})}\lesssim\frac{C}{|x|^{\gamma}}

with $\gamma=\frac{d-1}{2}-\delta$ . Secondly we use that $p_{0}<2$ , i.e. that $p=2$ is non endpoint. By Theorem 1.1 there exists $\delta_{0}>0$ sufficiently small such that $D^{r}\varphi_{n}$ is uniformly bounded in $L^{2-\delta_{0}}(\mathbb{R}^{d})$ and the same holds hence for $D^{r}\varphi$ and $D^{r}(\varphi_{n}-\varphi)$ . As a consequence we have

\|D^{r}(\varphi_{n}-\varphi)\|_{L^{2}(B_{\rho}^{c})}^{2}=\int_{B_{\rho}^{c}}|D^{r}(\varphi_{n}-\varphi)|^{\delta_{0}}|D^{r}(\varphi_{n}-\varphi)|^{2-\delta_{0}}dx\leq

\leq\frac{C}{\rho^{\gamma}}^{\delta_{0}}\|D^{r}(\varphi_{n}-\varphi)\|_{L^{2-\delta_{0}}(B_{\rho}^{c})}^{2-\delta}

with

\|D^{r}(\varphi_{n}-\varphi)\|_{L^{2-\delta_{0}}(B_{\rho}^{c})}\leq\|D^{r}(\varphi_{n}-\varphi)\|_{L^{2-\delta_{0}}(\mathbb{R}^{d})}=O(1).

This proves that $\|D^{r}(\varphi_{n}-\varphi)\|_{L^{2}(B_{\rho})}^{2}\lesssim\epsilon$ and hence that the embedding is compact.

5. Proof of Theorem 1.3

For easier reference we state the following.

Lemma 5.1 (pqr Lemma [9]).

Let $1\leq p<q<r\leq\infty$ and let $\alpha,\beta,\gamma>0$ . Then there are constants $\eta,c>0$ such that for any measurable function $f\in L^{p}(X)\cap L^{r}(X)$ , $X$ a measure space, with

\|f\|_{L^{p}}^{p}\leq\alpha,\quad\|f\|_{L^{q}}^{q}\geq\beta,\quad\|f\|_{L^{r}}^{r}\leq\gamma,\quad

one has (with $|\cdot|$ denoting the underlying measure on $X$ )

(5.1)

\left|\{x\in X:\ |f(x)|>\eta\}\right|\geq c\,.

Lemma 5.2 (Compactness up to translations in $\dot{H}^{s}$ [2]).

Let $s>0$ , $1<p<\infty$ and $u_{n}\in\dot{H}^{s}(\mathbb{R}^{d})\cap L^{p}(\mathbb{R}^{d})$ be a sequence with

(5.2)

\sup_{n}\left(\|u_{n}\|_{\dot{H}^{s}(\mathbb{R}^{d})}+\|u_{n}\|_{L^{p}(\mathbb{R}^{d})}\right)<\infty

and, for some $\eta>0$ , (with $|\cdot|$ denoting Lebesgue measure)

(5.3)

\inf_{n}\left|\{|u_{n}|>\eta\}\right|>0\,.

Then there is a sequence $(x_{n})\subset\mathbb{R}^{d}$ such that a subsequence of $u_{n}(\cdot+x_{n})$ has a weak limit $u\not\equiv 0$ in $\dot{H}^{s}(\mathbb{R}^{d})\cap L^{p}(\mathbb{R}^{d})$ .

The strategy to prove Theorem 1.3 follows the one developed in [2]. First we aim to show that the maximum of

W(\varphi)=\frac{\|D^{r}\varphi\|_{L^{2}(\mathbb{R}^{d})}}{\|\varphi\|_{L^{2}(\mathbb{R}^{d})}^{1-\frac{r}{S}}\,\|D^{S}\varphi\|_{L^{2}(\mathbb{R}^{d})}^{\frac{r}{S}}}\ \ \ \varphi\in H^{S,r}_{rad,+}(\mathbb{R}^{d}),

is achieved in $H^{S,r}_{rad,+}(\mathbb{R}^{d})$ . Let us consider a maximizing sequence $\varphi_{n}$ . Since $W$ is invariant under homogeneity $\varphi(x)\mapsto\lambda\varphi(x)$ and scaling $\varphi\mapsto\varphi(\lambda x)$ for any $\lambda>0$ , we can choose a maximizing sequence $\varphi_{n}$ such that

(5.4)

\|D^{r}\varphi_{n}\|_{L^{2}(\mathbb{R}^{d})}=C_{rad,+}(r,S,2,d)+o(1)

and

(5.5)

\|\varphi_{n}\|_{L^{2}(\mathbb{R}^{d})}=\|D^{S}\varphi_{n}\|_{L^{2}(\mathbb{R}^{d})}=1\,.

The key observation is that, since we are looking at a non-endpoint case (i.e. $p_{0}<2$ ), there exists $\epsilon>0$ such that from inequality (1.9) we infer that

(5.6)

\sup_{n}\max\left\{\|D^{r}\varphi_{n}\|_{L^{2-\epsilon}(\mathbb{R}^{d})},\|D^{r}\varphi_{n}\|_{L^{2+\epsilon}(\mathbb{R}^{d})}\right\}<\infty\,.

The $pqr$ -lemma (Lemma 5.1) now implies that

(5.7)

\inf_{n}\left|\{|D^{r}\varphi_{n}|>\eta\}\right|>0.

Next, we apply the compactness modulo translations lemma (Lemma 5.2) to the sequence $(D^{r}\varphi_{n})$ . This sequence is bounded in $\dot{H}^{S-r}$ by (5.5), and (5.2) and (5.3) are satisfied by (5.4) and (5.7). Thus possibly after passing to a subsequence, we have $D^{r}\varphi_{n}\rightharpoonup\psi\not\equiv 0$ in $H^{S-r}(\mathbb{R}^{d})$ . By the fact the embedding is compact we deduce that $\varphi_{n}(x)\rightarrow\psi\not\equiv 0$ in $\dot{H}^{r}(\mathbb{R}^{d})$ and hence $\psi$ is a maximizer for $W$ .
Now we conclude showing that $C_{rad,+}(r,S,2,d)<1$ .
Indeed if the best constant is $C_{rad,+}(r,S,2,d)=1$ , the maximizer $\psi$ achieves the equality in Hölder’s inequality, which means

(5.8)			$\displaystyle\int_{\mathbb{R}^{d}}\|\xi\|^{2r}\|\widehat{\psi}\|^{2}d\xi=\int_{\mathbb{R}^{d}}\|\widehat{\psi}\|^{2-\frac{2r}{S}}\|\xi\|^{2r}\|\widehat{\psi}\|^{\frac{2r}{S}}d\xi=$
(5.8)			$\displaystyle=\left(\int_{\mathbb{R}^{d}}\|\widehat{\psi}\|^{2}d\xi\right)^{1-\frac{r}{S}}\left(\int_{\mathbb{R}^{d}}\|\xi\|^{2S}\|\widehat{\psi}\|^{2}d\xi\right)^{\frac{r}{S}},$

where we used as conjugated exponents $\frac{S}{S-r}$ and $\frac{S}{r}$ . Now we recall that if $f\in L^{p}(\mathbb{R}^{d})$ and $g\in L^{q}(\mathbb{R}^{d})$ with $p$ and $q$ conjugated exponents achieve the equality in Hölder’s inequality then $|f|^{p}$ and $|g|^{q}$ shall be linearly dependent, i.e. for a suitable $\mu,|f|^{p}=\mu|g|^{q}$ almost everywhere. Therefore, calling $f=|\widehat{\psi}|^{2-\frac{2r}{S}}$ and $g=|\xi|^{2r}|\widehat{\psi}|^{\frac{2r}{S}}$ , the maximizer $\psi$ should satisfy $|\widehat{\psi}|^{2}=\mu|\xi|^{2S}|\widehat{\psi}|^{2}$ for a suitable $\mu$ which drives to the contradiction $\widehat{\psi}=0.$

6. Appendix.

The statement of Lemma 2.4 can be found in [12]. Somehow, due to the fact that in the original paper the proof of Lemma 2.4 is not easy readable, being a part of a more general statement, we give an alternative short proof.

Proof of Lemma 2.4.

We divide the integration domain in two subdomains:

\Omega=\{|x|<|y|/2\}\cup\{|x|>2|y|\}

and its complementary set $\Omega^{c}.$ In $\Omega$ we use

|x-y|\geq\frac{\max(|x|,|y|)}{2}

and via

(1+|x-y|^{2})^{(d-1)/2}\gtrsim(1+(\max(|x|,|y|))^{2})^{(d-1)/2}\geq

\geq\max(|x|,|y|))^{(d-1)}\gtrsim|x|^{(d-1+b)}|y|^{-b}

with $d-1+b>0,-b>0$ we deduce

\frac{1}{(1+|x-y|^{2})^{\gamma/2}}=\frac{1}{(1+|x-y|^{2})^{(d-1)/2}}\frac{1}{(1+|x-y|^{2})^{(\gamma-d+1)/2}}

\lesssim\frac{1}{|x|^{d-1+b}}|y|^{b}\frac{1}{(1+|x-y|^{2})^{(\gamma-d+1)/2}}\leq\frac{1}{|x|^{d-1+b}}|y|^{b}.

These estimates imply

(6.1)

\left|\int_{\Omega}\frac{f(y)dy}{(1+|x-y|^{2})^{\gamma/2}}\right|\lesssim\frac{1}{|x|^{d-1+b}}\left\||y|^{b}f\right\|_{L^{1}(\mathbb{R}^{d})}.

For the complementary domain $\Omega^{c}$ we use spherical coordinates $x=r\theta,y=\rho\omega,$ where $r=|x|,\rho=|y|.$ We have to estimate

\int_{\Omega^{c}}\frac{f(y)dy}{(1+|x-y|^{2})^{\gamma/2}}=\int_{r/2}^{2r}K(r,\rho)f(\rho)\rho^{d-1}d\rho,

where

(6.2)

K(r,\rho)=K_{\theta,\gamma}(r,\rho)=\int_{\mathbb{S}^{d-1}}(1+|r\theta-\rho\omega|^{2})^{-\gamma/2}d\omega.

To get the desired estimate

(6.3)

\left|\int_{\Omega^{c}}\frac{f(y)dy}{(1+|x-y|^{2})^{\gamma/2}}\right|\lesssim\frac{1}{|x|^{d-1+b}}\left\||y|^{b}f\right\|_{L^{1}(\mathbb{R}^{d})}

it is sufficient to check the pointwise estimate

(6.4)

K(r,\rho)\lesssim r^{-(d-1+b)}\rho^{b}\sim r^{-(d-1)}\ \ \mbox{for $r/2\leq\rho\leq 2r$.}

To deduce this pointwise estimate of the kernel $K$ we note first that $K$ does not depend on $\theta$ so we can take $\theta=e_{d}=(0,\cdots,0,1)$ and $\omega=(\omega^{\prime}\sin\varphi,\cos\varphi),$ $\omega^{\prime}\in\mathbb{S}^{d-2}$ and get

K(r,\rho)=c\int_{0}^{\pi}\frac{\sin^{d-2}\varphi d\varphi}{(1+r^{2}+\rho^{2}-2r\rho\cos\varphi)^{\gamma/2}}.

Using the relation

(1+r^{2}+\rho^{2}-2r\rho\cos\varphi)=1+(r-\rho)^{2}+r\rho\sin^{2}(\varphi/2),

we can use the

(1+r^{2}+\rho^{2}-2r\rho\cos\varphi)\gtrsim r\rho\sim r^{2}

when $\rho\sim r$ and $\varphi$ is not close to $0,$ say $\varphi\in(\pi/4,\pi).$ Then we get

\int_{\pi/4}^{\pi}\frac{\sin^{d-2}\varphi d\varphi}{(1+r^{2}+\rho^{2}-2r\rho\cos\varphi)^{\gamma/2}}\lesssim\int_{\pi/4}^{\pi}r^{-\gamma}d\varphi\lesssim r^{-\gamma}\leq r^{-d+1}.

For $\varphi$ close to $0$ , say $\varphi\leq\pi/4$ we use

\frac{\sin^{d-2}\varphi}{(1+r^{2}+\rho^{2}-2r\rho\cos\varphi)^{\gamma/2}}\lesssim\frac{\varphi^{d-2}}{(1+r\rho\varphi^{2})^{\gamma/2}}.

In this way, making the change of variable $r\varphi=\eta$ we get

\int_{0}^{\pi/4}\frac{\varphi^{d-2}d\varphi}{(1+r\rho\varphi^{2})^{\gamma/2}}\lesssim\int_{0}^{\infty}\frac{\varphi^{d-2}d\varphi}{(1+r^{2}\varphi^{2})^{\gamma/2}}\leq

\leq r^{-d+1}\int_{0}^{\infty}\frac{\eta^{d-2}d\eta}{(1+\eta^{2})^{\gamma/2}}\lesssim r^{-d+1}

in view of $\rho\sim r$ and $\gamma>d-1.$ Taking together the above estimates of the integrals over $(0,\pi/4)$ and $(\pi/4,\pi)$ , we arrive at (6.4).

This completes the proof of the Lemma.

∎

References

[1] H. Bahouri, J.-Y. Chemin, R. Danchin, Fourier Analysis and Nonlinear Partial Differential Equations, Springer, 2011.
[2] J.Bellazzini, R.L. Frank, N. Visciglia, Maximizers for Gagliardo-Nirenberg inequalities and related non-local problems, Math. Annalen (2014), no. 3-4, 653 – 673.
[3] J. Bellazzini, M. Ghimenti, C. Mercuri, V. Moroz, J. Van Schaftingen, Sharp Gagliardo-Nirenberg inequalities in fractional Coulomb-Sobolev spaces, Trans. Amer. Math. Soc. 370, 11, 8285 – 8310 (2018)
[4] J. Bellazzini, M. Ghimenti, and T. Ozawa, Sharp lower bounds for Coulomb energy, Math. Res. Lett. 23 (2016), no. 3, 621–632
[5] H. Brezis, P. Mironescu, Gagliardo-Nirenberg, composition and products in fractional Sobolev spaces. Dedicated to the memory of Tosio Kato. J. Evol. Equ. 1 (2001), no. 4, 387 – 404
[6] H. Brezis and P. Mironescu, Where Sobolev interacts with Gagliardo-Nirenberg, J. Funct. Anal. 277 (2019), no. 8, 2839 – 2864.
[7] Y. Cho, T. Ozawa, Sobolev inequality with symmetry, Commun. Contemp. Math. 11 (2009), no. 3, 355–365
[8] P.L. De Nápoli Symmetry breaking for an elliptic equation involving the Fractional Laplacian, Differential Integral Equations 31 (1/2) 75 - 94, January/February (2018).
[9] J. Fröhlich, E. H. Lieb, M. Loss, Stability of Coulomb systems with magnetic fields. I. The one-electron atom., Comm. Math. Phys. 104 (1986), no. 2, 251–270.
[10] K. Fujiwara, V.Georgiev, T. Ozawa, Higher order fractional Leibniz rule. J. Fourier Anal. Appl. 24 (2018), no. 3, 650 – 665.
[11] H. Hajaiej,L. Molinet, T. Ozawa, and B. Wang, Necessary and sufficient conditions for the fractional Gagliardo-Nirenberg inequalities and applications to Navier-Stokes and generalized boson equations, Harmonic analysis and nonlinear partial differential equations, 159 – 175, RIMS Kôkyûroku Bessatsu, B26, Res. Inst. Math. Sci. (RIMS), Kyoto, 2011
[12] P. D’Ancona, R. Luc $\grave{a}$ , Stein-Weiss and Caffarelli-Kohn-Nirenberg inequalities with angular integrability, J. Math. Anal. Appl., 388(2): 1061–1079, (2012)
[13] C. Mercuri, V. Moroz, J. Van Schaftingen Groundstates and radial solutions to nonlinear Schrödinger-Poisson-Slater equations at the critical frequency, Calc. Var. 55, 146 (2016)
[14] D. Ruiz, On the Schrödinger-Poisson-Slater system: behavior of minimizers, radial and nonradial cases, Arch. Ration. Mech. Anal. 198 (2010), no. 1, 349–368
[15] W. Sickel, L. Skrzypczak, Radial subspaces of Besov and Lizorkin-Triebel classes: extended Strauss lemma and compactness of embeddings, J. Fourier Anal. Appl. 6 (2000), no. 6, 639 – 662.
[16] W. Sickel, L. Skrzypczak, On the Interplay of Regularity and Decay in Case of Radial Functions II. Homogeneous Spaces, J Fourier Anal Appl (2012) 18:548 – 582 DOI 10.1007/s00041-011-9205-2
[17] W. A. Strauss. Existence of Solitary Waves in Higher Dimensions. Comm. Math. Phys. 55 (1977), 149–162.

		$\displaystyle\|\psi_{R}\star u(x)\|$
		$\displaystyle\leq C\left\|\frac{x}{R}\right\|^{-(d-1+b)}\int_{\mathbb{R}^{d}}\|u(Rz)\|\|z\|^{b}dz$
		$\displaystyle\leq CR^{(d-1+b)}\|x\|^{-(d-1+b)}\int_{\mathbb{R}^{d}}\|u(y)\|\left\|\frac{y}{R}\right\|^{b}\frac{dy}{R^{d}}$
		$\displaystyle\leq CR^{-1}\|x\|^{-(d-1+b)}\\|\|y\|^{b}u\\|_{L^{1}(\mathbb{R}^{d})}.$

Compact embeddings for fractional super and sub harmonic functions with radial symmetry

Abstract.

Key words and phrases:

2010 Mathematics Subject Classification:

1. Introduction

Theorem 1.1.

Remark 1.1.

Theorem 1.2.

Remark 1.2.

Theorem 1.3.

Corollary 1.1.

2. Interpolation inequalities for radial functions involving Riesz potentials.

Theorem 2.1.

Proposition 2.1.

Lemma 2.1.

Proof.

Lemma 2.2.

Proof.

Proof of Proposition 2.1.

Lemma 2.3.

Proof.

Proposition 2.2.

Remark 2.1.

Proof.

Lemma 2.4.

Proof.

3. Proof of Theorem 1.1

4. Proof of Theorem 1.2

Proposition 4.1.

5. Proof of Theorem 1.3

Lemma 5.1 (pqr Lemma [9]).

Lemma 5.2 (Compactness up to translations in H˙s\dot{H}^{s} [2]).

6. Appendix.

Proof of Lemma 2.4.

References

Lemma 5.2 (Compactness up to translations in $\dot{H}^{s}$ [2]).