Present address: ]Department of Physics, 104 Davey Lab, The Pennsylvania State University, University Park, Pennsylvania 16802, USA Present address: ]Graduate School of Engineering Science, Akita University, Akita, 010-8502, Japan
Bulk-edge Correspondence in the Adiabatic Heuristic Principle
Abstract
Using the Laughlin’s argument on a torus with two pin-holes, we numerically demonstrate that the discontinuities of the center-of-mass work well as an invariant of the pumping phenomena during the process of the flux-attachment, trading the magnetic flux for the statistical one. This is consistent with the bulk-edge correspondence of the fractional quantum Hall effect of anyons. We also confirm that the general feature of the edge states remains unchanged during the process while the topological degeneracy is discretely changed. This supports the stability of the quantum Hall edge states in the adiabatic heuristic principle.
Introduction
— Characterization of quantum matter with topological invariants is a modern notion in condensed matter physics Thouless et al. (1982); Kohmoto (1985); Hasan and Kane (2010); Qi and Zhang (2011). The adiabatic deformation of gapped systems is a conceptual basis in the theory of topological phases beyond the Landau’s symmetry breaking paradigm Wen (1989, 2017). Meanwhile, augmented by the symmetry, this notion leads to more unified picture exemplified by the “periodic table” for topologically nontrivial states Kitaev (2009); Schnyder et al. (2008); Qi et al. (2008); Ryu et al. (2010) and demonstrates the existence of rich topological phases. The adiabatic deformation also gives a useful way to characterize concrete models by reducing them to simple systems Greiter and Wilczek (1990, 1992, 2021); Hatsugai (2005, 2006, 2007); Kariyado et al. (2018).
The adiabatic heuristic argument of the quantum Hall (QH) effect Greiter and Wilczek (1990, 1992, 2021) is the historical example in which the adiabatic deformation has been successfully used. The fractional QH (FQH) effect Tsui et al. (1982); Laughlin (1983) is a topological ordered phase Wen (1995) with fractionalized excitations Haldane (1983); Halperin (1984); Arovas et al. (1984). Even though it is intrinsically a many-body problem of correlated electrons unlike the integer QH (IQH) effect Klitzing et al. (1980); Laughlin (1981); Thouless et al. (1982); Halperin (1982), the composite fermion theory Jain (1989, 2007) gives a unified scheme to describe their underlying physics: the FQH state at the filling factor with integers can be interpreted as the IQH state of the composite fermions. By continuously trading the external flux for the statistical one Wilczek (1982a, b), both states are adiabatically connected through intermediate systems of anyons (adiabatic heuristic principle Greiter and Wilczek (1990, 1992, 2021)). Even though the ground state degeneracy Haldane (1985); Wen and Niu (1990) is wildly changed in the periodic geometry Greiter and Wilczek (1992); Kudo and Hatsugai (2020), the energy gap remains open and its many-body Chern number Niu et al. (1985) works well as an adiabatic invariant Kudo and Hatsugai (2020).
Generally bulk topological invariants such as the Chern number are intimately related to the presence of gapless edge excitations. This is the so-called bulk-edge correspondence Wen (1990a); Hatsugai (1993a, b), which is a universal feature of topological phases Kane and Mele (2005); Haldane and Raghu (2008); Hasan and Kane (2010); Qi and Zhang (2011); Kariyado and Hatsugai (2015); Delplace et al. (2017); Sone and Ashida (2019); Hatsugai and Fukui (2016); Kuno and Hatsugai (2020); Mizoguchi et al. (2021); Yoshida et al. (2020). The edges of the QH systems demonstrate the nontrivial transport properties enriched by the bulk topology, which has attracted a great interest for over decades Laughlin (1981); Halperin (1982); MacDonald (1990); Wen (1990b, a, 1991); Johnson and MacDonald (1991); Wen (1992); Chamon and Wen (1994); Wen (1995); Meir (1994); Kane et al. (1994); Kane and Fisher (1995); Wan et al. (2002); Joglekar et al. (2003); Wan et al. (2003); Chang (2003); Hu et al. (2008, 2009); Wang et al. (2013a); Repellin et al. (2018); Fern et al. (2018); Wei et al. (2020); Ito and Shibata (2021); Khanna et al. (2021). The main goal in this work is to reveal how the quantum Hall edge states are evolved during the process of the flux-attachment in the adiabatic heuristic principle.
In this Letter, we analyze the fractional pumping phenomena associated with the Laughlin’s argument of the anyonic FQH effect. We show that the general feature of the energy spectrum with edges shows little change during the process of the flux-attachment while the topological degeneracy is wildly changed. Furthermore, the total jump of the center-of-mass works well as an invariant of this process, which is consistent with the bulk-edge correspondence of the FQH effect of anyons. This implies that the total jump of the center-of-mass characterizes the fractional charge pumping of the adiabatic heuristic principle. Also, this supports the stability of the QH edge states in the adiabatic heuristic principle.
Charge pumping
— Let us consider the QH system on a square lattice with sites, where and the periodic boundary condition is imposed. As shown in Fig. 1(a), local fluxes are set at two plaquettes with the same coordinate. Their distance is . Particles are pumped from to as varies from to [see Fig. 1(b)], which we call the (fractional) charge pump Thouless (1983); Wang et al. (2013b); Nakajima et al. (2016); Lohse et al. (2016); Guo et al. (2012); Xu et al. (2013); Zeng et al. (2015); Hu et al. (2016); Zeng et al. (2016); Nakagawa and Furukawa (2017); Taddia et al. (2017); Nakagawa et al. (2018) throughout this Letter.
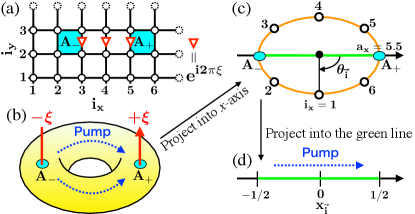
This charge pump can be mapped into the one-dimensional pump with edges [Fig. 1(d)]. As shown in Fig. 1(c), we first project the system into the -axis. Then, projecting it into the green line shown in Figs 1(c), we finally define a new coordinate for site as with , where is the coordinate of , see Fig. 1(d). In this projection, the two pin-holes are mapped into the edges .
The charge can be transformed from to as increases. The pumped charge is given by the integration of , where is the center-of-mass,
| (1) |
is the zero temperature density matrix in the grand canonical ensemble and is the number operator at the site . (In Sec. S1 of Supplemental Material sup , we derive the pumped charge by using the current operator.) As varies, jumps several times due to the sudden change of the particle number Hatsugai and Fukui (2016); Kuno and Hatsugai (2020, 2021). Accordingly, the pumped charge between the period is given by , where are the jumping points in the period and . Using the periodicity and , we get Hatsugai and Fukui (2016)
| (2) |
As shown below, the total jump , i.e., the sudden changes of the particle number, comes from the (dis)appearance of edge states. Equation (2) implies that the pumped charge is given only by the information of edges.
Bulk-edge correspondence
— In this Letter, we numerically show the following bulk-edge correspondence for the FQH states of anyons:
| (3) |
where is the many-body Chern number Niu et al. (1985) of the -fold degenerate ground state multiplet at . This is consistent with the Laughlin’s argument applied to the FQH systems Laughlin (1981); Halperin (1982); Thouless et al. (1982); Thouless (1983); Niu et al. (1985); Hatsugai (1993a); Thouless (1989); Hatsugai and Fukui (2016); Zeng et al. (2016); Grushin et al. (2015); Andrews et al. (2021) that implies . In the following, we clarify how the fractional charge pumping is deformed to the standard pumping phenomena by the flux-attachment transformation. As mentioned below, the relation in Eq. (3) results in the stability of the QH edge states in the adiabatic heuristic principle.
Fermion pumping
— As a first step, we confirm Eq. (3) for the IQH system of non-interacting fermions. The Hamiltonian is , where is the creation operator for a fermion on site and . The phase factors and describe the uniform magnetic field Hofstadter (1976); Hatsugai et al. (1999) and the local fluxes at [see Fig. 1(a)], respectively. We plot in Fig. 2(a) the single-particle energy with and , where is the total uniform fluxes. Each set of states forms the Landau level (LL) at . As increases, some edge states go over to the mid-gap region. In Fig. 2(b), we compute with in Eq. (1), where is the ground state completely occupying the 1st LL under the chemical potential (Fermi energy) . The sudden change of the particle number causes the jumps of at and . Both values of ’s are approximately , which is consistent with Fig. 2(a) where one edge state at goes over across and then another at goes back. Although a finite size effect gives , we confirm in the thermodynamic limit (see Sec. S2 of Supplemental Material sup ). This is consistent with Eq. (3) with and . The cases for and have been also confirmed.
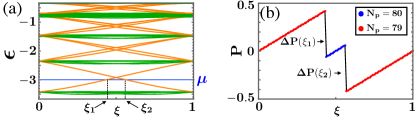
Normalized jumps
— As mentioned above, the jump are not quantized to due to the finite size effect. Let us then properly normalize each jumps: when jumps positively or negatively at , we assign it as or . Hereafter “” denotes this normalization; e.g., we have in Fig. 2(b). This gives the bulk-edge correspondence in Eq. (3) even for finite systems.
Fractional anyon pumping
—
Let us consider the fractional pumping of anyons. To this end, we take the Hamiltonian as , where the phase factor Wen et al. (1990); Hatsugai et al. (1991); fer depends on the configuration of all particles , which describes the fractional statistics . Note that although is the creation operator for a fermion, is the Hamiltonian of anyons and includes intrinsically the many-body interactions. Due to constraints of the braid group, depends on even for the same Wen et al. (1990); Einarsson (1990): the Hilbert space for (: coprime) is spanned by the basis , where is an additional internal degree of freedom. When a particle hops across the boundary in the direction, the label is shifted from to . As for the boundary in the direction, the phase factor is given. Thanks to this, global requirements of anyons hold GR ; Wen et al. (1990); Einarsson (1990); Hatsugai et al. (1991). Also, we introduce only for the basis with def .
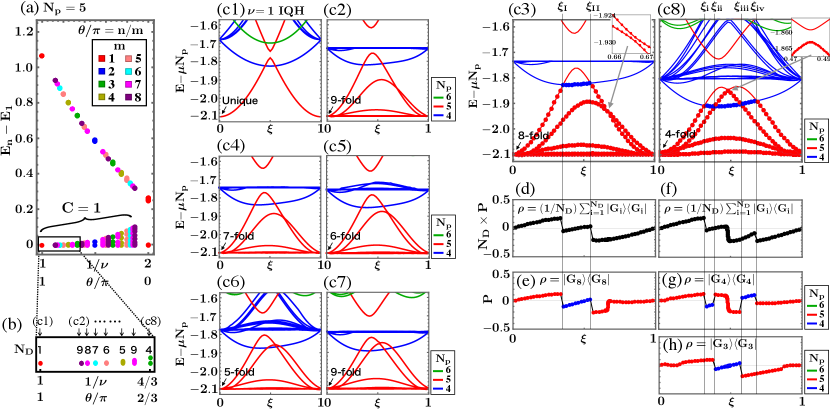
In the following, we focus on a family of the IQH states connected by trading the magnetic fluxes for statistical ones Jain (1989); Greiter and Wilczek (1990, 1992); Kudo and Hatsugai (2020): with . Fixing , and , we plot the energy gaps as functions of in Fig. 3(a). Due to the lattice, the topological degeneracy is lifted. We here define the low-energy states with as the ground state multiplet. The ground state at (: coprime) in Fig. 3(a) gives the degeneracy numerically [see Fig. 3(b)] and the Chern numbers of the multiplets are always Kudo and Hatsugai (2020). Namely, the many-body Chern number is used as an adiabatic invariant. The gap closing at is expected due to finite-size effects Kudo and Hatsugai (2020).
Let us investigate the pumping phenomena. As for each parameter shown in Fig. 3(b), we plot in Figs. 3(c1)-(c8) the eigenvalues of the Hamiltonian including the chemical potential, , as functions of with Np . Figure 3(c1) is in the same setting of Fig. 2 but for smaller system sizes. In Fig 3(c1), the particle number of the unique ground state is changed as increases due to the (dis)appearance of the edge state as mentioned previously. The gap between the two red lines at is a finite-size effect. As shown in Figs. 3(c1)-(c8), even though the topological degeneracy is wildly changed as and vary, the general feature of the spectra remains unchanged. The degenerate ground states at are lifted as increases and then one or two states float up in energy to cross with another state having one particle less.
Now we focus on the anyonic system in Fig. 3(c3) and show its the bulk-edge correspondence. Here , and at . To define the center-of-mass of the ground state multiplet suitably, we define the density matrix as , where is the -th lowest energy state. Using it with Eq. (1), we plot in Fig. 3(d). There are two jumps at and , where the th and th lowest energy states cross each other in the spectrum. Because of , the obtained jumps are solely given by with shown in Fig. 3(e). This figure gives , which implies in Fig. 3(d). This is consistent with Eq. (3) with . Because of , we ahve the fractional pumped charge . In this argument, we assume the absence of the gap closing between states with the same apart from since there are no symmetry except for the charge . The gap at between and is very small but is finite Two as shown in the inset.
Let us here mention the finite size effect in Fig. 3(d). The value of before normalizing is about , which is far away from . Although this value in the IQH system in Fig. 3(c1) is about the same magnitude (about , see the data point at of Fig. 4), it approaches as the system size increases as confirmed in Sec. S2 of Supplemental Material sup . Since the bulk gaps of the two systems are comparable and their system sizes are same, the deviation from in Fig. 3(d) is also expected to be the finite size effect.
Let us next focus on the system in Fig. 3(c8), where , and at . Unlike the previous case, there are four gap-closing points, , as for the lowest energy states. However, with in Fig. 3(f) jumps only at and because the jumps at cancel each other, see Figs. 3(g) and 3(h). Consequently, the total jump is given by , which is consistent with Eq. (3) with . This implies the fractional pumped charge . The gap at is very small but is finite as mentioned before, see the inset in Fig. 3(c8).
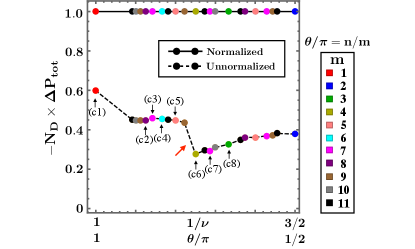
The results shown in Figs. 3(c3) and 3(c8) suggest that is the invariant of the bulk gap in the process of the flux-attachment. To demonstrate it, we plot the total jumps as functions of in Fig. 4, where both data before/after normalizing each jumps are shown. The normalized data justify that works well as the invariant. This nature is also indicated by the unnormalized data in Fig. 4: the plots are smooth as and vary even though (i) the degeneracy is wildly changed and (ii) the dimension of the Hamiltonian is discretely changed depending on the denominator of : e.g., with , for while for (this is due to the additional internal degree of the basis as mentioned above). We stress that this nontrivial smoothness in Fig. 4 implies the stability of the QH edge states in the adiabatic heuristic principle.
Conclusion
— In this Letter, we demonstrate the bulk-edge correspondence of the FQH states of anyons. The results indicate that the total jump of the center-of-mass, which corresponds to the many-body Chern number, is an invariant with respect to the flux-attachment. This implies the stability of edge states in the adiabatic heuristic principle. Recently, direct observation of the center of mass in pumping phenomena has been conducted in cold atoms Nakajima et al. (2016); Lohse et al. (2016). The behavior of the center-of-mass that we focus on would be observed in cold atoms although the experimental realization of the two-dimensional anionic system is still a challenging problem.
Acknowledgements.
We thank the Supercomputer Center, the Institute for Solid State Physics, the University of Tokyo for the use of the facilities. The work is supported in part by JSPS KAKENHI Grant Numbers JP17H06138, JP19J12317 (K.K.), and JP21K13849 (Y.K.).References
- Thouless et al. (1982) D. J. Thouless, M. Kohmoto, M. P. Nightingale, and M. den Nijs, Phys. Rev. Lett. 49, 405 (1982).
- Kohmoto (1985) M. Kohmoto, Annals of Physics 160, 343 (1985).
- Hasan and Kane (2010) M. Z. Hasan and C. L. Kane, Rev. Mod. Phys. 82, 3045 (2010).
- Qi and Zhang (2011) X.-L. Qi and S.-C. Zhang, Rev. Mod. Phys. 83, 1057 (2011).
- Wen (1989) X. G. Wen, Phys. Rev. B 40, 7387 (1989).
- Wen (2017) X.-G. Wen, Rev. Mod. Phys. 89, 041004 (2017).
- Kitaev (2009) A. Kitaev, AIP Conference Proceedings 1134, 22 (2009).
- Schnyder et al. (2008) A. P. Schnyder, S. Ryu, A. Furusaki, and A. W. W. Ludwig, Phys. Rev. B 78, 195125 (2008).
- Qi et al. (2008) X.-L. Qi, T. L. Hughes, and S.-C. Zhang, Phys. Rev. B 78, 195424 (2008).
- Ryu et al. (2010) S. Ryu, A. P. Schnyder, A. Furusaki, and A. W. W. Ludwig, New Journal of Physics 12, 065010 (2010).
- Greiter and Wilczek (1990) M. Greiter and F. Wilczek, Modern Physics Letters B 04, 1063 (1990).
- Greiter and Wilczek (1992) M. Greiter and F. Wilczek, Nuclear Physics B 370, 577 (1992).
- Greiter and Wilczek (2021) M. Greiter and F. Wilczek, (2021), arXiv:2105.05625 [cond-mat.mes-hall] .
- Hatsugai (2005) Y. Hatsugai, Journal of the Physical Society of Japan 74, 1374 (2005).
- Hatsugai (2006) Y. Hatsugai, Journal of the Physical Society of Japan 75, 123601 (2006).
- Hatsugai (2007) Y. Hatsugai, Journal of Physics: Condensed Matter 19, 145209 (2007).
- Kariyado et al. (2018) T. Kariyado, T. Morimoto, and Y. Hatsugai, Phys. Rev. Lett. 120, 247202 (2018).
- Tsui et al. (1982) D. C. Tsui, H. L. Stormer, and A. C. Gossard, Phys. Rev. Lett. 48, 1559 (1982).
- Laughlin (1983) R. B. Laughlin, Phys. Rev. Lett. 50, 1395 (1983).
- Wen (1995) X.-G. Wen, Advances in Physics 44, 405 (1995).
- Haldane (1983) F. D. M. Haldane, Phys. Rev. Lett. 51, 605 (1983).
- Halperin (1984) B. I. Halperin, Phys. Rev. Lett. 52, 1583 (1984).
- Arovas et al. (1984) D. Arovas, J. R. Schrieffer, and F. Wilczek, Phys. Rev. Lett. 53, 722 (1984).
- Klitzing et al. (1980) K. v. Klitzing, G. Dorda, and M. Pepper, Phys. Rev. Lett. 45, 494 (1980).
- Laughlin (1981) R. B. Laughlin, Phys. Rev. B 23, 5632 (1981).
- Halperin (1982) B. I. Halperin, Phys. Rev. B 25, 2185 (1982).
- Jain (1989) J. K. Jain, Phys. Rev. Lett. 63, 199 (1989).
- Jain (2007) J. K. Jain, Composite Fermions (Cambridge University Press, 2007).
- Wilczek (1982a) F. Wilczek, Phys. Rev. Lett. 48, 1144 (1982a).
- Wilczek (1982b) F. Wilczek, Phys. Rev. Lett. 49, 957 (1982b).
- Haldane (1985) F. D. M. Haldane, Phys. Rev. Lett. 55, 2095 (1985).
- Wen and Niu (1990) X. G. Wen and Q. Niu, Phys. Rev. B 41, 9377 (1990).
- Kudo and Hatsugai (2020) K. Kudo and Y. Hatsugai, Phys. Rev. B 102, 125108 (2020).
- Niu et al. (1985) Q. Niu, D. J. Thouless, and Y.-S. Wu, Phys. Rev. B 31, 3372 (1985).
- Wen (1990a) X. G. Wen, Phys. Rev. Lett. 64, 2206 (1990a).
- Hatsugai (1993a) Y. Hatsugai, Phys. Rev. Lett. 71, 3697 (1993a).
- Hatsugai (1993b) Y. Hatsugai, Phys. Rev. B 48, 11851 (1993b).
- Kane and Mele (2005) C. L. Kane and E. J. Mele, Phys. Rev. Lett. 95, 226801 (2005).
- Haldane and Raghu (2008) F. D. M. Haldane and S. Raghu, Phys. Rev. Lett. 100, 013904 (2008).
- Kariyado and Hatsugai (2015) T. Kariyado and Y. Hatsugai, Scientific Reports 5, 18107 (2015).
- Delplace et al. (2017) P. Delplace, J. B. Marston, and A. Venaille, Science 358, 1075 (2017).
- Sone and Ashida (2019) K. Sone and Y. Ashida, Phys. Rev. Lett. 123, 205502 (2019).
- Hatsugai and Fukui (2016) Y. Hatsugai and T. Fukui, Phys. Rev. B 94, 041102 (2016).
- Kuno and Hatsugai (2020) Y. Kuno and Y. Hatsugai, Phys. Rev. Research 2, 042024 (2020).
- Mizoguchi et al. (2021) T. Mizoguchi, T. Yoshida, and Y. Hatsugai, Phys. Rev. B 103, 045136 (2021).
- Yoshida et al. (2020) T. Yoshida, T. Mizoguchi, and Y. Hatsugai, (2020), arXiv:2012.05562 [cond-mat.mes-hall] .
- MacDonald (1990) A. H. MacDonald, Phys. Rev. Lett. 64, 220 (1990).
- Wen (1990b) X. G. Wen, Phys. Rev. B 41, 12838 (1990b).
- Wen (1991) X. G. Wen, Phys. Rev. B 43, 11025 (1991).
- Johnson and MacDonald (1991) M. D. Johnson and A. H. MacDonald, Phys. Rev. Lett. 67, 2060 (1991).
- Wen (1992) X.-G. Wen, International Journal of Modern Physics B 06, 1711 (1992).
- Chamon and Wen (1994) C. d. C. Chamon and X. G. Wen, Phys. Rev. B 49, 8227 (1994).
- Meir (1994) Y. Meir, Phys. Rev. Lett. 72, 2624 (1994).
- Kane et al. (1994) C. L. Kane, M. P. A. Fisher, and J. Polchinski, Phys. Rev. Lett. 72, 4129 (1994).
- Kane and Fisher (1995) C. L. Kane and M. P. A. Fisher, Phys. Rev. B 51, 13449 (1995).
- Wan et al. (2002) X. Wan, K. Yang, and E. H. Rezayi, Phys. Rev. Lett. 88, 056802 (2002).
- Joglekar et al. (2003) Y. N. Joglekar, H. K. Nguyen, and G. Murthy, Phys. Rev. B 68, 035332 (2003).
- Wan et al. (2003) X. Wan, E. H. Rezayi, and K. Yang, Phys. Rev. B 68, 125307 (2003).
- Chang (2003) A. M. Chang, Rev. Mod. Phys. 75, 1449 (2003).
- Hu et al. (2008) Z.-X. Hu, H. Chen, K. Yang, E. H. Rezayi, and X. Wan, Phys. Rev. B 78, 235315 (2008).
- Hu et al. (2009) Z.-X. Hu, E. H. Rezayi, X. Wan, and K. Yang, Phys. Rev. B 80, 235330 (2009).
- Wang et al. (2013a) J. Wang, Y. Meir, and Y. Gefen, Phys. Rev. Lett. 111, 246803 (2013a).
- Repellin et al. (2018) C. Repellin, A. M. Cook, T. Neupert, and N. Regnault, npj Quantum Materials 3, 14 (2018).
- Fern et al. (2018) R. Fern, R. Bondesan, and S. H. Simon, Phys. Rev. B 98, 155321 (2018).
- Wei et al. (2020) L.-X. Wei, N. Jiang, Q. Li, and Z.-X. Hu, Phys. Rev. B 101, 075137 (2020).
- Ito and Shibata (2021) T. Ito and N. Shibata, Phys. Rev. B 103, 115107 (2021).
- Khanna et al. (2021) U. Khanna, M. Goldstein, and Y. Gefen, Phys. Rev. B 103, L121302 (2021).
- Thouless (1983) D. J. Thouless, Phys. Rev. B 27, 6083 (1983).
- Wang et al. (2013b) L. Wang, M. Troyer, and X. Dai, Phys. Rev. Lett. 111, 026802 (2013b).
- Nakajima et al. (2016) S. Nakajima, T. Tomita, S. Taie, T. Ichinose, H. Ozawa, L. Wang, M. Troyer, and Y. Takahashi, Nature Physics 12, 296 (2016).
- Lohse et al. (2016) M. Lohse, C. Schweizer, O. Zilberberg, M. Aidelsburger, and I. Bloch, Nature Physics 12, 350 (2016).
- Guo et al. (2012) H. Guo, S.-Q. Shen, and S. Feng, Phys. Rev. B 86, 085124 (2012).
- Xu et al. (2013) Z. Xu, L. Li, and S. Chen, Phys. Rev. Lett. 110, 215301 (2013).
- Zeng et al. (2015) T.-S. Zeng, C. Wang, and H. Zhai, Phys. Rev. Lett. 115, 095302 (2015).
- Hu et al. (2016) H. Hu, H. Guo, and S. Chen, Phys. Rev. B 93, 155133 (2016).
- Zeng et al. (2016) T.-S. Zeng, W. Zhu, and D. N. Sheng, Phys. Rev. B 94, 235139 (2016).
- Nakagawa and Furukawa (2017) M. Nakagawa and S. Furukawa, Phys. Rev. B 95, 165116 (2017).
- Taddia et al. (2017) L. Taddia, E. Cornfeld, D. Rossini, L. Mazza, E. Sela, and R. Fazio, Phys. Rev. Lett. 118, 230402 (2017).
- Nakagawa et al. (2018) M. Nakagawa, T. Yoshida, R. Peters, and N. Kawakami, Phys. Rev. B 98, 115147 (2018).
- (80) See Supplemental Material at http://…., for details of the relation between the pumped charge and the current operator, and a finite size scaling analysis, which includes Ref. Hatsugai, 2004.
- Kuno and Hatsugai (2021) Y. Kuno and Y. Hatsugai, Phys. Rev. B 104, 045113 (2021).
- Thouless (1989) D. J. Thouless, Phys. Rev. B 40, 12034 (1989).
- Grushin et al. (2015) A. G. Grushin, J. Motruk, M. P. Zaletel, and F. Pollmann, Phys. Rev. B 91, 035136 (2015).
- Andrews et al. (2021) B. Andrews, M. Mohan, and T. Neupert, Phys. Rev. B 103, 075132 (2021).
- Hofstadter (1976) D. R. Hofstadter, Phys. Rev. B 14, 2239 (1976).
- Hatsugai et al. (1999) Y. Hatsugai, K. Ishibashi, and Y. Morita, Phys. Rev. Lett. 83, 2246 (1999).
- Wen et al. (1990) X. G. Wen, E. Dagotto, and E. Fradkin, Phys. Rev. B 42, 6110 (1990).
- Hatsugai et al. (1991) Y. Hatsugai, M. Kohmoto, and Y.-S. Wu, Phys. Rev. B 43, 10761 (1991).
- (89) The Hamiltonian is also expressed by using the creation-annihilation operators of hard-core bosons with the statistical flux. In the numerical calculations, we diagonalize it because of its technical simplicity.
- Einarsson (1990) T. Einarsson, Phys. Rev. Lett. 64, 1995 (1990).
- (91) A global requirement of anyons on a torus Einarsson (1990); Wen et al. (1990) is , where and are global move operators of particle along noncontractible loops on the torus in and directions, and is a local move operator of particle around particle .
- (92) The many-body Chern number that is invariant for the adiabatic heuristic principle is given by the following twisted boundary conditions Kudo and Hatsugai (2020): when an anyon hops across the boundary in () direction, the phase factor () is given to the basis . According to this rule in direction, we define the local fluxes only for .
- (93) For fermions, we change keeping constant. Such a change for anyons is prohibited by constraints of the braid group Einarsson (1990). Thus we change their in such a way that the total flux is same as that in the case of fermions.
- (94) Because of the mid-gap region, and at have edge modes. It is suggested that they are localized at the opposite edges each other. Either one is expected due to finite size effects.
- Hatsugai (2004) Y. Hatsugai, Journal of the Physical Society of Japan 73, 2604 (2004).
Supplemental Material
S1 Current operator and the pumped charge
In this appendix, we derive the pumped charge by using the current operator. We consider the Hamiltonian described in the paragraph Fractional anyon pumping in the main text:
| (S1) |
where is the creation operator for a fermion on site , and the phase factors , and describe the uniform fluxes, the local fluxes and the statistical phase , respectively. Using a unitary operator,
let us modify the Hamiltonian in Eq. (S1):
| (S2) |
where is used. We then define the current operator in the direction as
We now assume the following density matrix:
where is the ground state multiplet of the Hamiltonian . The measured current computed from is reduced to the Berry curvature Thouless (1983); Hatsugai and Fukui (2016):
where “tr” indicates the trace of a -dimensional matrix. The gauge transformation in Eq. (S2) implies , i.e.,
where is the center-of-mass defined in Eq. (1). By fixing the gauge properly Thouless (1983); Hatsugai (2004); Hatsugai and Fukui (2016), one can prepare as a well-defined function. Because of , we have , namely,
| (S3) |
The pumped charge is given by the integration of the current over . This clearly justifies the formulation using in the main text.
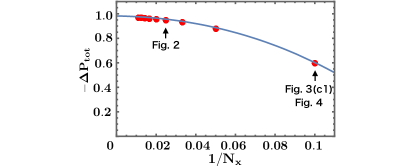
S2 Finite size effect
Let us discuss a finite size effect of numerical simulation. In Fig. S1, we plot the total jump at , where we fix the aspect ratio, the chemical potential, and flux per plaquette as , , and , respectively. As indicated in the figure, the systems in Figs. 2, 3(c1) and 4 are included. As the system size increases, approaches -1. This implies that the edge modes are somewhat spread over in a finite size system, which results in the deviation from at in Figs. 2 and 4.