A geometric interpretation for the Delta Conjecture

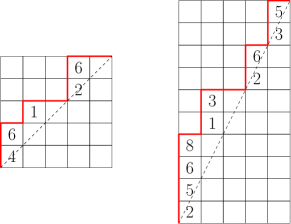
A labeled $(n,n)$ Dyck path or parking function is a Dyck path in the $n\times n$ grid whose vertical steps are labeled with positive integers, such that the labeling increases up each vertical run. See the left-most example in Figure 1. The Shuffle Theorem [CM] gives the following remarkable combinatorial formula for the evaluation $\nabla e_{n}$,

See Section 2 for relevant definitions. Similarly, given $\gcd(a,b)=1$ one can define a $(ka,kb)$ rational parking function as a lattice path (also known as a rational Dyck path) in the $(ka)\times(kb)$ grid that stays weakly above the line $y=ax/b$, starts in the southwest corner, and ends in the northeast corner, together with a column-strictly-increasing labeling of the up steps (see the middle path in Figure 1 for an example where $k=3$, $a=2$ and $b=1$). The combinatorial statistics $\mathrm{area}$ and $\mathrm{dinv}$ in the “combinatorial” right hand side of (1) has a natural generalization to rational parking functions.

However, to generalize the “algebraic” left hand side one needs to consider the Elliptic Hall Algebra $\mathcal{E}_{q,t}$ defined in [EHA] and extensively studied in the last two decades [RationalShuffle, BHMPS, BHMPS2, GN, SV1, SV2, Negut]. This is a remarkable algebra acting on the space $\Lambda(q,t)$ of symmetric functions in infinitely many variables with coefficients in $\mathbb{Q}(q,t)$. The Rational Shuffle Theorem, proposed by Bergeron, Garsia, Leven and Xin [RationalShuffle] (see also [GN] for $k=1$ case and a connection to Hilbert schemes), subsequently proven by Mellit [Mellit], states that

where $E_{ka,kb}$ is a particular element of $\mathcal{E}_{q,t}$. Note that our conventions for $a$ and $b$ are flipped from [RationalShuffle].

We will be primarily interested in the case $(ka,kb)=(K,k)$ where $K=k(n-k+1)$ (so that $a=n-k+1$ and $b=1$) and consider parking functions in the $K\times k$ rectangle. The corresponding symmetric function $E_{K,k}\cdot 1$ has degree $K$.

In a different line of work, Hikita [Hikita] gave an alternative geometric interpretation for $\nabla e_{n}$ in terms of affine Springer fibers. Given a nil-elliptic operator $\gamma\in\mathfrak{sl}_{n}[[\epsilon]]$, one can define the affine Springer fiber $\mathrm{Sp}_{\gamma}$ in the affine flag variety $\widetilde{\mathrm{Fl}}$. The group $S_{n}$ has a Springer-like representation in the (Borel-Moore) homology of $\mathrm{Sp}_{\gamma}$. Affine Springer fibers and their homology have been a subject of very active study in geometric representation theory, see [Yun] and references therein. In particular, $\mathrm{Sp}_{\gamma}$ is closely related to compactified Jacobians and Hilbert schemes of points on plane curve singularities [MY, MS, MaulikShen] and serves as a local model for fibers in the Hitchin integrable system [OY, OY2], while the point count of $\mathrm{Sp}_{\gamma}$ over a finite field is related to orbital integrals in number theory [KivTsai]. The conjectures of Oblomkov, Rasmussen and Shende [ORS] relate the homology of affine Springer fibers to Khovanov-Rozansky link homology.

If $\gamma$ is regular and semisimple, the geometry of $\mathrm{Sp}_{\gamma}$ is controlled by the characteristic polynomial $f_{\gamma}(z,\epsilon)=\det(\gamma-zI)$ and the spectral curve $\{f_{\gamma}(z,\epsilon)=0\}$. Hikita considered in [Hikita] the case when

and proved that $\mathrm{Sp}_{\gamma}$ admits an affine paving with cells in bijection with $(n,n)$ parking functions and the (Frobenius) character of the Borel-Moore homology of $\mathrm{Sp}_{\gamma}$ (as a bigraded $\mathbb{Q}S_{n}$-module) matches the right hand side of (1) (up to a minor twist). In particular, the dimension of the cells is closely related to the $\mathrm{dinv}$ statistics on parking functions. In [CO] Carlsson and Oblomkov used this work to construct a long-sought explicit basis in the space of diagonal coinvariants.

In [GMV] the second author, Mazin and Vazirani generalized the results of [Hikita] to $(a,b)$-parking functions with $\gcd(a,b)=1$ (and $k=1$ in the above notations): for

the affine Springer fiber also admits an affine paving with cells in bijection with $(a,b)$ parking functions. In particular, [GMV] clarified the relation between $(a,b)$ parking functions and a certain subset of (extended) affine permutations in $\widetilde{S_{a}}$.

In [GMO] the second author, Mazin and Oblomkov made progress towards the general non-coprime case $(ka,kb)$ by considering a more complicated class of “generic” spectral curves $\{f_{\gamma}(z,\epsilon)\}$. They proved that for such $\gamma$ the affine Springer fiber $\mathrm{Gr}_{\gamma}$ in the affine Grassmannian admits an affine paving with cells labeled by $(ka,kb)$-Dyck paths. It is plausible that the affine Springer fiber $\mathrm{Sp}_{\gamma}$ also admits an affine paving with cells labeled by $(ka,kb)$ parking functions, but we do not need it here.

Instead, we propose a different geometric model for a special subclass of the non-coprime case. Let $K=k(n-k+1)$, we consider a family of nil-elliptic elements $\gamma_{n,k,N}\in\mathfrak{sl}_{K}[[\epsilon]]$ with characteristic polynomials $z^{K}-\epsilon^{k+N}$.

Here we extend the basis periodically by $e_{i+K}=\epsilon e_{i}$. We also define a certain union $C$ of affine Schubert cells and the subvariety

We can now state our first main result:

It would be interesting to find the analogues of $X_{n,k}$ for more the more general family of symmetric functions $E_{km,kn}\cdot 1$ where $m$ and $n$ are coprime.

A second generalization of the Shuffle Theorem called the Delta Conjecture was formulated by Haglund, Remmel, and Wilson [HRW]. It involves a more general Macdonald eigenoperator $\Delta^{\prime}_{e_{k-1}}$ and relates it to stacked parking functions. See the image on the right of Figure 4 for an example of a $(n,k)$ stacked parking function for $n=6$ and $k=3$. The (Rise) Delta Conjecture (reformulated here in terms of stacked parking functions) states

This version of the Delta Conjecture was proven by [DAdderio] and independently by [BHMPS]. To the authors’ knowledge, the Valley version (involving a statistic $\mathrm{wdinv}$) of the conjecture remains open.

In [GGG1], we proved a formula that directly relates the Rational Shuffle Theorem to the Delta Conjecture.

The proof uses the relation between the Elliptic Hall Algebra and the Shuffle algebra and certain identities for $E_{K,k}$ studied in [BHMPS, BHMPS2, Negut].

Our main result is a geometric version of (2) by constructing a family of subvarieties $Y_{n,k,N}$ in the partial affine flag variety:

where $\leq$ above is dominance order on partitions. Equivalently, the Jordan type condition above may be written $\gamma^{n-k}\Lambda_{0}\subseteq\Lambda_{K-n}$. See Definition LABEL:def:Varieties for more details, here we identify the affine partial flag variety with the space of flags of lattices, and $\mathrm{JT}(\gamma|_{\Lambda_{0}/\Lambda_{K-n}})$ refers to the Jordan type of the induced action of $\gamma=\gamma_{n,k,N}$ on the quotient $\Lambda_{0}/\Lambda_{K-n}$.

In fact, part (b) follows from Theorem 1.5 and part (a). Part (a) is proved similarly to the main result of [GG], see below.

Lastly, we use Theorem 1.5 and the Rational Shuffle Theorem to give a new combinatorial proof of the (Rise) Delta Conjecture.

In a previous work, the first and third authors [GG] studied a family of varieties called $\Delta$-Springer fibers (see also [Griffin, Griffin2, GLW]). We use an equivalent definition following [GG, Lemma 3.3].

One of the main results of [GG] gives a representation-theoretic description for the cohomology of $\Delta$-Springer fiber.

We can relate the variety $Y_{n,k}$ to the $\Delta$-Springer fibers as follows: consider the projection to the affine Grassmannian

which sends a flag of lattices $\Lambda_{\bullet}$ to $\Lambda_{0}$.

To prove Theorem 1.10, we consider the $K$-dimensional space $\Lambda_{0}/\epsilon\Lambda_{0}$, and the partial flag $F_{i}=\Lambda_{i}/\epsilon\Lambda_{0}$. The operator $\overline{\gamma}$ is given by the restriction of $\gamma_{n,k,N}$ to $\Lambda_{0}/\epsilon\Lambda_{0}$, and the key Lemma LABEL:lem:eval-in-sub shows that it has Jordan type $\nu=(n-k)^{k}+\mu$ for some $\mu$. Now the conditions defining $Y_{n,k,N}$ translate to the conditions for $Z_{n,\nu,k}$, see Section LABEL:sec:_geometry for details.

The proof of Theorem 1.9 heavily used the work of Borho and MacPherson [BM] on partial resolutions of the nilpotent cone. Note that by [HottaSpringer] the modified Hall-Littlewood polynomial in the Theorem 1.9 can be interpreted as the graded Frobenius character of the homology of the classical Springer fiber, which coincides with the fiber of the projection $X_{n,k,N}\to\widetilde{\mathrm{Gr}}$.

We use this idea to develop a sheaf-theoretic generalization of Theorem 1.9, and use it to prove Theorem 1.5(a).

The paper is organized as follows. In Section 2, we give a combinatorial background on symmetric functions, parking functions and affine permutations. In Section 3, we translate parking function combinatorics and diagonal inversions in terms of a class of affine permutations called $\gamma$-restricted, and we prove several useful lemmas concerning them. Sections LABEL:sec:_geometry, LABEL:sec:_Springer_action, and LABEL:sec:_X_dimensions are focused on the geometry of affine Springer fibers. In Section LABEL:sec:_geometry we introduce the varieties $X_{n,k,N}$ and $Y_{n,k,N}$ and prove Theorem 1.6(a). In Section LABEL:sec:_Springer_action, we use Springer theory and work of Borho and MacPherson to show there are compatible symmetric group actions on the Borel-Moore homologies of $X_{n,k,N}$ and $Y_{n,k,N}$. We then prove Theorem 1.6(a), a geometric version of the skewing formula. In Section LABEL:sec:_X_dimensions, we construct an affine paving of $X_{n,k}$. This allows us to prove Theorem 1.3 and complete the proof of Theorem 1.6(b).

We conclude the introduction with a few possible further directions.

In particular, our varieties depend on a parameter $N$. However, we show in Theorems 1.3 and  1.6 that when $N\geq k$ these varieties are independent of $N$ and the graded Frobenius character of their Borel-Moore homologies give the symmetric functions in the Rational Shuffle Theorem and Delta Theorem. However, when $0\leq N<k$, there exist examples of $X_{n,k,N}$ (respectively $Y_{n,k,N}$) whose Borel-Moore homology groups differ from the terms in the Rational Shuffle Theorem (respectively Delta Theorem). Therefore, one could define new symmetric functions $f_{n,k,N}$ and $g_{n,k,N}$ by

Another natural question is whether our varieties can be generalized to give geometric interpretations of other symmetric functions coming from Macdonald-theoretic or Elliptic Hall Algebra operators.

We refer to [Macdonald] for details on many of the standard definitions in this section. We will work in the ring of symmetric functions $\Lambda$ in infinitely many variables $x_{1},x_{2},\ldots$ over $\mathbb{Q}(q,t)$. We will use elementary symmetric functions

where $\lambda$ is a partition. We also have monomial symmetric functions

where $(i_{1},\ldots,i_{\ell})$ is any tuple of distinct positive integers such that $i_{j}<i_{j+1}$ whenever $\lambda_{j}=\lambda_{j+1}$. Both $\{m_{\lambda}\}$ and $\{e_{\lambda}\}$ form a basis of $\Lambda$.

We also use the basis of Schur functions $s_{\lambda}$, defined as $s_{\lambda}=\sum K_{\lambda\mu}m_{\mu}$ where the coefficients $K_{\lambda\mu}$ are the Kostka numbers, which count the number of column-strict Young tableaux of shape $\lambda$ and content $\mu$. We draw our Young tableaux in French notation:

and the above tableau has content $(2,1,0,1,3)$, with the $i$th entry indicating the multiplicity of $i$ in the tableau. Its shape is $(4,2,1)$, indicating the length of each row from bottom to top.

The Schur functions and Hall inner product are directly tied to the representation theory of the symmetric group $S_{n}$. The irreducible representations $V_{\lambda}$ of $S_{n}$ are indexed by partitions $\lambda$ of $n$, and the Frobenius map $\mathrm{Frob}$ takes a representation $V=\bigoplus_{\lambda}(V_{\lambda})^{\oplus c_{\lambda}}$ to $\sum c_{\lambda}s_{\lambda}$. Clearly $\mathrm{Frob}$ is additive across direct sum, and it has the remarkable property of being multiplicative across induced tensor product:

We make use of a (doubly) graded version of the Frobenius map as follows.

We use the adjoint operators to multiplication operators with respect to the Hall inner product.

We will make use of the following lemma from [GG].

Throughout this subsection, let $k$ and $K$ be positive integers such that $k|K$. Let $\mathrm{RD}_{K,k}$ be the set of $K\times k$ rational Dyck paths (height $K$ and width $k$), and let $\mathrm{PF}_{K,k}$ be the labeled parking functions on elements of $\mathrm{RD}_{K,k}$. That is, the set of labelings of the vertical runs of elements of $\mathrm{RD}_{K,k}$ by positive integers such that the labeling weakly increases up each vertical run.

To define $\mathrm{pathdinv}(D)$, recall that the arm of a box above a Dyck path in the $K\times k$ grid is the number of boxes to its right that still lie above the Dyck path. The leg is the number of boxes below it that still lie above the Dyck path.

To define the $\mathrm{tdinv}$ statistic on rational parking functions, we use the same conditions on pairs of boxes identified in Lemma 2.14, which we call attacking pairs.

Note that $\mathrm{maxtdinv}(D)$ can be achieved by taking $\mathrm{tdinv}(D)$ of the labeling of $D$ obtained by labeling using $1,2,3,\ldots$ across diagonals from left to right, starting from the bottom-most diagonal and moving upwards. Alternatively, $\mathrm{maxtdinv}(D)$ is the number of attacking pairs $(a,b)$ such that $a$ and $b$ are boxes directly to the right of an up-step of $D$.

Define the rank of the box in row $j$ and column $i$ of the $K\times k$ rectangle to be

In other words, we put $1$ in the box in the SW corner, and fill the rest of the ranks by increasing them by $k$ or $k+1$, respectively, as we go North from $(i,j)$ to $(i,j+1)$ if $j$ is not divisible or divisible by $n-k+1$, respectively. As we go East from $(i,j)$ to $(i+1,j)$, we decrease by $K$. The ranks of the boxes in the case of $K=12$ and $k=4$ is shown in Figure 2.

The following is easily verified and we omit the proof.

We will need the following statement relating ranks to attacking pairs.

We now show a correspondence between certain attacking pairs and the complement squares to those that contribute to pathdinv. To do so, we first rephrase the definition of pathdinv in a way that we used in [GGG1]. The proof simply follows from analyzing both sides of the inequality in Definition 2.7, and we omit it.

We use the notation of [GGG1, HRW] here, and recall the definition of a stacked parking function that can be used to reformulate the Rise version of the Delta conjecture.

We write $\mathcal{LD}(S)$ is the set of stacked parking functions with respect to $S$, and

We will also need the following construction from [GGG1] relating rational parking functions with the stacked ones. Set $K=k(n-k+1)$. Given a standard parking function in the $K\times k$ rectangle, we call a label $a$ big if $a>n$, and small if $a\leq n$. We denote by $b_{i}$ the number of big labels in the $i$-th column, and by $s_{i}$ the number of small labels. Note that

We construct a map $F$ from the set of standard admissible $(K,k)$ parking functions to the set $\mathcal{LD}_{n,k}^{\mathrm{stack}}$ as follows:

• •

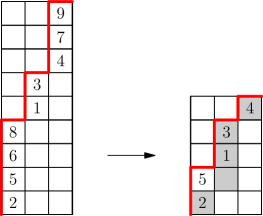
  The parking function $F(\pi)$ is obtained by erasing all big labels in $\pi$ and the north steps to the left of them.

• •

  In particular, the length of the $i$th vertical run of the lattice path for $F(\pi)$ is equal to $s_{i}$.

• •

  The height of the stack in column $i$ is given by $h_{i}=n-k+1-b_{i}$.

See Figure 4 for an example.

One can define the analogues of $\mathrm{area}$ and $\mathrm{dinv}$ statistics for stacked parking functions. These appear in the Delta Theorem, but we do not need them in this paper and refer to [GGG1, Section 4.1] for all definitions and a detailed discussion.

We now establish notation on affine permutations.

In order for $\omega$ to be well defined, we need $\omega(1),\dots,\omega(K)$ to have pairwise distinct remainders modulo $K$. By reducing $\omega(i)$ modulo $K$, we get a projection $\widetilde{S_{K}}\to S_{K}$.

One can check that $\deg\omega$ is always an integer and $\deg(\omega_{1}\omega_{2})=\deg\omega_{1}+\deg\omega_{2}.$

We have $\deg(\mathbf{t}_{\lambda})=|\lambda|=\sum_{i=1}^{K}\lambda_{i}.$ Furthermore, any affine permutation can be uniquely written as

We will need a special affine permutation $\gamma=\gamma_{n,k,N}\in\widetilde{S}_{K}$ defined as follows: